Skip to main content
\(\require{cancel}\)
Physics LibreTexts

5.4.10: Bubble Inside a Uniform Solid Sphere

  1. Last updated
  2. Save as PDF
  • Page ID
    8140

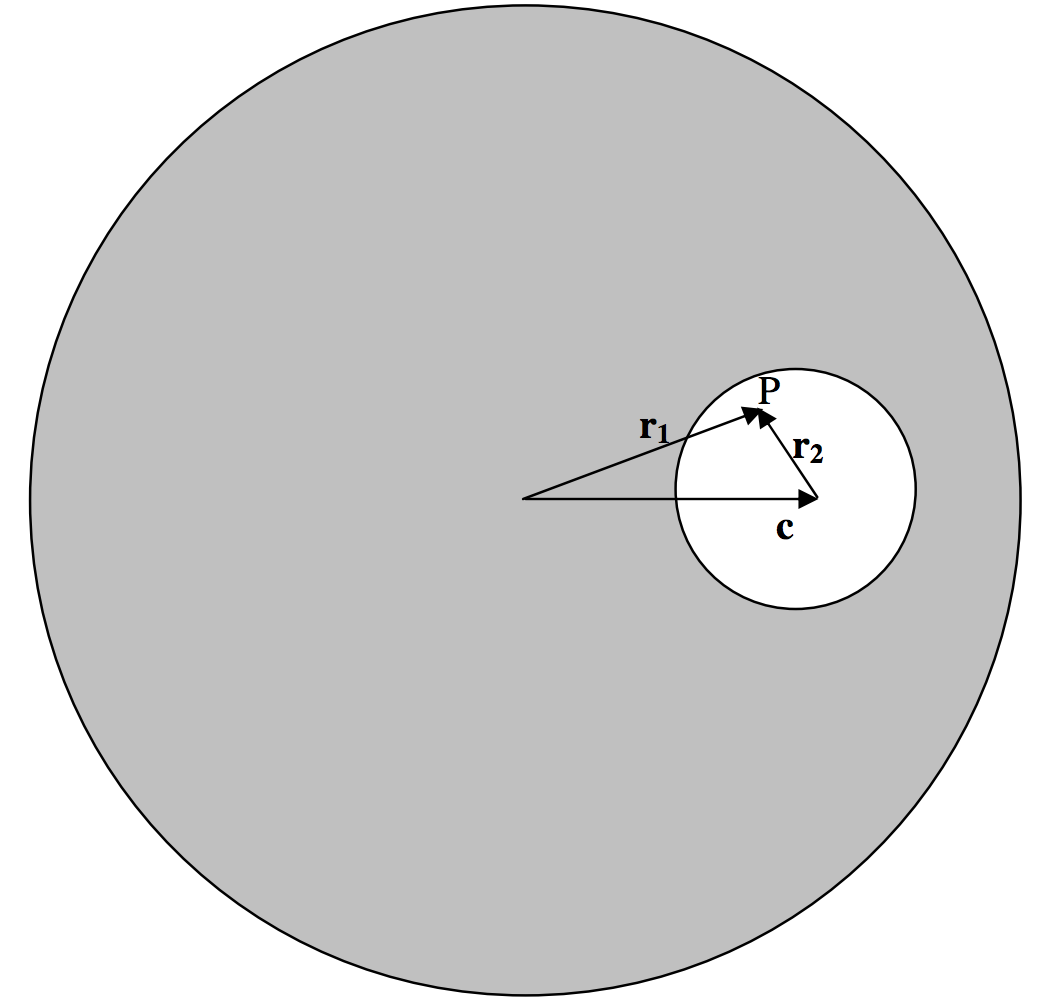
    • Figure 5.11.png
    \(\text{FIGURE V.11}\)

    \(\text{P}\) is a point inside the bubble. The field at \(\text{P}\) is equal to the field due to the entire sphere minus the field due to the missing mass of the bubble. That is, it is

    \[\textbf{g} = -\frac{4}{3} \pi G ρ \textbf{r}_1 - (-\frac{4}{3} \pi G ρ \textbf{r}_2) = -\frac{4}{3} \pi G ρ ( \textbf{r}_1 - \textbf{r}_2) = -\frac{4}{3} \pi G ρ \textbf{c}. \label{5.4.26} \tag{5.4.26}\]

    That is, the field at \(\text{P}\) is uniform (i.e. is independent of the position of \(\text{P}\)) and is parallel to the line joining the centres of the two spheres.