4.4: The Paraboloid

Last updated
Save as PDF

Page ID: 6808

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

The Equation \(x^2 = 4qz = 2lz\) is a parabola in the \(xz\)-plane. The distance between vertex and focus is \(q\), and the length of the semi latus rectum \(l = 2q\). The Equation can also be written

\[\frac{x^2}{a^2} = \frac{z}{h} \label{4.4.1} \tag{4.4.1}\]

Here \(a\) and \(h\) are distances such that \(x = a\) when \(z = h\), and the length of the semi latus rectum is \(l = a^2 /(2h)\).

If this parabola is rotated through \(360^\circ\) about the \(z\)-axis, the figure swept out is a paraboloid of revolution, or circular paraboloid. Many telescope mirrors are of this shape. The Equation to the circular paraboloid is

\[\frac{x^2}{a^2} + \frac{y^2}{a^2} = \frac{z}{h}. \label{4.4.2} \tag{4.4.2}\]

The cross-section at \(z = h\) is a circle of radius \(a\).

The Equation \[\frac{x^2}{a^2} + \frac{y^2}{b^2} = \frac{z}{h} , \label{4.4.3} \tag{4.4.3}\]

in which we shall choose the \(x\)- and \(y\)-axes such that \(a > b\), is an elliptic paraboloid and, if \(a ≠ b\), is not formed by rotation of a parabola. At \(z = h\), the cross section is an ellipse of semi major and minor axes equal to \(a\) and \(b\) respectively. The section in the plane \(y = 0\) is a parabola of semi latus rectum \(a^2 /(2h)\). The section in the plane \(x = 0\) is a parabola of semi latus rectum \(b^2 /(2h)\). The elliptic paraboloid lies entirely above the \(xy\)-plane.

The Equation

\[\frac{x^2}{a^2} - \frac{y^2}{b^2} = \frac{z}{h} \label{4.4.4} \tag{4.4.4}\]

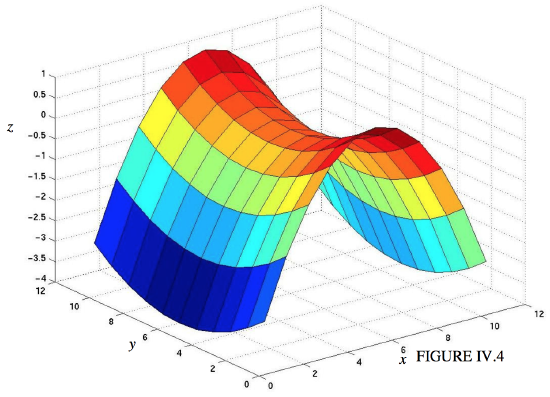
is a hyperbolic paraboloid, and its shape is not quite so easily visualized. Unlike the elliptic paraboloid, it extends above and below the plane. It is a saddle-shaped surface, with the saddle point at the origin. The section in the plane \(y = 0\) is the "nose down" parabola \(x^2 = a^2 z / h\) extending above the xy-plane. The section in the plane \(x = 0\) is the "nose up" parabola \(y^2 = -b^2 z /h \) extending below the \(xy\)-plane. The section in the plane \(z = h\) is the hyperbola

\[\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1. \label{4.4.5} \tag{4.4.5}\]

The section with the plane \(z = −h\) is the conjugate hyperbola

\[\frac{x^2}{a^2} - \frac{y^2}{b^2} = -1 . \label{4.4.6} \tag{4.4.6}\]

The section with the plane \(z = 0\) is the asymptotes

\[\frac{x^2}{a^2} - \frac{y^2}{b^2} = 0 . \label{4.4.7} \tag{4.4.7}\]

The surface for \(a = 3\), \(b = 2\), \(h = 1\) is drawn in figure \(\text{IV.4}\).

Figure 4.4.1.png