9.4: Appendix 9A The Gravitational Field of a Spherical Shell of Matter .

When analyzing gravitational interactions between uniform spherical bodies we assumed we could treat each sphere as a point-like mass located at the center of the sphere and then use the Universal Law of Gravitation to determine the force between the two point like objects. We shall now justify that assumption. For simplicity we only need to consider the interaction between a spherical object and a point-like mass. We would like to determine the gravitational force on the point-like object of mass \(m_{1}\) due to the gravitational interaction with a solid uniform sphere of mass \(m_{2}\) and radius R . In order to determine the force law we shall first consider the interaction between the point-like object and a uniform spherical shell of mass \(m_{s}\) and radius R . We will show that:

1) The gravitational force acting on a point-like object of mass \(m_{1}\) located a distance r > R from the center of a uniform spherical shell of mass \(m_{s}\) and radius R is the same force that would arise if all the mass of the shell were placed at the center of the shell.

2) The gravitational force on an object of mass \(m_{1}\) placed inside a spherical shell of matter is zero.

The force law summarizes these results:

\[\overrightarrow{\mathbf{F}}_{s, 1}(r)=\left\{\begin{array}{cc}
-G \frac{m_{s} m_{1}}{r^{2}} \hat{\mathbf{r}}, & r>R \\
\overrightarrow{\mathbf{0}}, & r<R
\end{array}\right. \nonumber \]

where \(\hat{\mathbf{r}}\) is the unit vector located at the position of the object and pointing radially away from the center of the shell.

For a uniform spherical distribution of matter, we can divide the sphere into thin shells. Then the force between the point-like object and each shell is the same as if all the mass of the shell were placed at the center of the shell. Then we add up all the contributions of the shells (integration), the spherical distribution can be treated as point-like object located at the center of the sphere justifying our assumption.

Thus it suffices to analyze the case of the spherical shell. We shall first divide the shell into small area elements and calculate the gravitational force on the point-like object due to one element of the shell and then add the forces due to all these elements via integration.

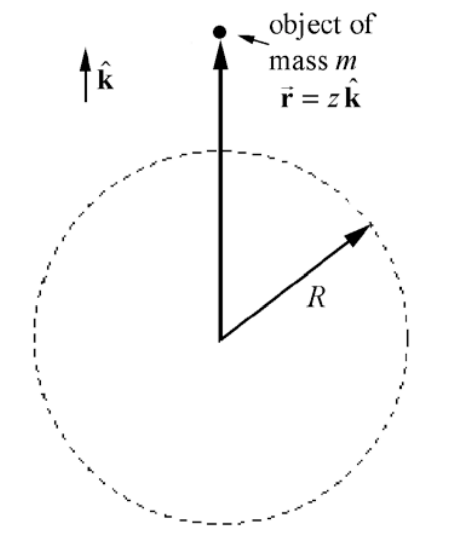
We begin by choosing a coordinate system. Choose our z -axis to be directed from the center of the sphere to the position of the object, at position \(\overrightarrow{\mathbf{r}}=z \hat{\mathbf{k}}\), so that \(z \geq 0\) (Figure 9A.1 shows the object lying outside the shell with \(z>R\))

Figure 9A.1 Object lying outside shell with \(z>R\)

Choose spherical coordinates as shown in Figure 9A.2.

Figure 9A.2 Spherical coordinates

For a point on the surface of a sphere of radius r = R , the Cartesian coordinates are related to the spherical coordinates by

\[\begin{array}{l}
x=R \sin \theta \cos \phi \\
y=R \sin \theta \sin \phi \\
z=R \cos \theta
\end{array} \nonumber \]

where \(0 \leq \theta \leq \pi\) and \(0 \leq \phi \leq 2 \pi\).

Note that the angle θ in Figure 9A.2 and Equations (9.A.1) is not the same as that in plane polar coordinates or cylindrical coordinates. The angle θ is known as the colatitude, the complement of the latitude. We now choose a small area element shown in Figure 9A.3.

Figure 9A.3 Infinitesimal area element

The infinitesimal area element on the surface of the shell is given by

\[d a=R^{2} \sin \theta d \theta d \phi \nonumber \]

Then the mass dm contained in that element is

\[d m=\sigma d a=\sigma R^{2} \sin \theta d \theta d \phi \nonumber \]

where \(\sigma\) is the surface mass density given by

\[\sigma=m_{s} / 4 \pi R^{2} \nonumber \]

The gravitational force \(\overrightarrow{\mathbf{F}}_{d m, m_{1}}\) on the object of mass \(m_{1}\) that lies outside the shell due to the infinitesimal piece of the shell (with mass dm ) is shown in Figure 9A.4.

Figure 9A.4 Force on a point-like object due to piece of shell

The contribution from the piece with mass dm to the gravitational force on the object of mass \(m_{1}\) that lies outside the shell has a component pointing in the negative \(\hat{\mathbf{k}}\)-direction and a component pointing radially away from the z -axis. By symmetry there is another mass element with the same differential mass \(d m^{\prime}=d m\) on the other side of the shell with same co-latitude θ but with \(\phi\) replaced by \(\phi \pm \pi\); this replacement changes the sign of x and y in Equations (9.A.1) but leaves z unchanged. This other mass element produces a gravitational force that exactly cancels the radial component of the force pointing away from the z -axis. Therefore the sum of the forces of these differential mass elements on the object has only a component in the negative \(\hat{\mathbf{k}}\)-direction (Figure 9A.5).

Figure 9A.5 Symmetric cancellation of components of force

Therefore we need only the z -component vector of the force due to the piece of the shell on the point-like object.

Figure 9A.6 Geometry for calculating the force due to piece of shell.

From the geometry of the set-up (Figure 9A.6) we see that

\[\left(d \overrightarrow{\mathbf{F}}_{s, 1}\right)_{z} \equiv d F \hat{\mathbf{k}}=-G \frac{m_{1} d m}{r_{s 1}^{2}} \cos \alpha \hat{\mathbf{k}} \nonumber \]

Thus

\[d F_{z}=-G \frac{m_{1} d m}{r_{s 1}^{2}} \cos \alpha=-\frac{G m_{s} m_{1}}{4 \pi} \frac{\cos \alpha \sin \theta d \theta d \phi}{r_{s 1}^{2}} \nonumber \]

The integral of the force over the surface is then

\[F_{z}=-G m_{1} \int_{\theta=0}^{\theta=\pi \phi=2 \pi} \int_{\phi=0} \frac{d m \cos \alpha}{r_{s 1}^{2}}=-\frac{G m_{s} m_{1}^{\theta=\pi \phi=2 \pi}}{4 \pi} \int_{\theta=0}^{\infty} \int_{\phi=0} \frac{\cos \alpha \sin \theta d \theta d \phi}{r_{s 1}^{2}} \nonumber \]

The \(\phi\)-integral is straightforward yielding

\[F_{z}=-\frac{G m_{s} m_{1}^{\theta=\pi}}{2} \int_{\theta=0}^{\cos \alpha \sin \theta d \theta} \nonumber \]

From Figure 9A.6 we can use the law of cosines in two different ways

\[\begin{array}{l}
r_{s 1}^{2}=R^{2}+z^{2}-2 R z \cos \theta \\
R^{2}=z^{2}+r_{s 1}^{2}-2 r_{s, 1} z \cos \alpha
\end{array} \nonumber \]

Differentiating the first expression in (9.A.5), with R and z constant yields,

\[2 r_{s, 1} d r_{s, 1}=2 R z \sin \theta d \theta \nonumber \]

Hence

\[\sin \theta d \theta=\frac{r_{s, 1}}{R z} d r_{s, 1} \nonumber \]

and from the second expression in (9.A.5) we have that

\[\cos \alpha=\frac{1}{2 z r_{s, 1}}\left[\left(z^{2}-R^{2}\right)+r_{s 1}^{2}\right] \nonumber \]

We now have everything we need in terms of \(r_{s, 1}\).

For the case when \(z>R, r_{s, 1}\) varies from z − R to z + R. Substituting Equations (9.A.7) and (9.A.8) into Equation (9.A.3) and using the limits for the definite integral yields

\[\begin{aligned}
F_{z} &=-\frac{G m_{s} m_{1}^{\theta=\pi}}{2} \int_{\theta=0}^{\cos \alpha \sin \theta} d \theta \\
&=-\frac{G m_{s} m_{1}}{2} \frac{1}{2 z} \int_{z-R}^{z+R} \frac{1}{r_{s, 1}}\left[\left(z^{2}-R^{2}\right)+r_{s, 1}^{2}\right] \frac{1}{r_{s, 1}^{2}} \frac{r_{s, 1} d r_{s, 1}}{R z} \\
&=-\frac{G m_{s} m_{1}}{2} \frac{1}{2 R z^{2}}\left[\left(z^{2}-R^{2}\right) \int_{z-R}^{z+R} \frac{d r_{s, 1}}{r_{s, 1}^{2}}+\int_{z-R}^{z+R} d r_{s, 1}\right]
\end{aligned} \nonumber \]

No tables should be needed for these; the result is

\[\begin{aligned}
F_{z} &=-\frac{G m_{s} m_{1}}{2} \frac{1}{2 R z^{2}}\left[-\frac{\left(z^{2}-R^{2}\right)}{r_{s, 1}}+r_{s, 1}\right]_{z-R}^{z+R} \\
&=-\frac{G m_{s} m_{1}}{2} \frac{1}{2 R z^{2}}[-(z-R)+(z+R)+2 R] \\
&=-\frac{G m_{s} m_{1}}{z^{2}}
\end{aligned} \nonumber \]

For the case when \(z<R, r_{s, 1}\) varies from R − z to R + z . Then the integral is

\[\begin{aligned}
F_{z} &=-\frac{G m_{s} m_{1}}{2} \frac{1}{2 R z^{2}}\left[-\frac{\left(z^{2}-R^{2}\right)}{r_{s, 1}}+r_{s, 1}\right]_{R-z}^{R+z} \\
&=-\frac{G m_{s} m_{1}}{2} \frac{1}{2 R z^{2}}[-(z-R)-(z+R)+2 z] \\
&=0
\end{aligned} \nonumber \]

So we have demonstrated the proposition that for a point-like object located on the z - axis a distance z from the center of a spherical shell, the gravitational force on the point like object is given by

\[\overrightarrow{\mathbf{F}}_{s, 1}(r)=\left\{\begin{aligned}
-G \frac{m_{s} m_{1}}{z^{2}} \hat{\mathbf{k}}, & z>R \\
\overrightarrow{\mathbf{0}}, & z<R
\end{aligned}\right. \nonumber \]

This proves the result that the gravitational force inside the shell is zero and the gravitational force outside the shell is equivalent to putting all the mass at the center of the shell.