6.8: Field on the Axis of a Long Solenoid
- Page ID
- 7798
The solenoid, of radius \(a\), is wound with \(n\) turns per unit length of a wire carrying a current in the direction indicated by the symbols \(\bigotimes\) and \(\bigodot\).
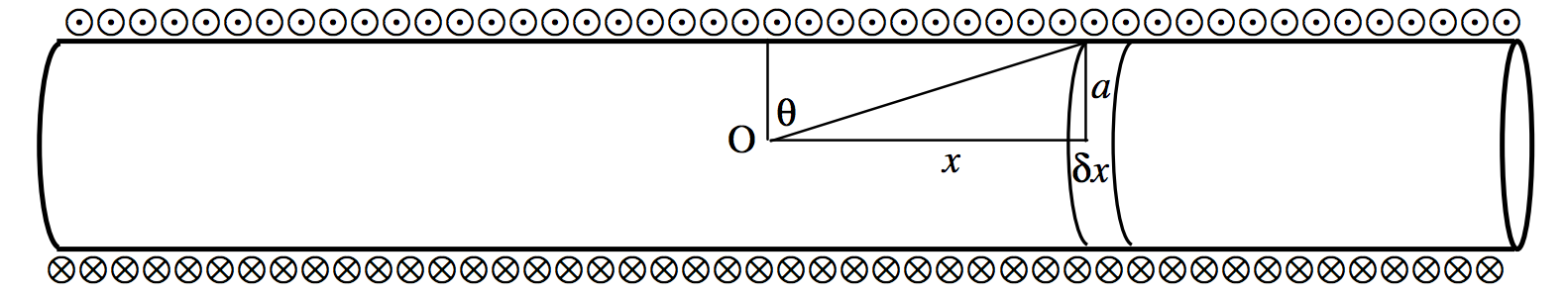
\(\text{FIGURE VI.8}\)
At a point O on the axis of the solenoid the contribution to the magnetic field arising from an elemental ring of width \(\delta x\) (hence having \(n\, δx\) turns) at a distance \(x\) from O is
\[\delta B = \frac{\mu n \,\delta x\, I a^2}{2(a^2+x^2)^{3/2}}=\frac{\mu nI}{2a}\cdot \frac{a^3 \delta x}{(a^2+x^2)^{3/2}}.\label{6.8.1}\]
This field is directed towards the right.
Let us express this in terms of the angle \(θ\).
We have \(x=a \tan \theta ,\, \delta x = a \sec^2 \theta \, \delta \theta ,\text{ and }\frac{a^3}{(a^2+x^2)^{3/2}}=\cos^3 \theta \). Equation \ref{6.8.1} becomes
\[\delta B = \frac{1}{2}\mu nI\cos \theta .\]
If the solenoid is of infinite length, to find the field from the entire infinite solenoid, we integrate from \(θ = \pi/2 \text{ to }0\) and double it. Thus
\[B=\mu nI \int_0^{\pi/2}\cos \theta \,d\theta.\]
Thus the field on the axis of the solenoid is
\[B=\mu n I.\]
This is the field on the axis of the solenoid. What happens if we move away from the axis? Is the field a little greater as we move away from the axis, or is it a little less? Is the field a maximum on the axis, or a minimum? Or does the field go through a maximum, or a minimum, somewhere between the axis and the circumference? We shall answer these questions in section 6.11.


