5.1: Optical Telescopes
- Page ID
- 30877
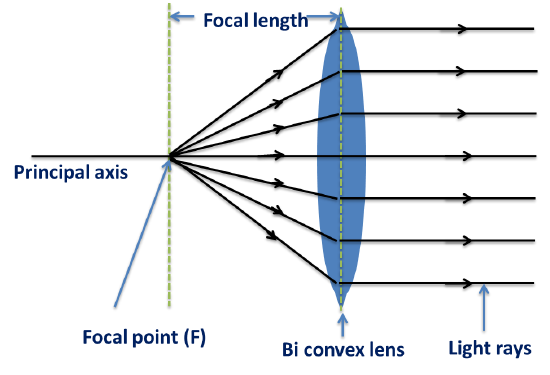 Biconvex Lens used to focus light rays onto a focal point.
Biconvex Lens used to focus light rays onto a focal point.
Since the time of Galileo (Chapter 3), astronomers have used telescopes to examine the planets and stars. While Galileo did not invent the telescope, he made significant improvements in the original design and was the first to use to study the night sky. Galileo used a refracting telescope, one that uses a curved lens to focus light rays to a focal point. Refraction results from the bending of light waves as it travels from one medium to another. So, as light waves travel from air to a curved lens, the waves become bent. The shape of the lens then focuses the light waves by causing the parallel rays to a single point. Also, the Sun appears distorted at sunset as the Sun’s rays pass through a thicker layer of atmosphere, causing additional refraction.
The lens of your eye works the same way. It bends the light entering you eye to focus it onto your retina. The retina is located at the focal plane, the plane where light from different directions comes into focus. Using a single convex lens (a lens that bulges outward), images appear upside on the focal plane. Fortunately, our brain can interpret the signals from the retina to put things right side up. For telescopes, additional lenses or a compound lenses can be used to turn images right side up for many spyglasses, although this is not a serious problem in astronomy, as there is not defined up or down in space.
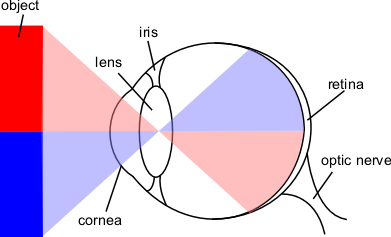 Eye Diagram
Eye Diagram
You may be familiar with the popular image of an astronomer squinting into an eyepiece to look at distant objects. However, modern professional astronomers do not really spend much time looking through eyepieces. They spend most of their time in front of a computer, looking at images or data on a screen. Modern observatories are computerized. Astronomers will book time for observations and enter the coordinates of the object(s) they want to view and the computer then positions the telescope where they want to look. Instead of an eyepiece, the images are focused onto a charged couple device (CCD) that converts the light waves into an electronic signal that computer processes. The CCDs used in telescopes are like those used in digital cameras and operate on the same principle.
 A charged couple device.
A charged couple device.
https:/commons.wikimedia.org/wiki/File:CCD_Sensor_Sony_Video_Camera.jpg;
Isaac Newton invented the other major category of telescope, the reflecting telescope. Newton replaced the primary lens with a curved mirror made of metal. In a Newtonian reflector, light reflects off the mirror and is converged to the focal plane. However, before it reaches the focal plane, it encounters a second mirror at a forty-five-degree angle. This redirects the light into an eyepiece on the side of the telescope. The second mirror does limit some of the light that can reach the primary mirror, but this is compensated with a greater light-gathering power or “light bucket.”
 A refracting (right) and reflecting (left) telescopes.
A refracting (right) and reflecting (left) telescopes.
https:/commons.wikimedia.org/wiki/File:OpenStax_Astronomy_refracting_and_reflecting_telescopes.jpg;
Because many people found the side mounted eyepiece awkward, an alternative to the Newtonian reflector, the Cassegrain reflector. Instead of reflecting the image off to the side, the secondary mirror in a Cassegrain telescope focuses the light back down through a hole in the primary mirror. A third reflecting telescope design, the Nasmyth, using three mirrors to refocus the light and divert out the side to an image processing or coudé room. Some large telescopes may include both a Cassegrain and Nasmyth configuration.
 A Cassegrain Telescope.
A Cassegrain Telescope.
https:/commons.wikimedia.org/wiki/File:Cassegrain-Telescope.jpg;

A Nasmyth Telescope.
All modern large telescopes are reflectors, either Cassegrain, Nasmyth, or a combination. This is because of several limitations of refracting telescopes. One is chromatic aberration, which is caused by the fact that light of different wavelengths is bent at slightly different angles. This is how a prism separates white light into its component colors. Chromatic aberration results in a rainbow-colored halo around certain objects. Another limitation with refractors is spherical aberration, which creates distortions in the shape of the object. Newton’s desire to eliminate chromatic and spherical aberration led to his invention of the Newtonian reflector.
 https://en.wikipedia.org/wiki/Chroma...ns_diagram.svg
https://en.wikipedia.org/wiki/Chroma...ns_diagram.svgHowever, the biggest limitations of refractors result from the glass of the lens itself. Glass absorbs some of the light passing through it, which limits its light bucket. In contrast, reflector focuses nearly all the incident light. A glass lens must have two perfectly polished sides, while a mirror only requires one. Most importantly, however, is the fact that a lens can only be supported around the edges. This causes “lens sag” in which the lens sags under its own weight. This puts an upper limit on how large a lens can be and thus limits its light bucket. A mirror can be supported across its entire back, giving it a much larger light-gathering power. Many major observatories even employ a primary mirror made up of several circular or hexagonal mirrors, enabling an even larger light-gathering power.
The larger the lens or mirror diameter, the greater the light gathering area. The largest optical telescopes today have a mirror with a diameter of about 10 meters, though some can increase its overall light gathering area by employing multiple mirrors of up to 10 meters.
The two important properties of a telescope are light-gathering power (light bucket) and resolving power. Light bucket improves out ability to see distant or faint objects and is proportional to the square of the radius of the primary mirror. Resolving power measures the ability of a telescope to distinguish objects that are close together. Most commonly, it is expressed as angular resolution, which is the minimum angular separation that the telescope can distinguish. The smaller the resolution, the better it can distinguish between close objects. Resolution is proportional to wavelength and inversely proportional to the size of the mirror. Larger telescopes have better resolution because of less interference of the light waves. Too much interference can produce rings around an image of a star. The limit on resolution is also referred to as the telescope’s diffraction limit. Thus, but light-gather power and resolution improve with the size of the telescope.
What may surprise some people is that magnification is the least important property of a telescope. Computer processing can enlarge and sharpen image, reducing the need for the image to be made larger on the telescope’s focal plane.
So, what do astronomers use telescopes for? Obviously, they use them for imaging, takin pictures of objects in the sky. Because the images processed by computers, they are filtered through detectors the record only one color of light at a time. The computer then combines several images to make a full-color images. Astronomical detectors can also record forms of light that is invisible to our eyes, such as infrared. In such images, the computer produces a false-color image where it assigns different colors of visible light to represent invisible wavelength.
Astronomers also use telescopes for spectroscopy (Chapter 4). By breaking light into spectrum, astronomers can identify absorption and emissions lines as well use the peak brightness to determine the temperature of the object. By graphing the relative brightness of light at differing wavelengths, astronomers can study details of the spectrum. Using the Doppler effect, astronomers can measure the movement of an object and its rotational velocity.
Finally, astronomers can also perform time monitoring to measure how the light output of an object can vary with time. By plotting brightness measurements over a period, astronomers can study the behavior of stars and even detect exoplanets (Chapter 16) by looking for dips in brightness or subtle Doppler shifts in its light output.

