19.3: Examples

Problem-Solving Strategy: Static Equilibrium

1. Identify the object to be analyzed. For some systems in equilibrium, it may be necessary to consider more than one object. Identify all forces acting on the object. Identify the questions you need to answer. Identify the information given in the problem. In realistic problems, some key information may be implicit in the situation rather than provided explicitly.
2. Set up a free-body diagram for the object. (a) Choose the xy-reference frame for the problem. Draw a free-body diagram for the object, including only the forces that act on it. When suitable, represent the forces in terms of their components in the chosen reference frame. As you do this for each force, cross out the original force so that you do not erroneously include the same force twice in equations. Label all forces—you will need this for correct computations of net forces in the x- and y-directions. For an unknown force, the direction must be assigned arbitrarily; think of it as a ‘working direction’ or ‘suspected direction.’ The correct direction is determined by the sign that you obtain in the final solution. A plus sign (+) means that the working direction is the actual direction. A minus sign (−) means that the actual direction is opposite to the assumed working direction. (b) Choose the location of the rotation axis; in other words, choose the pivot point with respect to which you will compute torques of acting forces. On the free-body diagram, indicate the location of the pivot and the lever arms of acting forces—you will need this for correct computations of torques. In the selection of the pivot, keep in mind that the pivot can be placed anywhere you wish, but the guiding principle is that the best choice will simplify as much as possible the calculation of the net torque along the rotation axis.
3. Set up the equations of equilibrium for the object. (a) Use the free-body diagram to write a correct equilibrium condition Equation 12.2.9 for force components in the x-direction. (b) Use the free-body diagram to write a correct equilibrium condition Equation 12.2.13 for force components in the y-direction. (c) Use the free-body diagram to write a correct equilibrium condition Equation 12.2.11 for torques along the axis of rotation. Use Equation 12.2.12 to evaluate torque magnitudes and senses.
4. Simplify and solve the system of equations for equilibrium to obtain unknown quantities. At this point, your work involves algebra only. Keep in mind that the number of equations must be the same as the number of unknowns. If the number of unknowns is larger than the number of equations, the problem cannot be solved.
5. Evaluate the expressions for the unknown quantities that you obtained in your solution. Your final answers should have correct numerical values and correct physical units. If they do not, then use the previous steps to track back a mistake to its origin and correct it. Also, you may independently check for your numerical answers by shifting the pivot to a different location and solving the problem again, which is what we did in Example 12.1.

 

Whiteboard Problem \(\PageIndex{1}\): Seesaw

Consider the see-saw in the above figure - two masses attached to a massless board, balanced on a point between them.

1. If \(d_1\) = 37.5 cm, \(d_2\) = 113 cm, and \(m_1\) = 15 kg,  what should \(m_2\) be so that this board is balanced?
2. How much force is the balance point acting on the board with?

 

 

Whiteboard Problem \(\PageIndex{2}\): Steel Beam

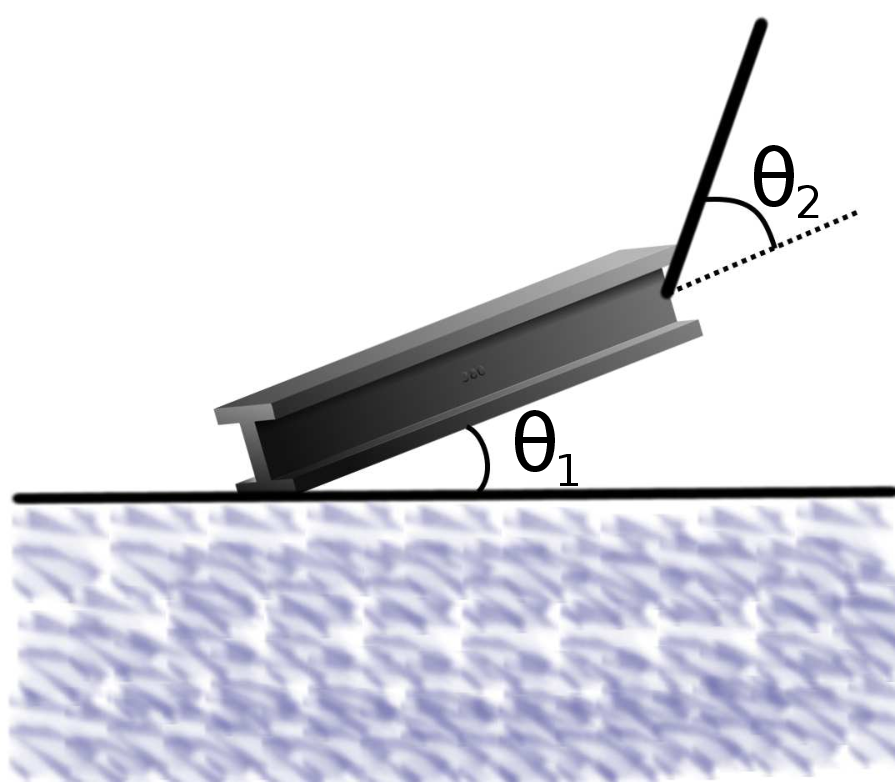
Consider the steel beam shown in the figure, with a mass of 2450 kg, being held in place by a crane. The angle between the horizontal and the beam is 15\(^\circ\), and the angle between the axis of the beam and the cable is 63\(^\circ\).

1. What is the tension in the cable, if the length of the beam is 6.5 m?
2. How much force, and in which direction, is the ground acting on the beam with?

Whiteboard Problem \(\PageIndex{3}\): Sliding Penguin

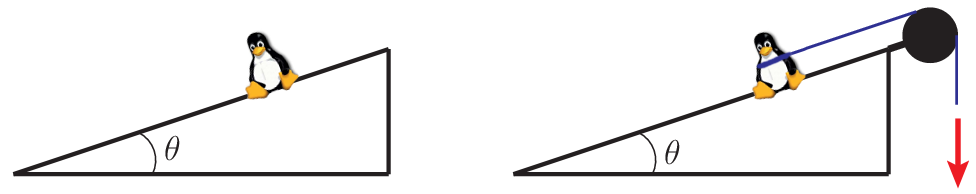
Consider a penguin sitting on a ramp, as shown in the figure on the left. The ramp makes an angle of 15\(^\circ\) with respect to the floor, the mass of the penguin is 45 kg, and the coefficient of static friction between the penguin and the ramp is 0.30.

1. If the penguin is not moving, how large is the frictional force acting on it?
2. Now I tie a rope to the penguin, as shown in the figure on the right. This rope goes over a frictionless, massless pulley. How hard must I pull on the rope before the penguin just starts to move?

 

Whiteboard Problem \(\PageIndex{4}\): Sliding Penguin Reduex

Consider a penguin sitting on a ramp as shown in the lefthand figure for Whiteboard Problem \(\PageIndex{3}\) (without the rope). This is an Emperor Penguin, so naturally it has a mass of 45 kg.

1. If the coefficient of static friction between the ramp and the penguin is 0.40, what is the maximum angle the ramp can have if the penguin is going to remain stationary?
2. If I increase the angle a little bit from part (a) then penguin will start to slide. Say I increase this angle by 10\%, and the coefficient of kinetic friction between the penguin and the ramp is 0.30, what will the acceleration of the penguin be?

 

Whiteboard Problem \(\PageIndex{5}\): Curling for Torque!

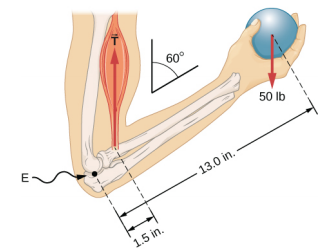
A weightlifter is holding a 50.0-lb weight (equivalent to 222.4 N) with his forearm, as shown in the Figure. His forearm is positioned at \(\theta = 60^\circ\) with respect to his upper arm, and supported by the biceps muscle, which causes a torque around the elbow (labeled ``E''). You can assume the tension $T$ on the bicep muscle is directed straight up, opposite the direction of gravity, and you can ignore the weight of the arm.

1. What tension force is in the bicep muscle? (That is, ``find \(T\)''!)
2. What is the magnitude of the force at the elbow joint?
3. In what direction (describe or find an angle!) is the force at the elbow joint acting?

Note: this problem came from the Open Stax textbook University Physics Volume 1, and they solve it there using the following free body diagram. You are welcome to  do that as well - but do you think that coordinate system is the best choice?

Example \(\PageIndex{6}\): The Torque Balance

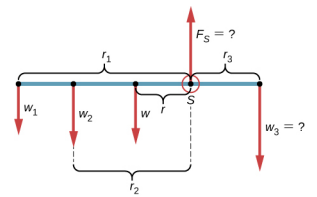
Three masses are attached to a uniform meter stick, as shown in Figure \(\PageIndex{1}\). The mass of the meter stick is 150.0 g and the masses to the left of the fulcrum are m₁ = 50.0 g and m₂ = 75.0 g. Find the mass m3 that balances the system when it is attached at the right end of the stick, and the normal reaction force at the fulcrum when the system is balanced.

Figure \(\PageIndex{1}\): In a torque balance, a horizontal beam is supported at a fulcrum (indicated by S) and masses are attached to both sides of the fulcrum. The system is in static equilibrium when the beam does not rotate. It is balanced when the beam remains level.

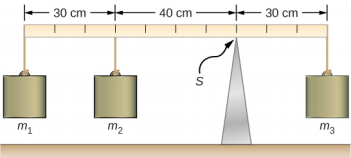
Strategy

For the arrangement shown in the figure, we identify the following five forces acting on the meter stick:

1. w₁ = m₁g is the weight of mass m₁;
2. w₂ = m₂g is the weight of mass m₂;
3. w = mg is the weight of the entire meter stick;
4. w₃ = m₃g is the weight of unknown mass m₃;
5. F_S is the normal reaction force at the support point S.

We choose a frame of reference where the direction of the y-axis is the direction of gravity, the direction of the xaxis is along the meter stick, and the axis of rotation (the z-axis) is perpendicular to the x-axis and passes through the support point S. In other words, we choose the pivot at the point where the meter stick touches the support. This is a natural choice for the pivot because this point does not move as the stick rotates. Now we are ready to set up the free-body diagram for the meter stick. We indicate the pivot and attach five vectors representing the five forces along the line representing the meter stick, locating the forces with respect to the pivot Figure \(\PageIndex{2}\). At this stage, we can identify the lever arms of the five forces given the information provided in the problem. For the three hanging masses, the problem is explicit about their locations along the stick, but the information about the location of the weight w is given implicitly. The key word here is “uniform.” We know from our previous studies that the CM of a uniform stick is located at its midpoint, so this is where we attach the weight w, at the 50-cm mark.

Figure \(\PageIndex{2}\): Free-body diagram for the meter stick. The pivot is chosen at the support point S.

Solution

With Figure \(\PageIndex{1}\) and Figure \(\PageIndex{2}\) for reference, we begin by finding the lever arms of the five forces acting on the stick:

\[\begin{split} r_{1} & = 30.0\; cm + 40.0\; cm = 70.0\; cm \\ r_{2} & = 40.0\; cm \\ r & = 50.0\; cm - 30.0\; cm = 20.0\; cm \\ r_{S} & = 0.0\; cm\; (because\; F_{S}\; is\; attached\; at\; the\; pivot) \\ r_{3} & = 30.0\; cm \ldotp \end{split}\]

Now we can find the five torques with respect to the chosen pivot:

\[\begin{split} \tau_{1} & = +r_{1} w_{1} \sin 90^{o} = +r_{1} m_{1} g \quad (counterclockwise\; rotation,\; positive\; sense) \\ \tau_{2} & = +r_{2} w_{2} \sin 90^{o} = +r_{2} m_{2} g \quad (counterclockwise\; rotation,\; positive\; sense) \\ \tau & = +rw \sin 90^{o} = +rmg \quad \quad \quad (gravitational\; torque) \\ \tau_{S} & = r_{S} F_{S} \sin \theta_{S} = 0 \quad \quad \quad \quad \quad (because\; r_{S} = 0\; cm) \\ \tau_{3} & = -r_{3} w_{3} \sin 90^{o} = -r_{3} m_{3} g \quad (counterclockwise\; rotation,\; negative\; sense) \end{split}\]

The second equilibrium condition (equation for the torques) for the meter stick is

\[\tau_{1} + \tau_{2} + \tau + \tau_{S} + \tau_{3} = 0 \ldotp\]

When substituting torque values into this equation, we can omit the torques giving zero contributions. In this way the second equilibrium condition is

\[+r_{1} m_{1} g + r_{2} m_{2} g + rmg - r_{3} m_{3} g = 0 \ldotp \label{12.17}\]

Selecting the +y-direction to be parallel to \(\vec{F}_{S}\), the first equilibrium condition for the stick is

\[-w_{1} - w_{2} - w + F_{S} - w_{3} = 0 \ldotp\]

Substituting the forces, the first equilibrium condition becomes

\[-m_{1} g - m_{2} g - mg + F_{S} - m_{3} g = 0 \ldotp \label{12.18}\]

We solve these equations simultaneously for the unknown values m₃ and F_S. In Equation \ref{12.17}, we cancel the g factor and rearrange the terms to obtain

\[r_{3} m_{3} = r_{1} m_{1} + r_{2} m_{2} + rm \ldotp\]

To obtain m₃ we divide both sides by r₃, so we have

\[\begin{split} m_{3} & = \frac{r_{1}}{r_{3}} m_{1} + \frac{r_{2}}{r_{3}} m_{2} + \frac{r}{r_{3}} m \\ & = \frac{70}{30} (50.0\; g) + \frac{40}{30} (75.0\; g) + \frac{20}{30} (150.0\; g) = 315.0 \left(\dfrac{2}{3}\right)\; g \simeq 317\; g \ldotp \end{split} \label{12.19}\]

To find the normal reaction force, we rearrange the terms in Equation \ref{12.18}, converting grams to kilograms:

\[\begin{split} F_{S} & = (m_{1} + m_{2} + m + m_{3}) g \\ & = (50.0 + 75.0 + 150.0 + 316.7) \times (10^{-3}\; kg) \times (9.8\; m/s^{2}) = 5.8\; N \ldotp \end{split} \label{12.20}\]

Significance

Notice that Equation \ref{12.17} is independent of the value of g. The torque balance may therefore be used to measure mass, since variations in g-values on Earth’s surface do not affect these measurements. This is not the case for a spring balance because it measures the force.

Exercise \(\PageIndex{7}\)

Repeat Example 12.3 using the left end of the meter stick to calculate the torques; that is, by placing the pivot at the left end of the meter stick.

In the next example, we show how to use the first equilibrium condition (equation for forces) in the vector form given by Equation 12.2.9 and Equation 12.2.10. We present this solution to illustrate the importance of a suitable choice of reference frame. Although all inertial reference frames are equivalent and numerical solutions obtained in one frame are the same as in any other, an unsuitable choice of reference frame can make the solution quite lengthy and convoluted, whereas a wise choice of reference frame makes the solution straightforward. We show this in the equivalent solution to the same problem. This particular example illustrates an application of static equilibrium to biomechanics.

Exercise \(\PageIndex{8}\)

Repeat Example 12.4 assuming that the forearm is an object of uniform density that weighs 8.896 N.

Example\(\PageIndex{9}\): A Ladder Resting Against a Wall

A uniform ladder is L = 5.0 m long and weighs 400.0 N. The ladder rests against a slippery vertical wall, as shown in Figure \(\PageIndex{6}\). The inclination angle between the ladder and the rough floor is \(\beta\) = 53°. Find the reaction forces from the floor and from the wall on the ladder and the coefficient of static friction \(\mu_{s}\) at the interface of the ladder with the floor that prevents the ladder from slipping.

Figure \(\PageIndex{6}\) A 5.0-m-long ladder rests against a frictionless wall.

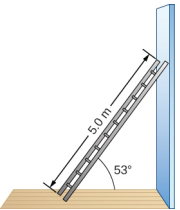
Strategy

We can identify four forces acting on the ladder. The first force is the normal reaction force N from the floor in the upward vertical direction. The second force is the static friction force f = \(\mu_{s}\)N directed horizontally along the floor toward the wall—this force prevents the ladder from slipping. These two forces act on the ladder at its contact point with the floor. The third force is the weight w of the ladder, attached at its CM located midway between its ends. The fourth force is the normal reaction force F from the wall in the horizontal direction away from the wall, attached at the contact point with the wall. There are no other forces because the wall is slippery, which means there is no friction between the wall and the ladder. Based on this analysis, we adopt the frame of reference with the y-axis in the vertical direction (parallel to the wall) and the x-axis in the horizontal direction (parallel to the floor). In this frame, each force has either a horizontal component or a vertical component but not both, which simplifies the solution. We select the pivot at the contact point with the floor. In the free-body diagram for the ladder, we indicate the pivot, all four forces and their lever arms, and the angles between lever arms and the forces, as shown in Figure \(\PageIndex{7}\). With our choice of the pivot location, there is no torque either from the normal reaction force N or from the static friction f because they both act at the pivot.

Figure \(\PageIndex{7}\): Free-body diagram for a ladder resting against a frictionless wall.

Solution

From the free-body diagram, the net force in the x-direction is

\[+f - F = 0 \label{12.28}\]

the net force in the y-direction is

\[+ N - w = 0 \label{12.29}\]

and the net torque along the rotation axis at the pivot point is

\[\tau_{w} + \tau_{F} = 0 \ldotp \label{12.30}\]

where \(\tau_{w}\) is the torque of the weight w and \(\tau_{F}\) is the torque of the reaction F. From the free-body diagram, we identify that the lever arm of the reaction at the wall is r_F = L = 5.0 m and the lever arm of the weight is r_w = \(\frac{L}{2}\) = 2.5 m. With the help of the free-body diagram, we identify the angles to be used in Equation 12.2.12 for torques: \(\theta_{F}\) = 180° − \(\beta\) for the torque from the reaction force with the wall, and \(\theta_{w}\) = 180° + (90° − \(\beta\)) for the torque due to the weight. Now we are ready to use Equation 12.2.12 to compute torques:

\[\tau_{w} = r_{w} w \sin \theta_{w} = r_{w} w \sin (180^{o} + 90^{o} - \beta) = - \frac{L}{2} w \sin (90^{o} - \beta) = - \frac{L}{2} w \cos \beta\]

\[\tau_{F} = r_{F} F \sin \theta_{F} = r_{F} F \sin (180^{o} - \beta) = LF \sin \beta \ldotp\]

We substitute the torques into Equation \ref{12.30} and solve for F :

\[- \frac{L}{2} w \cos \beta + LF \sin \beta = 0 \label{12.31}\]

\[F = \frac{w}{2} \cot \beta = \frac{400.0\; N}{2} \cot 53^{o} = 150.7\; N\]

We obtain the normal reaction force with the floor by solving Equation \ref{12.29}: N = w = 400.0 N. The magnitude of friction is obtained by solving Equation \ref{12.28}: f = F = 150.7 N. The coefficient of static friction is \(\mu_{s}\) = \(\frac{f}{N}\) = \(\frac{150.7}{400.0}\) = 0.377.

The net force on the ladder at the contact point with the floor is the vector sum of the normal reaction from the floor and the static friction forces:

\[\vec{F}_{floor} = \vec{f} + \vec{N} = (150.7\; N)(- \hat{i}) + (400.0\; N)(+ \hat{j}) = (-150.7\; \hat{i} + 400.0\; \hat{j}) N \ldotp\]

Its magnitude is

\[F_{floor} = \sqrt{f^{2} + N^{2}} = \sqrt{150.7^{2} + 400.0^{2}}\; N = 427.4\; N\]

and its direction is

\[\varphi = \tan^{-1} \left(\dfrac{N}{f}\right) = \tan^{-1} \left(\dfrac{400.0}{150.7}\right) = 69.3^{o}\; above\; the\; floor \ldotp\]

We should emphasize here two general observations of practical use. First, notice that when we choose a pivot point, there is no expectation that the system will actually pivot around the chosen point. The ladder in this example is not rotating at all but firmly stands on the floor; nonetheless, its contact point with the floor is a good choice for the pivot. Second, notice when we use Equation 12.2.12 for the computation of individual torques, we do not need to resolve the forces into their normal and parallel components with respect to the direction of the lever arm, and we do not need to consider a sense of the torque. As long as the angle in Equation 12.2.12 is correctly identified—with the help of a free-body diagram—as the angle measured counterclockwise from the direction of the lever arm to the direction of the force vector, Equation 12.2.12 gives both the magnitude and the sense of the torque. This is because torque is the vector product of the lever-arm vector crossed with the force vector, and Equation 12.2.12 expresses the rectangular component of this vector product along the axis of rotation.

Significance

This result is independent of the length of the ladder because L is canceled in the second equilibrium condition, Equation \ref{12.31}. No matter how long or short the ladder is, as long as its weight is 400 N and the angle with the floor is 53°, our results hold. But the ladder will slip if the net torque becomes negative in Equation \ref{12.31}. This happens for some angles when the coefficient of static friction is not great enough to prevent the ladder from slipping.

Exercise \(\PageIndex{10}\)

For the situation described in Example 12.5, determine the values of the coefficient \(\mu_{s}\) of static friction for which the ladder starts slipping, given that β is the angle that the ladder makes with the floor.

Example \(\PageIndex{11}\): Forces on Door Hinges

A swinging door that weighs w = 400.0 N is supported by hinges A and B so that the door can swing about a vertical axis passing through the hinges Figure \(\PageIndex{8}\). The door has a width of b = 1.00 m, and the door slab has a uniform mass density. The hinges are placed symmetrically at the door’s edge in such a way that the door’s weight is evenly distributed between them. The hinges are separated by distance a = 2.00 m. Find the forces on the hinges when the door rests half-open.

Figure \(\PageIndex{8}\): A 400-N swinging vertical door is supported by two hinges attached at points A and B.

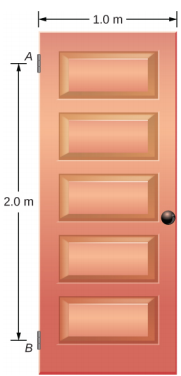
Strategy

The forces that the door exerts on its hinges can be found by simply reversing the directions of the forces that the hinges exert on the door. Hence, our task is to find the forces from the hinges on the door. Three forces act on the door slab: an unknown force \(\vec{A}\) from hinge A, an unknown force \(\vec{B}\) from hinge B, and the known weight \(\vec{w}\) attached at the center of mass of the door slab. The CM is located at the geometrical center of the door because the slab has a uniform mass density. We adopt a rectangular frame of reference with the y-axis along the direction of gravity and the x-axis in the plane of the slab, as shown in panel (a) of Figure \(\PageIndex{9}\), and resolve all forces into their rectangular components. In this way, we have four unknown component forces: two components of force \(\vec{A}\) (A_x and A_y), and two components of force \(\vec{B}\) (B_x and B_y). In the free-body diagram, we represent the two forces at the hinges by their vector components, whose assumed orientations are arbitrary. Because there are four unknowns (A_x, B_x, A_y, and B_y), we must set up four independent equations. One equation is the equilibrium condition for forces in the x-direction. The second equation is the equilibrium condition for forces in the y-direction. The third equation is the equilibrium condition for torques in rotation about a hinge. Because the weight is evenly distributed between the hinges, we have the fourth equation, A_y = B_y. To set up the equilibrium conditions, we draw a free-body diagram and choose the pivot point at the upper hinge, as shown in panel (b) of Figure \(\PageIndex{9}\). Finally, we solve the equations for the unknown force components and find the forces.

Figure \(\PageIndex{9}\): (a) Geometry and (b) free-body diagram for the door.

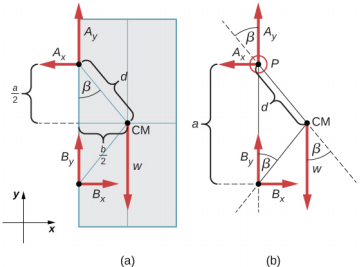
Solution

From the free-body diagram for the door we have the first equilibrium condition for forces:

in the x-direction, \(-A_{x} + B_{x} = 0 \Rightarrow A_{x} + B_{x}\) in y-direction, \(+ A_{y} + B_{y} - w = 0 \Rightarrow A_{y} = B_{y} = \frac{w}{2} = \frac{400.0\; N}{2} = 200.0\; N \ldotp\)

We select the pivot at point P (upper hinge, per the free-body diagram) and write the second equilibrium condition for torques in rotation about point P:

pivot at P:

\[\tau_{w} + \tau_{Bx} + \tau_{By} = 0 \ldotp \label{12.32}\]

We use the free-body diagram to find all the terms in this equation:

\[\begin{split} \tau_{w} & = dw \sin (- \beta) = -dw \sin \beta = -dw \frac{\frac{b}{2}}{d} = -w \frac{b}{2} \\ \tau_{Bx} & = a B_{x} \sin 90^{o} = + a B_{x} \\ \tau_{By} & = a B_{y} \sin 180^{o} = 0 \ldotp \end{split}\]

In evaluating sin \(\beta\), we use the geometry of the triangle shown in part (a) of the figure. Now we substitute these torques into Equation \ref{12.32} and compute B_x:

pivot at P: \(-w \frac{b}{2} + a B_{x} = 0 \Rightarrow B_{x} = w \frac{b}{2a} = (400.0\; N) \frac{1}{2\; \cdotp 2} = 100.0\; N \ldotp\)

Therefore the magnitudes of the horizontal component forces are A_x = B_x = 100.0 N. The forces on the door are

at the upper hinge: \(\vec{F}_{A\; on\; door} = -100.0\; N\; \hat{i} + 200.0\; N\; \hat{j}\)at the lower hinge: \(\vec{F}_{B\; on\; door} = +100.0\; N\; \hat{i} + 200.0\; N\; \hat{j} \ldotp\)

The forces on the hinges are found from Newton’s third law as

on the upper hinge: \(\vec{F}_{door\; on\; A} = 100.0\; N\; \hat{i} - 200.0\; N\; \hat{j}\)on the lower hinge: \(\vec{F}_{door\; on\; B} = - 100.0\; N\; \hat{i} - 200.0\; N\; \hat{j} \ldotp\)

Significance

Note that if the problem were formulated without the assumption of the weight being equally distributed between the two hinges, we wouldn’t be able to solve it because the number of the unknowns would be greater than the number of equations expressing equilibrium conditions.

Exercise \(\PageIndex{11}\)

Solve the problem in Example 12.6 by taking the pivot position at the center of mass.

Exercise \(\PageIndex{12}\)

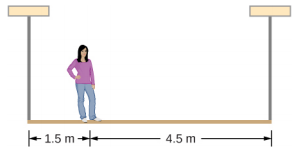
A 50-kg person stands 1.5 m away from one end of a uniform 6.0-m-long scaffold of mass 70.0 kg. Find the tensions in the two vertical ropes supporting the scaffold.

Exercise \(\PageIndex{13}\)

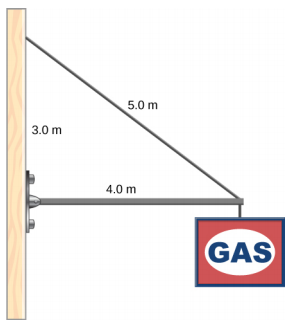
A 400.0-N sign hangs from the end of a uniform strut. The strut is 4.0 m long and weighs 600.0 N. The strut is supported by a hinge at the wall and by a cable whose other end is tied to the wall at a point 3.0 m above the left end of the strut. Find the tension in the supporting cable and the force of the hinge on the strut.

Example \(\PageIndex{14}\): A Simple Spring Problem

Consider a spring of unknown spring constant. You first want to find out what the spring constant actually is, and then use the spring to determine the mass of an unknown object. To do this, first you measure the equilibrium length of the spring to be 10 cm. Then, you put a mass of 5 kg on the end, hang it vertically, and observe that the spring stretches to a total length of 12 cm. What is the spring constant?

Now that you know the spring constant, you put the unknown mass on the spring and notice that it stretches to a length of 17 cm. What is the mass of this object?

Solution

1. Translate: We will use the following variables:
   \[y_0=10 \text{ cm},\quad y_1=12\text{ cm},\quad y_2=17\text{ cm},m_1=5\text{ kg},\quad m_2=?.\]
   Notice that we are not specifying the coordinate system quite yet - since the spring is hanging vertically, the y-coordinate might end up being negative. These are just the lengths of the various quantities we measured.
2. Model: Since the only thing we know about this system are lengths and masses, we are clearly going to have to use Hooke's law, \(F_{sp,y}=-k(y-y_0)\) (in the y-direction). Since this spring is hanging vertically, it makes sense to use Newton's 2nd law to model the equilbrium situation.
3. Solve: First, we write the condition for equilbrium when the known mass is hung on the spring. Here, we are going to take the vertical direction to be the y-coordinate, with positive upwards.
   \[\Sigma F_y = 0\rightarrow F_{sp,y}+F_{g,y}=0\rightarrow -k((-y_1)-(-y_0))+m_1g(-y_1)=0\rightarrow k=\frac{m_1gy_1}{y_1-y_0}\simeq 294\text{ Nm}\]
   Notice carefully what we did with the coordinates - we made all of them negative, with the top of the spring being the origin. Also take care that you do the conversions from centimeters to meters in the final calculation!
   Now that we have the spring constant, we can do the same thing for the unknown mass. This equation is going to look very similar, but switching around our known and unknown variables:
   \[\Sigma F_y = 0\rightarrow F_{sp,y}+F_{g,y}=0\rightarrow -k((-y_2)-(-y_0))+m_2g(-y_2)=0\rightarrow m_2=\frac{k(y_2-y_0)}{gy_2}\simeq 12.4\text {kg}.\]
   Again, take careful note of what happens algebraically with the signs.
4. Check: This is consistent with our intuition - the spring stretched more for a heavier mass. Since the amount of stretching was 5 cm as compared to 2 cm, we would expect that the mass is also more then twice as big, which it is!

Notice that although the stretching length was exactly 3.5 times bigger (7 cm / 2 cm = 3.5), the unknown mass was not 3.5 times bigger: 3.5*5 kg = 17.5 kg. We can see why this happens by using the last formula we wrote down, but plugging in the equation for the spring constant we found in the first part:

\[m_2=\frac{k(y_2-y_0)}{gy_2}=\left(\frac{m_1gy_1}{y_1-y_0} \right)\left(\frac{(y_2-y_0)}{gy_2}\right)=\left(\frac{y_1}{y_2}\right)\left(\frac{y_2-y_0}{y_1-y_0}\right)m_1.\]

So, the ratio between \(m_1\) and \(m_2\) is not simply the ratio of the displacements \((y_2-y_0)/(y_1-y_0)\), but is also scaled by the ratio of the stretch, \(y_1/y_2\).

 

Example \(\PageIndex{15}\): Dropping and object on a weighing scale.

(Short version) Suppose you drop a 5-kg object on a spring scale from a height of 1 m. If the spring constant is \(k\) = 20, 000 N/m, what will the scale read?

(Long version) OK, let’s break that up into parts. Suppose that a spring scale is just a platform (of negligible mass) sitting on top of a spring. If you put an object of mass \(m\) on top of it, the spring compresses so that (in equilibrium) it exerts an upwards force that matches that of gravity.

1. If the spring constant is \(k\) and the object’s mass is \(m\) and the whole system is at rest, what distance is the spring compressed?
2. If you drop the object from a height \(h\), what is the (instantaneous) maximum compression of the spring as the object is brought to a momentary rest? (This part is an energy problem! Assume that \(h\) is much greater than the actual compression of the spring, so you can neglect that when calculating the change in gravitational potential energy.)
3. What mass would give you that same compression, if you were to place it gently on the scale, and wait until all the oscillations died down?
4. OK, now answer the question at the top!

Solution

(a) The forces acting on the object sitting at rest on the platform are the force of gravity, \(F^G_{E,o} = −mg\), and the normal force due to the platform, \(F^n_{p,o}\). This last force is equal, in magnitude, to the force exerted on the platform by the spring (it has to be, because the platform itself is being pushed down by a force \(F^n_{o,p} = −F^n_{p,o}\), and this has to be balanced by the spring force). This means we can, for practical purposes, pretend the platform is not there and just set the upwards force on the object equal to the spring force, \(F^{spr}_{s,p} = −k(x − x_0)\). So, Newton’s second law gives

\[ F_{n e t}=F_{E, o}^{G}+F_{s, p}^{s p r}=m a=0 \label{eq:6.33} .\]

For a compressed spring, \(x − x_0\) is negative, and we can just let \(d = x_0 − x\) be the distance the spring is compressed. Then Equation (\ref{eq:6.33}) gives

\[ -m g+k d=0 \nonumber \]

so

\[ d = mg/k \label{eq:6.34} \]

when you just set an object on the scale and let it come to rest.

(b) This part, as the problem says, is a conservation of energy situation. The system formed by the spring, the object and the earth starts out with some gravitational potential energy, and ends up, with the object momentarily at rest, with only spring potential energy:

\begin{align}
U_{i}^{G}+U_{i}^{s p r} &=U_{f}^{G}+U_{f}^{s p r} \nonumber \\
m g y_{i}+0 &=m g y_{f}+\frac{1}{2} k d_{\max }^{2} \label{eq:6.35}
\end{align}

where I have used the subscript “max” on the compression distance to distinguish it from what I calculated in part (a) (this kind of makes sense also because the scale is going to swing up and down, and we want only the maximum compression, which will give us the largest reading). The problem said to ignore the compression when calculating the change in \(U^G\), meaning that, if we measure height from the top of the scale, \(y_i = h\) and \(y_f = 0\). Then, solving Equation (\ref{eq:6.35}) for \(d_{max}\), we get

\[ d_{\max }=\sqrt{\frac{2 m g h}{k}} \label{eq:6.36} .\]

(c) For this part, let us rewrite Equation (\ref{eq:6.34}) as \(m_{eq} = kd_{max}/g\), where \(m_{eq}\) is the “equivalent” mass that you would have to place on the scale (gently) to get the same reading as in part (b). Using then Equation (\ref{eq:6.36}),

\[ m_{e q}=\frac{k}{g} \sqrt{\frac{2 m g h}{k}}=\sqrt{\frac{2 m k h}{g}} \label{eq:6.37} .\]

(d) Now we can substitute the values given: \(m\) = 5 kg, \(h\) = 1 m, \(k\) = 20, 000 N/m. The result is \(m_{eq}\) = 143 kg.

(Note: if you found the purely algebraic treatment above confusing, try substituting numerical values in Eqs. (\ref{eq:6.34}) and (\ref{eq:6.36}). The first equation tells you that if you just place the 5-kg mass on the scale it will compress a distance \(d\) = 2.45 mm. The second tells you that if you drop it it will compress the spring a distance \(d_{max}\) = 70 mm, about 28.6 times more, which corresponds to an “equivalent mass” 28.6 times greater than 5 kg, which is to say, 143 kg. Note also that 143 kg is an equivalent weight of 309 pounds, so if you want to try this on a bathroom scale I’d advise you to use smaller weights and drop them from a much smaller height!)