11.8: Interpreting an Optically Thick Profile
- Page ID
- 8036
This chapter has been concerned with optically thick lines and with the curve of growth – and how one might recover the parameters \(g\) and \(l\) from the curve of growth even if the profiles of individual lines are not resolved. This section is written rather as an afterthought, albeit an important one, and it does not concern the curve of growth. It discusses how one might analyse the profile of a line that is well resolved, but is not optically thin. I use as an example a line that is thermally broadened and which, if optically thin, would have a gaussian profile. The optically thick line is no longer gaussian, but can one recover \(g\) (and hence \(T\)) from it? Just for a change, and because it is a while since we used the term “source function”, I’ll deal with an emission line.
As discussed in Section 5.7, the radiance of a slab of gas of source function \(S\) is given by
\[\label{11.8.1}I_\lambda (x)=S\int_0^{\tau(x)}e^{-\tau}\,d\tau = S(1-e^{-\tau(x)}).\]
Here, \(x=\lambda - \lambda_0\). For a thermally-broadened line, this becomes
\[\label{11.8.2}I_\lambda (x)=S\left [ 1-\text{exp}\left \{ -\tau (0) \text{exp}(-x^2 \ln 2/g^2 )\right \} \right ] ,\]
where \(g=V_m \lambda_0/ \sqrt{\ln 2}.\)
In figure XI.7, I draw two such profiles, in which the line strength of one is \(A\) times the line strength of the other. The lines, perhaps, belong to the same multiplet and the ratio of the line strengths is known. (I am here using the term line strength in the technical sense of Chapter 7.) Figure XI.7 was drawn with \(A = 2\), but since the lines are not optically thin, neither the ratio of their areas nor the ratio of their heights is two.
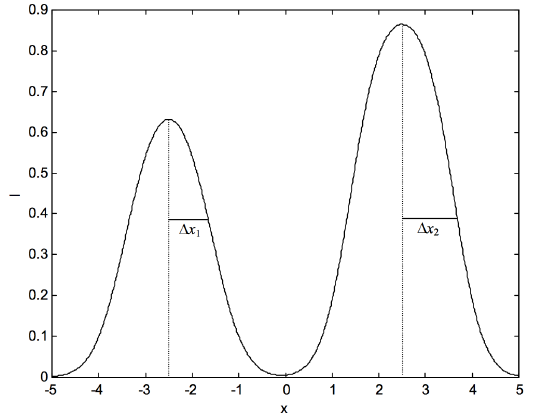
\(\text{FIGURE XI.7}\)
We can measure the half widths \(\Delta x_1\text{ and }\Delta x_2\) of the two profiles at the same height.
Then:
\[\label{11.8.3}S[1-\text{exp}\{-\tau(0)\text{exp}(-(\Delta x_1)^2\ln 2/g^2)\}]=S[1-\text{exp}\{-A\tau(0)\text{exp}(-(\Delta x_2)^2\ln 2/g^2)\}],\]
after which
\[\label{11.8.4}\frac{(\Delta x_1)\ln 2}{g^2}=\frac{(\Delta x_2)\ln 2}{g^2}-\ln A,\]
and hence
\[\label{11.8.5}g^2=\frac{[(\Delta x_1)^2-(\Delta x_2)^2]}{\ln A}.\]
The same technique can be used for lorentzian profiles.


