1.1: Three Models of Spacetime
- Page ID
- 3417
Learning Objectives
- Discuss the three models of spacetime
- Aristotelian spacetime
- Galilean spacetime
- Einstein’s spacetime
“The test of a first-rate intelligence is the ability to hold two opposing ideas in mind at the same time and still retain the ability to function.” —F. Scott Fitzgerald
Time and space together make spacetime, Figure \(\PageIndex{1}\), the stage on which physics is played out. Until 1905, physicists were trained to accept two mutually contradictory theories of spacetime. I’ll call these the Aristotelian and Galilean views, although my colleagues from that era would have been offended to be accused of even partial Aristotelianism.
Aristotelian Spacetime
Figure \(\PageIndex{2}\) shows an observer and two clocks, represented using the graphical conventions of Figure \(\PageIndex{1}\) (3). The existence of such a material object at a certain place and time constitutes an event, which we idealize as a point, Figure \(\PageIndex{2}\) (2). Spacetime consists of the set of all events. As time passes, a physical object traces out a continuous curve, a set of events known in relativistic parlance as its worldline. Since paper and computer screens are two-dimensional, the drawings only represent one dimension of space plus one dimension of time, which in relativity we call “\(1+1\) dimensions.” The real universe has three spatial dimensions, so real spacetime has \(3+1\) dimensions. Most, but not all, of the interesting phenomena in special relativity can be understood in \(1+1\) dimensions, so whenever possible in this book I will draw \(1+1\)-dimensional figures without apology or explanation.
The relativist’s attitude is that events and relationships between events are primary, while coordinates such as \(x\) and \(t\) are secondary and possibly irrelevant. Coordinates let us attach labels like (\(x,t\)) to points, but this is like God asking Adam to name all the birds and animals: the animals didn’t care about the names. Figure \(\PageIndex{2}\) (3) shows the use of vectors to indicate relationships between points. Vector \(o\) is an observer-vector, connecting two points on the world-line of the person. It points from the past into the future. The vector s connecting the two clocks is a vector of simultaneity. The clocks have previously been synchronized side by side, and if we assume that transporting them to separate locations doesn’t disrupt them, then the fact that both clocks read two minutes after three o’clock tells us that the two events occur at the same time.
The Aristotelian model of spacetime is characterized by a set of rules about what vectors are valid observer- and simultaneity vectors. We require that every \(o\) vector be parallel to every other, and likewise for \(s\) vectors. But, as is usual with vectors, we allow the arrow to be drawn anywhere without considering the different locations to have any significance; that is, our model of spacetime doesn’t allow different regions to have different properties.
When Einstein was a university student, these rules (phrased differently) were the ones he was taught to use in describing electricity and magnetism. He later recalled imagining himself on a motorcycle, riding along next to a light wave and trying to imagine how his observations could be reconciled with Maxwell’s equations. I don’t know whether he was ever brave enough to describe this daydream to his professors, but if he had, their answer would have been essentially that his hypothetical \(o\) vector was illegal. The good \(o\) vectors were thought to be the ones that represented an observer at rest relative to the ether, a hypothetical all-pervasive medium whose vibrations were electromagnetic waves. However silly this might seem to us a hundred years later, it was in fact strongly supported by the evidence. A vast number of experiments had verified the validity of Maxwell’s equations, and it was known that if Maxwell’s equations were valid in coordinates (\(x,t\)) defined by an observer \(o\), they would become invalid under the transformation \((x', t') = (x + vt, t)\) to coordinates defined by an observer \(o'\) in motion at velocity \(v\) relative to \(o\).
Galilean Spacetime
But the Aristotelian model was already known to be wrong when applied to material objects. The classic empirical demonstration of this fact came around 1610 with Galileo’s discovery of four moons orbiting Jupiter, Figure \(\PageIndex{4}\). Aristotelianism in its ancient form was originally devised as an explanation of why objects always seemed to settle down to a natural state of rest according to an observer standing on the earth’s surface. But as Jupiter flew across the heavens, its moons circled around it, without showing any natural tendency to fall behind it like a paper cup thrown out the window of a car. Just as an observer \(o_1\) standing on the earth would consider the earth to be at rest, \(o_2\) hovering in a balloon at Jupiter’s cloud tops would say that the Jovian clouds represented an equally “natural” state of rest.
Figure \(\PageIndex{4}\): A simulation of how Jupiter and its moons might appear at intervals of three hours through a telescope. Because we see the moons’ circular orbits edge on, their world-lines appear sinusoidal. Over this time period, the innermost moon, \(Io\), completes half a cycle.
We are thus led to a different, Galilean, set of rules for \(o\) and \(s\) vectors. All \(s\) vectors are parallel to one another, but any vector \(t\) is not parallel to an \(s\) vector is a valid \(o\) vector. (We may wish to require that it point into the future rather than the past, but Newton’s laws are symmetric under time-reversal, so this is not strictly necessary.)
Galilean spacetime, unlike Aristotelian spacetime, has no universal notion of “same place.” I can drive to Gettysburg, Pennsylvania, and stand in front of the brass plaque that marks the site of the momentous Civil War battle. But am I really in the same place? An observer on another planet would say that our planet had moved through space since 1863.
Parallelism
Note that our geometrical description includes a notion of parallelism, but not of angular measure. We don’t know or care whether the “angle” between an \(s\) and an \(o\) is \(90\) degrees. One represents a distance, while the other represents an interval of time, and we can’t define the angle between a distance and a time. The same was true in the Aristotelian model; the vectors in Fgure \(\PageIndex{3}\) were drawn perpendicular to one another simply as a matter of convention, but any other angle could have been used.
The Galilean Twin Paradox
Alice and Betty are identical twins. Betty goes on a space voyage, traveling away from the earth along vector \(o_1\) and then turning around and coming back on \(o_2\). Meanwhile, Alice stays on earth. Because this is an experiment involving material objects, and the conditions are similar to those under which Galilean relativity has been repeatedly verified by experiment, we expect the results to be consistent with Galilean relativity’s claim that motion is relative. Therefore it seems that it should be equally valid to consider Betty and the spaceship as having been at rest the whole time, while Alice and the planet earth traveled away from the spaceship along \(o_3\) and then returned via \(o_4\). But this is not consistent with the experimental results, which show that Betty undergoes a violent acceleration at her turnaround point, while Alice and the other inhabitants of the earth feel no such effect.
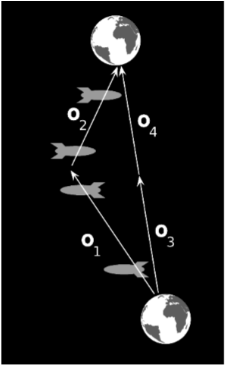
The paradox is resolved by realizing that Galilean relativity defines unambiguously whether or not two vectors are parallel. It’s true that we could fix a frame of reference in which \(o_1\) represented the spaceship staying at rest, but \(o_2\) is not parallel to \(o_1\), so in this frame we still have a good explanation for why Betty feels an acceleration: she has gone from being at rest to being in motion. Regardless of which frame of reference we pick, and regardless of whether we even fix a frame of reference, \(o_3\) and \(o_4\) are parallel to one another, and this explains why Alice feels no effect.
Einstein’s Spacetime
We have two models of spacetime, neither of which is capable of describing all the phenomena we observe. Because of the relatively crude state of technology ca. 1900, it required considerable insight for Einstein to piece together a fragmentary body of indirect evidence and arrive at a consistent and correct model of spacetime. Today, the evidence is part of everyday life. For example, every time you use a GPS receiver, you’re using Einstein’s theory of relativity. Somewhere between 1905 and today, technology became good enough to allow conceptually simple experiments that students in the early \(20^{th}\) century could only discuss in terms like “Imagine that we could...”
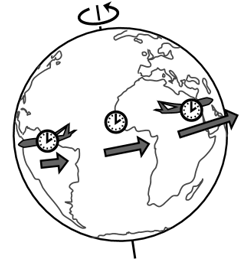
A good jumping-on point is 1971. In that year, J.C. Hafele and R.E. Keating brought atomic clocks aboard commercial airliners, Fgure \(\PageIndex{7}\), and went around the world, once from east to west and once from west to east. Hafele and Keating observed that there was a discrepancy between the times measured by the traveling clocks and the times measured by similar clocks that stayed home at the U.S. Naval Observatory in Washington. The east-going clock lost time, ending up off by \(-59 \pm 10\) nanoseconds, while the west-going one gained \(273 \pm 7\) ns.
We are used to thinking of time as absolute and universal, so it is disturbing to find that it can flow at a different rates for different observers. Nevertheless, the effects that Hafele and Keating observed were small. This makes sense: Galilean relativity had already been thoroughly verified for material objects such as clocks, planets, and airplanes, so a new theory like Einstein’s had to agree with Galileo’s to a good approximation, within the Galilean theory’s realm of applicability. This requirement of backward-compatibility is known as the correspondence principle.
It’s also reassuring that the effects on time were small compared to the three-day lengths of the plane trips. There was therefore no opportunity for paradoxical scenarios such as one in which the east going experimenter arrived back in Washington before he left and then convinced himself not to take the trip. A theory that maintains this kind of orderly relationship between cause and effect is said to satisfy causality.
Hafele and Keating were testing specific quantitative predictions of relativity, and they verified them to within their experiment’s error bars. Let’s work backward instead, and inspect the empirical results for clues as to how time works. The disagreements among the clocks suggest that simultaneity is not absolute: different observers have different notions of simultaneity, as suggested in Figure \(\PageIndex{9}\). Just as Galilean relativity freed the \(o\) vectors from the constraint of being parallel to one another, Einstein frees the \(s\) vectors. Galileo made “same place” into an ambiguous concept, while Einstein did the same with “simultaneous.” But because a particular observer does have methods of synchronizing clocks (e.g., Einstein synchronization, example \(\PageIndex{4}\)), the definition of simultaneity isn’t completely arbitrary. For each \(o\) vector we have a corresponding \(s\) vector, which represents that observer’s opinion as to what constitutes simultaneity. Because the convention on a Cartesian \(x - t\) graph is to draw the axes at right angles to one another, we refer to such a pair of vectors as orthogonal, but the word is not to be interpreted literally, since we can’t define an actual angle between a time interval and a spatial displacement.

What, then, are the rules for orthogonality? Figure \(\PageIndex{10}\) shows three possibilities. In each case, we have an initial pair of vectors \(o_1\) and \(s_1\) that we assume are orthogonal, and we then draw a new pair \(o_2\) and \(s_2\) for a second observer who is in motion relative to the first. The Galilean case, where \(s_2\) remains parallel to \(s_1\), has already been ruled out. The second case is the one in which \(s\) rotates in the same direction as \(o\). This one is forbidden by causality, because if we kept on rotating, we could eventually end up rotating \(o\) by \(180\) degrees, so by a continued process of acceleration, we could send an observer into a state in which her sense of time was reversed. We are left with only one possibility for Einstein’s spacetime, which is the one in which a clockwise rotation of \(o\) causes a counterclockwise rotation of \(s\), like closing a pair of scissors.
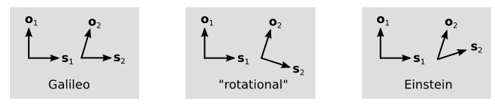
Now there is a limit to how far this process can go, or else the \(s\) and \(o\) would eventually lie on the same line. But this is impossible, for a valid \(s\) vector can never be a valid \(o\), nor an \(o\) a valid \(s\). Such a possibility would mean that an observer would describe two different points on his own world-line as simultaneous, but an observer for whom no time passes is not an observer at all, since observation implies collecting data and then being able to remember it at some later time. We conclude that there is a diagonal line that forms the boundary between the set of possible \(s\) vectors and the set of valid \(o\) vectors. This line has some slope, and the inverse of this slope corresponds to some velocity, which is apparently a universal and fixed property of Einstein’s spacetime. This velocity we call \(c\), and the correspondence principle tells us that \(c\) must be very large, because otherwise Einsteinian, or “relativistic,” effects such as time distortion would have been large even for motion at everyday speeds; in the Hafele-Keating experiment they were quite small, even at the high speed of a passenger jet.
Although \(c\) is a large number when expressed in meters per second, for convenience in relativity we will always choose units such that \(c = 1\). The boundary between \(s\) and \(o\) vectors then appears on spacetime diagrams as a diagonal line at \(\pm 45\) degrees. In more than one spatial dimension, this boundary forms a cone,Ffigure \(\PageIndex{11}\), and for reasons that will become more clear in a moment, this cone is called the light cone. Vectors lying inside the light cone are referred to as timelike, those outside as spacelike, and those on the cone itself as lightlike or null.

An important advantage of Einstein’s relativity over Galileo’s is that it is compatible with the empirical observation that some phenomena travel at a certain fixed speed. Light travels at a fixed speed, and so do other phenomena such as gravitational waves (first directly detected in 2016). So do all massless particles (subsection 4.3.4). This fixed speed is \(c\), and all observers agree on it. In 1905, the only phenomenon known to travel at \(c\) was light, so \(c\) is usually described as the “speed of light,” but from the modern point of view it functions more as a kind of conversion factor between our units of measurement for time and space. It is a property of spacetime, not a property of light.
More fundamentally, \(c\) is the maximum speed of cause and effect. If we could propagate cause and effect, e.g., by transmitting a signal, at a speed greater than \(c\), then the following argument shows that we would be violating either causality or the principle that motion is relative. If a signal could be propagated at a speed greater than \(c\), then the vector \(r\) connecting the cause and the effect would be spacelike. By opening and closing the “scissors” of Figure \(\PageIndex{10}\), we can always find an observer \(o\) who considers \(r\) to be a vector of simultaneity. Thus if faster-than-light propagation is possible, then instantaneous propagation is possible, at least for some observer. Since motion is relative, this must be possible for all observers, regardless of their state of motion. Therefore any spacelike vector is one along which we can send a signal. But by adding two spacelike vectors we can make a vector lying in the past timelike light cone, so by relaying the signal we could send a message into the past, violating causality.
In interpreting this argument, note that neither the relativity of motion nor causality is a logical necessity; they are both just generalizations based on a body of evidence. For more on causality, and its uncertain empirical status, see section 2.1.
The Ring Laser Gyroscope
If you’ve flown in a jet plane, you can thank relativity for helping you to avoid crashing into a mountain or an ocean. Figure \(\PageIndex{12}\) shows a standard piece of navigational equipment called a ring laser gyroscope. A beam of light is split into two parts, sent around the perimeter of the device, and reunited. Since the speed of light is constant, we expect the two parts to come back together at the same time. If they don’t, it’s evidence that the device has been rotating. The plane’s computer senses this and notes how much rotation has accumulated.

No Frequency - Dependence
Relativity has only one universal speed, so it requires that all light waves travel at the same speed, regardless of their frequency and wavelength. Presently the best experimental tests of the invariance of the speed of light with respect to wavelength come from astronomical observations of gamma-ray bursts, which are sudden outpourings of high-frequency light, believed to originate from a supernova explosion in another galaxy. One such observation, in 2009, found that the times of arrival of all the different frequencies in the burst differed by no more than \(2\) seconds out of a total time in flight on the order of ten billion years!
Einstein's Train
The Fgure \(\PageIndex{13}\) shows a famous thought experiment devised by Einstein. A train is moving at constant velocity to the right when bolts of lightning strike the ground near its front and back. Alice, standing on the dirt at the midpoint of the flashes, observes that the light from the two flashes arrives simultaneously, so she says the two strikes must have occurred simultaneously. Bob, meanwhile, is sitting aboard the train, at its middle. He passes by Alice at the moment when Alice later figures out that the flashes happened. Later, he receives flash \(2\), and then flash \(1\). He infers that since both flashes traveled half the length of the train,flash \(2\) must have occurred first. How can this be reconciled with Alice’s belief that the flashes were simultaneous?
Figure \(\PageIndex{14}\) shows the corresponding spacetime diagram. It seems paradoxical that Alice and Bob disagree on simultaneity, but this is only because we have an ingrained prejudice in favor of Galilean relativity. Alice’s method of determining that \(1\) and \(2\) were simultaneous is valid, and is known as Einstein synchronization. The dashed line connecting \(1\) and \(2\) is orthogonal to Alice’s worldline. But Bob has a different opinion about what constitutes simultaneity. The slanted dashed line is orthogonal to his worldline. According to Bob, \(2\) happened before the time represented by this line, \(1\) after.

Example \(\PageIndex{4}\) is of course impractical as described, since real trains don’t travel at speeds anywhere near \(c\) relative to the dirt. We say that their speeds are “nonrelativistic.” Because Einstein coined the term “relativity,” and his version of relativity superseded Galileo’s, the unmodified word is normally understood to refer to Einsteinian relativity. A physicist who studies Einstein-relativity is a relativist. A material object moving at a speed very close to \(c\) is described as ultrarelativistic. One often hears lay people describing relativity in terms of certain effects that would happen “if you went at the speed of light.” In fact, as we’ll see in chapter 3 and chapter 4, it is not possible to accelerate material objects to \(c\), and in any case that isn’t necessary. Relativistic effects exist at all speeds, but they’re weak at speeds small compared to \(c\).
Numerical value of c
In this book we’ll use units in which \(c = 1\). However, many beginners are vexed by the question of why \(c\) has the particular value it does in a given system of units such as the SI. Related to this is the question of whether \(c\) could ever change, so that measuring it today and measuring it tomorrow would give slightly different results. In a system of units where \(c\) has units, its value is what it is only because of our choice of units, and there is no meaningful way to test whether it changes.
Let’s take the SI as an example of a system of units. The SI was originally set up so that the meter and the second were defined in terms of properties of our planet. The meter was one forty-millionth of the earth’s circumference, and the second was \(1/86,400\) of a mean solar day. Thus when we express \(c\) as \(3.0 \times 10^8 m/s\), we are basically specifying the factor by which \(c\) exceeds the speed at which a point on the equator goes around the center of the earth (with additional conversion factors of \(40,000,000\) and \(86,400\) thrown in). Since the properties of our planet are accidents of the formation of the solar system, there is no physical theory that can tell us why \(c\) has this value in the original French-Revolutionary version of the SI.
The base units of the SI were redefined over the centuries. Today, the second is defined in terms of an atomic standard, and the meter is defined as \(1/299,792,458\) of a light-second. Therefore \(c\) has a defined value of exactly \(299,792,458 m/s\). Again, we find that the numerical value of \(c\) has no fundamental significance; it is merely a matter of definition. It is possible to form the unitless ratio \(\alpha = e^2/\tilde{h} c \approx 1/137\), called the fine structure constant. Its value does not depend on our choice of units, so it is possible to do experiments to look for changes in its value over time, e.g., by comparing the spectrum of hydrogen on earth with the spectrum of distant stars, whose light has taken billions of years to get to us. Claims have even been made to the effect that these observations do show a change, although this appears to have been a mistake. If such a change did occur, we would not be able to attribute it unambiguously to a change in \(c\) rather than a change in \(\tilde{h}\) or the fundamental charge. The standards used to define our units could change over time. The platinum-iridium standard for the kilogram in Paris is suspected to have lost about \(50\) µg of mass over the last century. Even the atomic standard used to define the second could be changing due to physics beyond our present knowledge. A change in \(c\) might produce such a change, but any such change could also be produced by changes in other physical constants, such as the others occurring in the fine structure constant. Such issues are discussed at greater length in Section 9.6.
Discussion question \(\PageIndex{1}\)
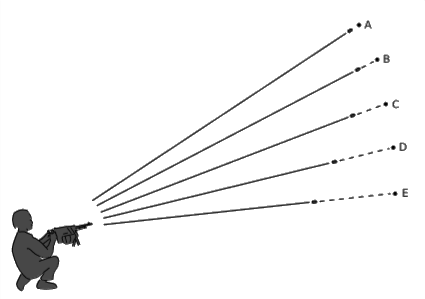
The machine-gunner in the Fgure \(\PageIndex{15}\) sends out a spray of bullets. Suppose that the bullets are being shot into outer space, and that the distances traveled are trillions of miles (so that the human figure in the diagram is not to scale). After a long time, the bullets reach the points shown with dots which are all equally far from the gun. Their arrivals at those points are events \(A\) through \(E\), which happen at different times. The chain of impacts extends across space at a speed greater than \(c\). Does this violate special relativity?