Skip to main content
\(\require{cancel}\)
Physics LibreTexts

5.4: Rotation Operators in Spin Space

  1. Last updated
    08:28, 16 Nov 2014
  2. Save as PDF

Let us, for the moment, forget about the spatial position of the particle, and concentrate on its spin state. A general spin state $ A$ is represented by the ket

 

$\displaystyle \vert A\rangle = \langle +\vert A\rangle \vert+\rangle + \langle -\vert A\rangle \vert-\rangle$ (439)

 

 

in spin space. In Section 4.3, we were able to construct an operator $ R_z({\mit\Delta}\varphi)$ that rotates the system through an angle $ {\mit\Delta}\varphi$ about the $ z$ -axis in position space. Can we also construct an operator $ T_z({\mit\Delta}\varphi)$ that rotates the system through an angle $ {\mit\Delta}\varphi$ about the $ z$ -axis in spin space? By analogy with Equation (351), we would expect such an operator to take the form

 

$\displaystyle T_z({\mit\Delta}\varphi) = \exp(-{\rm i} \,S_z\, {\mit\Delta}\varphi/\hbar).$ (440)

 

 

Thus, after rotation, the ket $ \vert A\rangle$ becomes

 

$\displaystyle \vert A_R\rangle = T_z({\mit\Delta}\varphi)\, \vert A\rangle.$ (441)

 

 

To demonstrate that the operator (440) really does rotate the spin of the system, let us consider its effect on $ \langle S_x\rangle$ . Under rotation, this expectation value changes as follows:

 

$\displaystyle \langle S_x\rangle \rightarrow \langle A_R\vert \,S_x\, \vert A_R \rangle = \langle A\vert \,T_z^{\dag }\, S_x \,T_z \,\vert A\rangle.$ (442)

 

 

Thus, we need to compute

 

$\displaystyle \exp(\,{\rm i}\,S_z\, {\mit\Delta}\varphi/\hbar)\, S_x \, \exp(-{\rm i}\,S_z \,{\mit\Delta}\varphi/\hbar).$ (443)

 

 

This can be achieved in two different ways.

First, we can use the explicit formula for $ S_x$ given in Equation (427). We find that Equation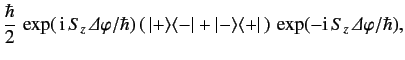 (443) becomes

 

$\displaystyle \frac{\hbar}{2} \,\exp(\,{\rm i}\,S_z\, {\mit\Delta}\varphi/\hbar... ...rt-\rangle \langle +\vert\,)\, \exp(-{\rm i}\,S_z \,{\mit\Delta}\varphi/\hbar),$ (444)

 

 

or

 

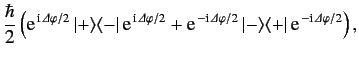$\displaystyle \frac{\hbar}{2} \left( {\rm e}^{\,{\rm i}\,{\mit\Delta}\varphi/2}... ...ert-\rangle \langle +\vert\,{\rm e}^{\,-{\rm i}\,{\mit\Delta}\varphi/2}\right),$ (445)

 

 

which reduces to

 

$\displaystyle S_x\,\cos{\mit\Delta}\varphi - S_y\,\sin{\mit\Delta}\varphi,$ (446)

 

 

where use has been made of Equations (427)-(429).

A second approach is to use the so called Baker-Hausdorff lemma. This takes the form

 

$\displaystyle \exp(\,{\rm i}\, G\,\lambda)\,A\, \exp(-{\rm i} \,G \,\lambda)$ $\displaystyle = A + {\rm i} \,\lambda\, [G,A] + \left(\frac{{\rm i}^2 \lambda^2}{2!}\right) [G, [G,A]]+$    
  $\displaystyle + \left(\frac{{\rm i}^3\lambda^3}{3!}\right)[G, [G, [G,A]]]+\cdots,$ (447)

 

 

where $ G$ is an Hermitian operator, and $ \lambda$ a real parameter. The proof of this lemma is left as an exercise. Applying the Baker-Hausdorff lemma to Equation (443), we obtain

 

$\displaystyle S_x + \left(\frac{{\rm i}\,{\mit\Delta}\varphi}{\hbar}\right) [S_... ...\frac{{\rm i}\,{\mit\Delta}\varphi}{\hbar}\right)^2 [S_z, [S_z, S_x]] + \cdots,$ (448)

 

 

which reduces to

 

$\displaystyle S_x\left[ 1- \frac{({\mit\Delta}\varphi)^2}{2!} + \frac{({\mit\De... ...{\mit\Delta}\varphi)^3}{3!}+ \frac{({\mit\Delta}\varphi)^5}{5!} +\cdots\right],$ (449)

 

 

or

 

$\displaystyle S_x\,\cos{\mit\Delta}\varphi - S_y\,\sin{\mit\Delta}\varphi,$ (450)

 

 

where use has been made of Equation (417). The second proof is more general than the first, because it only uses the fundamental commutation relation (417), and is, therefore, valid for systems with spin angular momentum higher than one-half.

For a spin one-half system, both methods imply that

 

$\displaystyle \langle S_x \rangle \rightarrow \langle S_x\rangle \,\cos{\mit\Delta}\varphi - \langle S_y\rangle\,\sin{\mit\Delta}\varphi$ (451)

 

 

under the action of the rotation operator (440). It is straightforward to show that

 

$\displaystyle \langle S_y \rangle \rightarrow \langle S_y\rangle \,\cos{\mit\Delta}\varphi + \langle S_x\rangle\,\sin{\mit\Delta}\varphi.$ (452)

 

 

Furthermore,

 

$\displaystyle \langle S_z \rangle \rightarrow\langle S_z\rangle,$ (453)

 

 

because $ S_z$ commutes with the rotation operator. Equations (451)-(453) demonstrate that the operator (440) rotates the expectation value of $ {\bf S}$ by an angle $ {\mit\Delta}\varphi$ about the $ z$ -axis. In fact, the expectation value of the spin operator behaves like a classical vector under rotation:

 

$\displaystyle \langle S_k \rangle \rightarrow \sum_l R_{k\,l}\, \langle S_l\rangle,$ (454)

 

 

where the $ R_{k\,l}$ are the elements of the conventional rotation matrix for the rotation in question. It is clear, from our second derivation of the result (451), that this property is not restricted to the spin operators of a spin one-half system. In fact, we have effectively demonstrated that

 

$\displaystyle \langle J_k \rangle \rightarrow \sum_l R_{k\,l} \,\langle J_l\rangle,$ (455)

 

 

where the $ J_k$ are the generators of rotation, satisfying the fundamental commutation relation $ {\bf J}\times {\bf J} = {\rm i}\,\hbar\, {\bf J}$ , and the rotation operator about the $ k$ th axis is written $ R_k ({\mit\Delta}\varphi) = \exp(-{\rm i}\,J_k\, {\mit\Delta}\varphi/\hbar)$ .

Consider the effect of the rotation operator (440) on the state ket (439). It is easily seen that

 

$\displaystyle T_z({\mit\Delta}\varphi)\,\vert A\rangle = {\rm e}^{-{\rm i}\,{\m... ... e}^{\,{\rm i}\,{\mit\Delta}\varphi/2}\, \langle -\vert A\rangle \vert-\rangle.$ (456)

 

 

Consider a rotation by $ 2\pi$ radians. We find that

 

$\displaystyle \vert A\rangle \rightarrow T_z(2\pi)\,\vert A\rangle = -\vert A\rangle.$ (457)

 

 

Note that a ket rotated by $ 2\pi$ radians differs from the original ket by a minus sign. In fact, a rotation by $ 4\pi$ radians is needed to transform a ket into itself. The minus sign does not affect the expectation value of $ {\bf S}$ , since $ {\bf S}$ is sandwiched between $ \langle A\vert$ and $ \vert A\rangle$ , both of which change sign. Nevertheless, the minus sign does give rise to observable consequences, as we shall see presently.

Contributors