S11. The Friedmann Equation - SOLUTIONS

Exercise 11.1.1

Answer

K.E. is \(\frac{1}{2}mv^2\), where \(v = \dot a \ell\), so the test particle's kinetic energy is

\[\begin{equation*}
\begin{aligned}
\frac{1}{2}m\dot a^2 \ell ^2
\end{aligned}
\end{equation*}\]

Exercise 11.1.2

Answer

P.E. is \(-\frac{GM(<d)m}{d}\), where by \(M(<d)\) we mean the mass contained in the sphere of radius \(d\), so \(M(<d) = \frac{4}{3}\pi d^3 \rho.\)

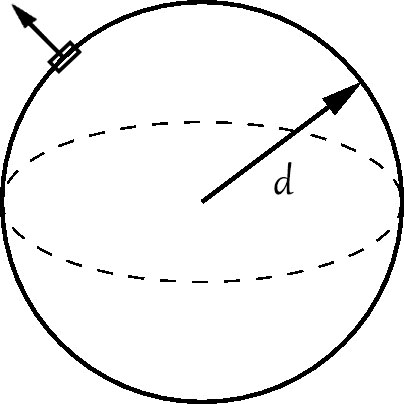
Therefore the test particle's potential energy is

\[\begin{equation*}
\begin{aligned}
-G \frac{4}{3} \pi \rho d^2 m
\end{aligned}
\end{equation*}\]

Exercise 11.1.3

Answer

\[\begin{equation*}
\begin{aligned}
\frac{1}{2}m\dot a^2 \ell ^2 - G \frac{4}{3} \pi \rho d^2 m = \kappa
\end{aligned}
\end{equation*}\]

Exercise 11.1.4

Answer

Recall that \(d = a \ell \; \Longrightarrow \; \ell = \frac{d}{a}\), substituting this in and rearranging our equation we get

\[\begin{equation*}
\begin{aligned}
\frac{1}{2}md^2 \frac{\dot a^2}{a^2} - \frac{1}{2}md^2 \frac{8\pi G\rho}{3} = \kappa
\end{aligned}
\end{equation*}\]

dividing through by \(\frac{1}{2}md^2\) gives

\[\begin{equation*}
\begin{aligned}
\frac{\dot a^2}{a^2} - \frac{8\pi G\rho}{3} = \frac{2\kappa}{m}\frac{1}{d^2}
\end{aligned}
\end{equation*}\]

Then we substitute back in \(d = a \ell\) and solve for \(\big(\frac{\dot a}{a}\big)^2\):

\[\begin{equation*}
\begin{aligned}
\left(\frac{\dot a}{a}\right)^2 = \frac{8\pi G \rho}{3} + \frac{2\kappa}{ml^2} \times \frac{1}{a^2}
\end{aligned}
\end{equation*}\]