3.5: Spherical Triangles
( \newcommand{\kernel}{\mathrm{null}\,}\)
As with plane triangles, we denote the three angles by A, B, C and the sides opposite to them by a, b, c. We are fortunate in that we have four formulas at our disposal for the solution of a spherical triangle, and, as with plane triangles, the art of solving a spherical triangle entails understanding which formula is appropriate under given circumstances. Each formula contains four elements (sides and angles), three of which, in a given problem, are assumed to be known, and the fourth is to be determined.
Three important points are to be noted before we write down the formulas.
- The formulas are valid only for triangles in which the three sides are arcs of great circles. They will not do, for example, for a triangle in which one side is a parallel of latitude.
- The sides of a spherical triangle, as well as the angles, are all expressed in angular measure (degrees and minutes) and not in linear measure (metres or kilometres). A side of 50∘ means that the side is an arc of a great circle subtending an angle of 50∘ at the centre of the sphere.
- The sum of the three angles of a spherical triangle add up to more than 180∘.
In this section are now given the four formulas without proof, the derivations being given in a later section. The four formulas may be referred to as the sine formula, the cosine formula, the polar cosine formula, and the cotangent formula. Beneath each formula is shown a spherical triangle in which the four elements contained in the formula are highlighted.
The sine formula: sinasinA=sinbsinB(=sincsinC)
FIGURE III.10
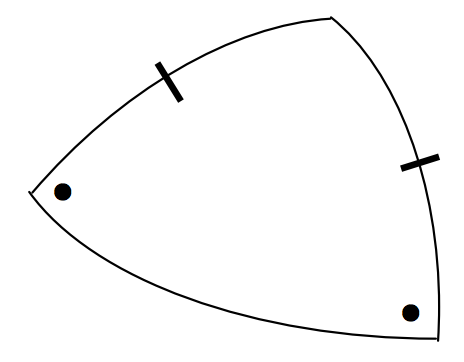
The cosine formula: cosa=cosbcosc+sinbsinccosA
FIGURE III.11

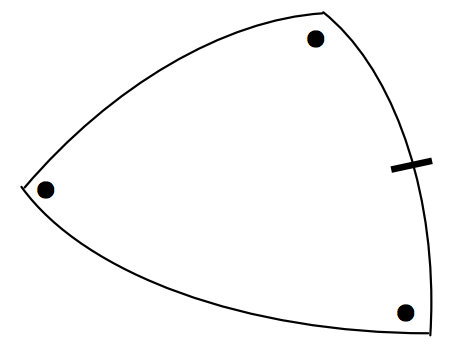
The polar cosine formula: cosA=−cosBcosC+sinBsinCcosa
FIGURE III.12
The cotangent formula: cosbcosA=sinbcotc−sinAcotC
FIGURE III.13
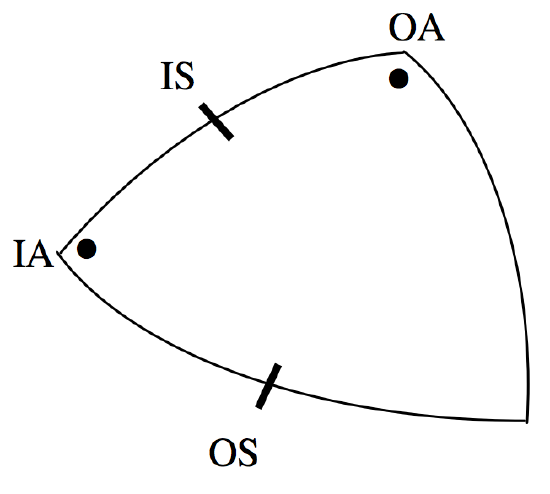
The cotangent formula is a particularly useful and frequently needed formula, and it is unfortunate that it is not only difficult to commit to memory but, even with the formula written out in front of one, it is often difficult to decide which is b, which is A and so on. However, it should be noted from the drawing that the four elements, side-angle-side-angle, lie adjacent to each other in the triangle, and they may be referred to as outer side (OS), inner angle (IA), inner side (IS) and outer angle (OA) respectively. Many people find that the formula is much easier to use when written in the form
cos(IS)cos(IA)=sin(IS)cot(OS)−sin(IA)cot(OA)
The reader will shortly be offered a goodly number of examples in the use of these formulas. However, during the course of using the formulas, it will be found that there is frequent need to solve deceptively simple trigonometric Equations of the type
4.737sinθ+3.286cosθ=5.296
After perhaps a brief pause, one of several methods may present themselves to the reader - but not all methods are equally satisfactory. I am going to suggest four possible ways of solving this Equation. The first method is one that may occur very quickly to the reader as being perhaps rather obvious - but there is a cautionary tale attached to it. While the method may seem very obvious, a difficulty does arise, and the reader would be advised to prefer one of the less obvious methods. There are, incidentally, two solutions to the Equation between 0∘ and 360∘. They are 31∘58′.6 and 78∘31′.5.
Method i
The obvious method is to isolate cosθ :
cosθ=1.611 686−1.441 570 sinθ.
Although the constants in the problem were given to four significant figures, do not be tempted to round off intermediate calculations to four. It is a common fault to round off intermediate calculations prematurely. The rounding-off can be done at the end.
Square both sides, and write the left hand side, cos2θ, as 1−sin2θ. We now have a quadratic Equation in sinθ :
3.078 125sin2θ−4.646 717sinθ+1.597 532=0.
The two solutions for sinθ are 0.529 579 and 0.908 014 and the four values of θ that satisfy these values of sinθ are 31∘58′.6, 148∘ 01′.4, 78∘ 31′.5 and 101∘28′.5.
Only two of these angles are solutions of the original Equation. The fatal move was to square both sides of the original Equation, so that we have found solutions not only to
cosθ=1.611 686−1.441 570sinθ
but also to the different Equation
−cosθ=1.611 686−1.441 570 sinθ.
This generation of extra solutions always occurs whenever we square an Equation. For this reason, method (i), however tempting, should be avoided, particularly when programming a computer to carry out a computation automatically and uncritically.
If in doubt whether you have obtained a correct solution, substitute your solution in the original Equation. You should always do this with any Equation of any sort, anyway.
Method ii
This method makes use of the identities
sinθ=2t1+t2,cosθ=1−t21+t2,
where t=tan12θ.
When applied to the original Equation, this results in the quadratic Equation in t:
8.582t2−9.474t+2.010=0
with solutions t=0.286528andt=0.817410
The only values of θ between 0∘ and 360∘ that satisfy these are the two correct solutions 31∘ 58′.6 and 78∘ 31′.5.
It is left as an exercise to show, using this method algebraically, that the solutions to the Equation
asinθ+bcosθ=c
are given by tan12θ=a±√a2+b2−c2b+c.
This shows that there are no real solutions if a2+b2<c2, one real solution if a2+b2=c2, and two real solutions if a2+b2>c2.
Method iii
We divide the original Equation
4.737sinθ+3.286cosθ=5.296
by the "hypotenuse" of 4.737 and 3.286; that is, by √(4.7372+3.2862)=5.765151.
Thus 0.821 661 sinθ+0.569 976cosθ=0.918 623
Now let 0.821 661=cosα and 0.569976=sinα (which we can, since these numbers now satisfy sin2αcos2α=1) so that α=34∘ 44′.91.
We have cosαsinθ+sinαcosθ=0.918 623
or sin(θ+α)=0.918623
from which θ+α=66∘ 43′.54 or 113∘ 16′.46
Therefore θ=31∘ 58′.6 or 78∘ 31′.5
Method iv
Methods ii and iii give explicit solutions, so there is perhaps no need to use numerical methods. Nevertheless, the reader might like to solve, by Newton-Raphson iteration, the Equation
f(θ)=asinθ+bcosθ−c=0,
for which f′(θ)=acosθ−bsinθ.
Using the values of a, b and c from the example above and using the Newton-Raphson algorithm, we find with a first guess of 45∘ the following iterations, working in radians:
0.785 3980.417 8410.541 4990.557 7970.558 1040.558 104=31∘58′.6
The reader should verify this calculation, and, using a different first guess, show that NewtonRaphson iteration quickly leads to 78∘ 31′.5.
Having now cleared that small hurdle, the reader is invited to solve the spherical triangle problems below. Although these twelve problems look like pointless repetitive work, they are in fact all different. Some have two solutions between 0∘ and 360∘ ; others have just one. After solving each problem, the reader should sketch each triangle - especially those that have two solutions - in order to see how the two-fold ambiguities arise. The reader should also write a computer program that will solve all twelve types of problem at the bidding of the user. Answers should be given in degrees, minutes and tenths of a minute, and should be correct to that precision. For example, the answer to one of the problems is 47∘ 37′.3. An answer of 47∘ 37′.2 or 47∘ 37′.4 should be regarded as wrong. In celestial mechanics, there is no place for answers that are "nearly right". An answer is either right or it is wrong. (This does not mean, of course, that an angle can be measured with no error at all; but the answer to a calculation given to a tenth of an arcminute should be correct to a tenth of an arcminute.)
All angles and sides in degrees
10.a=64b=33c=37C=?11.a=39b=48C=74c=?12.a=16b=37C=42B=?13.a=21b=43A=29c=?14.a=67b=54A=39B=?15.a=49b=59A=14C=?16.A=24B=72c=19a=?17.A=79B=84c=12C=?18.A=62B=49a=44b=?19.A=59B=32a=62c=?20.A=47B=57a=22C=?21.A=79B=62C=48c=?
Solutions
10. 28∘18′.2
11. 49∘32′.4
12. 117∘31′.0
13. 30∘46′.7 or 47∘37′.3
14. 33∘34′.8
15. 3∘18′.1 or 162∘03′.9
16. 7∘38′.2
17. 20∘46′.6
18. 36∘25′.5
19. 76∘27′.7
20. 80∘55′.7 or 169∘05′.2
21. 28∘54′.6
Derivation of the formulas
Before moving on to further problems and applications of the formulas, it is time to derive the four formulas which, until now, have just been given without proof. We start with the cosine formula. There is no loss of generality in choosing rectangular axes such that the point A of the spherical triangle ABC is on the z-axis and the point B and hence the side c are in the zx-plane. The sphere is assumed to be of unit radius.
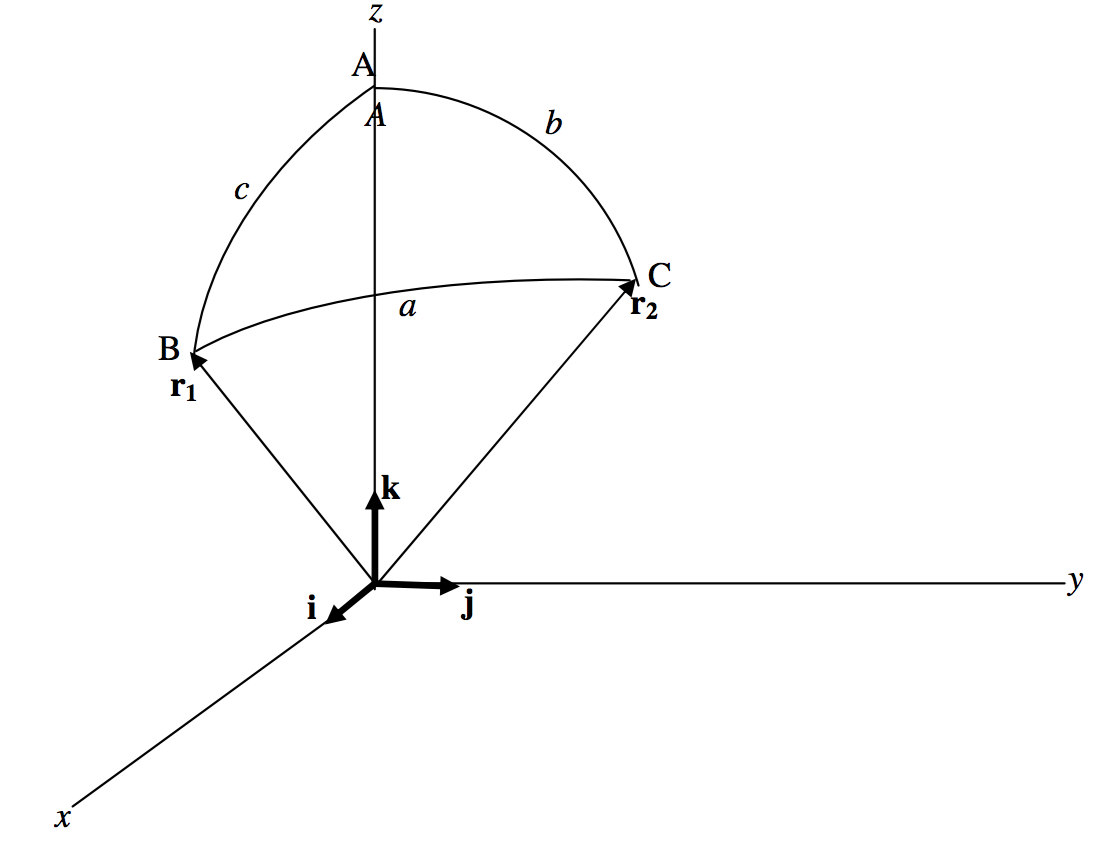
FIGURE III.14
If i, j and k are unit vectors directed along the x−, y− and z−axes respectively, inspection of the figure will show that the position vectors of the points B and C with respect to the centre of the sphere are
r1=isinc+kcosc
and r2=isinbcosA+jsinbsinA+kcosb
respectively.
The scalar product of these vectors (each of magnitude unity) is just the cosine of the angle between them, namely cosa, from which we obtain immediately
cosa=cosbcosc+sinbsinccosA.
To obtain the sine formula, we isolate cosA from this Equation, square both sides, and write 1−sin2A for cos2A. Thus,
(sinbsinccosA)2=(cosa−cosbcosc)2,
and when we have carried out these operations we obtain
sin2A=sin2bsin2c−cos2a−cos2bcos2c+2cosacosbcoscsin2bsin2c.
In the numerator, write 1−cos2b for sin2b and 1−cos2c for sin2c, and divide both sides by sin2a. This results in
sin2Asin2a=1−cos2a−cos2b−cos2c+2cosacosbcoscsin2asin2bsin2c.
At this stage the reader may feel that we are becoming bogged down in heavier and heavier algebra and getting nowhere. But, after a careful look at Equation 3.5.12, it may be noted with some delight that the next line is:
Therefore sinAsina=sinBsinb=sinCsinc.
The derivation of the polar cosine formula may also bring a small moment of delight. In figure III.15, A′B′C′ is a spherical triangle. ABC is also a spherical triangle, called the polar triangle to A′B′C′. It is formed in the following way. The side BC is an arc of a great circle 90∘ from A′; that is, BC is part of the equator of which A′ is pole. Likewise CA is 90∘ from B′ and AB is 90∘ from C′. In the drawing, the side B′C′ of the small triangle has been extended to meet the sides AB and CA of the large triangle. It will be evident from the drawing that the angle A of the large
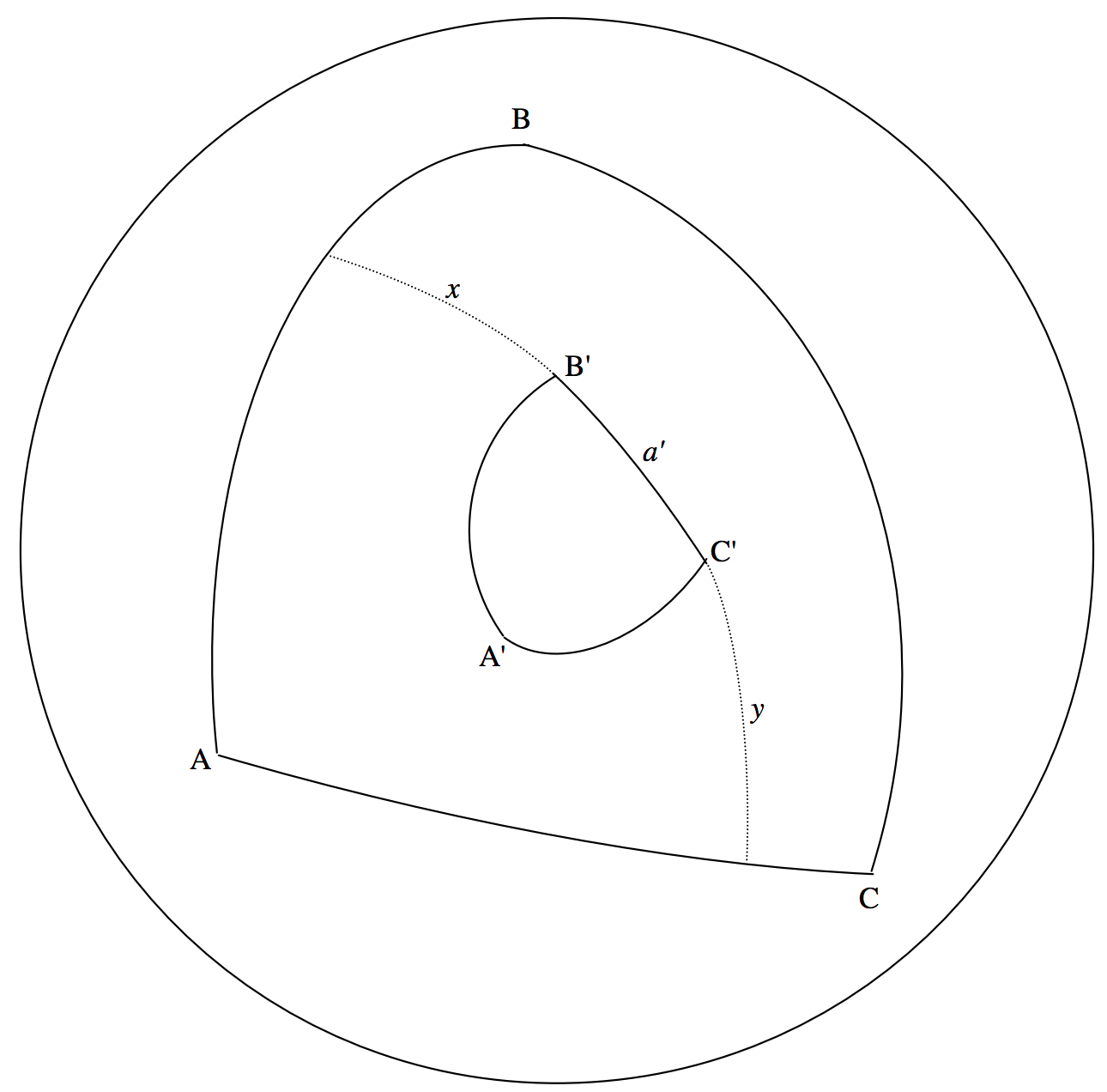
FIGURE III.15
triangle is equal to x+a′+y. Further, from the way in which the triangle ABC was formed, x+a′ and a′+y are each equal to 90∘. From these relations, we see that
A+A=[(x+a′)+y]+[x+(a′+y)]
or 2A=180∘+x+y=180∘+A−a′
Therefore A=180∘−a′
In a similar manner, B=180∘−b′ and C=180∘−c′
Now, suppose f(A′,B′,C′,a′,b′,c′)=0 is any relation between the sides and angles of the triangle A′B′C′. We may replace a′ by 180∘−A, b′ by 180∘−B, and so on, and this will result in a relation between A, B, C, a, b and c ; that is, it will result in a relation between the sides and angles of the triangle ABC.
For example, the Equation
cosa′=cosb′cosc′+sinb′sinc′cosA′
is valid for the triangle A′B′C′. By making these substitutions, we find the following formula valid for triangle ABC:
−cosA=cosBcosC−sinBsinCcosa,
which is the polar cosine formula.
The reader will doubtless like to try starting from the sine and cotangent formulas for the triangle A′B′C′ and deduce corresponding polar formulas for the triangle ABC, though this, unfortunately, may give rise to some anticlimactic disappointment.
I know of no particularly interesting derivation of the cotangent formula, and I leave it to the reader to work through the rather pedestrian algebra. Start from
cosa=cosbcosc+sinbsinccosA
and cosc=cosacosb+sinasinbcosC.
Eliminate cosc (but retain sinc) from these Equations, and write 1−sin2b for cos2b. Finally substitute sincsinAsinC for sina, and, after some tidying up, the cotangent formula should result.
At this stage, we have had some practice in solving the four spherical triangle formulas, and we have derived them. In this section we encounter examples in which the problem is not merely to solve a triangle, but to gain some experience in setting up a problem and deciding which triangle has to be solved.
The coordinates of the Dominion Astrophysical Observatory, near Victoria, British Columbia, are
Latitude 48∘31′.3N Longitude 123∘25′.0W
and the coordinates of the David Dunlap Observatory, near Toronto, Ontario, are
Latitude 43∘51′.8N Longitude 79∘25′.3W
How far is Toronto from Victoria, and what is the azimuth of Toronto relative to Victoria?
The triangle to be drawn and solved is the triangle PVT, where P is the Earth's north pole, V is Victoria, and T is Toronto. On figure III.16 are marked the colatitudes of the two cities and the difference between their longitudes.
The great circle distance ω between the two observatories is easily given by the cosine formula:
cosω=cos41∘28′.7cos46∘08′.2+sin41∘28′.7sin46∘08′.2cos43∘59′.7
From this, we find ω=30∘22′.7 or 0.53021 radians. The radius of the Earth is 6371 km, so the distance between the observatories is 3378 km or 2099 miles.
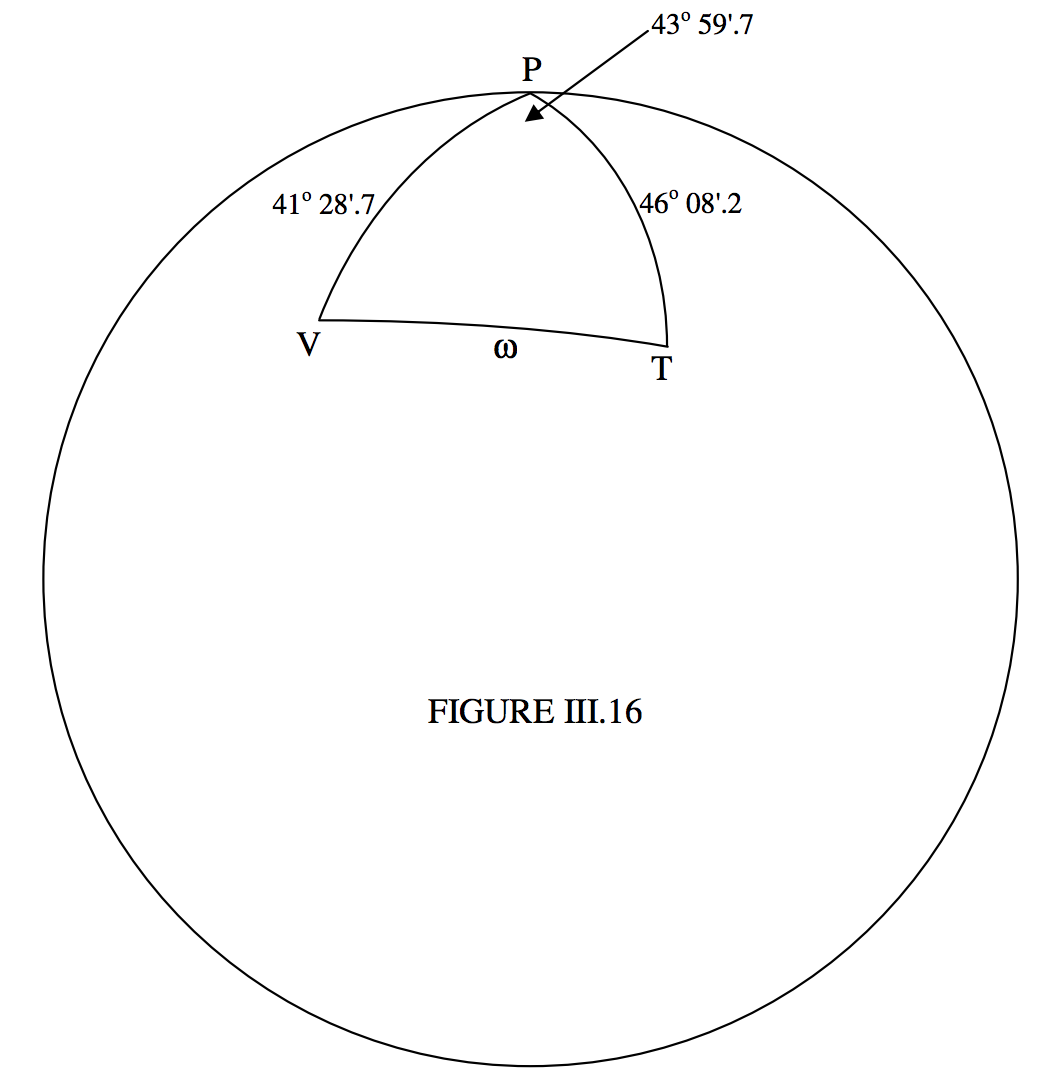
Now that we have found ω, we can find the azimuth, which is the angle V, from the sine formula:
sinV=sin46∘08′.2 sin43∘59′.7sin30∘22′.7=0.990 275
and hence V=82∘00′.3
But we should now remember that sin−10.990 275 has two values between 0∘ and 180∘, namely 82∘00′.3 and 97∘59′.7.
Usually it is obvious from inspection of a drawing which of the two values of sin−1 is the required one. Unfortunately, in this case, both values are close to 90∘, and it may not be immediately obvious which of the two values we require. However, it will be noticed that Toronto has a more southerly latitude than Victoria, and this should easily resolve the ambiguity.
We could, of course, have found the azimuth V by using the cotangent formula, without having to calculate ω first. Thus
cos41∘28′.7cos43∘59′.7=sin41∘28′.7cot46∘08′.2−sin43∘59′.7cotV
There is only one solution for V between 0∘ and 180∘, and it is the correct one, namely 82∘00′.3. A good drawing will show the reader why the correct solution was the acute rather than the obtuse angle (in our drawing the angle was made to be close to 90∘ in order not to bias the reader one way or the other), but in any case all readers, especially those who were trapped into choosing the obtuse angle, should take careful note of the difficulties that can be caused by the ambiguity of the function sin−1. Indeed it is the strong advice of the author never to use the sine formula, in spite of the ease of memorizing it. The cotangent formula is more difficult to commit to memory, but it is far more useful and not so prone to quadrant mistakes.
Consider two points, A and B, at latitude 20∘N, longitude 25∘E, and latitude 72∘N, longitude 44∘E. Where are the poles of the great circle passing through these two points? We shall present two methods of doing the problem. First, by solving spherical triangles. And second, kindly suggested to me by Achintya Pal, using the methods of algebraic coordinate geometry.
Let us call the colatitude and longitude of the first point (θ1,ϕ1) and of the second point (θ2,ϕ2) We shall consider the question answered if we can find the coordinates (θ0,ϕ0) of the poles Q and Q′ of the great circle passing through the two points. In figure III.17, P is the north pole of the Earth, A and B are the two points in question, and Q is one of the two poles of the great circle joining A and B. The figure also shows the triangle PQA. We’ll suppose that the origin for longitudes (“Greenwich”) is behind the plane of the paper. The east longitudes of Q, A and B are, respectively, ϕ0,ϕ1,ϕ2; and their colatitudes are θ0,θ1,θ2.
0=cosθ0cosθ1+sinθ0sinθ1cos(ϕ1−ϕ0),
from which
tanθ0=−1tanθ1cos(ϕ1−ϕ0).
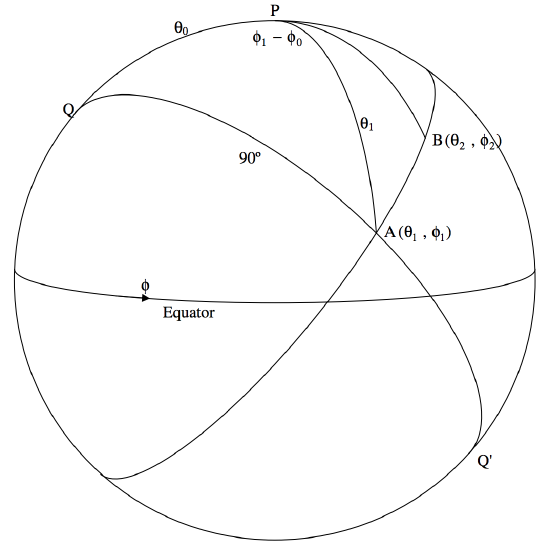
FIGURE III.17
Similarly from triangle PQB we would obtain
tanθ0=−1tanθ2cos(ϕ2−ϕ0).
These are two Equations in θ0 and ϕ0, so the problem is in principle solved. Equate the righthand sides of the two Equations, expand the terms cos(ϕ1−ϕ0) and cos(ϕ2−ϕ0), gather the terms in sinϕ0 and cosϕ0, eventually to obtain
tanϕ0=tanθ1cosϕ1−tanθ2cosϕ2tanθ2sinϕ2−tanθ1sinϕ1.
If we substitute the angles given in the original problem, we obtain
tanϕ0=tan70∘cos25∘−tan18∘cos44∘tan18∘sin44∘−tan70∘sin25∘=−2.412 091 0
from which ϕ0=112∘31′.1or292∘31′.1
Note that we get two values for ϕ0 differing by 180∘, as expected.
We then use either of the Equations for tanθ0 to obtain θ0 (It is good practice to use both of them as a check on the arithmetic.) The north polar distance, or colatitude, must be between 0∘ and 180∘, so there is no ambiguity of quadrant.
With ϕ0=112∘31′.1, we obtain θ0=96∘47′.1, i.e. latitude 6∘47′.1 S.
and with ϕ0=292∘31′.1, we obtain θ0=83∘12′.9, i.e. latitude 6∘47′.1 N.
and these are the coordinates of the two poles of the great circle passing through A and B. The reader is strongly urged actually to carry out these computations numerically in order to be quite sure that the quadrants are correct and unambiguous. Indeed, dealing with the quadrant problem may be regarded as the most important part of the exercise.
We arrived at Equation 3.5.17 and 3.5.18 by solving two spherical triangles by the methods of spherical trigonometry. The second method, suggested, as mentioned above, by Achintya Pal, uses the methods of algebraic coordinate geometry in three dimensions to arrive at the same Equations. We refer coordinates to axes Oxyz. O is the centre of the Earth, taken to be of unit radius. OP is the z-axis. The Ox and Oy axes are not drawn in figure III.17, but the x-axis may be taken to be directed somewhere to the rear of the drawing (away from the reader), and the y-axis somewhere in the front of the drawing, both being, of course, in the plane of the equator.
Let us write the Equation to the plane containing A and B in the form
lx+my+nz=0
Here (l,m,n) are the direction cosines of the normal to the plane AB, and are given by
l=sinθ0cosϕ0m=sinθ0sinϕ0n=cosθ0
The (x,y,z) coordinates of the point A are
x=sinθ1cosϕ1y=sinθ1sinϕ1x=cosθ1
On substitution of Equations 3.5.21a,b,c and 3.5.22a,b,c into Equation 3.5.20 we obtain:
sinθ0cosϕ0sinθ1cosϕ1+sinθ0sinϕ0sinθ1sinϕ1+cosθ0cosθ1=0
After some very modest algebraic manipulation (e.g., start by dividing by sinθ1cosθ0) we very soon arrive again at Equation 3.5.17, and in a similar manner at Equation 3.5.18.
As a bonus we note that any point having spherical coordinates (θ,ϕ) lying on the great circle whole pole is at (θ0,ϕ0) satisfies the Equation
cotθ=−tanθ0cos(ϕ−ϕ)
This Equation may be regarded as the (θ,ϕ) Equation to the great circle AB, and it answers the problem converse to the one originally posed: What is the Equation to the great circle whose pole is at (θ0,ϕ0) ?
Here is a challenging exercise and an important one in meteor astronomy. Two shower meteors are seen, diverging from a common radiant. One starts at right ascension 6 hours, declination +65 degrees, and finishes at right ascension 1 hour, declination +75 degrees. The second starts at right ascension 5 h, declination +35 degrees, and finishes at right ascension 3 hours, declination +15 degrees. Where is the radiant?
The assiduous student will make a good drawing of the celestial sphere, illustrating the situation as accurately as possible. The calculation will require some imaginative manipulation of spherical triangles. After arriving at what you believe to be the correct answer, look at your drawing to see whether it is reasonable. The next step might be to develop a general trigonometrical expression for the answer in terms of the original data, or to program the calculation for a computer, so that it is henceforth available for any similar calculation. Or one can go yet further, and write a computer program that will give a least-squares solution for the radiant for many more than two meteors in the shower. I find for the answer to the above problem that the radiant is at right ascension 7.26 hours and declination +43.8 degrees.
Uniqueness of Solutions
The reader who has by now worked through a variety of problems in the solution of a triangle will have noticed that, given three elements of a triangle, sometimes there is a unique solution, whereas sometimes there are two possible triangles that satisfy the original data. Yet again, it may sometimes be found that there is no possible solution, meaning that there is no possible triangle that satisfies the given data, which must therefore be presumed incorrect. I am very much indebted to Alan Johnstone for lengthy discussions on this problem, and indeed for pointing out that some of the “solutions” given in an earlier version of these notes were in fact invalid (and have now been corrected). I believe the following criteria determine how many valid solutions there are for a given triplet of data, for plane triangles and for spherical triangles.
We may be given three elements of a triangle,
Thus
- Three sides: a,b,c,
- Two sides and the included angle: b,c,A.
- Two sides and a nonincluded angle: a,b,A.
- Two angles and a common side: a,B,C.
- Two angles and another side: A,B,a.
- Three angles: A,B,C.
Question:
Which of these give a unique solution, and which admit of two solutions? And which are impossible triangles? I believe the answers are as follows:
Plane Triangles
i. Let d=a+b−c,e=b+c−a,f=c+a−b
For a valid triangle, d, e and f must all be positive. If so, there is a unique solution.
ii. There is a unique solution.
iii. If a>b there is a unique solution.
If a=b, there is a unique solution if A<90∘. Otherwise there is no valid triangle.
If a<b there are zero, one or two solutions, according as to whether
sinA>ab,sinA=ab or sinA<ab.
iv. There is a unique solution.
v. There is a unique solution.
vi. There is a unique solution except that only the relative lengths of the sides are determined.
Spherical Triangles
i. Let d=a+b−c,e=b+c−a,f=c+a−b
For a valid triangle, d, e and f must all be positive. If so, there is a unique solution.)
ii. There is a unique solution.
iii. If sinA>sinasinb, there is no real solution.
If A=a=b=90∘, then B=90∘, and c and C are equal but indeterminate.
Otherwise:
If a>b there is a unique solution.
If a=b, there is a unique solution if a<90∘. Otherwise there is no real solution.
If a<b there are one or two solutions, according as to whether
sinA=sinasinb or sinA<sinasinb.
iv. There is a unique solution.
v. If sinA=sinAsinB, there is no real solution.
If A=B=a=90∘, then b=90∘, and c and C are equal but indeterminate.
Otherwise:
If A>B there is a unique solution.
If A=B, there is a unique solution if a<90∘. Otherwise there is no real solution.
If A<B there are one or two solutions, according as to whether
sina=sinAsinB or sina<sinAsinB.


