11.1: Heat Death
( \newcommand{\kernel}{\mathrm{null}\,}\)
The Second Law of Thermodynamics Revisited
The Second Law of Thermodynamics introduced in the previous chapters states that thermal energy will always spontaneously transfer from higher temperature to lower temperature. In order to aid in our study of other thermodynamic processes, such as phase changes, a more general version of the Second Law can be stated in terms of energy concentration and dispersion: any spontaneous process must move an isolated system toward a state of more uniform dispersion of energy throughout the system. By isolated system we mean one for which energy does not leave or enter. For example, we know that higher temperature means a greater average thermal energy per molecule, so we can think of temperature as a measure of the concentration of thermal energy in an object. If we consider a hot object in a cold room as our complete system, then the thermal energy in our system is not very well dispersed because it’s more concentrated in the hot object. The Second Law of thermodynamics predicts that energy should move from the hot object to the cold environment to better disperse the energy, and that is what we observe. However, the heat transfer will only occur until the thermal energy is maximally dispersed, which corresponds to thermal equilibrium, and is indicated by the object and environment having the same temperature.
Everyday Examples: Sweating, Dew, and Rain
When we sweat to exhaust thermal energy by evaporation we aren’t actively grabbing the hottest water molecules, pulling then away from their neighbors, and throwing them into the gas phase. The evaporation happens spontaneously because thermal energy stored in water molecules that are stuck together is relatively concentrated compared to thermal energy stored in water molecules zipping around in the air and free to disperse. The transfer of thermal energy to sweat (by conduction), followed by evaporation is a spontaneous process because it increases the dispersion of energy throughout the system made up of you, the sweat, and the surrounding air. Therefore, evaporation of sweat is a spontaneous process.
When the relative humidity reaches 100% then evaporation has maximally dispersed the available thermal energy. Any additional evaporation would begin to over-concentrate energy in the air and decrease the overall level of energy dispersion. Therefore, we don’t see evaporation occurring once 100% humidity is reached. In fact if the humidity gets pushed above 100% (by a drop in air temperature without a loss of water vapor) then energy is over-concentrated in the air and thus increasing dispersion of energy requires that water molecules come out of the vapor phase and condensation occurs spontaneously. When the liquid condenses on surfaces we call it dew, when the liquid condenses on particles in the air and falls to the ground we call it rain.
Reinforcement Exercise
You place -10 °C ice in warm room. Will thermal energy flow from room air to ice, or from ice to warm air? Explain in terms of thermal energy concentration and dispersion and the Second Law of Thermodynamics.
When the ice warms up to 0 °C it begins to melt instead of increase temperature. Based on the Second Law of Thermodynamics, which of the following processes provides more ways of dispersing thermal energy through the ice and air?
- Thermal energy is removed from the warm room and stored as kinetic energy (vibrations) in ice molecules, which increases the ice temperature.
- Thermal energy is removed from the warm room and stored as kinetic energy in vibrations and rotations of liquid water molecules, which requires melting the ice instead of raising its temperature.
Entropy
Entropy (S) is a measure of energy dispersion within a system. An increase in the entropy corresponds to an increase in dispersion of energy. A decrease in entropy would correspond to energy being less dispersed, or increasing energy concentration. Therefore the Second Law of Thermodynamics can also be stated as: a process will happen spontaneously if it increases the total entropy of an isolated system. The change in entropy for a constant-temperature process can be calculated from the heat transferred (Q) and the temperature at which the transfer occurs (T) as:
ΔS=QT
Notice that we definitely need to use the Kelvin absolute temperature scale when working with the change in entropy equation, or else we might find ourselves attempting to divide by zero. Let’s apply this equation and the entropy version of the Second Law of Thermodynamics to the second part of the previous Reinforcement Exercise.
Example 11.1.1: Entropy Change of Melting
If you place ice in a warm room and leave it alone, it will melt. The ice melting would be a spontaneous process because it will happen all on it’s own, so we should find that melting increases the total entropy (ΔS > 0). Let’s check that out. We’ll keep it simple and calculate change in entropy of one kilogram of ice, which melts at 0 °C, or 273 K.
Next we calculate the change in entropy for the room. The same heat that went into melting the ice came out of the room, so the Q for the room is the same as for the ice, only negative. Let’s pick a typical room temperature of 20 °C for our example, which is 293 K:
ΔSroom=Q/T=−mLfT=−(1kg)(334,000J/kg)293K=−1140J/K
Now we just add up the two entropy changes to get the total:
ΔST=ΔSice+ΔSroom=1223J/K+(−1140)J/K=17J/K
Our entropy change is greater than zero, so the Second Law states that ice melting in warm room is a spontaneous process, which we observe it to be.
Maximum Efficiency
Spontaneous processes are inherently irreversible. For example, we could not reverse the spontaneous process of thermal energy transfer from your body to sweat and then to the environment by evaporation. Imagine trying to run around and grab all the water vapor molecules and shove them back into the liquid on our skin and then make those water molecules collide with skin molecules in just the right way to conduct thermal energy back into your body! Good luck. If the process could be reversed, the net entropy change would be zero, but that is not a real possibility. Any real process increases the entropy because irreversible at some level, meaning energy is further dispersed throughout the system without a realistic opportunity to put it back where it was. We have arrived at yet another version of the Second Law of Thermodynamics: any real process increases the total entropy of the universe. For example, even if you could reverse the evaporation process we described above, the exhaust heat you released during that running around would decrease the concentration of energy in your body and disperse it throughout the room.The system, which includes you, would not cause have returned to the original conditions at all. In fact, the total entropy would have increased by even more than before you tried to reverse the process. For example, as you ran around, more sweat would have evaporated and you would have to chase those molecules down as well, and so on–you could never win! We can’t keep the entropy of the universe from increasing.
Everyday Examples: Human Mechanical Efficiency
Muscle contraction relies on the release of chemical potential energy stored in ATP molecules. Before contraction, that energy is concentrated into certain molecules in certain areas of a muscle cell. When that energy is released, the entropy of the molecules decreases, so the entropy of their environment must increase by more, and this is achieved because most of the energy released from the ATP molecules is degraded to heat and distributed to the environment. After contraction, a muscle cell must be reorganized, which decreases entropy, but we know overall entropy must increase for any real process, so some additional thermal energy must be dispersed to the environment during reorganization in order to provide the necessary entropy increase. All of this thermal energy is “wasted” because it is came from stored chemical potential energy, but is not available for use by the body to do work. Therefore, entropy and the Second Law of Thermodynamics limit the efficiency of the human body.[1]
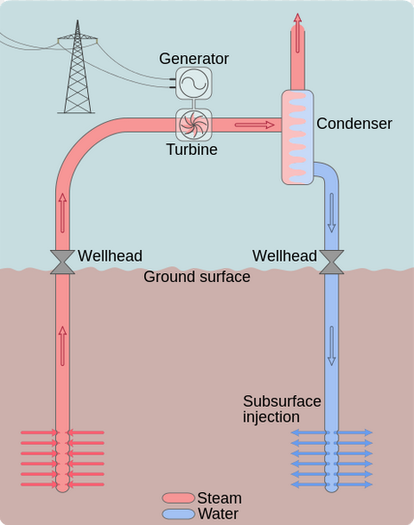
Everyday Example: Geothermal Heat Engine
Let’s imagine molten rock from the Earth’s mantle pushes partway through the Earth’s crust, and keeps a region of bedrock at a constant temperature of 300 °C . If the rock was not too deep, we could install pipes in the rock, and then boil water by running it through the pipes. We would basically have a giant pressure cooker! Rather than cook food, we could release the pressurized steam to push on a piston or spin a turbine. After releasing the pressure and getting some work out, we would be left with lower pressure steam. We could condense the steam back to water by running it through pipes exposed to the 20 °C air above ground. Thermal energy would transfer from the steam to the air as exhaust heat, the steam would condense into liquid water, and we could start again.
Machines like the one described that convert thermal energy into mechanical energy are called a heat engines. Your car is powered by an internal combustion heat engine. Let’s see how entropy and the Second Law of Thermodynamics determine the efficiency of our geothermal heat engine.
First we calculate the entropy change when 1000 J of thermal energy transfers out of the rock to the water to run the engine, remembering to convert the 300 °C rock temperature to Kelvin by adding 273 K:



The First Law of Thermodynamics told us that you cannot build an engine that is more than 100 % efficient because energy cannot be created. Even worse, the Second Law tells us that even if we managed to eliminate all mechanical inefficiencies, such as friction, we still can’t get up to 100 % because all engines must exhaust some energy in order to increase entropy overall. The theoretical maximum efficiency, which is always less than 100 %, is known as the Carnot efficiency (ec) and depends only on the high and low operating temperatures (TH and TL), as we saw in the previous example. Most of the work we did in the previous example can be short-cut by the equation for Carnot Efficiency (ec):

Reinforcement Exercise: Carnot Efficiency
Check that the previous short-cut equation gives the correct maximum efficiency for our geothermal heat engine.
What is the Carnot efficiency of our geothermal heat engine if the hot rock was actually at 550 C° instead of 300 C° ?
You will find that this engine is more efficient just because the hot operating temperature higher, even though it still works in the same way and nothing else has changed. The efficiency increased because the input energy started out more concentrated and less dispersed (indicated by higher temperature), so less of that energy had to become dispersed in the environment, or wasted, in order to ensure that entropy increased by a sufficient amount to satisfy the Second Law of Thermodynamics. Thermal energy that starts out concentrated (at high temperature) is known as high quality energy.
Reinforcement Exercise:
A particular internal combustion engine in a car operates between the temperature of the hot combustion gasses immediately after combustion, and the outside air temperature. These are typically 1000 K and 290 K. What is the theoretical maximum efficiency of such engines?
Actual automobile engines have efficiencies that are about 75 % of the maximum theoretical efficiency. Roughly how efficient are actual automobile engines?
Heat Death
In addition to limiting our efficiency in doing mechanical work, the Second Law drives our bodies toward higher entropy, which means thermal equilibrium with the environment. Unless the environmental temperature happens to be near body temperature, reaching thermal equilibrium means death. Life also requires concentration of chemical potential energy, but due to the the Second Law we tend toward chemical equilibrium, which is not survivable. Concentrations of electrical energy drive your nervous system, but due to the Second Law we are constantly at risk of reaching an internal electrical equilibrium with no electrical activity. Life is a constant battle against various types of equilibrium that would correspond to maximum entropy, but also to death. The work necessary to fight off our own entropy increase is what we consider basic metabolism. Doing that work, and even taking in the energy required to do that work, involves real processes that provide even more opportunity for entropy increases in a seemingly viscous cycle. You can’t beat the Second Law of Thermodynamics! Even as we manage to prevent our own entropy increase we cause the entropy of the environment to increase by a greater amount than what we prevented in ourselves. In fact, a complete dispersion of energy, so that all matter is at equilibrium, and no processes remain which would increase the entropy, and nothing really happens all, is one possible fate of the universe which has been dubbed heat death. At least we don’t expect heat death of the universe to occur for at least 10100 years. [3]
- "Thermodynamics of Skeletal Muscle Fiber: Do We Need to Redefine "Active" and "Resting" States?" by I. Y. Cristlieb and E. Cesarman, Cadiovascular and Pulmonary Research Center, The Medical College of Pennsylvania/Hahnemann University↵
- "Carnot heat engine" by Wikipedia↵
- OpenStax, College Physics. OpenStax CNX. Mar 6, 2019 http://cnx.org/contents/031da8d3-b525-429c-80cf-6c8ed997733a@14.78↵