6.2: Parallel Plate Waveguide- Introduction
( \newcommand{\kernel}{\mathrm{null}\,}\)
A parallel plate waveguide is a device for guiding the propagation of waves between two perfectly-conducting plates. Our primary interest in this structure is as a rudimentary model applicable to a broad range of engineering problems. Examples of such problems include analysis of the fields within microstrip line and propagation of radio waves in the ionosphere.
Figure 6.2.1 shows the geometry of interest. Here the plates are located at z=0 and z=a. The plates are assumed to be infinite in extent, and therefore there is no need to consider fields in the regions z<0 or z>a. For this analysis, the region between the plates is assumed to consist of an ideal (lossless) material exhibiting real-valued permeability μ and real-valued permittivity ϵ.
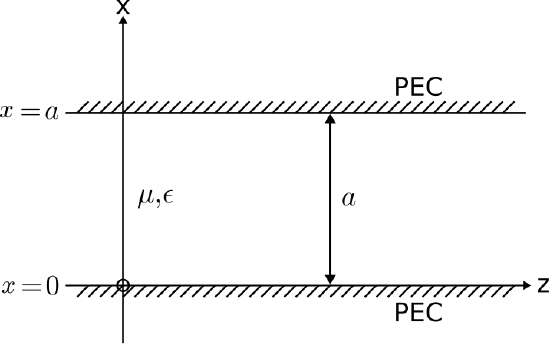 Figure 6.2.1: Geometry for analysis of fields in a parallel plate waveguide (CC BY-SA 4.0 (modified))
Figure 6.2.1: Geometry for analysis of fields in a parallel plate waveguide (CC BY-SA 4.0 (modified))
Let us limit our attention to a region within the waveguide which is free of sources. Expressed in phasor form, the electric field intensity is governed by the wave equation
∇2˜E+β2˜E=0
where
β=ω√μϵ
Equation ??? is a partial differential equation. This equation, combined with boundary conditions imposed by the perfectly-conducting plates, is sufficient to determine a unique solution. This is most easily done in Cartesian coordinates, as we shall now demonstrate. First we express ˜E in Cartesian coordinates:
˜E=ˆx˜Ex+ˆy˜Ey+ˆz˜Ez
This facilitates the decomposition of Equation ??? into separate equations governing the ˆx, ˆy, ˆz components of ˜E:
∇2˜Ex+β2˜Ex=0∇2˜Ey+β2˜Ey=0∇2˜Ez+β2˜Ez=0
Next we observe that the operator ∇2 may be expressed in Cartesian coordinates as follows:
∇2=∂2∂x2+∂2∂y2+∂2∂z2
so the equations governing the Cartesian components of ˜E may be written as follows:
∂2∂x2˜Ex+∂2∂y2˜Ex+∂2∂z2˜Ex=−β2˜Ex∂2∂x2˜Ey+∂2∂y2˜Ey+∂2∂z2˜Ey=−β2˜Ey∂2∂x2˜Ez+∂2∂y2˜Ez+∂2∂z2˜Ez=−β2˜Ez
Let us restrict our attention to scenarios that can be completely described in two dimensions; namely x and z, and for which there is no variation in y. This is not necessarily required, however, it is representative of a broad class of relevant problems, and allows Equations 6.2.9-6.2.11 to be considerably simplified. If there is no variation in y, then partial derivatives with respect to y are zero, yielding:
∂2∂x2˜Ex+∂2∂z2˜Ex=−β2˜Ex∂2∂x2˜Ey+∂2∂z2˜Ey=−β2˜Ey∂2∂x2˜Ez+∂2∂z2˜Ez=−β2˜Ez
The solution to the parallel plate waveguide problem may now be summarized as follows: The electric field ˜E (Equation ???) is the solution to simultaneous Equations 6.2.13-6.2.15 subject to the boundary conditions that apply at the PEC surfaces at z=0 and z=d; namely, that the tangent component of ˜E is zero at these surfaces.
At this point, the problem has been reduced to a routine exercise in the solution of partial differential equations. However, a somewhat more useful approach is to first decompose the total electric field into transverse electric (TE) and transverse magnetic (TM) components,1 and determine the solutions to these components separately. The TE component of the electric field is parallel to the plates, and therefore transverse (perpendicular) to the plane shown in Figure 6.2.1. Thus, the TE component has ˜Ex=˜Ez=0, and only ˜Ey may be non-zero. The TM component of the magnetic field intensity (˜H) is parallel to the plates, and therefore transverse to the plane shown in Figure 6.2.1. Thus, the TM component has ˜Hx=˜Hz=0, and only ˜Hy may be non-zero. As always, the total field is the sum of the TE and TM components.
The TE and TM solutions are presented in Sections 6.3 (Electric component of the TE solution), 6.4 (Magnetic component of the TE solution), and 6.5 (Electric component of the TM solution). The magnetic component of the TM solution can be determined via a straightforward variation of the preceding three cases, and so is not presented here.
Presentation of the solution for the fields in a parallel plate waveguide under the conditions described in the section continues in Section 6.3.
- For a refresher on TE-TM decomposition, see Section 5.5.↩


