2.8B: Power of a Refracting Interface
( \newcommand{\kernel}{\mathrm{null}\,}\)
Figure II.12 shows a refracting interface of radius of curvature r separating media of indices n1 and n2.
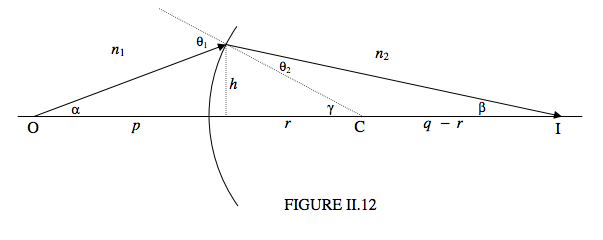
I show a real object at O, a real image at I and the centre of curvature at C. Remember that angles are small and the “lens” is thin. We see that h=αp=βq=γr. By Euclid, θ1=α+γ and θ2=γ−β, and by Snell, n1θ1=n2θ2. From these we obtain
n2q=−n1p+n2−n1r.
Thus the power is n2−n1r. The reader should try this for other situations (virtual object, virtual image, concave interface, and so on) to see that you always get the same result.


