13.2: Vertical spring-mass system

Consider the vertical spring-mass system illustrated in Figure \(\PageIndex{1}\).

Figure \(\PageIndex{1}\): A vertical spring-mass system.

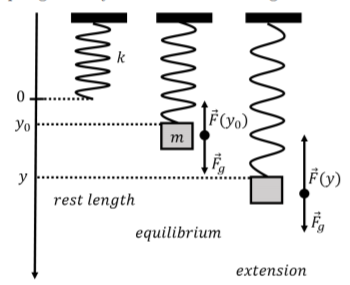
When no mass is attached to the spring, the spring is at rest (we assume that the spring has no mass). We choose the origin of a one-dimensional vertical coordinate system (\(y\) axis) to be located at the rest length of the spring (left panel of Figure \(\PageIndex{1}\)). When a mass \(m\) is attached to the spring, the spring will extend and the end of the spring will move to a new equilibrium position, \(y_0\), given by the condition that the net force on the mass \(m\) is zero. The only forces exerted on the mass are the force from the spring and its weight. The condition for the equilibrium is thus:

\[\begin{aligned} \sum F_y = F_g - F(y_0) &=0\\[4pt] mg - ky_0 &= 0 \\[4pt] \therefore mg &= ky_0\end{aligned}\]

Now, consider the forces on the mass at some position \(y\) when the spring is extended downwards relative to the equilibrium position (right panel of Figure \(\PageIndex{1}\)). Newton’s Second Law at that position can be written as:

\[\begin{aligned} \sum F_y = mg - ky &= ma\\[4pt] \therefore m \frac{d^2y}{dt^2}& = mg - ky \end{aligned}\]

Note that the net force on the mass will always be in the direction so as to “restore” the position of the mass back to the equilibrium position, \(y_0\). If the mass had been moved upwards relative to \(y_0\), the net force would be downwards.

We can substitute the equilibrium condition, \(mg = ky_0\), into the equation that we obtained from Newton’s Second Law:

\[\begin{aligned} m \frac{d^2y}{dt^2}& = mg - ky \\[4pt] m \frac{d^2y}{dt^2}&= ky_0 - ky\\[4pt] m \frac{d^2y}{dt^2}&=-k(y-y_0) \\[4pt] \therefore \frac{d^2y}{dt^2} &= -\frac{k}{m}(y-y_0)\end{aligned}\]

Consider a new variable, \(y'=y-y_0\). This is the same as defining a new \(y'\) axis that is shifted downwards by \(y_0\); in other words, this the same as defining a new \(y'\) axis whose origin is at \(y_0\) (the equilibrium position) rather than at the position where the spring is at rest. Noting that the second time derivative of \(y'(t)\) is the same as that for \(y(t)\):

\[\begin{aligned} \frac{d^2y}{dt^2} &= \frac{d^2}{dt^2} (y' + y_0) = \frac{d^2y'}{dt^2}\\[4pt]\end{aligned}\]

we can write the equation of motion for the mass, but using \(y'(t)\) to describe its position:

\[\begin{aligned} \frac{d^2y'}{dt^2} &= \frac{k}{m}y'\end{aligned}\]

This is the same equation as that for the simple harmonic motion of a horizontal spring-mass system (Equation 13.1.2), but with the origin located at the equilibrium position instead of at the rest length of the spring. In other words, a vertical spring-mass system will undergo simple harmonic motion in the vertical direction about the equilibrium position. In general, a spring-mass system will undergo simple harmonic motion if a constant force that is co-linear with the spring force is exerted on the mass (in this case, gravity). That motion will be centered about a point of equilibrium where the net force on the mass is zero rather than where the spring is at its rest position.

Exercise \(\PageIndex{1}\)

How does the period of motion of a vertical spring-mass system compare to the period of a horizontal system (assuming the mass and spring constant are the same)?

1. The period of the vertical system will be larger.
2. The period of the vertical system will be smaller.
3. The period will be the same.

Answer

Two-spring-mass system

Consider a horizontal spring-mass system composed of a single mass, \(m\), attached to two different springs with spring constants \(k_1\) and \(k_2\), as shown in Figure \(\PageIndex{2}\).

Figure \(\PageIndex{2}\): A mass attached to two different springs.

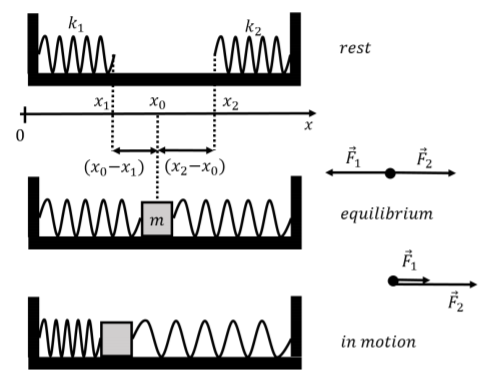
We introduce a horizontal coordinate system, such that the end of the spring with spring constant \(k_1\) is at position \(x_1\) when it is at rest, and the end of the \(k_2\) spring is at \(x_2\) when it is as rest, as shown in the top panel. A mass \(m\) is then attached to the two springs, and \(x_0\) corresponds to the equilibrium position of the mass when the net force from the two springs is zero. We will assume that the length of the mass is negligible, so that the ends of both springs are also at position \(x_0\) at equilibrium. You can see in the middle panel of Figure \(\PageIndex{2}\) that both springs are in extension when in the equilibrium position. It is possible to have an equilibrium where both springs are in compression, if both springs are long enough to extend past \(x_0\) when they are at rest.

If we assume that both springs are in extension at equilibrium, as shown in the figure, then the condition for equilibrium is given by requiring that the sum of the forces on the mass is zero when the mass is located at \(x_0\). The extension of the spring on the left is \(x_0 - x_1\), and the extension of the spring on the right is \(x_2-x_0\):

\[\begin{aligned} \sum F_x = -k_1(x_0-x_1) + k_2 (x_2 - x_0) &= 0\\[4pt] -k_1x_0+k_1x_1+k_2x_2-k_2x_0 &=0\\[4pt] -(k_1+k_2)x_0 +k_1x_1+k_2x_2 &=0\\[4pt] \therefore k_1x_1+k_2x_2 &=(k_1+k_2)x_0\end{aligned}\]

Note that if the mass is displaced from \(x_0\) in any direction, the net force on the mass will be in the direction of the equilibrium position, and will act to “restore” the position of the mass back to \(x_0\).

When the mass is at some position \(x\), as shown in the bottom panel (for the \(k_1\) spring in compression and the \(k_2\) spring in extension), Newton’s Second Law for the mass is:

\[\begin{aligned} -k_1(x-x_1) + k_2 (x_2 - x) &= m a \\[4pt] -k_1x +k_1x_1 + k_2 x_2 - k_2 x &= m \frac{d^2x}{dt^2}\\[4pt] -(k_1+k_2)x + k_1x_1 + k_2 x_2&= m \frac{d^2x}{dt^2}\end{aligned}\]

Note that, mathematically, this equation is of the form \(-kx + C =ma\), which is the same form of the equation that we had for the vertical spring-mass system (with \(C=mg\)), so we expect that this will also lead to simple harmonic motion. We can use the equilibrium condition (\(k_1x_1+k_2x_2 =(k_1+k_2)x_0\)) to re-write this equation:

\[\begin{aligned} -(k_1+k_2)x + k_1x_1 + k_2 x_2&= m \frac{d^2x}{dt^2}\\[4pt] -(k_1+k_2)x + (k_1+k_2)x_0&= m \frac{d^2x}{dt^2}\\[4pt] \therefore -(k_1+k_2) (x-x_0) &= m \frac{d^2x}{dt^2}\end{aligned}\]

Let us define \(k=k_1+k_2\) as the “effective” spring constant from the two springs combined. We can also define a new coordinate, \(x' = x-x_0\), which simply corresponds to a new \(x\) axis whose origin is located at the equilibrium position (in a way that is exactly analogous to what we did in the vertical spring-mass system). We can thus write Newton’s Second Law as:

\[\begin{aligned} -(k_1+k_2) (x-x_0) &= m \frac{d^2x}{dt^2}\\[4pt] -kx' &= m \frac{d^2x'}{dt^2}\\[4pt] \therefore \frac{d^2x'}{dt^2} &= -\frac{k}{m}x'\end{aligned}\]

and we find that the motion of the mass attached to two springs is described by the same equation of motion for simple harmonic motion as that of a mass attached to a single spring. In this case, the mass will oscillate about the equilibrium position, \(x_0\), with a an effective spring constant \(k=k_1+k_2\). Combining the two springs in this way is thus equivalent to having a single spring, but with spring constant \(k=k_1+k_2\). The angular frequency of the oscillations is given by:

\[\begin{aligned} \omega = \sqrt{\frac{k}{m}}=\sqrt{\frac{k_1+k_2}{m}}\end{aligned}\]