Skip to main content
Physics LibreTexts

5.E: Practice

  1. Last updated
  2. Save as PDF
  • Page ID
    68762

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    Conceptual Questions

    6.2 Friction

    1. The glue on a piece of tape can exert forces. Can these forces be a type of simple friction? Explain, considering especially that tape can stick to vertical walls and even to ceilings.
    2. When you learn to drive, you discover that you need to let up slightly on the brake pedal as you come to a stop or the car will stop with a jerk. Explain this in terms of the relationship between static and kinetic friction.
    3. When you push a piece of chalk across a chalkboard, it sometimes screeches because it rapidly alternates between slipping and sticking to the board. Describe this process in more detail, in particular, explaining how it is related to the fact that kinetic friction is less than static friction. (The same slip-grab process occurs when tires screech on pavement.)
    4. A physics major is cooking breakfast when she notices that the frictional force between her steel spatula and Teflon frying pan is only 0.200 N. Knowing the coefficient of kinetic friction between the two materials, she quickly calculates the normal force. What is it?

    Problems

    6.2 Friction

    1. (a) When rebuilding his car’s engine, a physics major must exert 3.00 x 102 N of force to insert a dry steel piston into a steel cylinder. What is the normal force between the piston and cylinder? (b) What force would he have to exert if the steel parts were oiled?
    2. (a) What is the maximum frictional force in the knee joint of a person who supports 66.0 kg of her mass on that knee? (b) During strenuous exercise, it is possible to exert forces to the joints that are easily 10 times greater than the weight being supported. What is the maximum force of friction under such conditions? The frictional forces in joints are relatively small in all circumstances except when the joints deteriorate, such as from injury or arthritis. Increased frictional forces can cause further damage and pain.
    3. Suppose you have a 120-kg wooden crate resting on a wood floor, with coefficient of static friction 0.500 between these wood surfaces. (a) What maximum force can you exert horizontally on the crate without moving it? (b) If you continue to exert this force once the crate starts to slip, what will its acceleration then be? The coefficient of sliding friction is known to be 0.300 for this situation.
    4. (a) If half of the weight of a small 1.00 x 103-kg utility truck is supported by its two drive wheels, what is the maximum acceleration it can achieve on dry concrete? (b) Will a metal cabinet lying on the wooden bed of the truck slip if it accelerates at this rate? (c) Solve both problems assuming the truck has four-wheel drive.
    5. A team of eight dogs pulls a sled with waxed wood runners on wet snow (mush!). The dogs have average masses of 19.0 kg, and the loaded sled with its rider has a mass of 210 kg. (a) Calculate the acceleration of the dogs starting from rest if each dog exerts an average force of 185 N backward on the snow. (b) Calculate the force in the coupling between the dogs and the sled.
    6. Consider the 65.0-kg ice skater being pushed by two others shown below. (a) Find the direction and magnitude of Ftot, the total force exerted on her by the others, given that the magnitudes F1 and F2 are 26.4 N and 18.6 N, respectively. (b) What is her initial acceleration if she is initially stationary and wearing steel-bladed skates that point in the direction of Ftot? (c) What is her acceleration assuming she is already moving in the direction of Ftot? (Remember that friction always acts in the direction opposite that of motion or attempted motion between surfaces in contact.)

    (a) Overhead view of two ice skaters pushing on a third. One skater pushes with a force F one, represented by an arrow pointing to the right, and a second skater pushes with a force F two, represented by an arrow pointing up. Vector F one and vector F two are along the arms of the two skaters acting on the third skater. A vector diagram is shown in the form of a right triangle in which the base is vector F one pointing to the right, and perpendicular to F one is vector F two pointing up. The resultant vector is shown by the hypotenuse pointing up and to the right and is labeled as vector F sub tot. (b) Free body diagram showing only the forces F sub one and F sub 2 acting on the skater.

    1. Show that the acceleration of any object down a frictionless incline that makes an angle \(\theta\) with the horizontal is a = g sin \(\theta\). (Note that this acceleration is independent of mass.)

    An illustration of block on a slope. The slope angles down and to the right at an angle of theta degrees to the horizontal. The block has an acceleration a parallel to the slope, toward its bottom. The following forces are shown: N perpendicular to the slope and pointing out of it, and w which equals m times g vertically down. An x y coordinate system is shown tilted so that positive x is downslope, parallel to the surface, and positive y is perpendicular to the slope, pointing out of the surface.

    1. Show that the acceleration of any object down an incline where friction behaves simply (that is, where fk = \(\mu_{k}\)N) is a = g(sin \(\theta\) − \(\mu_{k}\) cos \(\theta\)). Note that the acceleration is independent of mass and reduces to the expression found in the previous problem when friction becomes negligibly small (\(\mu_{k}\) = 0).

    An illustration of block on a slope. The slope angles down and to the right at an angle of theta degrees to the horizontal. The block has an acceleration, a, parallel to the slope, toward its bottom. The following forces are shown: f in a direction parallel to the slope toward its top, N perpendicular to the slope and pointing out of it, w sub x in a direction parallel to the slope toward its bottom, and w sub y perpendicular to the slope and pointing into it. An x y coordinate system is shown tilted so that positive x is downslope, parallel to the surface, and positive y is perpendicular to the slope, pointing out of the surface.

    1. Calculate the deceleration of a snow boarder going up a 5.00° slope, assuming the coefficient of friction for waxed wood on wet snow. The result of the preceding problem may be useful, but be careful to consider the fact that the snow boarder is going uphill.
    2. A machine at a post office sends packages out a chute and down a ramp to be loaded into delivery vehicles. (a) Calculate the acceleration of a box heading down a 10.0° slope, assuming the coefficient of friction for a parcel on waxed wood is 0.100. (b) Find the angle of the slope down which this box could move at a constant velocity. You can neglect air resistance in both parts.
    3. If an object is to rest on an incline without slipping, then friction must equal the component of the weight of the object parallel to the incline. This requires greater and greater friction for steeper slopes. Show that the maximum angle of an incline above the horizontal for which an object will not slide down is \(\theta\) = tan−1 \(\mu_{s}\). You may use the result of the previous problem. Assume that a = 0 and that static friction has reached its maximum value.

    An illustration of a block mass m on a slope. The slope angles up and to the right at an angle of theta degrees to the horizontal. The mass feels force w sub parallel in a direction parallel to the slope toward its bottom, and f in a direction parallel to the slope toward its top.

    1. Calculate the maximum acceleration of a car that is heading down a 6.00° slope (one that makes an angle of 6.00° with the horizontal) under the following road conditions. You may assume that the weight of the car is evenly distributed on all four tires and that the coefficient of static friction is involved—that is, the tires are not allowed to slip during the deceleration. (Ignore rolling.) Calculate for a car: (a) On dry concrete. (b) On wet concrete. (c) On ice, assuming that \(\mu_{s}\) = 0.100, the same as for shoes on ice.
    2. Calculate the maximum acceleration of a car that is heading up a 4.00° slope (one that makes an angle of 4.00° with the horizontal) under the following road conditions. Assume that only half the weight of the car is supported by the two drive wheels and that the coefficient of static friction is involved—that is, the tires are not allowed to slip during the acceleration. (Ignore rolling.) (a) On dry concrete. (b) On wet concrete. (c) On ice, assuming that \(\mu_{s}\) = 0.100, the same as for shoes on ice.
    3. Repeat the preceding problem for a car with four-wheel drive.
    4. A freight train consists of two 8.00 x 105-kg engines and 45 cars with average masses of 5.50 x 105 kg. (a) What force must each engine exert backward on the track to accelerate the train at a rate of 5.00 x 10−2 m/s2 if the force of friction is 7.50 x 105 N, assuming the engines exert identical forces? This is not a large frictional force for such a massive system. Rolling friction for trains is small, and consequently, trains are very energy-efficient transportation systems. (b) What is the force in the coupling between the 37th and 38th cars (this is the force each exerts on the other), assuming all cars have the same mass and that friction is evenly distributed among all of the cars and engines?
    5. Consider the 52.0-kg mountain climber shown below. (a) Find the tension in the rope and the force that the mountain climber must exert with her feet on the vertical rock face to remain stationary. Assume that the force is exerted parallel to her legs. Also, assume negligible force exerted by her arms. (b) What is the minimum coefficient of friction between her shoes and the cliff?

    A mountain climber is drawn leaning away from the rock face with her feet against the rock face. The rope extends up from the climber at an angle of 31 degrees to the vertical. The climbers legs are straight and make an angle of fifteen degrees with the rock face. The force vector F sub T starts at the harness and points away from the climber, along the rope. The force vector F sub legs starts at climber’s feet and points away from the rock, parallel to her legs.

    1. A contestant in a winter sporting event pushes a 45.0-kg block of ice across a frozen lake as shown below. (a) Calculate the minimum force F he must exert to get the block moving. (b) What is its acceleration once it starts to move, if that force is maintained?

    A block of ice is being pushed with a force F that is directed at an angle of twenty five degrees below the horizontal.

    1. The contestant now pulls the block of ice with a rope over his shoulder at the same angle above the horizontal as shown below. Calculate the minimum force F he must exert to get the block moving. (b) What is its acceleration once it starts to move, if that force is maintained?

    A block of ice is being pulled with a force F that is directed at an angle of twenty five degrees above the horizontal.

    1. At a post office, a parcel that is a 20.0-kg box slides down a ramp inclined at 30.0° with the horizontal. The coefficient of kinetic friction between the box and plane is 0.0300. (a) Find the acceleration of the box. (b) Find the velocity of the box as it reaches the end of the plane, if the length of the plane is 2 m and the box starts at rest.

    Contributors and Attributions

    Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).

    Conceptual Questions

    Newton's Law of Universal Gravitation

    1. Action at a distance, such as is the case for gravity, was once thought to be illogical and therefore untrue. What is the ultimate determinant of the truth in science, and why was this action at a distance ultimately accepted?
    2. In the law of universal gravitation, Newton assumed that the force was proportional to the product of the two masses (~m1m2). While all scientific conjectures must be experimentally verified, can you provide arguments as to why this must be? (You may wish to consider simple examples in which any other form would lead to contradictory results.)

    Gravitation Near Earth's Surface

    1. Must engineers take Earth’s rotation into account when constructing very tall buildings at any location other than the equator or very near the poles?

    Motion of a Charged Particle in a Magnetic Field

    3. At a given instant, an electron and a proton are moving with the same velocity in a constant magnetic field. Compare the magnetic forces on these particles. Compare their accelerations.

    4. Does increasing the magnitude of a uniform magnetic field through which a charge is traveling necessarily mean increasing the magnetic force on the charge? Does changing the direction of the field necessarily mean a change in the force on the charge?

    5. An electron passes through a magnetic field without being deflected. What do you conclude about the magnetic field?

    6. If a charged particle moves in a straight line, can you conclude that there is no magnetic field present?

    7. How could you determine which pole of an electromagnet is north and which pole is south?

    Problems

    Newton's Law of Universal Gravitation

    1. Evaluate the magnitude of gravitational force between two 5-kg spherical steel balls separated by a center-to-center distance of 15 cm.
    2. Estimate the gravitational force between two sumo wrestlers, with masses 220 kg and 240 kg, when they are embraced and their centers are 1.2 m apart.
    3. Astrology makes much of the position of the planets at the moment of one’s birth. The only known force a planet exerts on Earth is gravitational. (a) Calculate the gravitational force exerted on a 4.20-kg baby by a 100-kg father 0.200 m away at birth (he is assisting, so he is close to the child). (b) Calculate the force on the baby due to Jupiter if it is at its closest distance to Earth, some 6.29 x 1011 m away. How does the force of Jupiter on the baby compare to the force of the father on the baby? Other objects in the room and the hospital building also exert similar gravitational forces. (Of course, there could be an unknown force acting, but scientists first need to be convinced that there is even an effect, much less that an unknown force causes it.)
    4. A mountain 10.0 km from a person exerts a gravitational force on him equal to 2.00% of his weight. (a) Calculate the mass of the mountain. (b) Compare the mountain’s mass with that of Earth. (c) What is unreasonable about these results? (d) Which premises are unreasonable or inconsistent? (Note that accurate gravitational measurements can easily detect the effect of nearby mountains and variations in local geology.)
    5. The International Space Station has a mass of approximately 370,000 kg. (a) What is the force on a 150-kg suited astronaut if she is 20 m from the center of mass of the station? (b) How accurate do you think your answer would be?
    An image of the international space station is shown.
    Figure 13.33 (credit: ©ESA–David Ducros)
    1. Asteroid Toutatis passed near Earth in 2006 at four times the distance to our Moon. This was the closest approach we will have until 2060. If it has mass of 5.0 x 1013 kg , what force did it exert on Earth at its closest approach?
    2. (a) What was the acceleration of Earth caused by asteroid Toutatis (see previous problem) at its closest approach? (b) What was the acceleration of Toutatis at this point?

    Gravitation Near Earth's Surface

    1. (a) Calculate Earth’s mass given the acceleration due to gravity at the North Pole is measured to be 9.832 m/s2 and the radius of the Earth at the pole is 6356 km. (b) Compare this with the NASA’s Earth Fact Sheet value of 5.9726 x 1024 kg.
    2. (a) What is the acceleration due to gravity on the surface of the Moon? (b) On the surface of Mars? The mass of Mars is 6.418 x 1023 kg and its radius is 3.38 x 106 m.
    3. (a) Calculate the acceleration due to gravity on the surface of the Sun. (b) By what factor would your weight increase if you could stand on the Sun? (Never mind that you cannot.)
    4. The mass of a particle is 15 kg. (a) What is its weight on Earth? (b) What is its weight on the Moon? (c) What is its mass on the Moon? (d) What is its weight in outer space far from any celestial body? (e) What is its mass at this point?
    5. On a planet whose radius is 1.2 x 107 m, the acceleration due to gravity is 18 m/s2. What is the mass of the planet?
    6. The mean diameter of the planet Saturn is 1.2 x 108 m, and its mean mass density is 0.69 g/cm3. Find the acceleration due to gravity at Saturn’s surface.
    7. The mean diameter of the planet Mercury is 4.88 x 106 m, and the acceleration due to gravity at its surface is 3.78 m/s2. Estimate the mass of this planet.
    8. The acceleration due to gravity on the surface of a planet is three times as large as it is on the surface of Earth. The mass density of the planet is known to be twice that of Earth. What is the radius of this planet in terms of Earth’s radius?
    9. A body on the surface of a planet with the same radius as Earth’s weighs 10 times more than it does on Earth. What is the mass of this planet in terms of Earth’s mass?

    Contributors and Attributions

    Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).

    Conceptual Questions

    Problems

    Motion of a Charged Particle in a Magnetic Field

    25. A cosmic-ray electron moves at \(\displaystyle 7.5×10^6m/s\) perpendicular to Earth’s magnetic field at an altitude where the field strength is \(\displaystyle 1.0×10^{−5}T\). What is the radius of the circular path the electron follows?

    26. (a) Viewers of Star Trek have heard of an antimatter drive on the Starship Enterprise. One possibility for such a futuristic energy source is to store antimatter charged particles in a vacuum chamber, circulating in a magnetic field, and then extract them as needed. Antimatter annihilates normal matter, producing pure energy. What strength magnetic field is needed to hold antiprotons, moving at \(\displaystyle 5.0×10^7m/s\) in a circular path 2.00 m in radius? Antiprotons have the same mass as protons but the opposite (negative) charge.

    (b) Is this field strength obtainable with today’s technology or is it a futuristic possibility?

    27. (a) An oxygen-16 ion with a mass of \(\displaystyle 2.66×10^{−26}kg\) travels at \(\displaystyle 5.0×10^6m/s\) perpendicular to a 1.20-T magnetic field, which makes it move in a circular arc with a 0.231-m radius. What positive charge is on the ion?

    (b) What is the ratio of this charge to the charge of an electron? (c) Discuss why the ratio found in (b) should be an integer.

    28. An electron in a TV CRT moves with a speed of \(\displaystyle 6.0×10^7m/s\), in a direction perpendicular to Earth’s field, which has a strength of \(\displaystyle 5.0×10^{−5}T\). (a) What strength electric field must be applied perpendicular to the Earth’s field to make the electron moves in a straight line? (b) If this is done between plates separated by 1.00 cm, what is the voltage applied? (Note that TVs are usually surrounded by a ferromagnetic material to shield against external magnetic fields and avoid the need for such a correction.)

    29. (a) At what speed will a proton move in a circular path of the same radius as the electron in the previous exercise?

    (b) What would the radius of the path be if the proton had the same speed as the electron?

    (c) What would the radius be if the proton had the same kinetic energy as the electron?

    (d) The same momentum?

    30. (a) What voltage will accelerate electrons to a speed of \(\displaystyle 6.00×10^{−7}m/s\)? (b) Find the radius of curvature of the path of a proton accelerated through this potential in a 0.500-T field and compare this with the radius of curvature of an electron accelerated through the same potential.

    31. An alpha-particle (\(\displaystyle m=6.64×10^{−27}kg, q=3.2×10^{−19}C\)) travels in a circular path of radius 25 cm in a uniform magnetic field of magnitude 1.5 T.

    (a) What is the speed of the particle?

    (b) What is the kinetic energy in electron-volts?

    (c) Through what potential difference must the particle be accelerated in order to give it this kinetic energy?

    32. A particle of charge q and mass m is accelerated from rest through a potential difference V, after which it encounters a uniform magnetic field B. If the particle moves in a plane perpendicular to B, what is the radius of its circular orbit?

    Contributors and Attributions

    Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).

    Conceptual Questions

    Forces

    1. What properties do forces have that allow us to classify them as vectors?

    Mass and Weight

    1. What is the relationship between weight and mass? Which is an intrinsic, unchanging property of a body?
    2. How much does a 70-kg astronaut weight in space, far from any celestial body? What is her mass at this location?
    3. Which of the following statements is accurate?
      1. Mass and weight are the same thing expressed in different units.
      2. If an object has no weight, it must have no mass.
      3. If the weight of an object varies, so must the mass.
      4. Mass and inertia are different concepts.
      5. Weight is always proportional to mass.
    4. When you stand on Earth, your feet push against it with a force equal to your weight. Why doesn’t Earth accelerate away from you?
    5. How would you give the value of \(\vec{g}\) in vector form?

    Common Forces

    1. A table is placed on a rug. Then a book is placed on the table. What does the floor exert a normal force on?
    2. A particle is moving to the right. (a) Can the force on it to be acting to the left? If yes, what would happen? (b) Can that force be acting downward? If yes, why?

    Drawing Free-Body Diagrams

    1. In completing the solution for a problem involving forces, what do we do after constructing the free-body diagram? That is, what do we apply?
    2. If a book is located on a table, how many forces should be shown in a free-body diagram of the book? Describe them.
    3. If the book in the previous question is in free fall, how many forces should be shown in a free-body diagram of the book? Describe them.

    Problems

    5.1 Forces

    1. Two ropes are attached to a tree, and forces of \(\vec{F}_{1}\) = 2.0 \(\hat{i}\) + 4.0 \(\hat{j}\) N and \(\vec{F}_{2}\) = 3.0 \(\hat{i}\) + 6.0 \(\hat{j}\) N are applied. The forces are coplanar (in the same plane). (a) What is the resultant (net force) of these two force vectors? (b) Find the magnitude and direction of this net force.
    2. A telephone pole has three cables pulling as shown from above, with \(\vec{F}_{1}\) = (300.0 \(\hat{i}\) + 500.0 \(\hat{j}\)), \(\vec{F}_{2}\) = −200.0 \(\hat{i}\), and \(\vec{F}_{3}\) = −800.0 \(\hat{j}\). (a) Find the net force on the telephone pole in component form. (b) Find the magnitude and direction of this net force.

    Figure shows the coordinate axes, vector F1 at an angle of about 28 degrees with the positive y axis, vector F2 along the negative x axis and vector F3 along the negative y axis.

    1. Two teenagers are pulling on ropes attached to a tree. The angle between the ropes is 30.0°. David pulls with a force of 400.0 N and Stephanie pulls with a force of 300.0 N. (a) Find the component form of the net force. (b) Find the magnitude of the resultant (net) force on the tree and the angle it makes with David’s rope.

    5.4 Mass and Weight

    1. The weight of an astronaut plus his space suit on the Moon is only 250 N. (a) How much does the suited astronaut weigh on Earth? (b) What is the mass on the Moon? On Earth?
    2. Suppose the mass of a fully loaded module in which astronauts take off from the Moon is 1.00 x 104 kg. The thrust of its engines is 3.00 x 104 N. (a) Calculate the module’s magnitude of acceleration in a vertical takeoff from the Moon. (b) Could it lift off from Earth? If not, why not? If it could, calculate the magnitude of its acceleration.
    3. A rocket sled accelerates at a rate of 49.0 m/s2. Its passenger has a mass of 75.0 kg. (a) Calculate the horizontal component of the force the seat exerts against his body. Compare this with his weight using a ratio. (b) Calculate the direction and magnitude of the total force the seat exerts against his body.
    4. Repeat the previous problem for a situation in which the rocket sled decelerates at a rate of 201 m/s2. In this problem, the forces are exerted by the seat and the seat belt.
    5. A body of mass 2.00 kg is pushed straight upward by a 25.0 N vertical force. What is its acceleration?
    6. A car weighing 12,500 N starts from rest and accelerates to 83.0 km/h in 5.00 s. The friction force is 1350 N. Find the applied force produced by the engine.
    7. A body with a mass of 10.0 kg is assumed to be in Earth’s gravitational field with g = 9.80 m/s2. What is the net force on the body if there are no other external forces acting on the object?
    8. A fireman has mass m; he hears the fire alarm and slides down the pole with acceleration a (which is less than g in magnitude). (a) Write an equation giving the vertical force he must apply to the pole. (b) If his mass is 90.0 kg and he accelerates at 5.00 m/s2, what is the magnitude of his applied force?
    9. A baseball catcher is performing a stunt for a television commercial. He will catch a baseball (mass 145 g) dropped from a height of 60.0 m above his glove. His glove stops the ball in 0.0100 s. What is the force exerted by his glove on the ball?
    10. When the Moon is directly overhead at sunset, the force by Earth on the Moon, FEM, is essentially at 90° to the force by the Sun on the Moon, FSM, as shown below. Given that FEM = 1.98 x 1020 N and FSM = 4.36 x 1020 N, all other forces on the Moon are negligible, and the mass of the Moon is 7.35 x 1022 kg, determine the magnitude of the Moon’s acceleration.

    Figure shows a circle labeled moon. An arrow from it, pointing up is labeled F subscript EM. Another arrow from it pointing right is labeled F subscript SM.

    5.6 Common Forces

    1. A leg is suspended in a traction system, as shown below. (a) Which part of the figure is used to calculate the force exerted on the foot? (b) What is the tension in the rope? Here \(\vec{T}\) is the tension, \(\vec{w}_{leg}\) is the weight of the leg, and \(\vec{w}\) is the weight of the load that provides the tension.

    Figure shows a leg on the left. It is attached to a rope at the ankle and suspended from a pulley at the top. The rope continues to a second pulley on the right of the first, a third one to the bottom of the second, a fourth one to the bottom left of the third and a fifth one to the bottom right of the fourth. An object with weight w = mg is attached at the end of the rope. An arrow labeled w subscript leg points downwards from the ankle and an arrow labeled T points upwards from there. An arrow labeled T, from the fourth pulley to the third, forms an angle theta with the horizontal. Another arrow labeled T, from the fourth pulley to the fifth forms an angle minus theta with the horizontal.

    1. Suppose the shinbone in the preceding image was a femur in a traction setup for a broken bone, with pulleys and rope available. How might we be able to increase the force along the femur using the same weight?
    2. A team of nine members on a tall building tug on a string attached to a large boulder on an icy surface. The boulder has a mass of 200 kg and is tugged with a force of 2350 N. (a) What is magnitude of the acceleration? (b) What force would be required to produce a constant velocity?
    3. What force does a trampoline have to apply to Jennifer, a 45.0-kg gymnast, to accelerate her straight up at 7.50 m/s2? The answer is independent of the velocity of the gymnast—she can be moving up or down or can be instantly stationary.
    4. (a) Calculate the tension in a vertical strand of spider web if a spider of mass 2.00 x 10−5 kg hangs motionless on it. (b) Calculate the tension in a horizontal strand of spider web if the same spider sits motionless in the middle of it much like the tightrope walker in Figure 5.26. The strand sags at an angle of 12° below the horizontal. Compare this with the tension in the vertical strand (find their ratio).
    5. Suppose Kevin, a 60.0-kg gymnast, climbs a rope. (a) What is the tension in the rope if he climbs at a constant speed? (b) What is the tension in the rope if he accelerates upward at a rate of 1.50 m/s2?
    6. Show that, as explained in the text, a force F\(\perp\) exerted on a flexible medium at its center and perpendicular to its length (such as on the tightrope wire in Figure 5.26) gives rise to a tension of magnitude \(T = \frac{F_{\perp}}{2 \sin \theta}\).
    7. Consider Figure 5.28. The driver attempts to get the car out of the mud by exerting a perpendicular force of 610.0 N, and the distance she pushes in the middle of the rope is 1.00 m while she stands 6.00 m away from the car on the left and 6.00 m away from the tree on the right. What is the tension T in the rope, and how do you find the answer?
    8. A bird has a mass of 26 g and perches in the middle of a stretched telephone line. (a) Show that the tension in the line can be calculated using the equation \(T = \frac{mg}{2 \sin \theta}\). Determine the tension when (b) \(\theta\) = 5° and (c) \(\theta\) = 0.5°. Assume that each half of the line is straight.

    Figure shows a bird sitting on a wire that is fixed at both ends. The wire sags with its weight, forming an angle theta with the horizontal on either side.

    1. One end of a 30-m rope is tied to a tree; the other end is tied to a car stuck in the mud. The motorist pulls sideways on the midpoint of the rope, displacing it a distance of 2 m. If he exerts a force of 80 N under these conditions, determine the force exerted on the car.
    2. Consider the baby being weighed in the following figure. (a) What is the mass of the infant and basket if a scale reading of 55 N is observed? (b) What is tension T1 in the cord attaching the baby to the scale? (c) What is tension T2 in the cord attaching the scale to the ceiling, if the scale has a mass of 0.500 kg? (d) Sketch the situation, indicating the system of interest used to solve each part. The masses of the cords are negligible.

    Figure shows a baby in a basket attached to a spring scale, which in turn is fixed from a rigid support. Arrow T2 points down from the support. Another arrow T2 points up from the top of the scale. Arrow T1 points down from the bottom of the scale. Another arrow T1 points up from the basket. Arrow w points down from the basket. The scale has markings from 0 to 300 Newtons.

    1. What force must be applied to a 100.0-kg crate on a frictionless plane inclined at 30° to cause an acceleration of 2.0 m/s2 up the plane?

    Figure shows an object on a slope of 30 degrees. An arrow pointing up and parallel to the slope is labeled F.

    1. A 2.0-kg block is on a perfectly smooth ramp that makes an angle of 30° with the horizontal. (a) What is the block’s acceleration down the ramp and the force of the ramp on the block? (b) What force applied upward along and parallel to the ramp would allow the block to move with constant velocity?

    5.7 Drawing Free-Body Diagrams

    1. A ball of mass m hangs at rest, suspended by a string. (a) Sketch all forces. (b) Draw the free-body diagram for the ball.
    2. A car moves along a horizontal road. Draw a free-body diagram; be sure to include the friction of the road that opposes the forward motion of the car.
    3. A runner pushes against the track, as shown. (a) Provide a free-body diagram showing all the forces on the runner. (Hint: Place all forces at the center of his body, and include his weight.) (b) Give a revised diagram showing the xy-component form.

    A picture of a man running towards the right is shown. An arrow labeled F points up and right from the floor towards his foot.

    1. The traffic light hangs from the cables as shown. Draw a free-body diagram on a coordinate plane for this situation.

    Figure shows a traffic light hanging from a horizontal cable by three other cables, T1, T2 and T3. T1 hangs down and right making an angle of 41 degrees with the horizontal cable. T2 hangs down and left, making an angle of 63 degrees with the horizontal cable. These meet at a point and T3 hangs vertically down from here. The light is attached to T3. A vector pointing down from the light is labeled w equal to 200 newtons.

    Contributors and Attributions

    Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).

    Conceptual Questions

    Coulomb's Law

    20. Would defining the charge on an electron to be positive have any effect on Coulomb’s law?

    21. An atomic nucleus contains positively charged protons and uncharged neutrons. Since nuclei do stay together, what must we conclude about the forces between these nuclear particles?

    22. Is the force between two fixed charges influenced by the presence of other charges?

    Problems

    Coulomb's Law

    48. Two point particles with charges +3μC and +5μC are held in place by 3-N forces on each charge in appropriate directions. (a) Draw a free-body diagram for each particle. (b) Find the distance between the charges.

    49. Two charges +3μC and +12μC are fixed 1 m apart, with the second one to the right. Find the magnitude and direction of the net force on a −2-nC charge when placed at the following locations:

    (a) halfway between the two

    (b) half a meter to the left of the +3μC charge

    (c) half a meter above the +12μC charge in a direction perpendicular to the line joining the two fixed charges

    50. In a salt crystal, the distance between adjacent sodium and chloride ions is \(\displaystyle 2.82×10^{−10}m\). What is the force of attraction between the two singly charged ions?

    51. Protons in an atomic nucleus are typically \(\displaystyle 10^{−15}m\) apart. What is the electric force of repulsion between nuclear protons?

    52. Suppose Earth and the Moon each carried a net negative charge −Q. Approximate both bodies as point masses and point charges.

    (a) What value of Q is required to balance the gravitational attraction between Earth and the Moon?

    (b) Does the distance between Earth and the Moon affect your answer? Explain.

    (c) How many electrons would be needed to produce this charge?

    53. Point charges \(\displaystyle q_1=50μC\) and \(\displaystyle q_2=−25μC\) are placed 1.0 m apart. What is the force on a third charge \(\displaystyle q_3=20μC\) placed midway between \(\displaystyle q_1\) and \(\displaystyle q_2\)?

    54. Where must \(\displaystyle q_3\) of the preceding problem be placed so that the net force on it is zero?

    55. Two small balls, each of mass 5.0 g, are attached to silk threads 50 cm long, which are in turn tied to the same point on the ceiling, as shown below. When the balls are given the same charge Q, the threads hang at 5.0° to the vertical, as shown below. What is the magnitude of Q? What are the signs of the two charges?

    Two small balls are attached to threads which are in turn tied to the same point on the ceiling. The threads hang at an angle of 5.0 degrees to either side of the vertical. Each ball has a charge Q.

    56. Point charges \(\displaystyle Q_1=2.0μC\) and \(\displaystyle Q_2=4.0μC\) are located at \(\displaystyle \vec{r_1}=(4.0\hat{i}−2.0\hat{j}+5.0\hat{k})m\) and \(\displaystyle \vec{r_2}=(8.0\hat{i}+5.0\hat{j}−9.0\hat{k})m\). What is the force of \(\displaystyle Q_2\) on \(\displaystyle Q_1\)?

    57. The net excess charge on two small spheres (small enough to be treated as point charges) is Q. Show that the force of repulsion between the spheres is greatest when each sphere has an excess charge Q/2. Assume that the distance between the spheres is so large compared with their radii that the spheres can be treated as point charges.

    58. Two small, identical conducting spheres repel each other with a force of 0.050 N when they are 0.25 m apart. After a conducting wire is connected between the spheres and then removed, they repel each other with a force of 0.060 N. What is the original charge on each sphere?

    59. A charge q=2.0μC is placed at the point P shown below. What is the force on q?

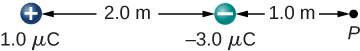
    Two charges are shown, placed on a horizontal line and separated by 2.0 meters. The charge on the left is a positive 1.0 micro Coulomb charge. The charge on the right is a negative 2.0 micro Coulomb charge. Point P is 1.0 to the right of the negative charge.

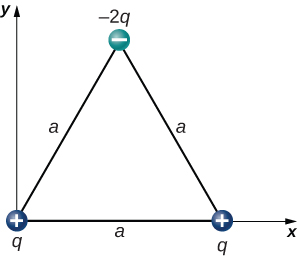
    60. What is the net electric force on the charge located at the lower right-hand corner of the triangle shown here?

    Charges are shown at the vertices of an equilateral triangle with sides length a. The bottom of the triangle is on the x axis of an x y coordinate system, and the bottom left vertex is at the origin. The charge at the origin is positive q. The charge at the bottom right hand corner is also positive q. The charge at the top vertex is negative two q.

    61. Two fixed particles, each of charge \(\displaystyle 5.0×10^{−6}C\), are 24 cm apart. What force do they exert on a third particle of charge \(\displaystyle −2.5×10^{−6}C\) that is 13 cm from each of them?

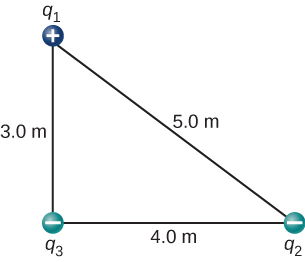
    62. The charges \(\displaystyle q_1=2.0×10^{−7}C,q_2=−4.0×10^{−7}C\), and \(\displaystyle q_3=−1.0×10^{−7}C\) are placed at the corners of the triangle shown below. What is the force on \(\displaystyle q_1\)?

    Charges are shown at the vertices of a right triangle. The bottom of the triangle is length 4 meters, the vertical side on the left is length 3 meters, and the hypotenuse is length 5 meters. The charge at the top is q sub one and positive, the charge at the bottom left is q sub 3 and negative and the charge at the bottom right is q sub 2 and negative.

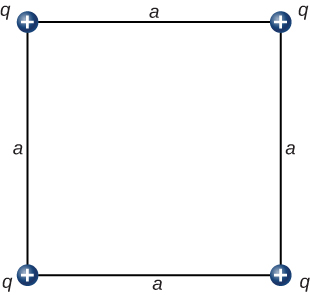
    63. What is the force on the charge q at the lower-right-hand corner of the square shown here?

    Charges are shown at the corners of a square with sides length a. All of the charges are positive and all are magnitude q.

    64. Point charges \(\displaystyle q_1=10μC\) and \(\displaystyle q_2=−30μC\) are fixed at \(\displaystyle r_1=(3.0\hat{i}−4.0\hat{j})m\) and \(\displaystyle r_2=(9.0\hat{i}+6.0\hat{j})m.\) What is the force of \(\displaystyle q_2\) on \(\displaystyle q_1\)?

    Contributors and Attributions

    Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).

    Conceptual Questions

    Torque

    1. What three factors affect the torque created by a force relative to a specific pivot point?
    2. Give an example in which a small force exerts a large torque. Give another example in which a large force exerts a small torque.
    3. When reducing the mass of a racing bike, the greatest benefit is realized from reducing the mass of the tires and wheel rims. Why does this allow a racer to achieve greater accelerations than would an identical reduction in the mass of the bicycle’s frame?
    4. Can a single force produce a zero torque?
    5. Can a set of forces have a net torque that is zero and a net force that is not zero?
    6. Can a set of forces have a net force that is zero and a net torque that is not zero?
    7. In the expression \(\vec{r} \times \vec{F}\) can \(|\vec{r}|\) ever be less than the lever arm? Can it be equal to the lever arm?

    Problems

    Torque

    1. Two flywheels of negligible mass and differ3ent radii are bonded together and rotate about a common axis (see below). The smaller flywheel of radius 30 cm has a cord that has a pulling force of 50 N on it. What pulling force needs to be applied to the cord connecting the larger flywheel of radius 50 cm such that the combination does not rotate?

    Figure shows two flywheels of different radii that are bonded together and rotate about a common axis. A force of 50 N is applied to the smaller flywheel. A force of unknown magnitude is applied to the larger flywheel and pulls it into the opposite direction.

    1. The cylinder head bolts on a car are to be tightened with a torque of 62.0 N·m. If a mechanic uses a wrench of length 20 cm, what perpendicular force must he exert on the end of the wrench to tighten a bolt correctly?
    2. (a) When opening a door, you push on it perpendicularly with a force of 55.0 N at a distance of 0.850 m from the hinges. What torque are you exerting relative to the hinges? (b) Does it matter if you push at the same height as the hinges? There is only one pair of hinges.
    3. When tightening a bolt, you push perpendicularly on a wrench with a force of 165 N at a distance of 0.140 m from the center of the bolt. How much torque are you exerting in newton-meters (relative to the center of the bolt)?
    4. What hanging mass must be placed on the cord to keep the pulley from rotating (see the following figure)? The mass on the frictionless plane is 5.0 kg. The inner radius of the pulley is 20 cm and the outer radius is 30 cm.

    Figure shows the pulley in which a mass of 5 kg rests on an inclined plane at a 45 degree angle and acts as a counterweight to an object of the unknown mass that hangs in the air.

    1. A simple pendulum consists of a massless tether 50 cm in length connected to a pivot and a small mass of 1.0 kg attached at the other end. What is the torque about the pivot when the pendulum makes an angle of 40° with respect to the vertical?
    2. Calculate the torque about the z-axis that is out of the page at the origin in the following figure, given that F1 = 3 N, F2 = 2 N, F3 = 3 N, F4 = 1.8 N.

    Figure shows the XY coordinate system. Force F1 is applied from the point that is located at the line that originates from the center of the coordinate system and is directed towards the top right corner. Point is 3 meters away from the origin and force F1 is directed towards the right bottom corner. Force F2 is applied from the point that is located at the Y axis, 2 meters above the center of the coordinate system. Force F2 forms 30 degree angle with the line parallel to the X axis and is directed towards the left bottom corner. Force F3 is applied from the center of coordinate system and is directed towards the left bottom corner. Force F4 is applied from the point that is located at the X axis, 2 meters to the right from the center of the coordinate system. Force F2 forms 20 degree angle with the line parallel to the Y axis and is directed towards the left bottom corner.

    1. A seesaw has length 10.0 m and uniform mass 10.0 kg and is resting at an angle of 30° with respect to the ground (see the following figure). The pivot is located at 6.0 m. What magnitude of force needs to be applied perpendicular to the seesaw at the raised end so as to allow the seesaw to barely start to rotate?

    Figure shows a seesaw. One of the ends of the seesaw rests on the ground forming 30 degree angle with it, another end is hanging in the air.

    1. A pendulum consists of a rod of mass 1 kg and length 1 m connected to a pivot with a solid sphere attached at the other end with mass 0.5 kg and radius 30 cm. What is the torque about the pivot when the pendulum makes an angle of 30° with respect to the vertical?
    2. A torque of 5.00 x 103 N • m is required to raise a drawbridge (see the following figure). What is the tension necessary to produce this torque? Would it be easier to raise the drawbridge if the angle \(\theta\) were larger or smaller?

    Figure shows the drawbridge that has a length of 6 meters. A force is applied at a 30 degree angle towards the drawbridge.

    1. A horizontal beam of length 3 m and mass 2.0 kg has a mass of 1.0 kg and width 0.2 m sitting at the end of the beam (see the following figure). What is the torque of the system about the support at the wall?

    Figure shows a horizontal beam that is connected to the wall. Beam has a length of 3 m and mass 2.0 kg. In addition, a mass of 1.0 kg and width 0.2 m sits at the end of the beam.

    1. What force must be applied to end of a rod along the x-axis of length 2.0 m in order to produce a torque on the rod about the origin of 8.0\(\hat{k}\) N • m?
    2. What is the torque about the origin of the force (5.0 \(\hat{i}\) − 2.0 \(\hat{j}\) + 1.0\(\hat{k}\)) N if it is applied at the point whose position is: \(\vec{r}\) = (−2.0 \(\hat{i}\) + 4.0\(\hat{j}\)) m?

    Contributors and Attributions

    Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).

    This page titled 5.E: Practice is shared under a CC BY-NC-SA 4.0 license and was authored, remixed, and/or curated by OpenStax via source content that was edited to the style and standards of the LibreTexts platform; a detailed edit history is available upon request.