Surface Force Apparatus
- Page ID
- 765
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Surface Force Apparatus (SFA) is an instrument that allows for very precise measurement of distance between interacting surfaces, as well as the forces of the interaction. When speaking of measuring molecular interactions, most people use affinity as a measure of strength. That is not true, as affinity only shows how likely it is for the bond to form, and not how physically strong it is. Few techniques exist that allow for a direct measurement of force, and Surface Force Apparatus (SFA) is one of them. What separates SFA from other techniques is its ability to measure forces directly as a function of distance between two samples. It is not a single molecule technique, i.e. the forces measured are results of interactions of cohorts of molecules, not a single molecule to molecule. It allows for quantification of weak interactions on the order of thermal energy (kBT) while determining absolute separations with almost Angstrom precision.
Principle of operation
The SFA consists of two silica cross cylinders, which are coated with a layer of silver and almost atomically smooth mica. The sample is located on top of the mica, where the contact point of the two cylinders would be.

Fig.1 Surface Force Apparatus, vertical cross-section.
http://www.chemengr.ucsb.edu/~ceweb/...li/mk2mk3.html
The two cylindrical lenses with their reflective coating and the medium in between create a Fabry-Perot interferometer. The only light that exits inside the resonant cavity is wavelengths that interfere constructively. See figure 2 for the principle of operation.

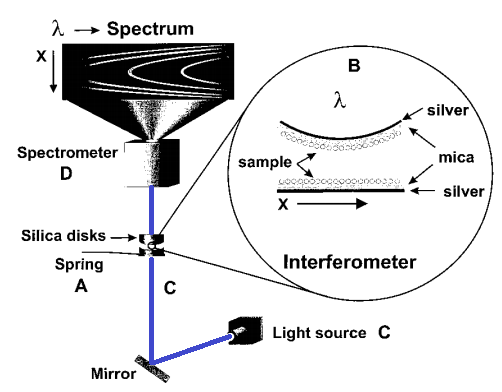
Fig. 2 Multiple internal reflections inside a Fabry-Perot interferometer Fig. 3 Schematic of SFA. (Leckband, et. al.)
Interferometry is then used to separate the interference fringes of equal chromatic order. As the distance between the lenses changes, the transmitted wavelength is shifted. During this time, the spring system holding on of the cylinders in place is used to record the forces (Helical spring and double cantilever spring in Fig.1). Typically the area of contact is about 5 microns squared, which amounts to about 300000 interacting pairs, which generates enough force to measure by the spring system. This spring system is a simple piece of mechanical engineering that allows for very small force changes by combining two springs together. Essentially, a micrometer screw (which is used for initial setup, while a piezo is used during an experiment for precise manipulations) pushes on a spring, which in turn pushes on another, double-cantilever spring (see Fig. 1). By combining two springs with very different spring constants one can vary the exerted force in very small increments.

3. Limitations
SFA has two major limitations. The studied molecules must be immobilized on the surface, and they must be homogeneously oriented. This is important because, essentially, the results are obtained from a cohort of molecules, and if some of them are oriented differently, the results are, ultimately, wrong. The goal of the experiment is to find out the force of interaction between one single molecule and another. To do that, the investigator has to know exactly how many molecules are in the interacting area, i.e. the area of contact. Knowing the surface are per molecule and the area of contact one can easily calculate the number of molecules at play. However, if some of them are oriented differently, i.e. in such a way that would prevent or alter their interactions with their counterparts on the other cylinder, the number obtained from the molecules per area calculation will no longer represent the true number of interacting molecules.
The second limitations is time resolution. Mechanical springs can only be moved so fast, so the typical resolution is tens of seconds. This limitation is intrinsic to the instrument design, which trades speed for precision. The large distance traveled by the thread of the micrometer screw or the piezo head is converted into very small distance traveled by the cylinder via the two spring system. This allows for very small increments of distance change, but limits the speed at which the distance can be changed.
References
- Jacob Israelachvili group, UC College of Engineering
- D. E. Leckband et al. "Force Probe Measurements of Antibody - Antigen Interactions", METHODS 20, 329 - 340 (2000)
- Raquel Orozco-Alcaraz and Tonya L. Kuhl. "Interaction Forces between DPPC Bilayers on Glass" Langmuir, 2013, 29 (1), pp 337-343

