1.3: Plane Areas

Plane areas in which the equation is given in $x-y$ coordinates

We have a curve $y = y(x)$ (Figure I.3) and we wish to find the position of the centroid of the area under the curve between $x = a$ and $x = b$. We consider an elemental slice of width $\delta x$ at a distance $x$ from the $y$ axis. Its area is $y \delta x$, and so the total area is

$$A = \int_a^b ydx \label{eq:1.3.1}$$

The first moment of area of the slice with respect to the $y$ axis is $x y \delta x$, and so the first moment of the entire area is $\int_a^b xydx$.

Therefore

$$\overline{x} = \frac{ \int_a^b xydyx }{\int_a^b ydyx} = \frac{\int_a^b xydyx}{A} \\label{eq:1.3.2}$$

For $\overline{y}$ we notice that the distance of the centroid of the slice from the $x$ axis is $\frac{1}{2}y$ , and therefore the first moment of the area about the $x$ axis is $\frac{1}{2} y.y \delta x$ .

Therefore

$$\overline{y} = \frac{ \int_a^b y^2dx }{2A} \label{eq:1.3.3}$$

Example $\PageIndex{1}$

Consider a semicircular lamina, $x^{2} + y^{2} = a^{2}$ , see Figure I.4:

We are dealing with the parts both above and below the \(x \) axis, so the area of the semicircle is $2\int_0^a ydx$ and the first moment of area is $2\int_0^a xydx$.

You should find $\overline{x} = 4a/ (3 \pi) = 0.4244a$.

Now consider the lamina $x^{2} + y^{2} = a^{2}$ , $y >0$ (Figure I.5):

The area of the elemental slice this time is $y \delta x$ (not $2 y \delta x$ ), and the integration limits are from $-a$ to $+a$. To find $\overline{y}$, use Equation $\ref{eq:1.3.3}$, and you should get $y = 0.4244a$ .

Plane areas in which the equation is given in polar coordinates.

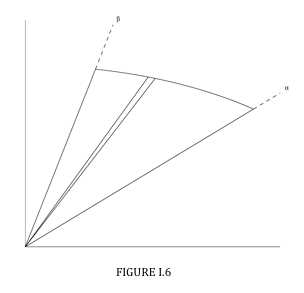
We consider an elemental triangular sector (Figure I.6) between $\theta$ and $\theta + \delta \theta$ . The "height" of the triangle is r and the "base" is $r \delta \theta$. The area of the triangle is $\frac{1}{2} r^{2} \delta \theta$.

Therefore the whole area =

$$\frac{1}{2} \int_ \alpha ^ \beta r^{2}d \theta \label{eq:1.3.4}$$

The horizontal distance of the centroid of the elemental sector from the origin (more correctly, from the "pole" of the polar coordinate system) is $\frac{2}{3} r \cos \theta$ . The first moment of area of the sector with respect to the $y$ axis is

$\frac{2}{3}r\cos \theta \times \frac{1}{2} r^{2} \delta \theta = \frac{1}{3}r^{3} \cos \theta \delta \theta$

so the first moment of area of the entire figure between $\theta = \alpha$ and $\theta = \beta$ is

$\frac{1}{3} \int_ \alpha ^ \beta r^3 \cos \theta d \theta$

Therefore

$$\overline{x} = \frac{2 \int_ \alpha ^ \beta r^{3}\cos \theta d \theta }{3\int_ \alpha ^ \beta r^{2} d \theta} \label{eq:1.3.5}$$

Similarly

$$\overline{x} = \frac{2 \int_ \alpha ^ \beta r^{3}\sin \theta d \theta }{3\int_ \alpha ^ \beta r^{2} d \theta} \label{eq:1.3.6}$$

Example $\PageIndex{2}$

Consider the semicircle $r = a$, $\theta = \frac{- \pi}{2}$ to $\frac{+ \pi}{2}$

$$\overline{x} = \frac{2a \int_{- \pi /2}^{+ \pi /2} \cos \theta d \theta }{3 \int_{- \pi /2}^{+ \pi /2} d \theta } = \frac{2a}{3 \pi } \int_{- \pi /2}^{+ \pi /2} \cos \theta d \theta = \frac{4a}{3 \pi} \label{eq:1.3.7}$$

The reader should now try to find the position of the centroid of a circular sector (slice of pizza!) of angle $2\alpha$ . The integration limits will be $- \alpha$ to $+ \alpha$.

When you arrive at a formula (which you should keep in a notebook for future reference), check that it goes to $\frac{4 \alpha}{ 3 \pi}$ if $\alpha = \frac{ \pi}{2}$, and to $\frac{2 \pi}{3}$ if $\alpha = 0$.