22.6: Different Fundamental Quantities

We stated at the beginning of this chapter that any mechanical quantity could be expressed in terms of three fundamental quantities, mass, length and time. But there is nothing particularly magic about these quantities. For example, we might decide that we could express any mechanical quantity in terms of, say, energy E, speed V and angular momentum J. We might then say that the dimensions of area could be expressed as E^-2V²J² .(Verify this!)

While agreeing that such a system might be possible, you might feel that it would be totally absurd and there is no point in reading further.

But stop! Such a system is not only possible, but it is normally and routinely used in the field of high-energy particle physics. That, perhaps, is a surprise, but, if you are thinking of taking an interest in particle physics, read on.

The units generally used in particle physics to express the fundamental quantities energy, speed and angular momentum are GeV (or MeV, or TeV , etc) for energy, the speed of light \(c\) for speed, and the modified Planck constant \(ħ\) for angular momentum. There are often referred to as “natural” units, the speed of light being a “natural” unit of speed and ħ being a “natural” unit for angular momentum, whereas metre, kilogram and second are not so “natural” in this sense as they are “man-made”. It is true that a GeV is not particularly “natural”, but at least a system with GeV, \(c\) and \(ħ\) as fundamental quantities is certainly more “natural” than metre-kilogram-second.

In any case, the dimensions of mass in this system are EV⁻². (You can see this immediately, for example from Einstein’s famous equation E = mc².) The units used in this system are GeV/c². Thus the rest mass of a proton is 0.9383 GeV/c², and the rest mass of an electron is 0.5110 MeV/c². One way to interpret this, if you like, is to say that the rest-mass energy of a proton (i.e. its m₀c²) is 0.9383 GeV.

Likewise the dimensions of linear momentum are EV⁻¹, and units in which it is expressed are GeV/c. (You can see this, for example, if you look at the energy and momentum of a photon: E = hv, p = \( h/ \gamma \), from which \( \frac{P}{E} = \frac{1}{v \gamma} = \frac{1}{c} \))

Torque (which has the same dimensions as energy) is equal to rate of change of angular momentum, from which we see that time has dimensions E−1J and could be expressed in units of ħ/GeV. Alternatively you can see that [time] = ħ/GeV immediately from Planck’s equation . \( E = h \omega\) And speed is distance over time, so that we see that distance, or length, has dimensions E⁻¹VJ, and hence units ħc/GeV.

Using data from the 2010 Particle Physics Booklet, I calculate as follows.

Mass: Length: Time: Energy: Linear Momentum:

1 GeV/c^2= 1.782 661 76 ~ 10^−27kg 1 ħc/GeV = 1.973 269 63 ~ 10^−16 m 1 ħ/GeV = 6.582 118 99 ~ 10^−26 s 1 GeV = 1.602 176 49 ~ 10^−10 J 1 GeV/c = 5.344 285 50 ~ 10^−19 kg m s^−1

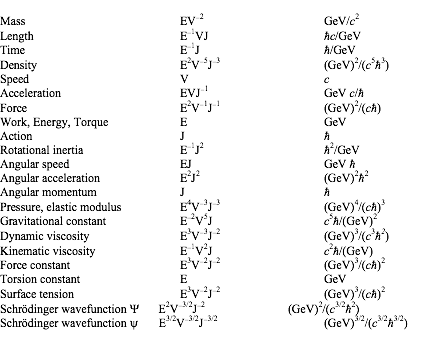
I give here a table of the dimensions (in terms of EVJ) of the same quantities as in the table of page 2. I dare say some of them are never likely to be needed, but some certainly will be needed, and, rather than predict which will be useful and which not, I might as well give them all. The dynamic viscosity of water at room temperature is about 10⁻³ kg m⁻¹ s⁻¹, or 10⁻³ dekapoise. I cannot imagine anyone needing to know that the dynamic viscosity of water at room temperature is about 7.3 ~ 10⁻¹⁸ (GeV)³/(c³ħ²) , or that its surface tension is so many (GeV)³/(cħ)² − but you never know