3.E: Two-Dimensional Kinematics (Exercises)
- Last updated
- Save as PDF
- Page ID
- 8795
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Conceptual Questions
3.2: Vector Addition and Subtraction: Graphical Methods
1. Which of the following is a vector: a person’s height, the altitude on Mt. Everest, the age of the Earth, the boiling point of water, the cost of this book, the Earth’s population, the acceleration of gravity?
2. Give a specific example of a vector, stating its magnitude, units, and direction.
3. What do vectors and scalars have in common? How do they differ?
4. Two campers in a national park hike from their cabin to the same spot on a lake, each taking a different path, as illustrated below. The total distance traveled along Path 1 is 7.5 km, and that along Path 2 is 8.2 km. What is the final displacement of each camper?
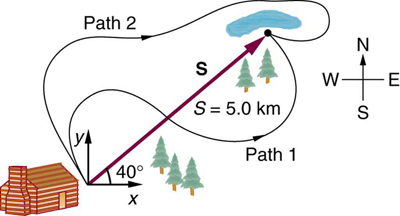
5. If an airplane pilot is told to fly 123 km in a straight line to get from San Francisco to Sacramento, explain why he could end up anywhere on the circle shown in Figure. What other information would he need to get to Sacramento?

6. Suppose you take two steps A and B (that is, two nonzero displacements). Under what circumstances can you end up at your starting point? More generally, under what circumstances can two nonzero vectors add to give zero? Is the maximum distance you can end up from the starting point A+B the sum of the lengths of the two steps?
7. Explain why it is not possible to add a scalar to a vector
8. If you take two steps of different sizes, can you end up at your starting point? More generally, can two vectors with different magnitudes ever add to zero? Can three or more?
3.3: Vector Addition and Subtraction: Analytical Methods
9. Suppose you add two vectors A and B. What relative direction between them produces the resultant with the greatest magnitude? What is the maximum magnitude? What relative direction between them produces the resultant with the smallest magnitude? What is the minimum magnitude?
10. Give an example of a nonzero vector that has a component of zero.
11. Explain why a vector cannot have a component greater than its own magnitude.
12. If the vectors A and B are perpendicular, what is the component of A along the direction of B? What is the component of B along the direction of A?
3.4: Projectile Motion
13. Answer the following questions for projectile motion on level ground assuming negligible air resistance (the initial angle being neither 0º nor 90º):
(a) Is the velocity ever zero?
(b) When is the velocity a minimum? A maximum?
(c) Can the velocity ever be the same as the initial velocity at a time other than at \(t=0\)?
(d) Can the speed ever be the same as the initial speed at a time other than at \(t=0\)?
14. Answer the following questions for projectile motion on level ground assuming negligible air resistance (the initial angle being neither \(0º\) nor \(90º\)):
(a) Is the acceleration ever zero?
(b) Is the acceleration ever in the same direction as a component of velocity?
(c) Is the acceleration ever opposite in direction to a component of velocity?
15. For a fixed initial speed, the range of a projectile is determined by the angle at which it is fired. For all but the maximum, there are two angles that give the same range. Considering factors that might affect the ability of an archer to hit a target, such as wind, explain why the smaller angle (closer to the horizontal) is preferable. When would it be necessary for the archer to use the larger angle? Why does the punter in a football game use the higher trajectory?
16. During a lecture demonstration, a professor places two coins on the edge of a table. She then flicks one of the coins horizontally off the table, simultaneously nudging the other over the edge. Describe the subsequent motion of the two coins, in particular discussing whether they hit the floor at the same time.
3.5: Addition of Velocities
17. What frame or frames of reference do you instinctively use when driving a car? When flying in a commercial jet airplane?
18. A basketball player dribbling down the court usually keeps his eyes fixed on the players around him. He is moving fast. Why doesn’t he need to keep his eyes on the ball?
19. If someone is riding in the back of a pickup truck and throws a softball straight backward, is it possible for the ball to fall straight down as viewed by a person standing at the side of the road? Under what condition would this occur? How would the motion of the ball appear to the person who threw it?
20. The hat of a jogger running at constant velocity falls off the back of his head. Draw a sketch showing the path of the hat in the jogger’s frame of reference. Draw its path as viewed by a stationary observer.
21. A clod of dirt falls from the bed of a moving truck. It strikes the ground directly below the end of the truck. What is the direction of its velocity relative to the truck just before it hits? Is this the same as the direction of its velocity relative to ground just before it hits? Explain your answers.
Problems & Exercises
3.2: Vector Addition and Subtraction: Graphical Methods
Use graphical methods to solve these problems. You may assume data taken from graphs is accurate to three digits.
22. Find the following for path A in Figure:
(a) the total distance traveled, and
(b) the magnitude and direction of the displacement from start to finish.
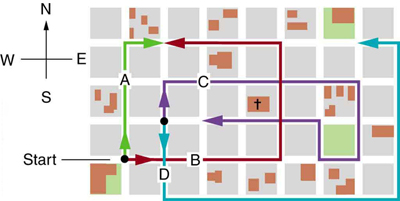
The various lines represent paths taken by different people walking in a city. All blocks are 120 m on a side.
solution:
(a) 480 m
(b) 379 m, 18.4º east of north
23. Find the following for path B in Figure:
(a) the total distance traveled, and
(b) the magnitude and direction of the displacement from start to finish.
24. Find the north and east components of the displacement for the hikers shown in Figure.

Solution
north component 3.21 km, east component 3.83 km
25. Suppose you walk 18.0 m straight west and then 25.0 m straight north. How far are you from your starting point, and what is the compass direction of a line connecting your starting point to your final position? (If you represent the two legs of the walk as vector displacements A and B , as in Figure, then this problem asks you to find their sum R=A+B.)
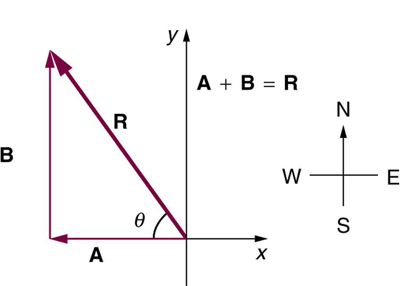
The two displacements A and B add to give a total displacement R having magnitude \(R\) and direction \(θ\).
26. Suppose you first walk 12.0 m in a direction 20º west of north and then 20.0 m in a direction 40.0º south of west. How far are you from your starting point, and what is the compass direction of a line connecting your starting point to your final position? (If you represent the two legs of the walk as vector displacements A and B, as in Figure, then this problem finds their sum R = A + B.)
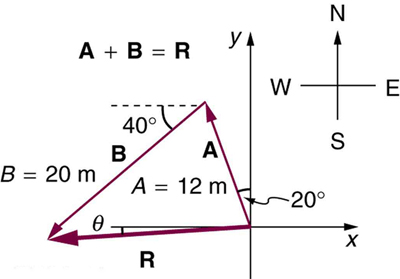
Solution
\(19.5 m, 4.65º\) south of west
27. Repeat the problem above, but reverse the order of the two legs of the walk; show that you get the same final result. That is, you first walk leg B, which is 20.0 m in a direction exactly 40º south of west, and then leg A size 12{A} {}, which is 12.0 m in a direction exactly 20º west of north. (This problem shows that A+B=B+A.)
28. (a) Repeat the problem two problems prior, but for the second leg you walk 20.0 m in a direction 40.0º north of east (which is equivalent to subtracting B from A —that is, to finding R'=A−B).
(b) Repeat the problem two problems prior, but now you first walk 20.0 m in a direction 40.0º south of west and then 12.0 m in a direction 20.0º east of south (which is equivalent to subtracting A from B —that is, to finding R''=B−A=−R'). Show that this is the case.
Solution
(a) \(26.6 m, 65.1º\) north of east
(b) \(26.6 m, 65.1º\) south of west
29. Show that the order of addition of three vectors does not affect their sum. Show this property by choosing any three vectors A, B and C, all having different lengths and directions. Find the sum A + B + C then find their sum when added in a different order and show the result is the same. (There are five other orders in which A, B, and C can be added; choose only one.)
30. Show that the sum of the vectors discussed in Example gives the result shown in Figure.
Solution
\(52.9 m, 90.1º\) with respect to the x-axis.
31. Find the magnitudes of velocities \(v_A\) and \(v_B\) in Figure
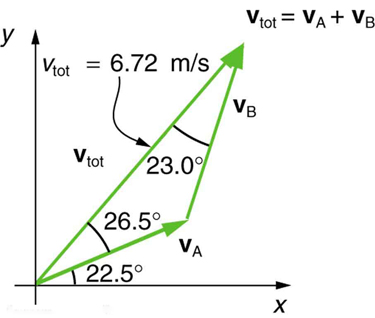
The two velocities \(v_A\) and \(v_B\) add to give a total \(v_{tot}\).
32. Find the components of \(v_{tot}\) along the x- and y-axes in Figure.
Solution
x-component 4.41 m/s
y-component 5.07 m/s
33. Find the components of \(v_{tot}\) along a set of perpendicular axes rotated \(30º\) counterclockwise relative to those in Figure.
3.3: Vector Addition and Subtraction: Analytical Methods
34. Find the following for path C in Figure:
(a) the total distance traveled and
(b) the magnitude and direction of the displacement from start to finish. In this part of the problem, explicitly show how you follow the steps of the analytical method of vector addition.

The various lines represent paths taken by different people walking in a city. All blocks are 120 m on a side.
Solution
(a) 1.56 km
(b) 120 m east
35. Find the following for path D in Figure:
(a) the total distance traveled and
(b) the magnitude and direction of the displacement from start to finish. In this part of the problem, explicitly show how you follow the steps of the analytical method of vector addition.
36. Find the north and east components of the displacement from San Francisco to Sacramento shown in Figure.

Solution
North-component 87.0 km, east-component 87.0 km
37. Solve the following problem using analytical techniques: Suppose you walk 18.0 m straight west and then 25.0 m straight north. How far are you from your starting point, and what is the compass direction of a line connecting your starting point to your final position? (If you represent the two legs of the walk as vector displacements A and B, as in Figure, then this problem asks you to find their sum R=A+B.)

The two displacements A and B add to give a total displacement R having magnitude \(R\) and direction \(θ\).
Note that you can also solve this graphically. Discuss why the analytical technique for solving this problem is potentially more accurate than the graphical technique.
38. Repeat Exercise using analytical techniques, but reverse the order of the two legs of the walk and show that you get the same final result. (This problem shows that adding them in reverse order gives the same result—that is, B + A = A + B.) Discuss how taking another path to reach the same point might help to overcome an obstacle blocking you other path.
Solution
30.8 m, 35.8 west of north
You drive 7.50 km in a straight line in a direction 15º.
(a) Find the distances you would have to drive straight east and then straight north to arrive at the same point. (This determination is equivalent to find the components of the displacement along the east and north directions.)
(b) Show that you still arrive at the same point if the east and north legs are reversed in order.
39. a) Do Exercise again using analytical techniques and change the second leg of the walk to 25.0 m straight south. (This is equivalent to subtracting B from A —that is, finding R'=A – B)
(b) Repeat again, but now you first walk 25.0 m size 12{"25" "." "0 m"} {} north and then 18.0 m size 12{"18" "." "0 m"} {} east. (This is equivalent to subtract A size 12{A} {} from B size 12{B} {} —that is, to find A=B+C size 12{A=B+C} {}. Is that consistent with your result?)
Solution
(a) \(30.8 m, 54.2º\) south of west
(b) \(30.8 m, 54.2º\) north of east
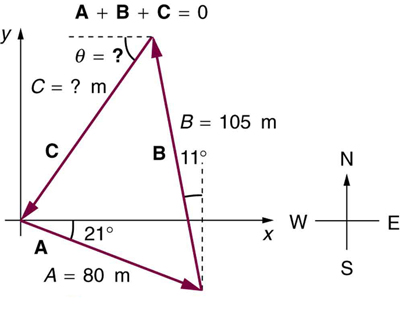
40. A new landowner has a triangular piece of flat land she wishes to fence. Starting at the west corner, she measures the first side to be 80.0 m long and the next to be 105 m. These sides are represented as displacement vectors A from B in Figure. She then correctly calculates the length and orientation of the third side \(C\). What is her result?
41. You fly 32.0 km size 12{"32" "." "0 km"} {} in a straight line in still air in the direction 35.0º size 12{"35"°} {} south of west.
(a) Find the distances you would have to fly straight south and then straight west to arrive at the same point. (This determination is equivalent to finding the components of the displacement along the south and west directions.)
(b) Find the distances you would have to fly first in a direction 45.0º south of west and then in a direction 45.0º west of north. These are the components of the displacement along a different set of axes—one rotated 45º.
Solution
18.4 km south, then 26.2 km west(b) 31.5 km at 45.0º south of west, then 5.56 km at 45.0º west of north
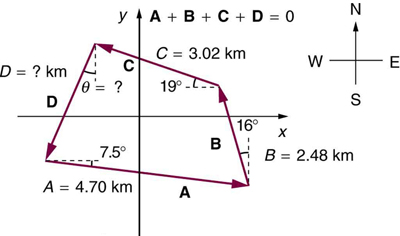
42. A farmer wants to fence off his four-sided plot of flat land. He measures the first three sides, shown as A, B, and C in Figure, and then correctly calculates the length and orientation of the fourth side \(D\). What is his result?
43. In an attempt to escape his island, Gilligan builds a raft and sets to sea. The wind shifts a great deal during the day, and he is blown along the following straight lines: 2.50 km 45.0º north of west; then 4.70 km 60.0º south of east; then 1.30km 25.0º south of west; then 5.10 km straight east; then 1.70km 5.00º east of north; then 7.20 km 55.0º south of west; and finally 2.80 km 10.0º north of east. What is his final position relative to the island?
Solution
\(7.34 km, 63.5º\) south of east
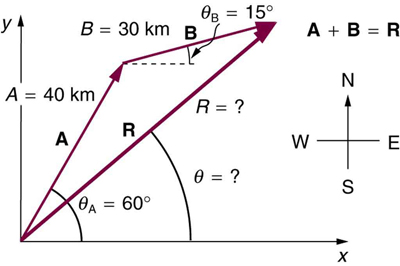
44. Suppose a pilot flies 40.0 km in a direction 60º north of east and then flies 30.0 km in a direction 15º north of east as shown in Figure. Find her total distance \(R\) from the starting point and the direction \(θ\) of the straight-line path to the final position. Discuss qualitatively how this flight would be altered by a wind from the north and how the effect of the wind would depend on both wind speed and the speed of the plane relative to the air mass.
3.4: Projectile Motion
45. A projectile is launched at ground level with an initial speed of 50.0 m/s at an angle of 30.0º above the horizontal. It strikes a target above the ground 3.00 seconds later. What are the \(x\) and \(y\) distances from where the projectile was launched to where it lands?
Solution
\(x=1.30 m×10%2\)
\(y=30.9 m.\)
46. A ball is kicked with an initial velocity of 16 m/s in the horizontal direction and 12 m/s in the vertical direction.
(a) At what speed does the ball hit the ground?
(b) For how long does the ball remain in the air?
(c)What maximum height is attained by the ball?
47. A ball is thrown horizontally from the top of a 60.0-m building and lands 100.0 m from the base of the building. Ignore air resistance.
(a) How long is the ball in the air?
(b) What must have been the initial horizontal component of the velocity?
(c) What is the vertical component of the velocity just before the ball hits the ground? (d) What is the velocity (including both the horizontal and vertical components) of the ball just before it hits the ground?
Solution
(a) 3.50 s
(b) 28.6 m/s
(c) 34.3 m/s
(d) 44.7 m/s, 50.2º below horizontal
48. (a) A daredevil is attempting to jump his motorcycle over a line of buses parked end to end by driving up a 32º ramp at a speed of 40.0 m/s (144 km/h). How many buses can he clear if the top of the takeoff ramp is at the same height as the bus tops and the buses are 20.0 m long?
(b) Discuss what your answer implies about the margin of error in this act—that is, consider how much greater the range is than the horizontal distance he must travel to miss the end of the last bus. (Neglect air resistance.)
49. An archer shoots an arrow at a 75.0 m distant target; the bull’s-eye of the target is at same height as the release height of the arrow.
(a) At what angle must the arrow be released to hit the bull’s-eye if its initial speed is 35.0 m/s? In this part of the problem, explicitly show how you follow the steps involved in solving projectile motion problems.
(b) There is a large tree halfway between the archer and the target with an overhanging horizontal branch 3.50 m above the release height of the arrow. Will the arrow go over or under the branch?
Solution
(a) 18.4º
(b) The arrow will go over the branch.
50. A rugby player passes the ball 7.00 m across the field, where it is caught at the same height as it left his hand.
(a) At what angle was the ball thrown if its initial speed was 12.0 m/s, assuming that the smaller of the two possible angles was used?
(b) What other angle gives the same range, and why would it not be used?
(c) How long did this pass take?
51. Verify the ranges for the projectiles in Figure(a) for \(θ=45º\) and the given initial velocities.
Solution
\(R=\frac{v^2_0}{sin2θ_0g}\)
For \(θ=45º,R=\frac{v^2_0}{g}\)
\(R=91.8m\) for \(v_0=30m/s; R=163m\) for \(v_0=40m/s; R=255m\) for \(v_=50m/s\).
52. Verify the ranges shown for the projectiles in Figure(b) for an initial velocity of 50 m/s at the given initial angles.
53. The cannon on a battleship can fire a shell a maximum distance of 32.0 km.
(a) Calculate the initial velocity of the shell.
(b) What maximum height does it reach? (At its highest, the shell is above 60% of the atmosphere—but air resistance is not really negligible as assumed to make this problem easier.)
(c) The ocean is not flat, because the Earth is curved. Assume that the radius of the Earth is \(6.37×10^3km\). How many meters lower will its surface be 32.0 km from the ship along a horizontal line parallel to the surface at the ship? Does your answer imply that error introduced by the assumption of a flat Earth in projectile motion is significant here?
Solution
(a) 560 m/s
(b) \(8.00×10^3m\)
(c) 80.0 m. This error is not significant because it is only 1% of the answer in part (b).
54. An arrow is shot from a height of 1.5 m toward a cliff of height \(H\) size 12{H} {}. It is shot with a velocity of 30 m/s at an angle of 60º above the horizontal. It lands on the top edge of the cliff 4.0 s later.
(a) What is the height of the cliff?
(b) What is the maximum height reached by the arrow along its trajectory?
(c) What is the arrow’s impact speed just before hitting the cliff?
55. In the standing broad jump, one squats and then pushes off with the legs to see how far one can jump. Suppose the extension of the legs from the crouch position is 0.600 m and the acceleration achieved from this position is 1.25 times the acceleration due to gravity, \(g\) size 12{g} {}. How far can they jump? State your assumptions. (Increased range can be achieved by swinging the arms in the direction of the jump.)
Solution
1.50 m, assuming launch angle of 45º
56. The world long jump record is 8.95 m (Mike Powell, USA, 1991). Treated as a projectile, what is the maximum range obtainable by a person if he has a take-off speed of 9.5 m/s? State your assumptions.
57. Serving at a speed of 170 km/h, a tennis player hits the ball at a height of 2.5 m and an angle \(θ\) below the horizontal. The base line is 11.9 m from the net, which is 0.91 m high. What is the angle \(θ\) such that the ball just crosses the net? Will the ball land in the service box, whose service line is 6.40 m from the net?
Solution
\(θ=6.1º\)
yes, the ball lands at 5.3 m from the net
58. A football quarterback is moving straight backward at a speed of 2.00 m/s when he throws a pass to a player 18.0 m straight downfield.
(a) If the ball is thrown at an angle of 25º relative to the ground and is caught at the same height as it is released, what is its initial speed relative to the ground?
(b) How long does it take to get to the receiver?
(c) What is its maximum height above its point of release?
59. Gun sights are adjusted to aim high to compensate for the effect of gravity, effectively making the gun accurate only for a specific range.
(a) If a gun is sighted to hit targets that are at the same height as the gun and 100.0 m away, how low will the bullet hit if aimed directly at a target 150.0 m away? The muzzle velocity of the bullet is 275 m/s.
(b) Discuss qualitatively how a larger muzzle velocity would affect this problem and what would be the effect of air resistance.
Solution
(a) −0.486 m
(b) The larger the muzzle velocity, the smaller the deviation in the vertical direction, because the time of flight would be smaller. Air resistance would have the effect of decreasing the time of flight, therefore increasing the vertical deviation.
60. An eagle is flying horizontally at a speed of 3.00 m/s when the fish in her talons wiggles loose and falls into the lake 5.00 m below. Calculate the velocity of the fish relative to the water when it hits the water.
61. An owl is carrying a mouse to the chicks in its nest. Its position at that time is 4.00 m west and 12.0 m above the center of the 30.0 cm diameter nest. The owl is flying east at 3.50 m/s at an angle 30.0º size 12{"30º} below the horizontal when it accidentally drops the mouse. Is the owl lucky enough to have the mouse hit the nest? To answer this question, calculate the horizontal position of the mouse when it has fallen 12.0 m.
Solution
4.23 m. No, the owl is not lucky; he misses the nest.
62. Suppose a soccer player kicks the ball from a distance 30 m toward the goal. Find the initial speed of the ball if it just passes over the goal, 2.4 m above the ground, given the initial direction to be 40º above the horizontal.
63. Can a goalkeeper at her/ his goal kick a soccer ball into the opponent’s goal without the ball touching the ground? The distance will be about 95 m. A goalkeeper can give the ball a speed of 30 m/s.
Solution
No, the maximum range (neglecting air resistance) is about 92 m.
64. The free throw line in basketball is 4.57 m (15 ft) from the basket, which is 3.05 m (10 ft) above the floor. A player standing on the free throw line throws the ball with an initial speed of 8.15 m/s, releasing it at a height of 2.44 m (8 ft) above the floor. At what angle above the horizontal must the ball be thrown to exactly hit the basket? Note that most players will use a large initial angle rather than a flat shot because it allows for a larger margin of error. Explicitly show how you follow the steps involved in solving projectile motion problems.
65. In 2007, Michael Carter (U.S.) set a world record in the shot put with a throw of 24.77 m. What was the initial speed of the shot if he released it at a height of 2.10 m and threw it at an angle of 38.0º above the horizontal? (Although the maximum distance for a projectile on level ground is achieved at 45º when air resistance is neglected, the actual angle to achieve maximum range is smaller; thus, 38º will give a longer range than 45º in the shot put.)
Solution
15.0 m/s
66. A basketball player is running at 5.00 m/s directly toward the basket when he jumps into the air to dunk the ball. He maintains his horizontal velocity.
(a) What vertical velocity does he need to rise 0.750 m above the floor?
(b) How far from the basket (measured in the horizontal direction) must he start his jump to reach his maximum height at the same time as he reaches the basket?
67. A football player punts the ball at a 45.0º angle. Without an effect from the wind, the ball would travel 60.0 m horizontally.
(a) What is the initial speed of the ball?
(b) When the ball is near its maximum height it experiences a brief gust of wind that reduces its horizontal velocity by 1.50 m/s. What distance does the ball travel horizontally?
Solution
(a) 24.2 m/s
(b) The ball travels a total of 57.4 m with the brief gust of wind.
68. Prove that the trajectory of a projectile is parabolic, having the form \(y=ax+bx^2\). To obtain this expression, solve the equation \(x=v_{0x}t\) for \(t\) and substitute it into the expression for \(y=v_{0y}t–(1/2)gt^2\) (These equations describe the \(x\) and \(y\) positions of a projectile that starts at the origin.) You should obtain an equation of the form \(y=ax+bx^2\) where \(a\) and \(b\) are constants.
69. Derive \(R=\frac{v^2_0sin2θ_0}{g}\) for the range of a projectile on level ground by finding the time \(t\) at which \(y\) becomes zero and substituting this value of \(t\) into the expression for \(x−x_0\), noting that \(R=x−x_0\)
Solution
\(y−y_0=0=v_{0y}t−\frac{1}{2}gt^2=(v_0sinθ)t−\frac{1}{2}gt^2\)
so that \(t=\frac{2(v0sinθ)}{g}\)
\(x−x_0=v_0xt=(v_0cosθ)t=R,\) and substituting for \(t\) gives:
\(R=v_0cosθ(\frac{2v_0sinθ}{g})=\frac{2v^2_0sinθcosθ}{g}\)
since \(2sinθcosθ=sin2θ\), the range is:
\(R=\frac{v_0^2sin2θ}{g}\)
70. Unreasonable Results
(a) Find the maximum range of a super cannon that has a muzzle velocity of 4.0 km/s.
(b) What is unreasonable about the range you found?
(c) Is the premise unreasonable or is the available equation inapplicable? Explain your answer.
(d) If such a muzzle velocity could be obtained, discuss the effects of air resistance, thinning air with altitude, and the curvature of the Earth on the range of the super cannon.
71. Construct Your Own Problem
Consider a ball tossed over a fence. Construct a problem in which you calculate the ball’s needed initial velocity to just clear the fence. Among the things to determine are; the height of the fence, the distance to the fence from the point of release of the ball, and the height at which the ball is released. You should also consider whether it is possible to choose the initial speed for the ball and just calculate the angle at which it is thrown. Also examine the possibility of multiple solutions given the distances and heights you have chosen.
3.5: Addition of Velocities
72. Bryan Allen pedaled a human-powered aircraft across the English Channel from the cliffs of Dover to Cap Gris-Nez on June 12, 1979.
(a) He flew for 169 min at an average velocity of 3.53 m/s in a direction \(45º\) south of east. What was his total displacement?
(b) Allen encountered a headwind averaging 2.00 m/s almost precisely in the opposite direction of his motion relative to the Earth. What was his average velocity relative to the air?
(c) What was his total displacement relative to the air mass?
Solution
(a) \(35.8 km, 45º\) south of east
(b) \(5.53 m/s, 45º\) south of east
(c) \(56.1 km, 45º\) south of east
73. A seagull flies at a velocity of 9.00 m/s straight into the wind.
(a) If it takes the bird 20.0 min to travel 6.00 km relative to the Earth, what is the velocity of the wind?
(b) If the bird turns around and flies with the wind, how long will he take to return 6.00 km?
(c) Discuss how the wind affects the total round-trip time compared to what it would be with no wind.
74. Near the end of a marathon race, the first two runners are separated by a distance of 45.0 m. The front runner has a velocity of 3.50 m/s, and the second a velocity of 4.20 m/s.
(a) What is the velocity of the second runner relative to the first?
(b) If the front runner is 250 m from the finish line, who will win the race, assuming they run at constant velocity?
(c) What distance ahead will the winner be when she crosses the finish line?
Solution
(a) 0.70 m/s faster
(b) Second runner wins
(c) 4.17 m
75. Verify that the coin dropped by the airline passenger in the Example travels 144 m horizontally while falling 1.50 m in the frame of reference of the Earth.
76. A football quarterback is moving straight backward at a speed of 2.00 m/s when he throws a pass to a player 18.0 m straight downfield. The ball is thrown at an angle of \(25.0º\) relative to the ground and is caught at the same height as it is released. What is the initial velocity of the ball relative to the quarterback ?
Solution
\(17.0 m/s, 22.1º\)
77. A ship sets sail from Rotterdam, The Netherlands, heading due north at 7.00 m/s relative to the water. The local ocean current is 1.50 m/s in a direction \(40.0º\) north of east. What is the velocity of the ship relative to the Earth?
78. (a) A jet airplane flying from Darwin, Australia, has an air speed of 260 m/s in a direction \(5.0º\) south of west. It is in the jet stream, which is blowing at 35.0 m/s in a direction \(15º\) south of east. What is the velocity of the airplane relative to the Earth?
(b) Discuss whether your answers are consistent with your expectations for the effect of the wind on the plane’s path.
Solution
(a) \(230 m/s, 8.0º\) south of west
(b) The wind should make the plane travel slower and more to the south, which is what was calculated.
79. (a) In what direction would the ship in Exercise have to travel in order to have a velocity straight north relative to the Earth, assuming its speed relative to the water remains \(7.00 m/s\)?
(b) What would its speed be relative to the Earth?
80. (a) Another airplane is flying in a jet stream that is blowing at 45.0 m/s in a direction 20º size 12{"20º"} {} south of east (as in Exercise). Its direction of motion relative to the Earth is 45.0º size 12{"45.0º"} {} south of west, while its direction of travel relative to the air is 5.00º size 12{5.00º} {} south of west. What is the airplane’s speed relative to the air mass? (b) What is the airplane’s speed relative to the Earth?
Solution
(a) 63.5 m/s
(b) 29.6 m/s
81. A sandal is dropped from the top of a 15.0-m-high mast on a ship moving at 1.75 m/s due south. Calculate the velocity of the sandal when it hits the deck of the ship:
(a) relative to the ship and
(b) relative to a stationary observer on shore.
(c) Discuss how the answers give a consistent result for the position at which the sandal hits the deck.
82. The velocity of the wind relative to the water is crucial to sailboats. Suppose a sailboat is in an ocean current that has a velocity of 2.20 m/s in a direction \(30.0º\) east of north relative to the Earth. It encounters a wind that has a velocity of 4.50 m/s in a direction of \(50.0º\) south of west relative to the Earth. What is the velocity of the wind relative to the water?
Solution
\(6.68 m/s, 53.3º\) south of west
83. The great astronomer Edwin Hubble discovered that all distant galaxies are receding from our Milky Way Galaxy with velocities proportional to their distances. It appears to an observer on the Earth that we are at the center of an expanding universe. Figure illustrates this for five galaxies lying along a straight line, with the Milky Way Galaxy at the center. Using the data from the figure, calculate the velocities:
(a) relative to galaxy 2 and
(b) relative to galaxy 5.
The results mean that observers on all galaxies will see themselves at the center of the expanding universe, and they would likely be aware of relative velocities, concluding that it is not possible to locate the center of expansion with the given information.
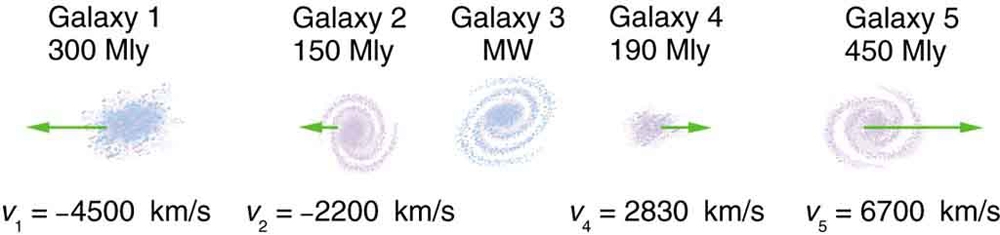
Five galaxies on a straight line, showing their distances and velocities relative to the Milky Way (MW) Galaxy. The distances are in millions of light years (Mly), where a light year is the distance light travels in one year. The velocities are nearly proportional to the distances. The sizes of the galaxies are greatly exaggerated; an average galaxy is about 0.1 Mly across.
84. (a) Use the distance and velocity data in Figure to find the rate of expansion as a function of distance.
(b) If you extrapolate back in time, how long ago would all of the galaxies have been at approximately the same position? The two parts of this problem give you some idea of how the Hubble constant for universal expansion and the time back to the Big Bang are determined, respectively.
Solution
(a) \(H_{average}=14.9\frac{km/s}{Mly}\)
(b) 20.2 billion years
85. An athlete crosses a 25-m-wide river by swimming perpendicular to the water current at a speed of 0.5 m/s relative to the water. He reaches the opposite side at a distance 40 m downstream from his starting point. How fast is the water in the river flowing with respect to the ground? What is the speed of the swimmer with respect to a friend at rest on the ground?
86. A ship sailing in the Gulf Stream is heading \(25.0º\) west of north at a speed of 4.00 m/s relative to the water. Its velocity relative to the Earth is \(4.80 m/s 5.00º\) west of north. What is the velocity of the Gulf Stream? (The velocity obtained is typical for the Gulf Stream a few hundred kilometers off the east coast of the United States.)
Solution
\(1.72 m/s, 42.3º\)north of east
87. An ice hockey player is moving at 8.00 m/s when he hits the puck toward the goal. The speed of the puck relative to the player is 29.0 m/s. The line between the center of the goal and the player makes a \(90.0º\) angle relative to his path as shown in Figure. What angle must the puck’s velocity make relative to the player (in his frame of reference) to hit the center of the goal?
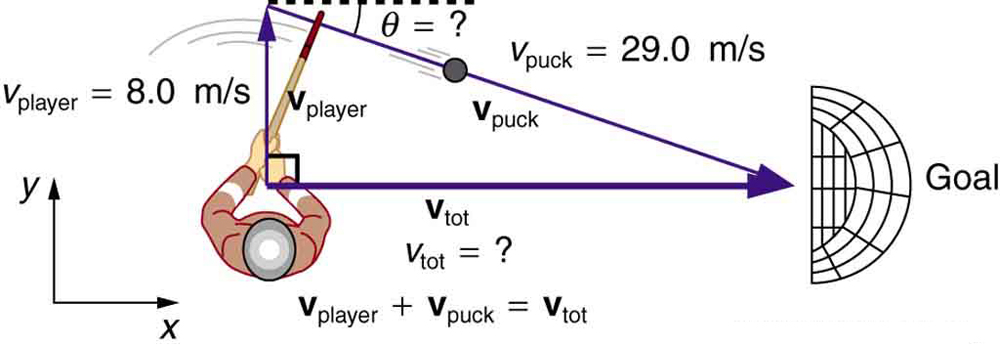
An ice hockey player moving across the rink must shoot backward to give the puck a velocity toward the goal.
88. Unreasonable Results
Suppose you wish to shoot supplies straight up to astronauts in an orbit 36,000 km above the surface of the Earth.
(a) At what velocity must the supplies be launched?
(b) What is unreasonable about this velocity?
(c) Is there a problem with the relative velocity between the supplies and the astronauts when the supplies reach their maximum height?
(d) Is the premise unreasonable or is the available equation inapplicable? Explain your answer.
89. Unreasonable Results
A commercial airplane has an air speed of \(280 m/s\) due east and flies with a strong tailwind. It travels 3000 km in a direction \(5º\) south of east in 1.50 h.
(a) What was the velocity of the plane relative to the ground?
(b) Calculate the magnitude and direction of the tailwind’s velocity.
(c) What is unreasonable about both of these velocities?
(d) Which premise is unreasonable?
90. Construct Your Own Problem
Consider an airplane headed for a runway in a cross wind. Construct a problem in which you calculate the angle the airplane must fly relative to the air mass in order to have a velocity parallel to the runway. Among the things to consider are the direction of the runway, the wind speed and direction (its velocity) and the speed of the plane relative to the air mass. Also calculate the speed of the airplane relative to the ground. Discuss any last minute maneuvers the pilot might have to perform in order for the plane to land with its wheels pointing straight down the runway.
Contributors and Attributions
Paul Peter Urone (Professor Emeritus at California State University, Sacramento) and Roger Hinrichs (State University of New York, College at Oswego) with Contributing Authors: Kim Dirks (University of Auckland) and Manjula Sharma (University of Sydney). This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).


