11.E: Fluid Statics (Exercises)

Conceptual Questions

11.1: What Is a Fluid?

1. What physical characteristic distinguishes a fluid from a solid?

2. Which of the following substances are fluids at room temperature: air, mercury, water, glass?

3. Why are gases easier to compress than liquids and solids?

4. How do gases differ from liquids?

11.2: Density

5. Approximately how does the density of air vary with altitude?

6. Give an example in which density is used to identify the substance composing an object. Would information in addition to average density be needed to identify the substances in an object composed of more than one material?

7. Figure shows a glass of ice water filled to the brim. Will the water overflow when the ice melts? Explain your answer.

11.3: Pressure

8. How is pressure related to the sharpness of a knife and its ability to cut?

9. Why does a dull hypodermic needle hurt more than a sharp one?

10. The outward force on one end of an air tank was calculated in Example. How is this force balanced? (The tank does not accelerate, so the force must be balanced.)

11. Why is force exerted by static fluids always perpendicular to a surface?

12. In a remote location near the North Pole, an iceberg floats in a lake. Next to the lake (assume it is not frozen) sits a comparably sized glacier sitting on land. If both chunks of ice should melt due to rising global temperatures (and the melted ice all goes into the lake), which ice chunk would give the greatest increase in the level of the lake water, if any?

13. How do jogging on soft ground and wearing padded shoes reduce the pressures to which the feet and legs are subjected?

14. Toe dancing (as in ballet) is much harder on toes than normal dancing or walking. Explain in terms of pressure.

15. How do you convert pressure units like millimeters of mercury, centimeters of water, and inches of mercury into units like newtons per meter squared without resorting to a table of pressure conversion factors?

11.4: Variation of Pressure with Depth in a Fluid

16. Atmospheric pressure exerts a large force (equal to the weight of the atmosphere above your body—about 10 tons) on the top of your body when you are lying on the beach sunbathing. Why are you able to get up?

17. Why does atmospheric pressure decrease more rapidly than linearly with altitude?

18. What are two reasons why mercury rather than water is used in barometers?

19. Figure shows how sandbags placed around a leak outside a river levee can effectively stop the flow of water under the levee. Explain how the small amount of water inside the column formed by the sandbags is able to balance the much larger body of water behind the levee.

Because the river level is very high, it has started to leak under the levee. Sandbags are placed around the leak, and the water held by them rises until it is the same level as the river, at which point the water there stops rising.

20. Why is it difficult to swim under water in the Great Salt Lake?

21. Is there a net force on a dam due to atmospheric pressure? Explain your answer.

22. Does atmospheric pressure add to the gas pressure in a rigid tank? In a toy balloon? When, in general, does atmospheric pressure not affect the total pressure in a fluid?

23. You can break a strong wine bottle by pounding a cork into it with your fist, but the cork must press directly against the liquid filling the bottle—there can be no air between the cork and liquid. Explain why the bottle breaks, and why it will not if there is air between the cork and liquid.

11.5: Pascal’s Principle

24. Suppose the master cylinder in a hydraulic system is at a greater height than the slave cylinder. Explain how this will affect the force produced at the slave cylinder.

11.6: Gauge Pressure, Absolute Pressure, and Pressure Measurement

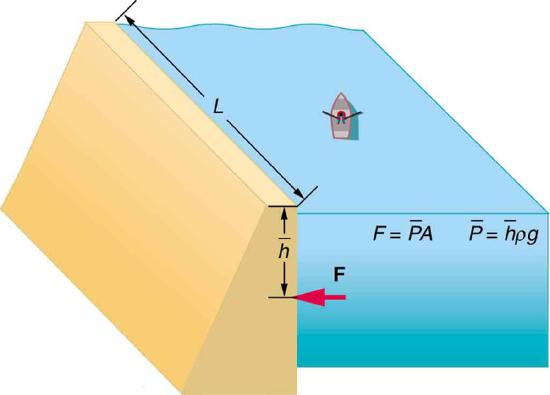
25. Explain why the fluid reaches equal levels on either side of a manometer if both sides are open to the atmosphere, even if the tubes are of different diameters.

26. Figure shows how a common measurement of arterial blood pressure is made. Is there any effect on the measured pressure if the manometer is lowered? What is the effect of raising the arm above the shoulder? What is the effect of placing the cuff on the upper leg with the person standing? Explain your answers in terms of pressure created by the weight of a fluid.

27. Considering the magnitude of typical arterial blood pressures, why are mercury rather than water manometers used for these measurements?

11.7: Archimedes’ Principle

28. More force is required to pull the plug in a full bathtub than when it is empty. Does this contradict Archimedes’ principle? Explain your answer.

29. Do fluids exert buoyant forces in a “weightless” environment, such as in the space shuttle? Explain your answer.

30. Will the same ship float higher in salt water than in freshwater? Explain your answer.

31. Marbles dropped into a partially filled bathtub sink to the bottom. Part of their weight is supported by buoyant force, yet the downward force on the bottom of the tub increases by exactly the weight of the marbles. Explain why.

11.8: Cohesion and Adhesion in Liquids: Surface Tension and Capillary Action

32. The density of oil is less than that of water, yet a loaded oil tanker sits lower in the water than an empty one. Why?

33. Is surface tension due to cohesive or adhesive forces, or both?

34. Is capillary action due to cohesive or adhesive forces, or both?

35. Birds such as ducks, geese, and swans have greater densities than water, yet they are able to sit on its surface. Explain this ability, noting that water does not wet their feathers and that they cannot sit on soapy water.

36. Water beads up on an oily sunbather, but not on her neighbor, whose skin is not oiled. Explain in terms of cohesive and adhesive forces.

37. Could capillary action be used to move fluids in a “weightless” environment, such as in an orbiting space probe?

38. What effect does capillary action have on the reading of a manometer with uniform diameter? Explain your answer.

39. Pressure between the inside chest wall and the outside of the lungs normally remains negative. Explain how pressure inside the lungs can become positive (to cause exhalation) without muscle action.

Problems & Exercises

11.2: Density

40. Gold is sold by the troy ounce (31.103 g). What is the volume of 1 troy ounce of pure gold?

Solution
\(1.610cm^3\)

41. Mercury is commonly supplied in flasks containing 34.5 kg (about 76 lb). What is the volume in liters of this much mercury?

42. (a) What is the mass of a deep breath of air having a volume of 2.00 L?

(b) Discuss the effect taking such a breath has on your body’s volume and density.

Solution
(a) 2.58 g
(b) The volume of your body increases by the volume of air you inhale. The average density of your body decreases when you take a deep breath, because the density of air is substantially smaller than the average density of the body before you took the deep breath.

43. A straightforward method of finding the density of an object is to measure its mass and then measure its volume by submerging it in a graduated cylinder. What is the density of a 240-g rock that displaces \(89.0cm^3\) of water? (Note that the accuracy and practical applications of this technique are more limited than a variety of others that are based on Archimedes’ principle.)

Solution
\(2.70g/cm^3\)

44. Suppose you have a coffee mug with a circular cross section and vertical sides (uniform radius). What is its inside radius if it holds 375 g of coffee when filled to a depth of 7.50 cm? Assume coffee has the same density as water.

45. (a) A rectangular gasoline tank can hold 50.0 kg of gasoline when full. What is the depth of the tank if it is 0.500-m wide by 0.900-m long?

(b) Discuss whether this gas tank has a reasonable volume for a passenger car.

Solution
(a) 0.163 m
(b) Equivalent to 19.4 gallons, which is reasonable

46. A trash compactor can reduce the volume of its contents to 0.350 their original value. Neglecting the mass of air expelled, by what factor is the density of the rubbish increased?

47. A 2.50-kg steel gasoline can holds 20.0 L of gasoline when full. What is the average density of the full gas can, taking into account the volume occupied by steel as well as by gasoline?

Solution
\(7.9×10^2kg/m^3\)

48. What is the density of 18.0-karat gold that is a mixture of 18 parts gold, 5 parts silver, and 1 part copper? (These values are parts by mass, not volume.) Assume that this is a simple mixture having an average density equal to the weighted densities of its constituents.

Solution
\(15.6g/cm^3\)

49. There is relatively little empty space between atoms in solids and liquids, so that the average density of an atom is about the same as matter on a macroscopic scale—approximately \(10^3kg/m^3\). The nucleus of an atom has a radius about \(10^{−5}\) that of the atom and contains nearly all the mass of the entire atom.

(a) What is the approximate density of a nucleus?

(b) One remnant of a supernova, called a neutron star, can have the density of a nucleus. What would be the radius of a neutron star with a mass 10 times that of our Sun (the radius of the Sun is \(7×10^8m\))?

Solution
(a) \(10^{18}kg/m^3\)
(b) \(2×10^4m\)

11.3: Pressure

50. As a woman walks, her entire weight is momentarily placed on one heel of her high-heeled shoes. Calculate the pressure exerted on the floor by the heel if it has an area of \(1.50cm^2\) and the woman’s mass is 55.0 kg. Express the pressure in Pa. (In the early days of commercial flight, women were not allowed to wear high-heeled shoes because aircraft floors were too thin to withstand such large pressures.)

Solution
\(3.59×10^6Pa\); or \(521lb/in^2\)

51. The pressure exerted by a phonograph needle on a record is surprisingly large. If the equivalent of 1.00 g is supported by a needle, the tip of which is a circle 0.200 mm in radius, what pressure is exerted on the record in \(N/m^2\)?

52. Nail tips exert tremendous pressures when they are hit by hammers because they exert a large force over a small area. What force must be exerted on a nail with a circular tip of 1.00 mm diameter to create a pressure of \(3.00×10^9N/m^2\)?(This high pressure is possible because the hammer striking the nail is brought to rest in such a short distance.)

Solution
\(2.36×10^3N\)

11.4: Variation of Pressure with Depth in a Fluid

53. What depth of mercury creates a pressure of 1.00 atm?

Solution
0.760 m

54. The greatest ocean depths on the Earth are found in the Marianas Trench near the Philippines. Calculate the pressure due to the ocean at the bottom of this trench, given its depth is 11.0 km and assuming the density of seawater is constant all the way down.

55. Verify that the SI unit of \(hρg\) is \(N/m^2\).

\((hρg)_{units}=(m)(kg/m^3)(m/s^2)=(kg⋅m^2)/(m^3⋅s^2)\)
\(=(kg⋅m/s^2)(1/m^2)\)
\(=N/m^2\)

56. Water towers store water above the level of consumers for times of heavy use, eliminating the need for high-speed pumps. How high above a user must the water level be to create a gauge pressure of \(3.00×10^5N/m^2\)?

57. The aqueous humor in a person’s eye is exerting a force of 0.300 N on the \(1.10-cm^2\) area of the cornea.

(a) What pressure is this in mm Hg?

(b) Is this value within the normal range for pressures in the eye?

Solution
(a) 20.5 mm Hg
(b) The range of pressures in the eye is 12–24 mm Hg, so the result in part (a) is within that range

58. How much force is exerted on one side of an 8.50 cm by 11.0 cm sheet of paper by the atmosphere? How can the paper withstand such a force?

59. What pressure is exerted on the bottom of a 0.500-m-wide by 0.900-m-long gas tank that can hold 50.0 kg of gasoline by the weight of the gasoline in it when it is full?

Solution
\(1.09×10^3N/m^2\)

60. Calculate the average pressure exerted on the palm of a shot-putter’s hand by the shot if the area of contact is \(50.0cm^2\) and he exerts a force of 800 N on it. Express the pressure in \(N/m^2\) and compare it with the \(1.00×10^6Pa\) pressures sometimes encountered in the skeletal system.

61. The left side of the heart creates a pressure of 120 mm Hg by exerting a force directly on the blood over an effective area of \(15.0cm^2\). What force does it exert to accomplish this?

Solution
24.0 N

62. Show that the total force on a rectangular dam due to the water behind it increases with the square of the water depth. In particular, show that this force is given by \(F=ρgh^2L/2\), where \(ρ\) is the density of water, \(h\) is its depth at the dam, and \(L\) is the length of the dam. You may assume the face of the dam is vertical. (Hint: Calculate the average pressure exerted and multiply this by the area in contact with the water. (See Figure.)

11.5: Pascal’s Principle

63. How much pressure is transmitted in the hydraulic system considered in Example? Express your answer in pascals and in atmospheres.

Solution
\(2.55×10^7Pa\); or 251 atm

64. What force must be exerted on the master cylinder of a hydraulic lift to support the weight of a 2000-kg car (a large car) resting on the slave cylinder? The master cylinder has a 2.00-cm diameter and the slave has a 24.0-cm diameter.

65. A crass host pours the remnants of several bottles of wine into a jug after a party. He then inserts a cork with a 2.00-cm diameter into the bottle, placing it in direct contact with the wine. He is amazed when he pounds the cork into place and the bottom of the jug (with a 14.0-cm diameter) breaks away. Calculate the extra force exerted against the bottom if he pounded the cork with a 120-N force.

Solution
\(5.76×10^3N\) extra force

66. A certain hydraulic system is designed to exert a force 100 times as large as the one put into it.

(a) What must be the ratio of the area of the slave cylinder to the area of the master cylinder?

(b) What must be the ratio of their diameters?

(c) By what factor is the distance through which the output force moves reduced relative to the distance through which the input force moves? Assume no losses to friction.

67. (a) Verify that work input equals work output for a hydraulic system assuming no losses to friction. Do this by showing that the distance the output force moves is reduced by the same factor that the output force is increased. Assume the volume of the fluid is constant.

(b) What effect would friction within the fluid and between components in the system have on the output force? How would this depend on whether or not the fluid is moving?

Solution
(a) \(V=d_iA_i=d_oA_o⇒d_o=d_i(\frac{A_i}{A_o})\).

Now, using equation:

\(\frac{F_1}{A_1}=\frac{F_2}{A_2}⇒F_o=F_i(\frac{A_o}{A_i})\).

Finally,

\(W_o=F_od_o=(\frac{F_iA_o}{A_i})(\frac{d_iA_i}{A_o})=F_id_i=W_i\).

In other words, the work output equals the work input.

(b) If the system is not moving, friction would not play a role. With friction, we know there are losses, so that \(W_{out}=W_{in}−W_f\); therefore, the work output is less than the work input. In other words, with friction, you need to push harder on the input piston than was calculated for the nonfriction case.

11.6: Gauge Pressure, Absolute Pressure, and Pressure Measurement

68. Find the gauge and absolute pressures in the balloon and peanut jar shown in Figure, assuming the manometer connected to the balloon uses water whereas the manometer connected to the jar contains mercury. Express in units of centimeters of water for the balloon and millimeters of mercury for the jar, taking \(h=0.0500 m\) for each.

Solution
Balloon:
Pg=5.00 cmH2O,
Pabs=1.035×103cmH2O.
Jar:
Pg=−50.0 mm Hg,
Pabs=710 mm Hg.

69. (a) Convert normal blood pressure readings of 120 over 80 mm Hg to newtons per meter squared using the relationship for pressure due to the weight of a fluid (\(P=hρg\)) rather than a conversion factor.

(b) Discuss why blood pressures for an infant could be smaller than those for an adult. Specifically, consider the smaller height to which blood must be pumped.

70. How tall must a water-filled manometer be to measure blood pressures as high as 300 mm Hg?

Solution
4.08 m

71. Pressure cookers have been around for more than 300 years, although their use has strongly declined in recent years (early models had a nasty habit of exploding). How much force must the latches holding the lid onto a pressure cooker be able to withstand if the circular lid is \(25.0 cm\) in diameter and the gauge pressure inside is 300 atm? Neglect the weight of the lid.

72. Suppose you measure a standing person’s blood pressure by placing the cuff on his leg 0.500 m below the heart. Calculate the pressure you would observe (in units of mm Hg) if the pressure at the heart were 120 over 80 mm Hg. Assume that there is no loss of pressure due to resistance in the circulatory system (a reasonable assumption, since major arteries are large).

Solution
\(ΔP=38.7 mm Hg\), Leg blood pressure\(=\frac{159}{119}.\)

73. A submarine is stranded on the bottom of the ocean with its hatch 25.0 m below the surface. Calculate the force needed to open the hatch from the inside, given it is circular and 0.450 m in diameter. Air pressure inside the submarine is 1.00 atm.

74. Assuming bicycle tires are perfectly flexible and support the weight of bicycle and rider by pressure alone, calculate the total area of the tires in contact with the ground. The bicycle plus rider has a mass of 80.0 kg, and the gauge pressure in the tires is \(3.50×10^5Pa\).

Solution
\(22.4cm^2\)

11.7: Archimedes’ Principle

75. What fraction of ice is submerged when it floats in freshwater, given the density of water at 0°C is very close to \(1000 kg/m^3\)?

Solution
\(91.7%\)

76. Logs sometimes float vertically in a lake because one end has become water-logged and denser than the other. What is the average density of a uniform-diameter log that floats with 20.0% of its length above water?

77. Find the density of a fluid in which a hydrometer having a density of \(0.750 g/mL\) floats with \(92.0%\) of its volume submerged.

Solution
\(815 kg/m^3\)

78. If your body has a density of \(995 kg/m^3\), what fraction of you will be submerged when floating gently in:

(a) Freshwater?

(b) Salt water, which has a density of \(1027 kg/m^3\)?

79. Bird bones have air pockets in them to reduce their weight—this also gives them an average density significantly less than that of the bones of other animals. Suppose an ornithologist weighs a bird bone in air and in water and finds its mass is \(45.0 g\) and its apparent mass when submerged is \(3.60 g\) (the bone is watertight).

(a) What mass of water is displaced?

(b) What is the volume of the bone?

(c) What is its average density?

Solution
(a) \(41.4 g\)
(b) \(41.4cm^3\)
(c) \(1.09 g/cm^3\)

80. A rock with a mass of 540 g in air is found to have an apparent mass of 342 g when submerged in water.

(a) What mass of water is displaced?

(b) What is the volume of the rock?

(c) What is its average density? Is this consistent with the value for granite?

81. Archimedes’ principle can be used to calculate the density of a fluid as well as that of a solid. Suppose a chunk of iron with a mass of 390.0 g in air is found to have an apparent mass of 350.5 g when completely submerged in an unknown liquid.

(a) What mass of fluid does the iron displace?

(b) What is the volume of iron, using its density as given in [link]

(c) Calculate the fluid’s density and identify it.

Solution
(a) 39.5 g
(b) \(50cm^3\)
(c) \(0.79g/cm^3\)
It is ethyl alcohol.

82. In an immersion measurement of a woman’s density, she is found to have a mass of 62.0 kg in air and an apparent mass of 0.0850 kg when completely submerged with lungs empty.

(a) What mass of water does she displace?

(b) What is her volume?

(c) Calculate her density.

(d) If her lung capacity is 1.75 L, is she able to float without treading water with her lungs filled with air?

83. Some fish have a density slightly less than that of water and must exert a force (swim) to stay submerged. What force must an 85.0-kg grouper exert to stay submerged in salt water if its body density is \(1015kg/m^3\)?

Solution
8.21 N

84. (a) Calculate the buoyant force on a 2.00-L helium balloon.

(b) Given the mass of the rubber in the balloon is 1.50 g, what is the net vertical force on the balloon if it is let go? You can neglect the volume of the rubber.

85. (a) What is the density of a woman who floats in freshwater with \(4.00%\) of her volume above the surface? This could be measured by placing her in a tank with marks on the side to measure how much water she displaces when floating and when held under water (briefly).

(b) What percent of her volume is above the surface when she floats in seawater?

Solution
(a) \(960kg/m^3\)
(b) \(6.34%\)
She indeed floats more in seawater.

86. A certain man has a mass of 80 kg and a density of \(955kg/m^3\) (excluding the air in his lungs).

(a) Calculate his volume.

(b) Find the buoyant force air exerts on him.

(c) What is the ratio of the buoyant force to his weight?

87. A simple compass can be made by placing a small bar magnet on a cork floating in water.

(a) What fraction of a plain cork will be submerged when floating in water?

(b) If the cork has a mass of 10.0 g and a 20.0-g magnet is placed on it, what fraction of the cork will be submerged?

(c) Will the bar magnet and cork float in ethyl alcohol?

Solution
(a) \(0.24\)
(b) \(0.68\)
(c) Yes, the cork will float because \(ρ_{obj}<ρ_{\text{ethyl alcohol}}(0.678g/cm^3<0.79g/cm^3)\)

88. What fraction of an iron anchor’s weight will be supported by buoyant force when submerged in saltwater?

89. Scurrilous con artists have been known to represent gold-plated tungsten ingots as pure gold and sell them to the greedy at prices much below gold value but deservedly far above the cost of tungsten. With what accuracy must you be able to measure the mass of such an ingot in and out of water to tell that it is almost pure tungsten rather than pure gold?

Solution
The difference is 0.006%.

90. A twin-sized air mattress used for camping has dimensions of 100 cm by 200 cm by 15 cm when blown up. The weight of the mattress is 2 kg. How heavy a person could the air mattress hold if it is placed in freshwater?

91. Referring to Figure, prove that the buoyant force on the cylinder is equal to the weight of the fluid displaced (Archimedes’ principle). You may assume that the buoyant force is \(F_2−F_1\) and that the ends of the cylinder have equal areas \(A\). Note that the volume of the cylinder (and that of the fluid it displaces) equals \((h_2−h_1)A\).

Solution
\(F_{net}=F_2−F_1=P_2A−P_1A=(P_2−P_1)A\)
\(=(h_2ρ_{fl}g−h_1ρ_{fl}g)A\)
\(=(h_2−h_1)ρ_{fl}gA\)

where \(ρ_{fl}\) = density of fluid. Therefore,

\(F_{net}=(h_2−h_1)Aρ_{fl}g=V_{fl}ρ_{fl}g=m_{fl}g=w_{fl}\)

where is \(w_{fl}\) the weight of the fluid displaced.

92. (a) A 75.0-kg man floats in freshwater with \(3.00%\) of his volume above water when his lungs are empty, and \(5.00%\) of his volume above water when his lungs are full. Calculate the volume of air he inhales—called his lung capacity—in liters.

(b) Does this lung volume seem reasonable?

11.8: Cohesion and Adhesion in Liquids: Surface Tension and Capillary Action

93. What is the pressure inside an alveolus having a radius of \(2.50×10^{−4}m\) if the surface tension of the fluid-lined wall is the same as for soapy water? You may assume the pressure is the same as that created by a spherical bubble.

Solution
\(592N/m^2\)

94. (a) The pressure inside an alveolus with a \(2.00×10^{−4}\)-m radius is \(1.40×10^3Pa\), due to its fluid-lined walls. Assuming the alveolus acts like a spherical bubble, what is the surface tension of the fluid?

(b) Identify the likely fluid. (You may need to extrapolate between values in Table.)

95. What is the gauge pressure in millimeters of mercury inside a soap bubble 0.100 m in diameter?

Solution
\(2.23×10^{−2}mm Hg\)

96. Calculate the force on the slide wire in Figure if it is 3.50 cm long and the fluid is ethyl alcohol.

97. Figure(a) shows the effect of tube radius on the height to which capillary action can raise a fluid.

(a) Calculate the height \(h\) for water in a glass tube with a radius of 0.900 cm—a rather large tube like the one on the left.

(b) What is the radius of the glass tube on the right if it raises water to 4.00 cm?

Solution
(a) \(1.65×10^{−3}m\)
(b) \(3.71×10^{–4}m\)

98. We stated in Example that a xylem tube is of radius \(2.50×10^{−5}m\). Verify that such a tube raises sap less than a meter by finding h for it, making the same assumptions that sap’s density is \(1050kg/m^3\), its contact angle is zero, and its surface tension is the same as that of water at \(20.0º C\).

99. What fluid is in the device shown in Figure if the force is \(3.16×10^{−3}N\) and the length of the wire is 2.50 cm? Calculate the surface tension \(γ\) and find a likely match from Table.

Solution
\(6.32×10^{−2}N/m\)
Based on the values in table, the fluid is probably glycerin.

100. If the gauge pressure inside a rubber balloon with a 10.0-cm radius is 1.50 cm of water, what is the effective surface tension of the balloon?

101. Calculate the gauge pressures inside 2.00-cm-radius bubbles of water, alcohol, and soapy water. Which liquid forms the most stable bubbles, neglecting any effects of evaporation?

Solution
\(P_w=14.6N/m^2,\)
\(P_a=4.46N/m^2,\)
\(P_{sw}=7.40N/m^2.\)
Alcohol forms the most stable bubble, since the absolute pressure inside is closest to atmospheric pressure.

102. Suppose water is raised by capillary action to a height of 5.00 cm in a glass tube.

(a) To what height will it be raised in a paraffin tube of the same radius?

(b) In a silver tube of the same radius?

103. Calculate the contact angle \(θ\) for olive oil if capillary action raises it to a height of 7.07 cm in a glass tube with a radius of 0.100 mm. Is this value consistent with that for most organic liquids?

Solution
\(5.1º\)
This is near the value of \(θ=0º\) for most organic liquids.

104. When two soap bubbles touch, the larger is inflated by the smaller until they form a single bubble.

(a) What is the gauge pressure inside a soap bubble with a 1.50-cm radius?

(b) Inside a 4.00-cm-radius soap bubble?

(c) Inside the single bubble they form if no air is lost when they touch?

105. Calculate the ratio of the heights to which water and mercury are raised by capillary action in the same glass tube.

Solution
\(−2.78\)
The ratio is negative because water is raised whereas mercury is lowered.

106. What is the ratio of heights to which ethyl alcohol and water are raised by capillary action in the same glass tube?

11.9: Pressures in the Body

107. During forced exhalation, such as when blowing up a balloon, the diaphragm and chest muscles create a pressure of 60.0 mm Hg between the lungs and chest wall. What force in newtons does this pressure create on the \(600cm^2\) surface area of the diaphragm?

Solurion
479 N

108. You can chew through very tough objects with your incisors because they exert a large force on the small area of a pointed tooth. What pressure in pascals can you create by exerting a force of \(500 N\) with your tooth on an area of \(1.00mm^2\)?

109. One way to force air into an unconscious person’s lungs is to squeeze on a balloon appropriately connected to the subject. What force must you exert on the balloon with your hands to create a gauge pressure of 4.00 cm water, assuming you squeeze on an effective area of \(50.0cm^2\)?

Solution
1.96 N

110. Heroes in movies hide beneath water and breathe through a hollow reed (villains never catch on to this trick). In practice, you cannot inhale in this manner if your lungs are more than 60.0 cm below the surface. What is the maximum negative gauge pressure you can create in your lungs on dry land, assuming you can achieve \(−3.00 cm\) water pressure with your lungs 60.0 cm below the surface?

Solution
\(−63.0 cm\) \(H_2O\)

111. Gauge pressure in the fluid surrounding an infant’s brain may rise as high as 85.0 mm Hg (5 to 12 mm Hg is normal), creating an outward force large enough to make the skull grow abnormally large.

(a) Calculate this outward force in newtons on each side of an infant’s skull if the effective area of each side is \(70.0cm^2\).

(b) What is the net force acting on the skull?

112. A full-term fetus typically has a mass of 3.50 kg.

(a) What pressure does the weight of such a fetus create if it rests on the mother’s bladder, supported on an area of \(90.0cm^2\)?

(b) Convert this pressure to millimeters of mercury and determine if it alone is great enough to trigger the micturition reflex (it will add to any pressure already existing in the bladder).

Solution
(a) \(3.81×10^3N/m^2\)
(b) \(28.7 mm Hg\), which is sufficient to trigger micturition reflex

113. If the pressure in the esophagus is \(−2.00 mm Hg\) while that in the stomach is \(+20.0 mm Hg\), to what height could stomach fluid rise in the esophagus, assuming a density of 1.10 g/mL? (This movement will not occur if the muscle closing the lower end of the esophagus is working properly.)

114. Pressure in the spinal fluid is measured as shown in Figure. If the pressure in the spinal fluid is 10.0 mm Hg:

(a) What is the reading of the water manometer in cm water?

(b) What is the reading if the person sits up, placing the top of the fluid 60 cm above the tap? The fluid density is 1.05 g/mL.

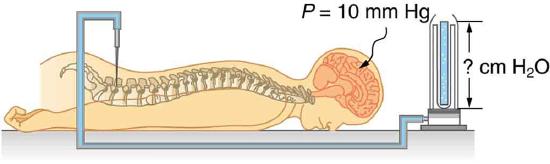
A water manometer used to measure pressure in the spinal fluid. The height of the fluid in the manometer is measured relative to the spinal column, and the manometer is open to the atmosphere. The measured pressure will be considerably greater if the person sits up.

Solution
(a) 13.6 m water
(b) 76.5 cm water

115. Calculate the maximum force in newtons exerted by the blood on an aneurysm, or ballooning, in a major artery, given the maximum blood pressure for this person is 150 mm Hg and the effective area of the aneurysm is \(20.0cm^2\). Note that this force is great enough to cause further enlargement and subsequently greater force on the ever-thinner vessel wall.

116. During heavy lifting, a disk between spinal vertebrae is subjected to a 5000-N compressional force.

(a) What pressure is created, assuming that the disk has a uniform circular cross section 2.00 cm in radius?

(b) What deformation is produced if the disk is 0.800 cm thick and has a Young’s modulus of \(1.5×10^9N/m^2\) ?

Solution
(a) \(3.98×10^6Pa\)
(b) \(2.1×10^{−3}cm\)

117. When a person sits erect, increasing the vertical position of their brain by 36.0 cm, the heart must continue to pump blood to the brain at the same rate.

(a) What is the gain in gravitational potential energy for 100 mL of blood raised 36.0 cm?

(b) What is the drop in pressure, neglecting any losses due to friction?

(c) Discuss how the gain in gravitational potential energy and the decrease in pressure are related.

118. (a) How high will water rise in a glass capillary tube with a 0.500-mm radius?

(b) How much gravitational potential energy does the water gain?

(c) Discuss possible sources of this energy.

Solution
(a) 2.97 cm
(b) \(3.39×10^{−6}J\)
(c) Work is done by the surface tension force through an effective distance \(h/2\) to raise the column of water.

119. A negative pressure of 25.0 atm can sometimes be achieved with the device in Figure before the water separates.

(a) To what height could such a negative gauge pressure raise water?

(b) How much would a steel wire of the same diameter and length as this capillary stretch if suspended from above?

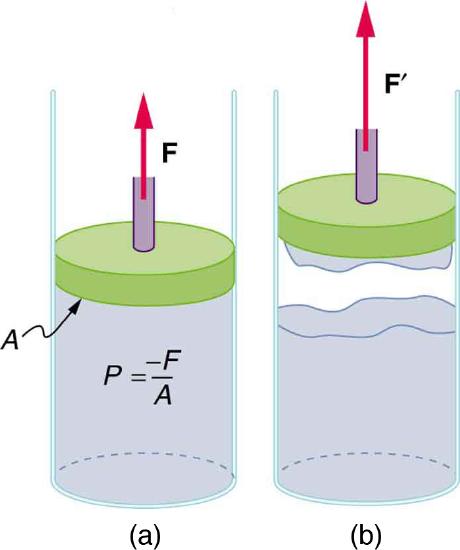
(a) When the piston is raised, it stretches the liquid slightly, putting it under tension and creating a negative absolute pressure \(P=−F/A\) (b) The liquid eventually separates, giving an experimental limit to negative pressure in this liquid.

120. Suppose you hit a steel nail with a 0.500-kg hammer, initially moving at \(15.0 m/s\) size 12{"15" "." 0`"m/s"} {} and brought to rest in 2.80 mm. (a) What average force is exerted on the nail? (b) How much is the nail compressed if it is 2.50 mm in diameter and 6.00-cm long? (c) What pressure is created on the 1.00-mm-diameter tip of the nail?

Solution
(a) \(2.01×10^4N\)
(b) \(1.17×10^{−3}m\)
(c) \(2.56×10^{10}N/m^2\)

121. Calculate the pressure due to the ocean at the bottom of the Marianas Trench near the Philippines, given its depth is \(11.0 km\) and assuming the density of sea water is constant all the way down.

(b) Calculate the percent decrease in volume of sea water due to such a pressure, assuming its bulk modulus is the same as water and is constant.

(c) What would be the percent increase in its density? Is the assumption of constant density valid? Will the actual pressure be greater or smaller than that calculated under this assumption?

122. The hydraulic system of a backhoe is used to lift a load as shown in Figure.

(a) Calculate the force \(F\) the slave cylinder must exert to support the 400-kg load and the 150-kg brace and shovel.

(b) What is the pressure in the hydraulic fluid if the slave cylinder is 2.50 cm in diameter?

(c) What force would you have to exert on a lever with a mechanical advantage of 5.00 acting on a master cylinder 0.800 cm in diameter to create this pressure?

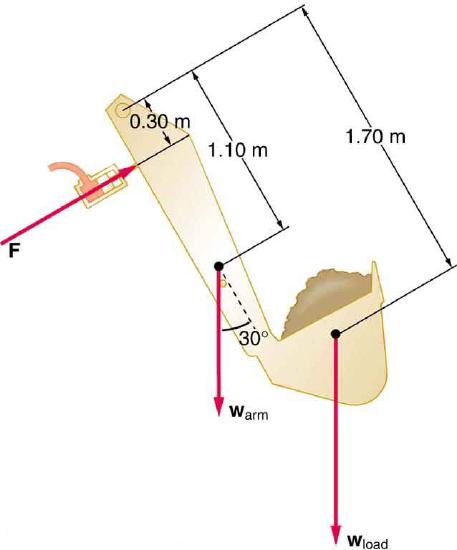
Hydraulic and mechanical lever systems are used in heavy machinery such as this back hoe.

Solution
(a) \(1.38×10^4N\)
(b) \(2.81×10^7N/m^2\)
(c) 283 N

123. Some miners wish to remove water from a mine shaft. A pipe is lowered to the water 90 m below, and a negative pressure is applied to raise the water.

(a) Calculate the pressure needed to raise the water.

(b) What is unreasonable about this pressure?

(c) What is unreasonable about the premise?

124. You are pumping up a bicycle tire with a hand pump, the piston of which has a 2.00-cm radius.

(a) What force in newtons must you exert to create a pressure of \(6.90×10^5Pa\)

(b) What is unreasonable about this (a) result?

(c) Which premises are unreasonable or inconsistent?

Solution
(a) 867 N
(b) This is too much force to exert with a hand pump.
(c) The assumed radius of the pump is too large; it would be nearly two inches in diameter—too large for a pump or even a master cylinder. The pressure is reasonable for bicycle tires.

125. Consider a group of people trying to stay afloat after their boat strikes a log in a lake. Construct a problem in which you calculate the number of people that can cling to the log and keep their heads out of the water. Among the variables to be considered are the size and density of the log, and what is needed to keep a person’s head and arms above water without swimming or treading water.

126. The alveoli in emphysema victims are damaged and effectively form larger sacs. Construct a problem in which you calculate the loss of pressure due to surface tension in the alveoli because of their larger average diameters. (Part of the lung’s ability to expel air results from pressure created by surface tension in the alveoli.) Among the things to consider are the normal surface tension of the fluid lining the alveoli, the average alveolar radius in normal individuals and its average in emphysema sufferers.

Contributors and Attributions

• Paul Peter Urone (Professor Emeritus at California State University, Sacramento) and Roger Hinrichs (State University of New York, College at Oswego) with Contributing Authors: Kim Dirks (University of Auckland) and Manjula Sharma (University of Sydney). This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).