13.1: Alternating current in an inductance

Last updated
Save as PDF

Page ID: 5494

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

In the Figure we see a current increasing to the right and passing through an inductor. As a consequence of the inductance, a back EMF will be induced, with the signs as indicated. I denote the back EMF by \(V = V_A - V_B\). The back EMF is given by \(V=L\dot I\).

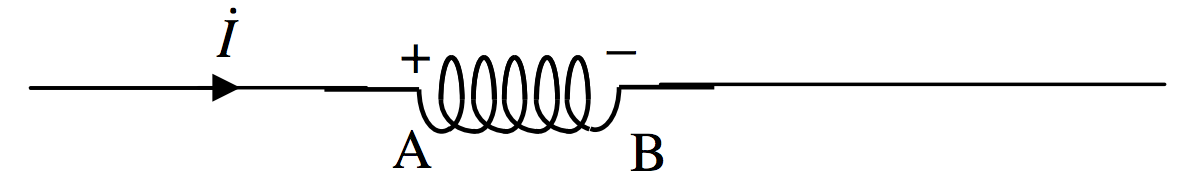
13.1.png
FIGURE \(\text{XIII.1}\)

Now suppose that the current is an alternating current given by

\[\label{13.1.1}I=\hat{I}\sin \omega t.\]

In that case \(\dot I = \hat{I}\omega \cos \omega t\), and therefore the back EMF is

\[\label{13.1.2}V=\hat{I}L\omega \cos \omega t,\]

which can be written

\[\label{13.1.3}V=\hat{V}\cos \omega t,\]

where the peak voltage is

\[\label{13.1.4}\hat{V}=L\omega \hat{I}\]

and, of course \(V_{\text{RMS}}=L\omega I_{\text{RMS}}\) (Section 13.11).

The quantity \(L\omega\) is called the inductive reactance \(X_L\). It is expressed in ohms (check the dimensions), and, the higher the frequency, the greater the reactance. (The frequency \(\nu\) is \(\omega/(2\pi)\).)

Comparison of equations \ref{13.1.1} and \ref{13.1.3} shows that the current and voltage are out of phase, and that \(V\) leads on \(I\) by 90^o, as shown in Figure XIII.2.

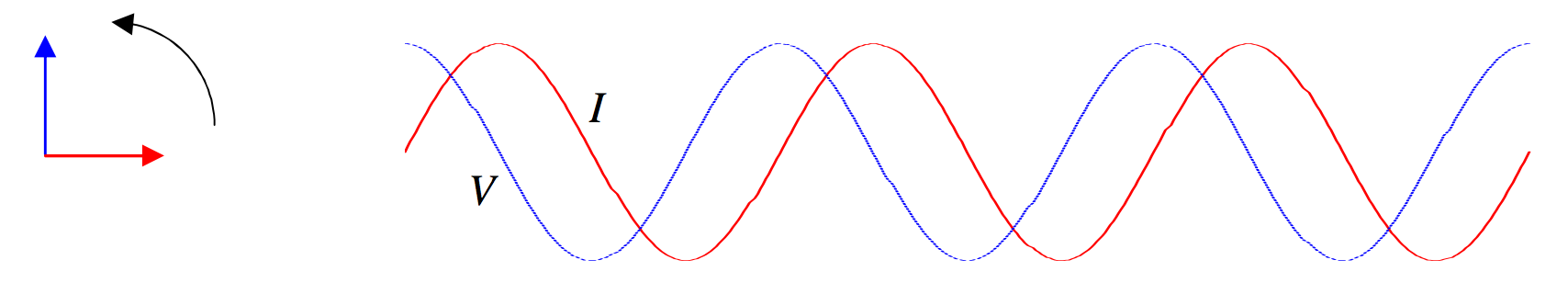
13.2.png

FIGURE \(\text{XIII.2}\)