7.3: Attenuation in Coaxial Cable
- Page ID
- 24824
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In this section, we consider the issue of attenuation in coaxial transmission line. Recall that attenuation can be interpreted in the context of the “lumped element” equivalent circuit transmission line model as the contributions of the resistance per unit length \(R'\) and conductance per unit length \(G'\). In this model, \(R'\) represents the physical resistance in the inner and outer conductors, whereas \(G'\) represents loss due to current flowing directly between the conductors through the spacer material.
The parameters used to describe the relevant features of coaxial cable are shown in Figure \(\PageIndex{1}\). In this figure, \(a\) and \(b\) are the radii of the inner and outer conductors, respectively. \(\sigma_{ic}\) and \(\sigma_{oc}\) are the conductivities (SI base units of S/m) of the inner and outer conductors, respectively. Conductors are assumed to be non-magnetic; i.e., having permeability \(\mu\) equal to the free space value \(\mu_0\). The spacer material is assumed to be a lossy dielectric having relative permittivity \(\epsilon_r\) and conductivity \(\sigma_s\).
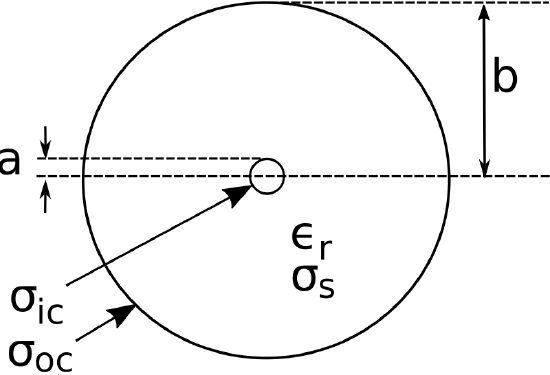 Figure \(\PageIndex{1}\): Parameters defining the design of a coaxial cable.
Figure \(\PageIndex{1}\): Parameters defining the design of a coaxial cable.
Resistance per unit length
The resistance per unit length is the sum of the resistances of the inner and outer conductor per unit length. The resistance per unit length of the inner conductor is determined by \(\sigma_{ic}\) and the effective cross-sectional area through which the current flows. The latter is equal to the circumference \(2\pi a\) times the skin depth \(\delta_{ic}\) of the inner conductor, so:
\[R_{ic}' \approx \frac{1}{\left(2\pi a \cdot \delta_{ic} \right) \sigma_{ic} } ~~~ \mbox{for} ~ \delta_{ic} \ll a \nonumber \]
This expression is only valid for \(\delta_{ic} \ll a\) because otherwise the cross-sectional area through which the current flows is not well-modeled as a thin ring near the surface of the conductor. Similarly, we find the resistance per unit length of the outer conductor is
\[R_{oc}' \approx \frac{1}{\left(2\pi b \cdot \delta_{oc} \right) \sigma_{oc} } ~~~ \mbox{for} ~ \delta_{oc} \ll t \nonumber \]
where \(\delta_{oc}\) is the skin depth of the outer conductor and \(t\) is the thickness of the outer conductor. Therefore, the total resistance per unit length is
\begin{align} R' &= R_{ic}' + R_{oc}' \nonumber \\ & \approx \frac{1}{\left(2\pi a \cdot \delta_{ic} \right) \sigma_{ic} } + \frac{1}{\left(2\pi b \cdot \delta_{oc} \right) \sigma_{oc} } \label{m0189_eRp1} \end{align}
Recall that skin depth depends on conductivity. Specifically:
\begin{align} \delta_{ic} &= \sqrt{2/\omega\mu\sigma_{ic}} \\ \delta_{oc} &= \sqrt{2/\omega\mu\sigma_{oc}} \end{align}
Expanding Equation \ref{m0189_eRp1} to show explicitly the dependence on conductivity, we find:
\[R' \approx \frac{1}{2\pi \sqrt{2/\omega\mu_0} } \left[ \frac{1}{a \sqrt{\sigma_{ic}} } + \frac{1}{b \sqrt{\sigma_{oc}} } \right] \nonumber \]
At this point it is convenient to identify two particular cases for the design of the cable. In the first case, “Case I,” we assume \(\sigma_{oc} \gg \sigma_{ic}\). Since \(b > a\), we have in this case
\begin{align} R' &\approx \frac{1}{2\pi \sqrt{2/\omega\mu_0} } \left[ \frac{1}{a \sqrt{\sigma_{ic}} } \right] \nonumber \\ &= \frac{1}{2\pi \delta_{ic} \sigma_{ic} }~\frac{1}{a} ~~~\mbox{(Case~I)}\end{align}
In the second case, “Case II,” we assume \(\sigma_{oc} = \sigma_{ic}\). In this case, we have
\begin{align} R' &\approx \frac{1}{2\pi \sqrt{2/\omega\mu_0} } \left[ \frac{1}{a \sqrt{\sigma_{ic}} } + \frac{1}{b \sqrt{\sigma_{ic}} } \right] \nonumber \\ &= \frac{1}{2\pi \delta_{ic} \sigma_{ic} }~\left[ \frac{1}{a} + \frac{1}{b} \right] ~~~\mbox{(Case~II)}\end{align}
A simpler way to deal with these two cases is to represent them both using the single expression
\[R' \approx \frac{1}{2\pi \delta_{ic} \sigma_{ic} }~\left[ \frac{1}{a} + \frac{C}{b} \right] \nonumber \]
where \(C=0\) in Case I and \(C=1\) in Case II.
Conductance per unit length
The conductance per unit length of coaxial cable is simply that of the associated coaxial structure at DC; i.e.,
\[G' = \frac{2\pi\sigma_s}{\ln\left(b/a\right)} \nonumber \]
Unlike resistance, the conductance is independent of frequency, at least to the extent that \(\sigma_s\) is independent of frequency.
Attenuation
The attenuation of voltage and current waves as they propagate along the cable is represented by the factor \(e^{-\alpha z}\), where \(z\) is distance traversed along the cable. It is possible to find an expression for \(\alpha\) in terms of the material and geometry parameters using:
\[\gamma \triangleq \sqrt{ \left( R' + j\omega L' \right) \left( G' + j\omega C' \right)} = \alpha + j\beta \label{m0189_fGamma} \]
where \(L'\) and \(C'\) are the inductance per unit length and capacitance per unit length, respectively. These are given by
\[L' = \frac{\mu}{2\pi}\ln{\left(b/a\right)} \nonumber \]
and
\[C' = \frac{2\pi\epsilon_0\epsilon_r}{\ln{\left(b/a\right)}} \nonumber \]
In principle we could solve Equation \ref{m0189_fGamma} for \(\alpha\). However, this course of action is quite tedious, and a simpler approximate approach facilitates some additional insights. In this approach, we define parameters \(\alpha_R\) associated with \(R'\) and \(\alpha_G\) associated with \(G'\) such that
\[e^{-\alpha_R z} e^{-\alpha_G z} = e^{-\left(\alpha_R+\alpha_G\right) z} = e^{-\alpha z} \nonumber \]
which indicates
\[\alpha = \alpha_R + \alpha_G \nonumber \]
Next we postulate
\[\alpha_R \approx K_R \frac{R'}{Z_0} \label{m0189_eAlphaR} \]
where \(Z_0\) is the characteristic impedance
\[Z_0 \approx \frac{\eta_0}{2\pi}\frac{1}{\sqrt{\epsilon_r}}\ln{\frac{b}{a}}~~~\mbox{(low loss)} \label{m0189_eZ0cc} \]
and where \(K_R\) is a unitless constant to be determined. The justification for Equation \ref{m0189_eAlphaR} is as follows: First, \(\alpha_R\) must increase monotonically with increasing \(R'\). Second, \(R'\) must be divided by an impedance in order to obtain the correct units of 1/m. Using similar reasoning, we postulate
\[\alpha_G \approx K_G G' Z_0 \label{m0189_eAlphaG} \]
where \(K_G\) is a unitless constant to be determined. The following example demonstrates the validity of Equations \ref{m0189_eAlphaR} and \ref{m0189_eAlphaG}, and will reveal the values of \(K_R\) and \(K_G\).
RG-59 is a popular form of coaxial cable having the parameters \(a \cong 0.292~\)mm, \(b \cong 1.855\) mm, \(\sigma_{ic} \cong 2.28 \times 10^7\) S/m, \(\sigma_s \cong 5.9 \times 10^{-5}\) S/m, and \(\epsilon_r \cong 2.25\). The conductivity \(\sigma_{oc}\) of the outer conductor is difficult to quantify because it consists of a braid of thin metal strands. However, \(\sigma_{oc}\gg\sigma_{ic}\), so we may assume Case I; i.e., \(\sigma_{oc}\gg\sigma_{ic}\), and subsequently \(C=0\).
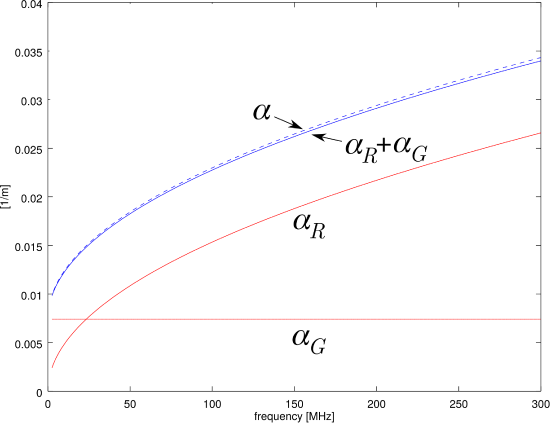 Figure \(\PageIndex{2}\): Comparison of \(\alpha=\operatorname{Re}\{\gamma\}\) to \(\alpha_R\), \(\alpha_G\), and \(\alpha_R + \alpha_G\) for \(K_R = K_G = 1/2\). The result for \(\alpha\) has been multiplied by 1.01; otherwise the curves would be too close to tell apart.
Figure \(\PageIndex{2}\): Comparison of \(\alpha=\operatorname{Re}\{\gamma\}\) to \(\alpha_R\), \(\alpha_G\), and \(\alpha_R + \alpha_G\) for \(K_R = K_G = 1/2\). The result for \(\alpha\) has been multiplied by 1.01; otherwise the curves would be too close to tell apart.
Figure \(\PageIndex{2}\) shows the components \(\alpha_G\) and \(\alpha_R\) computed for the particular choice \(K_R=K_G=1/2\). The figure also shows \(\alpha_G + \alpha_R\), along with \(\alpha\) computed using Equation \ref{m0189_fGamma}. We find that the agreement between these values is very good, which is compelling evidence that the ansatz is valid and \(K_R=K_G=1/2\).
Note that there is nothing to indicate that the results demonstrated in the example are not generally true. Thus, we come to the following conclusion:
The attenuation constant \(\alpha\approx\alpha_G+\alpha_R\) where \(\alpha_G\triangleq R'/2Z_0\) and \(\alpha_R\triangleq G'Z_0/2\).
Minimizing attenuation
Let us now consider if there are design choices which minimize the attenuation of coaxial cable. Since \(\alpha=\alpha_R+\alpha_G\), we may consider \(\alpha_R\) and \(\alpha_G\) independently. Let us first consider \(\alpha_G\):
\begin{align} \alpha_G &\triangleq \frac{1}{2}G'Z_0 \nonumber \\ &\approx \frac{1}{2} \cdot \frac{2\pi\sigma_s}{\ln\left(b/a\right)} \cdot \frac{1}{2\pi} \frac{\eta_0}{\sqrt{\epsilon_r}} \ln\left(b/a\right) \nonumber \\ &= \frac{\eta_0}{2}~\frac{\sigma_s}{\sqrt{\epsilon_r}}\end{align}
It is clear from this result that \(\alpha_G\) is minimized by minimizing \(\sigma_s/\sqrt{\epsilon_r}\). Interestingly the physical dimensions \(a\) and \(b\) have no discernible effect on \(\alpha_G\). Now we consider \(\alpha_R\):
\begin{align} \alpha_R &\triangleq \frac{R'}{2Z_0} \nonumber \\ & = \frac{1}{2} \frac{ \left(1/ 2\pi \delta_{ic} \sigma_{ic}\right)\left[ 1/a + C/b \right] }{ \left(1/2\pi\right) \left( \eta_0/\sqrt{\epsilon_r} \right) \ln\left(b/a\right) } \nonumber \\ &= \frac{\sqrt{\epsilon_r}}{2\eta_0\delta_{ic} \sigma_{ic}} \cdot \frac{ \left[ 1/a + C/b \right] }{ \ln\left(b/a\right) }\end{align}
Now making the substitution \(\delta_{ic} = \sqrt{2/\omega\mu_0\sigma_{ic}}\) in order to make the dependences on the constitutive parameters explicit, we find:
\[\alpha_R = \frac{1}{2\sqrt{2}\cdot\eta_0} \sqrt{\frac{\omega\mu_0 \epsilon_r}{\sigma_{ic}}} \cdot \frac{ \left[ 1/a + C/b \right] }{ \ln\left(b/a\right) } \nonumber \]
Here we see that \(\alpha_R\) is minimized by minimizing \(\epsilon_r/\sigma_{ic}\). It’s not surprising to see that we should maximize \(\sigma_{ic}\). However, it’s a little surprising that we should minimize \(\epsilon_r\). Furthermore, this is in contrast to \(\alpha_G\), which is minimized by maximizing \(\epsilon_r\). Clearly there is a tradeoff to be made here. To determine the parameters of this tradeoff, first note that the result depends on frequency: Since \(\alpha_R\) dominates over \(\alpha_G\) at sufficiently high frequency (as demonstrated in Figure \(\PageIndex{2}\)), it seems we should minimize \(\epsilon_r\) if the intended frequency of operation is sufficiently high; otherwise the optimum value is frequency-dependent. However, \(\sigma_s\) may vary as a function of \(\epsilon_r\), so a general conclusion about optimum values of \(\sigma_s\) and \(\epsilon_r\) is not appropriate.
However, we also see that \(\alpha_R\) – unlike \(\alpha_G\) – depends on \(a\) and \(b\). This implies the existence of a generally-optimum geometry. To find this geometry, we minimize \(\alpha_R\) by taking the derivative with respect to \(a\), setting the result equal to zero, and solving for \(a\) and/or \(b\). Here we go:
\[\frac{\partial}{\partial a}\alpha_R = \frac{1}{2\sqrt{2}\cdot\eta_0} \sqrt{\frac{\omega\mu_0 \epsilon_r}{\sigma_{ic}}} \cdot \frac{\partial}{\partial a} \frac{\left[ 1/a + C/b \right] }{ \ln\left(b/a\right) } \label{m0189_eDAlpha} \]
This derivative is worked out in an addendum at the end of this section. Using the result from the addendum, the right side of Equation \ref{m0189_eDAlpha} can be written as follows:
\[\frac{1}{2\sqrt{2}\cdot\eta_0} \sqrt{\frac{\omega\mu_0 \epsilon_r}{\sigma_{ic}}} \cdot \left[ \frac{-1}{a^2 \ln\left(b/a\right) } + \frac{ 1/a + C/b }{a \ln^2\left(b/a\right) } \right] \label{m0189_eDAlpha2} \]
In order for \(\partial\alpha_R/\partial a=0\), the factor in the square brackets above must be equal to zero. After a few steps of algebra, we find:
\[\ln\left(b/a\right) = 1+\frac{C}{b/a} \nonumber \]
In Case I (\(\sigma_{oc} \gg \sigma_{ic}\)), \(C=0\) so:
\[b/a=e\cong 2.72 ~~~ \mbox{(Case I)} \nonumber \]
In Case II (\(\sigma_{oc} = \sigma_{ic}\)), \(C=1\). The resulting equation can be solved by plotting the function, or by a few iterations of trial and error; either way one quickly finds
\[b/a\cong 3.59 ~~~ \mbox{(Case II)} \nonumber \]
Summarizing, we have found that \(\alpha\) is minimized by choosing the ratio of the outer and inner radii to be somewhere between \(2.72\) and \(3.59\), with the precise value depending on the relative conductivity of the inner and outer conductors.
Substituting these values of \(b/a\) into Equation \ref{m0189_eZ0cc}, we obtain:
\[Z_0 \approx \frac{59.9~\Omega}{\sqrt{\epsilon_r}} ~~\mbox{to}~~ \frac{76.6~\Omega}{\sqrt{\epsilon_r}} \label{m0189_eZ0Opt} \]
as the range of impedances of coaxial cable corresponding to physical designs that minimize attenuation.
Equation \ref{m0189_eZ0Opt} gives the range of characteristic impedances that minimize attenuation for coaxial transmission lines. The precise value within this range depends on the ratio of the conductivity of the outer conductor to that of the inner conductor.
Since \(\epsilon_r\ge 1\), the impedance that minimizes attenuation is less for dielectric-filled cables than it is for air-filled cables. For example, let us once again consider the RG-59 from Example \(\PageIndex{1}\). In that case, \(\epsilon_r\cong 2.25\) and \(C=0\), indicating \(Z_0\approx 39.9~\Omega\) is optimum for attenuation. The actual characteristic impedance of \(Z_0\) is about \(75~\Omega\), so clearly RG-59 is not optimized for attenuation. This is simply because other considerations apply, including power handling capability (addressed in Section 7.4) and the convenience of standard values (addressed in Section 7.5).
Addendum: Derivative of \(a^2\ln(b/a)\)
Evaluation of Equation \ref{m0189_eDAlpha} requires finding the derivative of \(a^2\ln(b/a)\) with respect to \(a\). Using the chain rule, we find:
\begin{align} \frac{\partial}{\partial a}\left[ a^2\ln\left(\frac{b}{a}\right) \right] = &\left[ \frac{\partial}{\partial a} a^2 \right] \ln\left(\frac{b}{a}\right) \nonumber \\ + &a^2 \left[ \frac{\partial}{\partial a} \ln\left(\frac{b}{a}\right) \right]\end{align}
Note
\[\frac{\partial}{\partial a} a^2 = 2a \nonumber \]
and
\begin{align} \frac{\partial}{\partial a} \ln\left(\frac{b}{a}\right) &= \frac{\partial}{\partial a} \left[ \ln\left(b\right) - \ln\left(a\right) \right] \nonumber \\ &=-\frac{\partial}{\partial a}\ln\left(a\right) \nonumber \\ &=-\frac{1}{a}\end{align}
So:
\begin{align} \frac{\partial}{\partial a}\left[ a^2\ln\left(\frac{b}{a}\right) \right] &= \left[ 2a \right] \ln\left(\frac{b}{a}\right) + a^2 \left[ -\frac{1}{a} \right] \nonumber \\ &= \boxed{ 2a \ln\left(\frac{b}{a}\right) - a } \end{align}
This result is substituted for \(a^2\ln(b/a)\) in Equation \ref{m0189_eDAlpha} to obtain Equation \ref{m0189_eDAlpha2}.


