5.2: Solution
( \newcommand{\kernel}{\mathrm{null}\,}\)
As stated before the continuity arguments for the derivative of the wave function do not apply for an infinite jump in the potential energy. This is easy to understand as we look at the behaviour of a low energy solution in one of the two outside regions (I or III). In this case the wave function can be approximated as
e±kr,k=√2mℏ2V0,
which decreases to zero faster and faster as V 0 becomes larger and larger. In the end the wave function can no longer penetrate the region of infinite potential energy. Continuity of the wave function now implies that ϕ( a)=ϕ(− a)= 0.
Defining
κ=√2mℏ2E,
we find that there are two types of solutions that satisfy the boundary condition:
ϕ2n+1(x)=cos(κ2n+1x),ϕ2n(x)=sin(κ2nx).
Here
κl=πl2a.
We thus have a series of eigen states ϕl(x),l=1,⋯,∞. The energies are
El=ℏ2π2l28a2
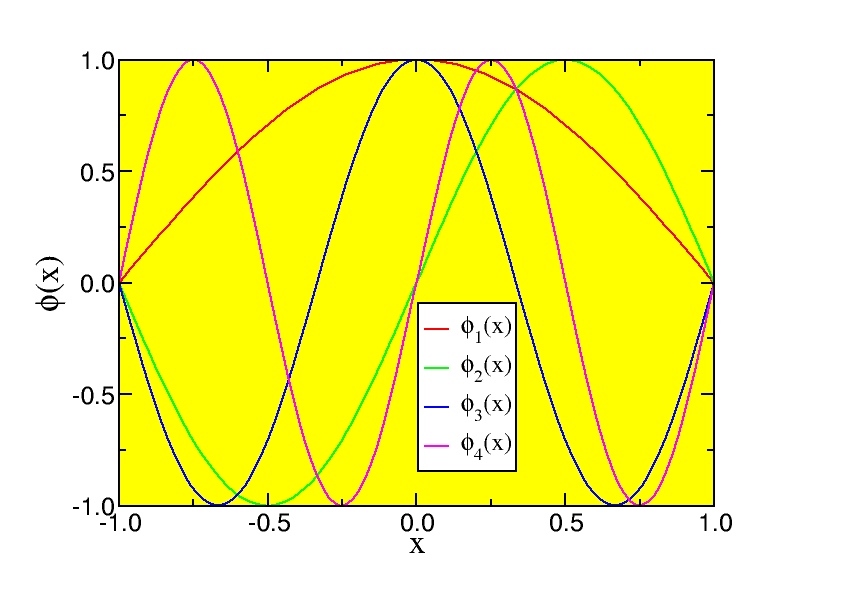
These wave functions are very good to illustrate the idea of normalisation. Let me look at the normalisation of the ground state (the lowest state), which is
We need to require
∫∞−∞|ϕ0(x)|2dx=1
where we need to consider the absolute value since A1 can be complex. We only have to integrate from −a to a, since the rest of the integral is zero, and we have
∫∞−∞|ϕ0(x)|2dx=|A|2∫a−acos2[πx2a]dx=|A|22aπ∫π2−π2cos2⌈y⌉dy=|A|22aπ∫π2−π212(1+cos⌈2y[])dy=|A|22aππ
Here we have changed variables from x to y=πx2a. We thus conclude that the choice
A=√12a
leads to a normalised wave function.


