4.4: The Heat Conduction Equation
( \newcommand{\kernel}{\mathrm{null}\,}\)
The situation described in Section 4.2 and in figure IV.1 was a steady-state situation, in which the temperature was a function of x but not of time. We are now going to consider a more general situation in which the temperature may vary in time as well as in space.
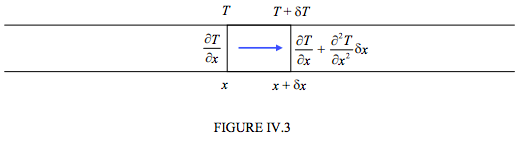
In this case the temperature gradient is written as a partial derivative, \( \frac{\partial T}{\partial x} and is not uniform down the length of the rod. We'll suppose it is \frac{ \deta T}{\partial x} at x and ∂T∂x+∂2T∂x2δx at x + δx.
Consider the heat flow into and out of the portion between x and x + δx. The rate of flow into this portion at x is −KA∂T∂x, and the rate of flow out at x + δx is −KA(∂T∂x+∂2T∂x2δx), so that the net flow of heat into that portion is KA∂2T∂x2δx. This must be equal to CρAδx∂T∂t, where ρ is the density (and hence ρAδx is the mass of the portion), and C is the specific heat capacity.
Therefore
Cρ∂T∂t=K∂2T∂x2.
This can be written
∂T∂t=D∂2T∂x2,
where
D=KCρ
is the thermal diffusivity (m2 s−1).
Equation 4.3.2 is the heat conduction equation. In three dimensions it is easy to show that it becomes
T=D∇2T.


