2.A: Vectors (Answers)
( \newcommand{\kernel}{\mathrm{null}\,}\)
Check Your Understanding
2.1. a. not equal because they are orthogonal;
b. not equal because they have different magnitudes;
c. not equal because they have different magnitudes and directions;
d. not equal because they are antiparallel;
e. equal.
2.2. 16 m; →D=−16mˆu
2.3. G = 28.2 cm, θG=291°
2.4. →D=(−5.0ˆi−3.0ˆj)cm; the fly moved 5.0 cm to the left and 3.0 cm down from its landing site.
2.5. 5.83 cm, 211°
2.6. →D=(−20m)ˆj
2.7. 35.1 m/s = 126.4 km/h
2.8. →G=(10.25ˆi−26.22ˆj)cm
2.9. D = 55.7 N; direction 65.7° north of east
2.10. ˆv=0.8ˆi+0.6ˆj,36.87° north of east
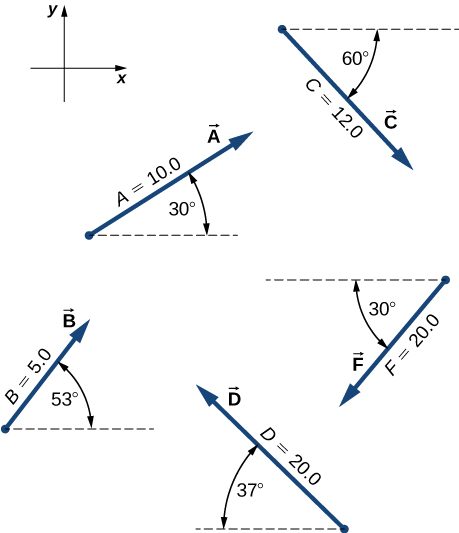
2.11. →A⋅→B=−57.3,→F⋅→C=27.8
2.13. 131.9°
2.14. W1=1.5J,W2=0.3J
2.15. →A×→B=−40.1ˆk or, equivalently, ∣→A×→B∣=40.1, and the direction is into the page; →C×→F=+157.6ˆk or, equivalently, ∣→C×→F∣=157.6, and the direction is out of the page.
2.16. a. −2ˆk,
b. 2,
c. 153.4°,
d. 135°
Conceptual Questions
1. scalar
3. answers may vary
5. parallel, sum of magnitudes, antiparallel, zero
7. no, yes
9. zero, yes
11. no
13. equal, equal, the same
15. a unit vector of the x-axis
17. They are equal.
19. yes
21. a. C=→A⋅→B
b. →C=→A·→B or →C=→A−→B
c. →C=→A×→B,
d. →C=A→B,
e. →C+2→A=→B,
f. →C=→A×→B,
g. left side is a scalar and right side is a vector,
h. →C=2→A×→B,
i. →C=→A/B,
j. →C=→A/B
23. They are orthogonal.
Problems
25. →h=−49mˆu, 49 m
27. 30.8 m, 35.7° west of north
29. 134 km, 80°
31. 7.34 km, 63.5° south of east
33. 3.8 km east, 3.2 km north, 7.0 km
35. 14.3 km, 65°
37. a. →A=+8.66ˆi+5.00ˆj,
b. →B=+3.01ˆi+3.99ˆj,
c. →C=+6.00ˆi−10.39ˆj,
d. →D⃗=−15.97ˆi+12.04ˆj,
f. →F=−17.32ˆi−10.00ˆj
39. a. 1.94 km, 7.24 km;
b. proof
41. 3.8 km east, 3.2 km north, 2.0 km, \(\vec{D}=(3.8\hat{i}+3.2\hat{j})km
43. P1(2.165m,1.250m),P2(−1.900m,3.290m),5.27m
45. 8.60 m, A(2√5m,0.647π),B(3√2m,0.75π)
47. a. →A+→B=−4ˆi−6ˆj, |→A+→B∣=7.211,θ=236.3°;
b. →A−→B=−2ˆi+2ˆj,∣→A−→B∣=2√2,θ=135°
49. a. →C=(5.0ˆi−1.0ˆj−3.0ˆk)m,C=5.92m;
b. →D=(4.0ˆi−11.0ˆj+15.0ˆk)m,D=19.03m.
51. →D=(3.3ˆi−6.6ˆj)km,ˆiistotheeast,7.34km,−63.5°
53. a.→R=−1.35ˆi−22.04ˆj,
b. →R=−17.98ˆi+0.89ˆj
55. →D=(200ˆi+300ˆj)yd, D = 360.5 yd, 56.3° north of east; The numerical answers would stay the same but the physical unit would be meters. The physical meaning and distances would be about the same because 1 yd is comparable with 1 m.
57. →R=−3ˆi−16ˆj
59. →E⃗=EˆE,Ex=+178.9V/m,Ey=−357.8V/m,Ez=0.0V/m,θE=−tan−1(2)
61. a. →RB=(12.278ˆi+7.089ˆj+2.500ˆk)km,\(→RD=(−0.262ˆi+3.000ˆk)km;
b. ∣→RB−→RD∣=14.414km|R→B−R→D|=14.414km
63. a. 8.66,
b. 10.39,
c. 0.866,
d. 17.32
65. θi=64.12°,θj=150.79°,θk=77.39°
67. a. −119.98ˆk
b. 0ˆk,
c. +93.69ˆk,
d. −240.0ˆk,
e. +3.993ˆk,
f. −3.009ˆk,
g. +14.99ˆk,
h. 0
69. a. 0,
b. 173,194,
c. +199,993ˆk
Additional Problems
71. a. 18.4 km and 26.2 km,
b. 31.5 km and 5.56 km
73. a. (r,φ+π/2),
b. (2r,φ+2π)(,
c. (3r,−φ)
75. dPM=33.12nmi=61.34km,dNP=35.47nmi=65.69km
77. proof
79. a. 10.00 m,
b. 5πm,
c. 0
81. 22.2 km/h, 35.8°. south of west
83. 240.2 m, 2.2° south of west
85. →B=−4.0ˆi+3.0ˆj or →B=4.0ˆi−3.0ˆj
87. proof
Challenge Problems
89. G⊥=2375√17≈9792
91. proof


