Skip to main content
Physics LibreTexts

18.2: Vectors

  1. Last updated
  2. Save as PDF
  • Page ID
    7941

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

    \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

    \( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

    ( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

    \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

    \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

    \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

    \( \newcommand{\Span}{\mathrm{span}}\)

    \( \newcommand{\id}{\mathrm{id}}\)

    \( \newcommand{\Span}{\mathrm{span}}\)

    \( \newcommand{\kernel}{\mathrm{null}\,}\)

    \( \newcommand{\range}{\mathrm{range}\,}\)

    \( \newcommand{\RealPart}{\mathrm{Re}}\)

    \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

    \( \newcommand{\Argument}{\mathrm{Arg}}\)

    \( \newcommand{\norm}[1]{\| #1 \|}\)

    \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

    \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    \( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

    \( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

    \( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

    \( \newcommand{\vectorC}[1]{\textbf{#1}} \)

    \( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

    \( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

    \( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

    \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

    \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

    \(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)

    Check Your Understanding

    2.1. a. Not equal because they are orthogonal; b. not equal because they have different magnitudes; c. not equal because they have different magnitudes and directions; d. not equal because they are antiparallel; e. equal.

    2.2. 16 m; \(\vec{D}\) = −16 m \(\hat{u}\)

    2.3. G = 28.2 cm, \(\theta_{G}\) = 291°

    2.4. \(\vec{D}\) = (−5.0 \(\hat{i}\) − 3.0 \(\hat{j}\))cm; the fly moved 5.0 cm to the left and 3.0 cm down from its landing site.

    2.5. 5.83 cm, 211°

    2.6. \(\vec{D}\) = (−20 m) \(\hat{j}\)

    2.7. 35.1 m/s = 126.4 km/h

    2.8. \(\vec{G}\) = (10.25 \(\hat{i}\) − 26.22 \(\hat{j}\))cm

    2.9. D = 55.7 N; direction 65.7° north of east

    2.10. \(\hat{v}\) = 0.8 \(\hat{i}\) + 0.6 \(\hat{j}\), 36.87° north of east

    2.11. \(\vec{A} \cdotp \vec{B}\) = −57.3, \(\vec{F} \cdotp \vec{C}\) = 27.8

    2.13. 131.9°

    2.14. W1 = 1.5 J, W2 = 0.3 J

    2.15. \(\vec{A} \times \vec{B}\) = −40.1 \(\hat{k}\) or, equivalently, |\(\vec{A} \times \vec{B}\)| = 40.1, and the direction is into the page; \(\vec{C} \times \vec{F}\) = + 157.6 \(\hat{k}\) or, equivalently, |\(\vec{C} \times \vec{F}\)| = 157.6, and the direction is out of the page.

    2.16. a. −2 \(\hat{k}\), b. 2, c. 153.4°, d. 135°

    Conceptual Questions

    1. Scalar

    3. Answers may vary

    5. Parallel, sum of magnitudes, antiparallel, zero

    7. Yes, yes

    9. Zero, yes

    11. No

    13. Equal, equal, the same

    15. A unit vector of the x-axis

    17. They are equal.

    19. Yes

    21. a. C = \(\vec{A} \cdotp \vec{B}\), b. \(\vec{C} = \vec{A} \times \vec{B}\) or \(\vec{C} = \vec{A} - \vec{B}\), c. \(\vec{C} = \vec{A} \times\vec{B}\), d. \(\vec{C}\) = A\(\vec{B}\), e. \(\vec{C} + 2 \vec{A} = \vec{B}\), f. \(\vec{C} = \vec{A} \times \vec{B}\), g. left side is a scalar and right side is a vector, h. \(\vec{C} = 2 \vec{A} \times \vec{B}\), i. \(\vec{C} = \frac{\vec{A}}{B}\), j. \(\vec{C} = \frac{\vec{A}}{B}\)

    23. They are orthogonal.

    Problems

    25. \(\vec{h}\) = −16.4 m \(\hat{u}\), 16.4 m

    27. 30.8 m, 35.7° west of north

    29. 134 km, 80°

    31. 7.34 km, 63.5° south of east

    33. 3.8 km east, 3.2 km north, 7.0 km

    35. 14.3 km, 65°

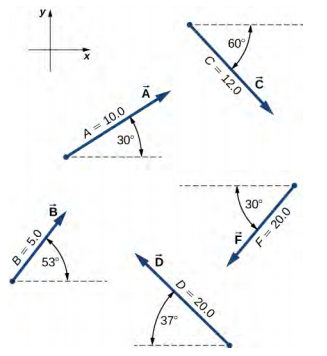
    37. a. \(\vec{A}\) = + 8.66 \(\hat{i}\) + 5.00 \(\hat{j}\)

    b. \(\vec{B}\) = + 3.01 \(\hat{i}\) + 3.99 \(\hat{j}\)

    c. \(\vec{C}\) = + 6.00 \(\hat{i}\) − 10.39 \(\hat{j}\)

    d. \(\vec{D}\) = −15.97 \(\hat{i}\) + 12.04 \(\hat{j}\)

    f. \(\vec{F}\) = −17.32 \(\hat{i}\) − 10.00 \(\hat{j}\)

    The x y coordinate system has positive x to the right and positive y up. Vector A has magnitude 10.0 and points 30 degrees counterclockwise from the positive x direction. Vector B has magnitude 5.0 and points 53 degrees counterclockwise from the positive x direction. Vector C has magnitude 12.0 and points 60 degrees clockwise from the positive x direction. Vector D has magnitude 20.0 and points 37 degrees clockwise from the negative x direction. Vector F has magnitude 20.0 and points 30 degrees counterclockwise from the negative x direction.

    39. a. 1.94 km, 7.24 km

    b. proof

    41. 3.8 km east, 3.2 km north, 2.0 km, \(\vec{D}\) = (3.8 \(\hat{i}\) + 3.2 \(\hat{j}\))km

    43. P1(2.165 m, 1.250 m), P2(−1.900 m, 3.290 m), 5.27 m

    45. 8.60 m, A(2\(\sqrt{5}\) m, 0.647\(\pi\)), B(3\(\sqrt{2}\) m, 0.75\(\pi\))

    47. a. \(\vec{A} + \vec{B}\) = −4 \(\hat{i}\) − 6 \(\hat{j}\), |\(\vec{A} + \vec{B}\)| = 7.211, \(\theta\) = 236.3°

    b. \(\vec{A} -\vec{B}\) = -2 \(\hat{i}\) + 2 \(\hat{j}\), |\(\vec{A} - \vec{B}\)| = 2\(\sqrt{2}\), \(\theta\) = 135°

    49. a. \(\vec{C}\) = (5.0 \(\hat{i}\) − 1.0 \(\hat{j}\) − 3.0 \(\hat{k}\))m, C = 5.92 m

    b. \(\vec{D}\) = (4.0 \(\hat{i}\) − 11.0 \(\hat{j}\) + 15.0 \(\hat{k}\))m, D = 19.03 m

    51. \(\vec{D}\) = (3.3 \(\hat{i}\) − 6.6 \(\hat{j}\))km, \(\hat{i}\) is to the east, 7.34 km, −63.5°

    53. a. \(\vec{R}\) = −1.35 \(\hat{i}\) − 22.04 \(\hat{j}\)

    b. \(\vec{R}\) = −17.98 \(\hat{i}\) + 0.89 \(\hat{j}\)

    55. \(\vec{D}\) = (200 \(\hat{i}\) + 300 \(\hat{j}\))yd, D = 360.5 yd, 56.3° north of east; The numerical answers would stay the same but the physical unit would be meters. The physical meaning and distances would be about the same because 1 yd is comparable with 1 m.

    57. \(\vec{R}\) = −3 \(\hat{i}\) − 16 \(\hat{j}\)

    59. \(\vec{E}\) = E \(\hat{E}\), Ex = + 178.9 V/m , Ey = −357.8 V/m, Ez = 0.0 V/m, \(\theta_{E}\) = −tan−1(2)

    61. a. \(\vec{R}_{B}\) = (12.278 \(\hat{i}\) + 7.089 \(\hat{j}\) + 2.500 \(\hat{k}\))km, \(\vec{R}_{D}\) = (−0.262 \(\hat{i}\) + 3.000 \(\hat{k}\))km

    b. |\(\vec{R}_{B} − \vec{R}_{D}\)| = 14.414 km

    63. a. 8.66

    b. 10.39

    c. 0.866

    d. 17.32

    65. \(\theta_{i}\) = 64.12°, \(\theta_{j}\) = 150.79°, \(\theta_{k}\) = 77.39°

    67. a. −119.98 \(\hat{k}\)

    b. 0 \(\hat{k}\)

    c. +93.69 \(\hat{k}\)

    d. −240.0 \(\hat{k}\)

    e. +3.993 \(\hat{k}\)

    f. −3.009 \(\hat{k}\)

    g. +14.99 \(\hat{k}\)

    h. 0

    69. a. 0

    b. 173,194

    c. +199,993 \(\hat{k}\)

    Additional Problems

    71. a. 18.4 km and 26.2 km

    b. 31.5 km and 5.56 km

    73. a. (r, \(\phi + \frac{\pi}{2}\))

    b. (2r, \(\phi + 2 \pi\))

    c. (3r, −\(\phi\))

    75. dPM = 33.12 nmi = 61.34 km, dNP = 35.47 nmi = 65.69 km

    77. proof

    79. a. 10.00 m

    b. 5\(\pi\) m, c. 0

    81. 22.2 km/h, 35.8° south of west

    83. 240.2 m, 2.2° south of west

    85. \(\vec{B}\) = −4.0 \(\hat{i}\) + 3.0 \(\hat{j}\) or \(\vec{B}\) = 4.0 \(\hat{i}\) − 3.0 \(\hat{j}\)

    87. proof

    Challenge Problems

    89. G\(\perp\) = 2375\(\sqrt{17}\) ≈ 9792

    91. proof

    Contributors and Attributions

    Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).

    This page titled 18.2: Vectors is shared under a CC BY 4.0 license and was authored, remixed, and/or curated by OpenStax via source content that was edited to the style and standards of the LibreTexts platform.