13.3: Temperature and Heat (Exercises)

Conceptual Questions

 

1. Heat transfer can cause temperature and phase changes. What else can cause these changes?
2. How does the latent heat of fusion of water help slow the decrease of air temperatures, perhaps preventing temperatures from falling significantly below 0°C, in the vicinity of large bodies of water?
3. What is the temperature of ice right after it is formed by freezing water?
4. If you place 0°C ice into 0°C water in an insulated container, what will the net result be? Will there be less ice and more liquid water, or more ice and less liquid water, or will the amounts stay the same?
5. What effect does condensation on a glass of ice water have on the rate at which the ice melts? Will the condensation speed up the melting process or slow it down?
6. In Miami, Florida, which has a very humid climate and numerous bodies of water nearby, it is unusual for temperatures to rise above about 38°C (100°F). In the desert climate of Phoenix, Arizona, however, temperatures rise above that almost every day in July and August. Explain how the evaporation of water helps limit high temperatures in humid climates.
7. In winter, it is often warmer in San Francisco than in Sacramento, 150 km inland. In summer, it is nearly always hotter in Sacramento. Explain how the bodies of water surrounding San Francisco moderate its extreme temperatures.
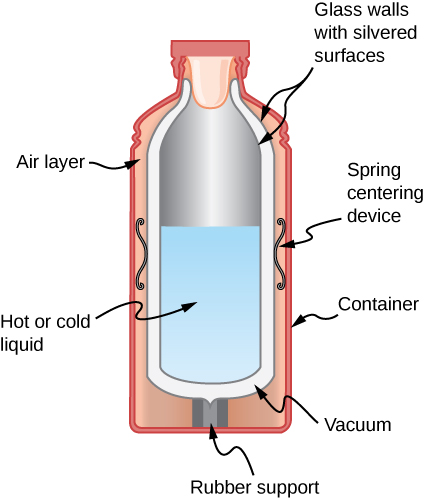
8. Shown below is a cut-away drawing of a thermos bottle (also known as a Dewar flask), which is a device designed specifically to slow down all forms of heat transfer. Explain the functions of the various parts, such as the vacuum, the silvering of the walls, the thin-walled long glass neck, the rubber support, the air layer, and the
9. 

Loose-fitting white clothing covering most of the body, shown below, is ideal for desert dwellers, both in the hot Sun and during cold evenings. Explain how such clothing is advantageous during both day and night.

10. Your house will be empty for a while in cold weather, and you want to save energy and money. Should you turn the thermostat down to the lowest level that will protect the house from damage such as freezing pipes, or leave it at the normal temperature? (If you don’t like coming back to a cold house, imagine that a timer controls the heating system so the house will be warm when you get back.) Explain your answer.
11. You pour coffee into an unlidded cup, intending to drink it 5 minutes later. You can add cream when you pour the cup or right before you drink it. (The cream is at the same temperature either way. Assume that the cream and coffee come into thermal equilibrium with each other very quickly.) Which way will give you hotter coffee? What feature of this question is different from the previous one?
12. On a cold winter morning, why does the metal of a bike feel colder than the wood of a porch?

Problems

13. How much heat transfer (in kilocalories) is required to thaw a 0.450-kg package of frozen vegetables originally at 0°C if their heat of fusion is the same as that of water?
14. A bag containing 0°C ice is much more effective in absorbing energy than one containing the same amount of 0°C water.

(a) How much heat transfer is necessary to raise the temperature of 0.800 kg of water from 0°C to 30.0°C?

(b) How much heat transfer is required to first melt 0.800 kg of 0°C ice and then raise its temperature?

(c) Explain how your answer supports the contention that the ice is more effective.

15.  (a) How much heat transfer is required to raise the temperature of a 0.750-kg aluminum pot containing 2.50 kg of water from 30.0°C to the boiling point and then boil away 0.750 kg of water?

(b) How long does this take if the rate of heat transfer is 500 W?

16. On a trip, you notice that a 3.50-kg bag of ice lasts an average of one day in your cooler. What is the average power in watts entering the ice if it starts at 0°C and completely melts to 0°C water in exactly one day?
17. On a certain dry sunny day, a swimming pool’s temperature would rise by 1.50°C if not for evaporation. What fraction of the water must evaporate to carry away precisely enough energy to keep the temperature constant?
18. (a) How much heat transfer is necessary to raise the temperature of a 0.200-kg piece of ice from −20.0°C to130.0°C, including the energy needed for phase changes?

(b) How much time is required for each stage, assuming a constant 20.0 kJ/s rate of heat transfer? (c) Make a graph of temperature versus time for this process.

19. In 1986, an enormous iceberg broke away from the Ross Ice Shelf in Antarctica. It was an approximately rectangular prism 160 km long, 40.0 km wide, and 250 m thick.

(a) What is the mass of this iceberg, given that the density of ice is \(917kg/m^3\)?

(b) How much heat transfer (in joules) is needed to melt it?

(c) How many years would it take sunlight alone to melt ice this thick, if the ice absorbs an average of \(100W/m^2\), 12.00 h per day?

20. How many grams of coffee must evaporate from 350 g of coffee in a 100-g glass cup to cool the coffee and the cup from 95.0°C to 45.0°C? Assume the coffee has the same thermal properties as water and that the average heat of vaporization is 2340 kJ/kg (560 kcal/g). Neglect heat losses through processes other than evaporation, as well as the change in mass of the coffee as it cools. Do the latter two assumptions cause your answer to be higher or lower than the true answer?
21. To help prevent frost damage, 4.00 kg of water at 0°Cis sprayed onto a fruit tree.

(a) How much heat transfer occurs as the water freezes?

(b) How much would the temperature of the 200-kg tree decrease if this amount of heat transferred from the tree? Take the specific heat to be 3.35kJ/kg⋅°C, and assume that no phase change occurs in the tree.

22. A 0.250-kg aluminum bowl holding 0.800kg of soup at 25.0°C is placed in a freezer. What is the final temperature if 388 kJ of energy is transferred from the bowl and soup, assuming the soup’s thermal properties are the same as that of water?
23. A 0.0500-kg ice cube at −30.0°C is placed in 0.400 kg of 35.0-°C water in a very well-insulated container. What is the final temperature?
24. If you pour 0.0100 kg of 20.0°C water onto a 1.20-kg block of ice (which is initially at −15.0°C), what is the final temperature? You may assume that the water cools so rapidly that effects of the surroundings are negligible.
25. (a) Calculate the rate of heat conduction through house walls that are 13.0 cm thick and have an average thermal conductivity twice that of glass wool. Assume there are no windows or doors. The walls’ surface area is \(120m^2\) and their inside surface is at 18.0°C, while their outside surface is at 5.00°C.(b) How many 1-kW room heaters would be needed to balance the heat transfer due to conduction?
26. The rate of heat conduction out of a window on a winter day is rapid enough to chill the air next to it. To see just how rapidly the windows transfer heat by conduction, calculate the rate of conduction in watts through a \(3.00-m^2\)window that is 0.634 cm thick (1/4 in.) if the temperatures of the inner and outer surfaces are 5.00°C and −10.0°C−, respectively. (This rapid rate will not be maintained—the inner surface will cool, even to the point of frost formation.)
27. Calculate the rate of heat conduction out of the human body, assuming that the core internal temperature is 37.0°C, the skin temperature is 34.0°C, the thickness of the fatty tissues between the core and the skin averages 1.00 cm, and the surface area is \(1.40m^2\).
28. Suppose you stand with one foot on ceramic flooring and one foot on a wool carpet, making contact over an area of \(80.0cm^2\) with each foot. Both the ceramic and the carpet are 2.00 cm thick and are 10.0°C on their bottom sides. At what rate must heat transfer occur from each foot to keep the top of the ceramic and carpet at 33.0°C?
29. A man consumes 3000 kcal of food in one day, converting most of it to thermal energy to maintain body temperature. If he loses half this energy by evaporating water (through breathing and sweating), how many kilograms of water evaporate?
30. A firewalker runs across a bed of hot coals without sustaining burns. Calculate the heat transferred by conduction into the sole of one foot of a firewalker given that the bottom of the foot is a 3.00-mm-thick callus with a conductivity at the low end of the range for wood and its density is \(300kg/m^3\). The area of contact is \(25.0cm^2\), the temperature of the coals is 700°C, and the time in contact is 1.00 s. Ignore the evaporative cooling of sweat.
31. Suppose a person is covered head to foot by wool clothing with average thickness of 2.00 cm and is transferring energy by conduction through the clothing at the rate of 50.0 W. What is the temperature difference across the clothing, given the surface area is \(1.40m^2\)?
32. A 0.800-kg iron cylinder at a temperature of \(1.00×10^3°C\) is dropped into an insulated chest of 1.00 kg of ice at its melting point. What is the final temperature, and how much ice has melted?
33. Repeat the preceding problem with 2.00 kg of ice instead of 1.00 kg.
34. Repeat the preceding problem with 0.500 kg of ice, assuming that the ice is initially in a copper container of mass 1.50 kg in equilibrium with the ice.
35. A 30.0-g ice cube at its melting point is dropped into an aluminum calorimeter of mass 100.0 g in equilibrium at 24.0°C with 300.0 g of an unknown liquid. The final temperature is 4.0°C. What is the heat capacity of the liquid?

Contributors and Attributions

Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).