4.2: Force and Mass
- Last updated
- Jan 14, 2019
- Save as PDF
- Page ID
- 16940

- Boundless
- Boundless
( \newcommand{\kernel}{\mathrm{null}\,}\)
Force
Force is any influence that causes an object to change, either concerning its movement, direction, or geometrical construction.
learning objectives
- Develop the relationship between mass and acceleration in determining force
Overview of Forces
In physics, a force is any influence that causes an object to undergo a certain change, either concerning its movement, direction, or geometrical construction. It is measured with the SI unit of Newtons. A force is that which can cause an object with mass to change its velocity, i.e., to accelerate, or which can cause a flexible object to deform. Force can also be described by intuitive concepts such as a push or pull. A force has both magnitude and direction, making it a vector quantity.
What is a force?: Describes what forces are and what they do.
Qualities of Force
The original form of Newton’s second law states that the net force acting upon an object is equal to the rate at which its momentum changes. This law is further given to mean that the acceleration of an object is directly proportional to the net force acting on the object, is in the direction of the net force, and is inversely proportional to the mass of the object.
As we mentioned, force is a vector quantity. A vector is a one dimensional array with elements of both magnitude and direction. In a force vector, the mass, m, is the magnitude component and the acceleration, a, is the directional component. The equation for force is written:
F=m⋅a
Related concepts to force include thrust, which increases the velocity of an object; drag, which decreases the velocity of an object; and torque which produces changes in rotational speed of an object. Forces which do not act uniformly on all parts of a body will also cause mechanical stresses, a technical term for influences which cause deformation of matter. While mechanical stress can remain embedded in a solid object, gradually deforming it, mechanical stress in a fluid determines changes in its pressure and volume.
Dynamics
Dynamics is the study of the forces that cause objects and systems to move. To understand this, we need a working definition of force. Our intuitive definition of force — that is, a push or a pull —is a good place to start. We know that a push or pull has both magnitude and direction (therefore, it is a vector quantity) and can vary considerably in each regard.
shows a few examples of the “push-pull” nature of force. The top left example is that of a pulley system. The force that someone would have to pull down on the cable would have to equal and exceed the force made by the mass the object and the effects of gravity on those object in order for the system to move up. The top right example shows that any object resting on a surface will still exert force on that surface. The bottom example is that of two magnets being attracted to each other due to magnetic force.
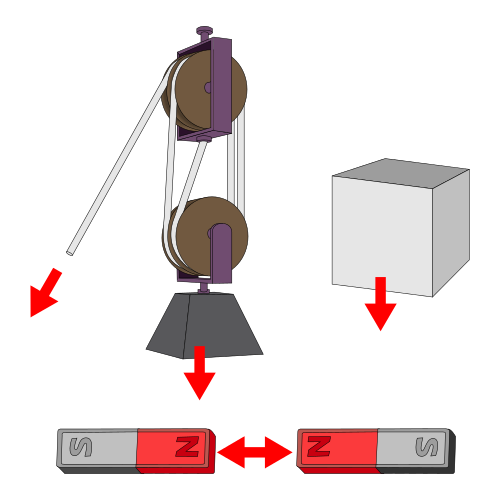
Examples of Force: Some situations in which forces are at play.
Mass
Mass is a physical property of matter that depends on size and shape of matter, and is expressed as kilograms by the SI system.
learning objectives
- Justify the significance of understanding mass in physics
What is Mass?
All elements have physical properties whose values can help describe an elements physical state. Changes to these properties can describe elemental transformations. Physical properties do not change the chemical nature of matter. The physical property we are covering in this atom is called mass.
Mass is defined as a quantitative measure of an object’s resistance to acceleration. The terms mass and weight are often interchanged, however it is incorrect to do so. Weight is a different property of matter that, while related to mass, is not mass, but rather the amount of gravitational force acting on a given body of matter. Mass is an intrinsic property that never changes.
Units of Mass
In order to measure something, a standard value must be established to use in relation to the object of measurement. This relation is called a unit. The International System of Units (SI) measures mass in kilograms, or kg. There are other units of mass, including the following (only the first two are accepted by the SI system):
- t – Tonne; 1t = 1000kg
- u – atomic mass unit; 1u ~= 1.66×10-27kg
- sl – slug
- lb – pound
Concepts Using Mass
- Weight – see
- Newtons Second Law – mass has a central role in determining the behavior of bodies. Newtons Second Law relates force f, exerted in a body of mass m, to the body’s acceleration a: F=ma
- Momentum – mass relates a body’s momentum, p, to its linear velocity, v: p=mv
- Kinetic Energy – mass relates kinetic energy, K to velocity, v: K=12m|v2|
Key Points
- Force is stated as a vector quantity, meaning it has elements of both magnitude and direction. Mass and acceleration respectively.
- In layman’s terms, force is a push or pull that can be defined in terms of various standards.
- Dynamics is the study of the force that causes objects and systems to move or deform.
- External forces are any outside forces that act on a body, and internal forces are any force acting within a body.
- Mass is defined as a quantitative measure of an object’s resistance to acceleration.
- According to Newton’s second law of motion, if a body of fixed mass m is subjected to a single force F, its acceleration a is given by F/m.
- Mass is central in many concepts of physics, including:weight, momentum, acceleration, and kinetic energy.
- According to Newton’s second law of motion, if a body of fixed mass m is subjected to a single force F, its acceleration a is given by F/m.
Key Terms
- force: A force is any influence that causes an object to undergo a certain change, either concerning its movement, direction or geometrical construction.
- velocity: A vector quantity that denotes the rate of change of position with respect to time, or a speed with a directional component.
- vector: A directed quantity, one with both magnitude and direction; the between two points.
- mass: The quantity of matter which a body contains, irrespective of its bulk or volume. It is one of four fundamental properties of matter. It is measured in kilograms in the SI system of measurement.
LICENSES AND ATTRIBUTIONS
CC LICENSED CONTENT, SHARED PREVIOUSLY
- Curation and Revision. Provided by: Boundless.com. License: CC BY-SA: Attribution-ShareAlike
CC LICENSED CONTENT, SPECIFIC ATTRIBUTION
- OpenStax College, College Physics. September 17, 2013. Provided by: OpenStax CNX. Located at: http://cnx.org/content/m42069/latest/?collection=col11406/latest. License: CC BY: Attribution
- OpenStax College, College Physics. September 17, 2013. Provided by: OpenStax CNX. Located at: http://cnx.org/content/m42069/latest/?collection=col11406/latest. License: CC BY: Attribution
- Force. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/Force. License: CC BY-SA: Attribution-ShareAlike
- vector. Provided by: Wiktionary. Located at: en.wiktionary.org/wiki/vector. License: CC BY-SA: Attribution-ShareAlike
- force. Provided by: Wiktionary. Located at: en.wiktionary.org/wiki/force. License: CC BY-SA: Attribution-ShareAlike
- velocity. Provided by: Wiktionary. Located at: en.wiktionary.org/wiki/velocity. License: CC BY-SA: Attribution-ShareAlike
- File:Force examples.svg - Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: en.Wikipedia.org/w/index.php?title=File:Force_examples.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- What is a force?. Located at: http://www.youtube.com/watch?v=HIZpG7h5b54. License: Public Domain: No Known Copyright. License Terms: Standard YouTube license
- Mass. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/Mass. License: CC BY-SA: Attribution-ShareAlike
- Special Relativity/Relativistic dynamics. Provided by: Wikibooks. Located at: en.wikibooks.org/wiki/Special_Relativity/Relativistic_dynamics%23Mass. License: CC BY-SA: Attribution-ShareAlike
- Physical properties. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/Physical_properties. License: CC BY-SA: Attribution-ShareAlike
- mass. Provided by: Wiktionary. Located at: en.wiktionary.org/wiki/mass. License: CC BY-SA: Attribution-ShareAlike
- File:Force examples.svg - Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: en.Wikipedia.org/w/index.php?title=File:Force_examples.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- What is a force?. Located at: http://www.youtube.com/watch?v=HIZpG7h5b54. License: Public Domain: No Known Copyright. License Terms: Standard YouTube license


