Micrometer
- Page ID
- 5176
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)A micrometer allows a measurement of the size of a body. It is one of the most accurate mechanical devices in common use. This document introduces this instrument.
If you are a student in the Physics laboratory at the University of Toronto, the material discussed here is also covered in the Commonly Used Instruments section of the First Year Laboratory Manual. There is also a videotape on using a Micrometer and a Vernier Caliper available from the laboratory technologists.
In order to effectively use this page, you need the Flash player. The Flash player is available free from http://www.macromedia.com/.
The above diagram shows a micrometer measuring the width of a pencil. The Thimble is rotated, causing it to move right or left. It is connected to the right-hand Measuring Rod, which also moves right or left. The measured size of the pencil is the distance between the two measuring rods when they are just in contact with the sides of the pencil.
Because of the mechanical advantage due to the fine pitch of the screw threads that move the thimble and the right-hand measuring rod, it is easy to use enough force in closing the rods on the object being measured to deform either the rods or the object. In the diagram you can see a Friction Screw, which applies "just enough" torque to rotate the thimble in a gentle manner.
Some micrometers implement the friction mechanism as a "barrel" attached to the thimble: it serves the same purpose.
You should always use the Friction Screw or its equivalent to close the rods on an object. Otherwise incorrect measurements will result!
There is also a Locking Lever which fixes the position of the thimble and right-hand measuring rod.
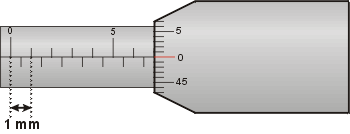
The diagram to the right is a close-up of the micrometer showing the thimble. The thimble rotates around a cylinder which has markings every one-half millimeter.
The left-hand side of the thimble has markings all around it. The line labelled 0 is the primary pointer. When it lines up with the central horizontal line on the cylinder, as shown, then the distance between the measuring rods is exactly an integral or half-integral number of millimeters.
In this case, then, the distance between the measuring rods is 7.000 mm. The upward line on the cylinder corresponding to 7 mm is barely visible under the thimble.
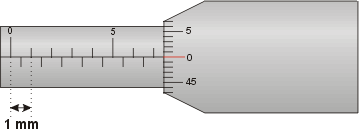
If we rotate the thimble one complete revolution so it moves to the right, it will look as shown.
Now the distance between the measuring rods is 7.500 mm. The downward line on the cylinder corresponding to 7.5 mm is barely visible.
Note that this is all consistent with the fact that the markings on the cylinder correspond to fifty divisions for a complete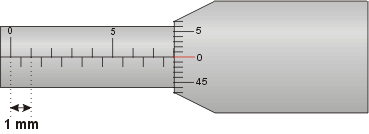 
revolution. Thus the numbers on the thimble correspond to hundredths of a millimeter.
One more complete revolution takes the distance to 8.000 mm, as shown.
Now imagine we turn the thimble just a little bit more, and end up with the situation shown.
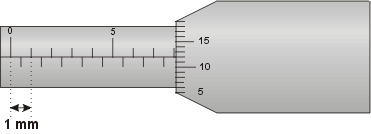We have increased the distance by 0.120 mm from 8.000, so the distance between the measuring rods is now 8.120 mm.
If we give the thimble one complete further rotation, we end up with the micrometer as shown to the right.
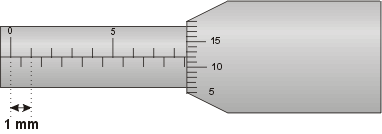Now the distance is 0.120 mm greater than 8.500 mm, so the distance is 8.620 mm.
Finally, if we turn the thimble a tiny bit further, it might end up like the figure to the right.
Now the distance is greater than 8.620 mm, but clearly less than 8.630 mm. We might estimate this reading to be 8.624 mm.
Depending on your eyes and screen resolution, you might be fairly confident that the reading is less than, say, 8.627 mm and similarly confident that it is greater than 8.621 mm. Thus you might assign a Reading Error to this measurement of 0.003 mm
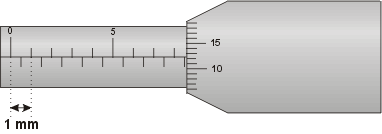So, we would report the distance as 8.624 ± 0.003 mm.
Depending on your eyes and the particular micrometer that you use, assigning a Reading Error of ± 0.003 mm is probably reasonable. This is 3 micro-meters!
There is an animation of reading the micrometer. You may access the animation by clicking on the red button to the right. It will appear in a separate window, and has a file size of 31k.
In the animation, as opposed to a real micrometer, we only do readings of the distance to the nearest hundredth of a millimeter.