6.4: The Compton Effect
( \newcommand{\kernel}{\mathrm{null}\,}\)
By the end of this section, you will be able to:
- Describe Compton’s experiment
- Explain the Compton wavelength shift
- Describe how experiments with X-rays confirm the particle nature of radiation
Two of Einstein’s influential ideas introduced in 1905 were the theory of special relativity and the concept of a light quantum, which we now call a photon. Beyond 1905, Einstein went further to suggest that freely propagating electromagnetic waves consisted of photons that are particles of light in the same sense that electrons or other massive particles are particles of matter. A beam of monochromatic light of wavelength λ (or equivalently, of frequency f) can be seen either as a classical wave or as a collection of photons that travel in a vacuum with one speed, c (the speed of light), and all carrying the same energy, Ef=hf. This idea proved useful for explaining the interactions of light with particles of matter.
Momentum of a Photon
Unlike a particle of matter that is characterized by its rest mass m0, a photon is massless. In a vacuum, unlike a particle of matter that may vary its speed but cannot reach the speed of light, a photon travels at only one speed, which is exactly the speed of light. From the point of view of Newtonian classical mechanics, these two characteristics imply that a photon should not exist at all. For example, how can we find the linear momentum or kinetic energy of a body whose mass is zero? This apparent paradox vanishes if we describe a photon as a relativistic particle. According to the theory of special relativity, any particle in nature obeys the relativistic energy equation
E2=p2c2+m20c4.
This relation can also be applied to a photon. In Equation ???, E is the total energy of a particle, p is its linear momentum, and m0 is its rest mass. For a photon, we simply set m0=0 in Equation ???, which leads to the expression for the momentum pf of a photon
pf=Efc.
Here the photon’s energy Ef is the same as that of a light quantum of frequency f, which we introduced to explain the photoelectric effect:
Ef=hf=hcλ.
The wave relation that connects frequency f with wavelength λ and speed c also holds for photons:
λf=c
Therefore, a photon can be equivalently characterized by either its energy and wavelength, or its frequency and momentum. Equations ??? and ??? can be combined into the explicit relation between a photon’s momentum and its wavelength:
pf=hλ.
Notice that this equation gives us only the magnitude of the photon’s momentum and contains no information about the direction in which the photon is moving. To include the direction, it is customary to write the photon’s momentum as a vector:
→pf=ℏ→l.
In Equation ???, ℏ=h/2π is the reduced Planck’s constant (pronounced “h-bar”), which is just Planck’s constant divided by the factor 2π. Vector →l is called the “wave vector” or propagation vector (the direction in which a photon is moving). The propagation vector shows the direction of the photon’s linear momentum vector. The magnitude of the wave vector is
k=|→k|=2π/λ
and is called the wave number. Notice that this equation does not introduce any new physics. We can verify that the magnitude of the vector in Equation ??? is the same as that given by Equation ???.
The Compton Effect
The Compton effect is the term used for an unusual result observed when X-rays are scattered on some materials. By classical theory, when an electromagnetic wave is scattered off atoms, the wavelength of the scattered radiation is expected to be the same as the wavelength of the incident radiation. Contrary to this prediction of classical physics, observations show that when X-rays are scattered off some materials, such as graphite, the scattered X-rays have different wavelengths from the wavelength of the incident X-rays. This classically unexplainable phenomenon was studied experimentally by Arthur H. Compton and his collaborators, and Compton gave its explanation in 1923.
To explain the shift in wavelengths measured in the experiment, Compton used Einstein’s idea of light as a particle. The Compton effect has a very important place in the history of physics because it shows that electromagnetic radiation cannot be explained as a purely wave phenomenon. The explanation of the Compton effect gave a convincing argument to the physics community that electromagnetic waves can indeed behave like a stream of photons, which placed the concept of a photon on firm ground.
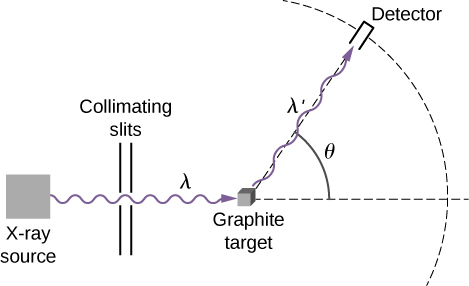
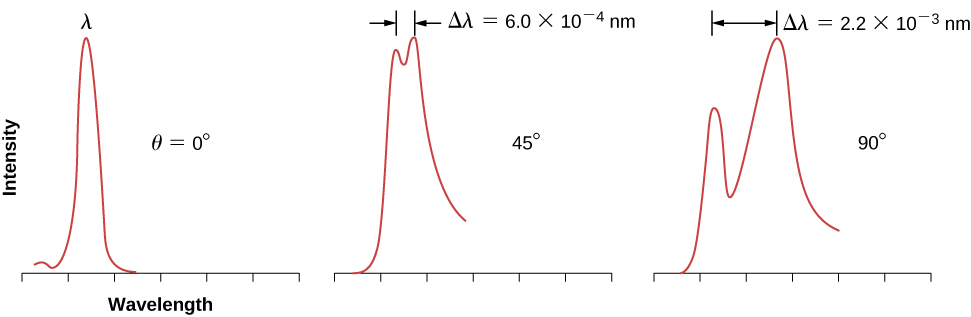
The schematics of Compton’s experimental setup are shown in Figure 6.4.1. The idea of the experiment is straightforward: Monochromatic X-rays with wavelength λ are incident on a sample of graphite (the “target”), where they interact with atoms inside the sample; they later emerge as scattered X-rays with wavelength λ′. A detector placed behind the target can measure the intensity of radiation scattered in any direction θ with respect to the direction of the incident X-ray beam. This scattering angle, θ, is the angle between the direction of the scattered beam and the direction of the incident beam. In this experiment, we know the intensity and the wavelength λ of the incoming (incident) beam; and for a given scattering angle θ, we measure the intensity and the wavelength λ′ of the outgoing (scattered) beam. Typical results of these measurements are shown in Figure 6.4.2, where the x-axis is the wavelength of the scattered X-rays and the y-axis is the intensity of the scattered X-rays, measured for different scattering angles (indicated on the graphs). For all scattering angles (except for θ=0°), we measure two intensity peaks. One peak is located at the wavelength λ, which is the wavelength of the incident beam. The other peak is located at some other wavelength, λ'. The two peaks are separated by Δλ, which depends on the scattering angle θ of the outgoing beam (in the direction of observation). The separation Δλ is called the Compton shift.
Compton Shift
As given by Compton, the explanation of the Compton shift is that in the target material, graphite, valence electrons are loosely bound in the atoms and behave like free electrons. Compton assumed that the incident X-ray radiation is a stream of photons. An incoming photon in this stream collides with a valence electron in the graphite target. In the course of this collision, the incoming photon transfers some part of its energy and momentum to the target electron and leaves the scene as a scattered photon. This model explains in qualitative terms why the scattered radiation has a longer wavelength than the incident radiation. Put simply, a photon that has lost some of its energy emerges as a photon with a lower frequency, or equivalently, with a longer wavelength. To show that his model was correct, Compton used it to derive the expression for the Compton shift. In his derivation, he assumed that both photon and electron are relativistic particles and that the collision obeys two commonsense principles:
- the conservation of linear momentum and
- the conservation of total relativistic energy.
In the following derivation of the Compton shift, E_f and \vec{p}_f denote the energy and momentum, respectively, of an incident photon with frequency f. The photon collides with a relativistic electron at rest, which means that immediately before the collision, the electron’s energy is entirely its rest mass energy, m_0c^2. Immediately after the collision, the electron has energy E and momentum \vec{p}, both of which satisfy Equation \ref{6.19}. Immediately after the collision, the outgoing photon has energy \vec{\tilde{E}}_f, momentum \vec{\tilde{p}}_f, and frequency f'. The direction of the incident photon is horizontal from left to right, and the direction of the outgoing photon is at the angle θ, as illustrated in Figure \PageIndex{1}. The scattering angle θ is the angle between the momentum vectors \vec{p}_f and \vec{\tilde{p}}_f, and we can write their scalar product:
\vec{p} \cdot \vec{\tilde{p}}_f = p_f\vec{p}_f \cos \, \theta. \label{6.23}
Following Compton’s argument, we assume that the colliding photon and electron form an isolated system. This assumption is valid for weakly bound electrons that, to a good approximation, can be treated as free particles. Our first equation is the conservation of energy for the photon-electron system:
E_f + m_0c^2 = \tilde{E}_f + E. \label{6.24}
The left side of this equation is the energy of the system at the instant immediately before the collision, and the right side of the equation is the energy of the system at the instant immediately after the collision. Our second equation is the conservation of linear momentum for the photon–electron system where the electron is at rest at the instant immediately before the collision:
\vec{p}_f = \vec{\tilde{p}}_f + \vec{p}. \label{6.25}
The left side of this equation is the momentum of the system right before the collision, and the right side of the equation is the momentum of the system right after collision. The entire physics of Compton scattering is contained in these three preceding equations––the remaining part is algebra. At this point, we could jump to the concluding formula for the Compton shift, but it is beneficial to highlight the main algebraic steps that lead to Compton’s formula, which we give here as follows.
We start with rearranging the terms in Equation \ref{6.24} and squaring it:
[(E_f - \tilde{E}_f) + m_0c^2]^2 = E^2. \nonumber
In the next step, we substitute Equation \ref{6.19} for E^2, simplify, and divide both sides by c^2 to obtain
(E_f / c - \tilde{E}_f / c)^2 + 2m_0c (E_f / c - \tilde{E}_f / c) = p^2. \nonumber
Now we can use Equation \ref{6.21} to express this form of the energy equation in terms of momenta. The result is
(p_f - \tilde{p}_f)^2 + 2m_0 c(p_f - \tilde{p}_f) = p^2. \label{6.26}
To eliminate p^2, we turn to the momentum equation Equation \ref{6.25}, rearrange its terms, and square it to obtain
\begin{align*} (\vec{p}_f - \vec{\tilde{p}}_f)^2 &= p^2 \\[4pt] &= p_f^2 + \tilde{p}_f^2 - 2p_f \tilde{p}_f \, \cos \, \theta. \end{align*} \nonumber
The product of the momentum vectors is given by Equation \ref{6.23}. When we substitute this result for p^2 in Equation \ref{6.26}, we obtain the energy equation that contains the scattering angle θ:
(p_f - \tilde{p}_f)^2 + 2m_0c(p_f - \tilde{p}_f) = p_f^2 + \tilde{p}_f^2 - 2p_f \tilde{p}_f \, \cos \, \theta. \nonumber
With further algebra, this result can be simplified to
\dfrac{1}{\tilde{p}_f} - \dfrac{1}{p_f} = \dfrac{1}{m_0c}(1 - \cos \, \theta). \label{6.27}
Now recall Equation \ref{6.21} and write: 1/\tilde{p}_f = \lambda' /h and 1/p_f = \lambda /h. When these relations are substituted into Equation \ref{6.27}, we obtain the relation for the Compton shift:
\lambda' - \lambda = \dfrac{h}{m_0c}(1 - \cos \, \theta). \label{6.28}
The factor h/m_0c is called the Compton wavelength of the electron:
\lambda_c = \dfrac{h}{m_0c} = 0.00243 \, nm = 2.43 \, pm. \label{6.29}
Denoting the shift as \Delta \lambda = \lambda' - \lambda, the concluding result can be rewritten as
\Delta \lambda = \lambda_c (1 - \cos \, \theta). \label{6.30}
This formula for the Compton shift describes outstandingly well the experimental results shown in Figure \PageIndex{2}. Scattering data measured for molybdenum, graphite, calcite, and many other target materials are in accord with this theoretical result. The nonshifted peak shown in Figure \PageIndex{1} is due to photon collisions with tightly bound inner electrons in the target material. Photons that collide with the inner electrons of the target atoms in fact collide with the entire atom. In this extreme case, the rest mass in Equation \ref{6.29} must be changed to the rest mass of the atom. This type of shift is four orders of magnitude smaller than the shift caused by collisions with electrons and is so small that it can be neglected.
Compton scattering is an example of inelastic scattering, in which the scattered radiation has a longer wavelength than the wavelength of the incident radiation. In today’s usage, the term “Compton scattering” is used for the inelastic scattering of photons by free, charged particles. In Compton scattering, treating photons as particles with momenta that can be transferred to charged particles provides the theoretical background to explain the wavelength shifts measured in experiments; this is the evidence that radiation consists of photons.
An incident 71-pm X-ray is incident on a calcite target. Find the wavelength of the X-ray scattered at a 30°30° angle. What is the largest shift that can be expected in this experiment?
Strategy
To find the wavelength of the scattered X-ray, first we must find the Compton shift for the given scattering angle, \theta = 30°. We use Equation \ref{6.30}. Then we add this shift to the incident wavelength to obtain the scattered wavelength. The largest Compton shift occurs at the angle \theta when 1 - \cos \, \theta has the largest value, which is for the angle \theta = 180°.
Solution
The shift at \theta = 30° is
\begin{align*} \Delta \lambda &= \lambda_c (1 - \cos \, 30°) \\[4pt] &= 0.134 \lambda_c \\[4pt] &= (0.134)(2.43) \, pm \\[4pt] &= 0.32 \end{align*} \nonumber
This gives the scattered wavelength:
\begin{align*} \lambda' &= \lambda + \Delta \lambda \\[4pt] &= (71 + 0.325) \, pm \\[4pt] &= 71.325 \,pm. \end{align*} \nonumber
The largest shift is
\begin{align*} (\Delta \lambda )_{max} &= \lambda_c(1 − \cos \, 180°) \\[4pt] &= 2(2.43 \, pm) \\[4pt] &= 4.86 \, pm. \end{align*} \nonumber
Significance
The largest shift in wavelength is detected for the backscattered radiation; however, most of the photons from the incident beam pass through the target and only a small fraction of photons gets backscattered (typically, less than 5%). Therefore, these measurements require highly sensitive detectors.
An incident 71-pm X-ray is incident on a calcite target. Find the wavelength of the X-ray scattered at a 60° angle. What is the smallest shift that can be expected in this experiment?
- Answer
-
(\Delta \lambda)_{min} = 0 \, m at a 0° angle; 71.0 \, pm + 0.5 \lambda_c = 72.215 \, pm


