8.5.7: Transformers
- Page ID
- 101262
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Power loss in long transmission lines is related to the magnitude of the current. Specifically, the power loss can be decreased by decreasing the magnitude of the current.
The amount of power passed through transmission lines can be calculated by multiplying voltage by current. The same power can be transmitted using a very high voltage and a very low current as with a low voltage and high current.
Since power companies do not wish to waste power as it is transmitted to homes and businesses, they deliberately 'step up' the voltage and reduce the current before transmitting the power over extended distances. That type of power transmits well without great loss of energy but it cannot be used in household appliances. It becomes necessary to convert it back ('step down') to low voltage and high current for household use. That is the job of electrical transformers – those big gray barrels you see on power poles.
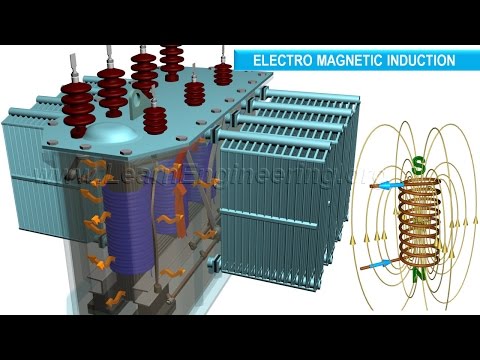
Transformers
When we move a wire through a magnetic field, a force is exerted on the charges in the wire and a current is induced. Essentially the same thing happens if we hold the wire steady and move the magnetic field by moving the magnet. Yet a third way of causing relative motion between the charges in a wire and a magnetic field is to expand or contract the field through the wire.
When a current begins to flow in a wire, a circular magnetic field forms around the wire. Within the first fractional second when the current begins to flow, the magnetic field expands outward from the wire. If a second wire is placed nearby, the expanding field will pass through the second wire and induce a brief current in the wire.
Consider the sketch above. When the knife switch is closed, current begins to flow in the first circuit and therefore, a magnetic field expands outward around the wire. When the magnetic field expands outward from the wire on the right side, it will pass through the wire in the second circuit. This relative motion between wire and field induces a current in the second circuit. The magnetic field expands outward for only a very short period of time and therefore, only a short jolt of current is induced in the second circuit. You can leave the knife switch closed and the current will continue to flow in the first circuit but no current is induced in the second circuit because the field is constant and therefore there is no relative motion between the field and the wire in the second circuit. When the knife switch is opened, the current in the first circuit ceases to flow and the magnetic field collapses back through the wire to zero. As the magnetic field collapses, it passes through the wire and once again we have relative motion between the wire in the second circuit and the magnetic field. Therefore, we once again have a short jolt of current induced in the second circuit. This second jolt of induced current will be flowing in the opposite direction of the first induced current. We can produce an alternating current in the second circuit simply by closing and opening the knife switch continuously in the first circuit.
Obviously, a transformer would have little use in the case of DC current because current is only induced in the second circuitwhen the first circuit is started or stopped. With AC current, however, since the current changes direction 60 times per second, the magnetic field would constantly be expanding and contracting through the second wire.
A transformer is a device used to increase or decrease alternating current voltages. They do this with essentially no loss of energy. A transformer has two coils, electrically insulated from each other as shown in the sketch. One coil is called the primary coil and the other is called the secondary coil. When the primary coil is connected to a source of AC voltage, the changing current creates a varying magnetic field. The varying magnetic field induces a varying EMF in the secondary coil. The EMF induced in the secondary coil is called the secondary voltage and is proportional to the primary voltage. The secondary voltage also depends on the ratio of turns on the secondary coil to turns on the primary coil.
(secondary voltage/primary voltage)=(number of turns on secondary/number of turns on primary)
VS/VP=NS/NP
If the secondary voltage is larger than the primary voltage, the transformer is called a step-up transformer. If the voltage out of the transformer is smaller than the voltage in, then the transformer is called a step-down transformer.
In an ideal transformer, the electric power put into the primary equals the electric power delivered by the secondary.
VP/IP=VS/IS
The current that flows in the primary depends on how much current is required by the secondary circuit.
IS/IP=VP/VS=NP/NS
A particular step-up transformer has 200 turns on the primary coil and 3000 turns on the secondary coil.
- If the voltage on the primary coil is 90.0 V, what is the voltage on the secondary coil?
- If the current in the secondary circuit is 2.00 A, what is the current in the primary coil?
- What is the power in the primary circuit?
- What is the power in the secondary circuit?
Solution
- VP/VS=NP/NS 90.0 V/VS=200/3000 VS=(90.0 V)(3000)/(200)=1350 V
- IS/IP=NP/NS 2.00 A/IP=200/3000 IP=30.0 A
- PP=VP/IP=(90.0 V)/(30.0 A)=2700 W
- PS=VS/IS=(1350 V)/(2.00 A)=2700 W
Launch the AC Transformer simulation below to visualize how we use a transformer to “step down” the high voltage on a residential power line:
Summary
- When a current begins to flow in a wire, a circular magnetic field forms around the wire.
- Within the first fractional second when the current begins to flow, the magnetic field expands outward from the wire.
- If a second wire is placed nearby, the expanding field will pass through the second wire and induce a brief current in the wire.
- A transformer is a device used to increase or decrease alternating current voltages.
- A transformer has two coils, electrically insulated from each other. One coil is called the primary coil and the other is called the secondary coil.
- The varying magnetic field induces a varying EMF in the secondary coil.
- The EMF induced in the secondary coil is called the secondary voltage and is proportional to the primary voltage. The secondary voltage also depends on the ratio of turns on the secondary coil to turns on the primary coil.
(secondary voltage/primary voltage)=(number of turns on secondary/number of turns on primary)
Review
- A step-down transformer has 7500 turns on its primary and 125 turns on its secondary. The voltage across the primary is 7200 V.
- What is the voltage across the secondary?
- The current in the secondary is 36 A. What current flows in the primary?
- The secondary of a step-down transformer has 500 turns. The primary has 15,000 turns.
- The EMF of the primary is 3600 V. What is the EMF of the secondary?
- The current in the primary is 3.0 A. What is the current in the secondary?
- An ideal step-up transformer’s primary coil has 500 turns and its secondary coil has 15,000 turns. The primary EMF is 120 V.
- Calculate the EMF of the secondary.
- If the secondary current is 3.0 A, what is the primary current?
- What power is drawn by the primary?
Explore More
Use this resource to answer the questions that follow.
- What type of transformer is used at the power station where the electric power is generated?
- What type of transformer is used at power sub-stations?
- What type of transformer is used inside cell phone chargers?
Additional Resources
Study Guide: Magnetism Study Guide
Real World Application: Transforming Your Life
Videos: