13.7: The RLC Series Acceptor Circuit

A resistance, inductance and a capacitance in series is called an "acceptor" circuit, presumably because, for some combination of the parameters, the magnitude of the inductance is a minimum, and so current is accepted most readily. We see in Figure $\text{XIII.5}$ an alternating voltage $V=\hat{V}e^{j\omega t}$ applied across such an $R$, $L$ and $C$.

FIGURE $\text{XIII.5}$

The impedance is

$$\label{13.7.1}Z=R+j\left (L\omega -\dfrac{1}{C\omega}\right ).$$

We can see that the voltage leads on the current if the reactance is positive; that is, if the inductive reactance is greater than the capacitive reactance; that is, if $\omega > 1/\sqrt{LC}$. (Recall that the frequency, $\nu$, is $\omega/(2\pi)$). If $\omega < 1/\sqrt{LC}$, the voltage lags behind the current. And if $\omega = 1/\sqrt{LC}$, the circuit is purely resistive, and voltage and current are in phase.

The magnitude of the impedance (which is equal to $\hat{V}/\hat{I}$) is

$$\label{13.7.2}|Z|=\sqrt{R^2+\left (L\omega - 1/(C\omega )\right )^2},$$

and this is least (and hence the current is greatest) when $\omega = 1/\sqrt{LC}$, the resonant frequency, which I shall denote by $\omega_0$.

It is of interest to draw a graph of how the magnitude of the impedance varies with frequency for various values of the circuit parameters. I can reduce the number of parameters by defining the dimensionless quantities

$$\label{13.7.3}\Omega = \omega / \omega_0$$

$$\label{13.7.4}Q=\dfrac{1}{R}\sqrt{\dfrac{L}{C}}$$

and

$$\label{13.7.5}z=\dfrac{|Z|}{R}.$$

You should verify that $Q$ is indeed dimensionless. We shall see that the sharpness of the resonance depends on $Q$, which is known as the quality factor (hence the symbol $Q$). In terms of the dimensionless parameters, Equation $\ref{13.7.2}$ becomes

$$\label{13.7.6}z=\sqrt{1+Q^2(\Omega -1/\Omega )^2}.$$

This is shown in Figure $\text{XIII.6}$, in which it can be seen that the higher the quality factor, the sharper the resonance.

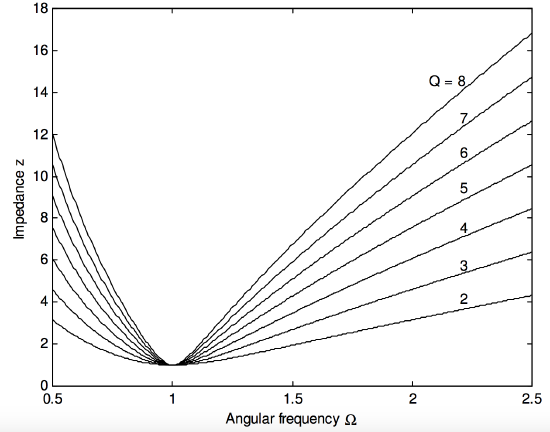
FIGURE $\text{XIII.6}$

In particular, it is easy to show that the frequencies at which the impedance is twice its minimum value are given by the positive solutions of

$$\label{13.7.7}\Omega^4 -\left (2+\dfrac{3}{Q^2}\right )\Omega^2+1=0.$$

If I denote the smaller and larger of these solutions by $\Omega_- \text{ and }\Omega_+$, then $\Omega_+ -\Omega_-$ will serve as a useful description of the width of the resonance, and this is shown as a function of quality factor in Figure $\text{XIII.7}$.

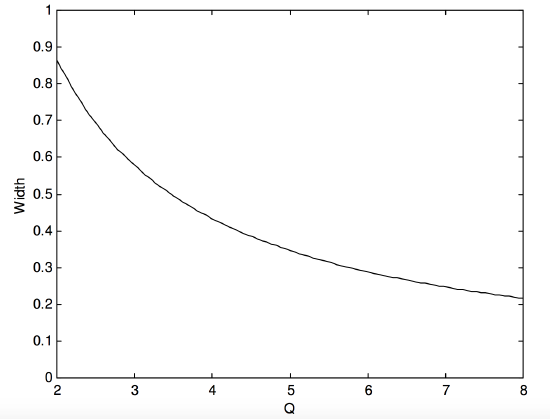
FIGURE $\text{XIII.7}$