2.3: 1.3 Galilean Relativity

I defined inertial mass conceptually as a measure of how hard it is to change an object's state of motion, the implication being that if you don't interfere, the object's motion won't change. Most people, however, believe that objects in motion have a natural tendency to slow down. Suppose I push my refrigerator to the west for a while at 0.1 m/s, and then stop pushing. The average person would say fridge just naturally stopped moving, but let's imagine how someone in China would describe the fridge experiment carried out in my house here in California. Due to the rotation of the earth, California is moving to the east at about 400 m/s. A point in China at the same latitude has the same speed, but since China is on the other side of the planet, China's east is my west. (If you're finding the three-dimensional visualization difficult, just think of China and California as two freight trains that go past each other, each traveling at 400 m/s.) If I insist on thinking of my dirt as being stationary, then China and its dirt are moving at 800 m/s to my west. From China's point of view, however, it's California that is moving 800 m/s in the opposite direction (my east). When I'm pushing the fridge to the west at 0.1 m/s, the observer in China describes its speed as 799.9 m/s. Once I stop pushing, the fridge speeds back up to 800 m/s. From my point of view, the fridge “naturally” slowed down when I stopped pushing, but according to the observer in China, it “naturally” sped up!

What's really happening here is that there's a tendency, due to friction, for the fridge to stop moving relative to the floor. In general, only relative motion has physical significance in physics, not absolute motion. It's not even possible to define absolute motion, since there is no special reference point in the universe that everyone can agree is at rest. Of course if we want to measure motion, we do have to pick some arbitrary reference point which we will say is standing still, and we can then define \(x\), \(y\), and \(z\) coordinates extending out from that point, which we can define as having \(x=0\), \(y=0\), \(z=0\). Setting up such a system is known as choosing a frame of reference. The local dirt is a natural frame of reference for describing a game of basketball, but if the game was taking place on the deck of a moving ocean liner, we would probably pick a frame of reference in which the deck was at rest, and the land was moving.

a / Galileo Galilei (1564-1642).

Galileo was the first scientist to reason along these lines, and we now use the term Galilean relativity to refer to a somewhat modernized version of his principle. Roughly speaking, the principle of Galilean relativity states that the same laws of physics apply in any frame of reference that is moving in a straight line at constant speed. We need to refine this statement, however, since it is not necessarily obvious which frames of reference are going in a straight line at constant speed. A person in a pickup truck pulling away from a stoplight could admit that the car's velocity is changing, or she could insist that the truck is at rest, and the meter on the dashboard is going up because the asphalt picked that moment to start moving faster and faster backward! Frames of reference are not all created equal, however, and the accelerating truck's frame of reference is not as good as the asphalt's. We can tell, because a bowling ball in the back of the truck, as in figure c, appears to behave strangely in the driver's frame of reference: in her rear-view mirror, she sees the ball, initially at rest, start moving faster and faster toward the back of the truck. This goofy behavior is evidence that there is something wrong with her frame of reference. A person on the sidewalk, however, sees the ball as standing still. In the sidewalk's frame of reference, the truck pulls away from the ball, and this makes sense, because the truck is burning gas and using up energy to change its state of motion.

We therefore define an inertial frame of reference as one in which we never see objects change their state of motion without any apparent reason. The sidewalk is a pretty good inertial frame, and a car moving relative to the sidewalk at constant speed in a straight line defines a pretty good inertial frame, but a car that is accelerating or turning is not a inertial frame.

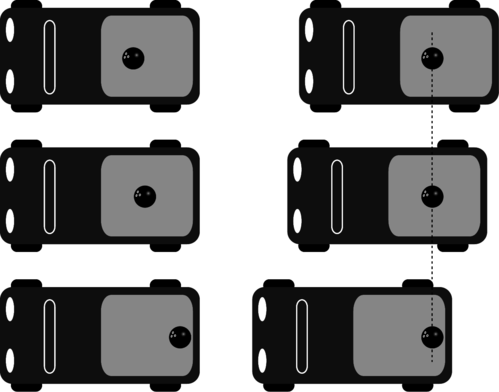
c / Left: In a frame of reference that speeds up with the truck, the bowling ball appears to change its state of motion for no reason. Right: In an inertial frame of reference, which the surface of the earth approximately is, the bowling ball stands still, which makes sense because there is nothing that would cause it to change its state of motion.

The principle of Galilean relativity states that inertial frames exist, and that the same laws of physics apply in all inertial frames of reference, regardless of one frame's straight-line, constant-speed motion relative to another.⁴

Another way of putting it is that all inertial frames are created equal. We can say whether one inertial frame is in motion or at rest relative to another, but there is no privileged “rest frame.” There is no experiment that comes out any different in laboratories in different inertial frames, so there is no experiment that could tell us which inertial frame is really, truly at rest.

b / The earth spins. People in Shanghai say they're at rest and people in Los Angeles are moving. Angelenos say the same about the Shanghainese.

Example 3: The speed of sound

\(\triangleright\) The speed of sound in air is only 340 m/s, so unless you live at a near-polar latitude, you're moving at greater than the speed of sound right now due to the Earth's rotation. In that case, why don't we experience exciting phenomena like sonic booms all the time? \(\triangleright\) It might seem as though you're unprepared to deal with this question right now, since the only law of physics you know is conservation of mass, and conservation of mass doesn't tell you anything obviously useful about the speed of sound or sonic booms. Galilean relativity, however, is a blanket statement about all the laws of physics, so in a situation like this, it may let you predict the results of the laws of physics without actually knowing what all the laws are! If the laws of physics predict a certain value for the speed of sound, then they had better predict the speed of the sound relative to the air, not their speed relative to some special “rest frame.” Since the air is moving along with the rotation of the earth, we don't detect any special phenomena. To get a sonic boom, the source of the sound would have to be moving relative to the air.

Example 4: The Foucault pendulum

d / Foucault demonstrates his pendulum to an audience at a lecture in 1851. Note that in the example of the bowling ball in the truck, I didn't claim the sidewalk was exactly a Galilean frame of reference. This is because the sidewalk is moving in a circle due to the rotation of the Earth, and is therefore changing the direction of its motion continuously on a 24-hour cycle. However, the curve of the motion is so gentle that under ordinary conditions we don't notice that the local dirt's frame of reference isn't quite inertial. The first demonstration of the noninertial nature of the earth-fixed frame of reference was by Foucault using a very massive pendulum (figure d) whose oscillations would persist for many hours without becoming imperceptible. Although Foucault did his demonstration in Paris, it's easier to imagine what would happen at the north pole: the pendulum would keep swinging in the same plane, but the earth would spin underneath it once every 24 hours. To someone standing in the snow, it would appear that the pendulum's plane of motion was twisting. The effect at latitudes less than 90 degrees turns out to be slower, but otherwise similar. The Foucault pendulum was the first definitive experimental proof that the earth really did spin on its axis, although scientists had been convinced of its rotation for a century based on more indirect evidence about the structure of the solar system.

e / Galileo's trial.

Although popular belief has Galileo being prosecuted by the Catholic Church for saying the earth rotated on its axis and also orbited the sun, Foucault's pendulum was still centuries in the future, so Galileo had no hard proof; Galileo's insights into relative versus absolute motion simply made it more plausible that the world could be spinning without producing dramatic effects, but didn't disprove the contrary hypothesis that the sun, moon, and stars went around the earth every 24 hours. Furthermore, the Church was much more liberal and enlightened than most people believe. It didn't (and still doesn't) require a literal interpretation of the Bible, and one of the Church officials involved in the Galileo affair wrote that “the Bible tells us how to go to heaven, not how the heavens go.” In other words, religion and science should be separate. The actual reason Galileo got in trouble is shrouded in mystery, since Italy in the age of the Medicis was a secretive place where unscrupulous people might settle a score with poison or a false accusation of heresy. What is certain is that Galileo's satirical style of scientific writing made many enemies among the powerful Jesuit scholars who were his intellectual opponents --- he compared one to a snake that doesn't know its own back is broken. It's also possible that the Church was far less upset by his astronomical work than by his support for atomism (discussed further in the next section). Some theologians perceived atomism as contradicting transubstantiation, the Church's doctrine that the holy bread and wine were literally transformed into the flesh and blood of Christ by the priest's blessing.

self-check:

What is incorrect about the following supposed counterexamples to the principle of inertia?

(1) When astronauts blast off in a rocket, their huge velocity does cause a physical effect on their bodies --- they get pressed back into their seats, the flesh on their faces gets distorted, and they have a hard time lifting their arms.

(2) When you're driving in a convertible with the top down, the wind in your face is an observable physical effect of your absolute motion.

(answer in the back of the PDF version of the book)

◊ Solved problem: a bug on a wheel — problem 12

Contributors and Attributions

Benjamin Crowell (Fullerton College). Conceptual Physics is copyrighted with a CC-BY-SA license.