10.13: Discharge of a Capacitor through an Inductance
( \newcommand{\kernel}{\mathrm{null}\,}\)
The circuit is shown in Figure X.11, and, once again, it is important to take care with the signs.
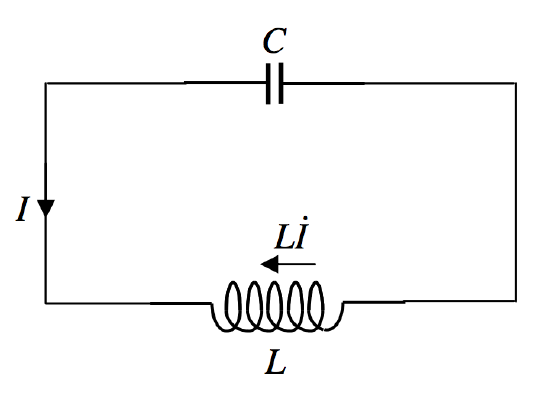
FIGURE X.11
If +Q is the charge on the left hand plate of the capacitor at some time (and −Q the charge on the right hand plate) the current I in the direction indicated is −˙Q and the potential difference across the plates is Q/C. The back EMF is in the direction shown, and we have
QC−L˙I=0,
or
QC+L¨Q=0.
This can be written
¨Q=−QLC,
which is simple harmonic motion of period 2π√LC. (verify that this has dimensions of time.) Thus energy sloshes to and fro between storage as charge in the capacitor and storage as current in the inductor.
If there is resistance in the circuit, the oscillatory motion will be damped, the charge and current eventually approaching zero. But, even if there is no resistance, the oscillation does not continue for ever. While the details are beyond the scope of this chapter, being more readily dealt with in a discussion of electromagnetic radiation, the periodic changes in the charge in the capacitor and the current in the inductor, result in an oscillating electromagnetic field around the circuit, and in the generation of an electromagnetic wave, which carries energy away at a speed of √1/(μ0ϵ0). Verify that this has the dimensions of speed, and that it has the value 2.998×108 m s−1. The motion in the circuit is damped just as if there were a resistance of √μ0/ϵ0=cμ0=1/(cϵ0) in the circuit. Verify that this has the dimensions of resistance and that it has a value of 376.7Ω. This effective resistance is called the impedance of free space.


