4.3: Fission
( \newcommand{\kernel}{\mathrm{null}\,}\)
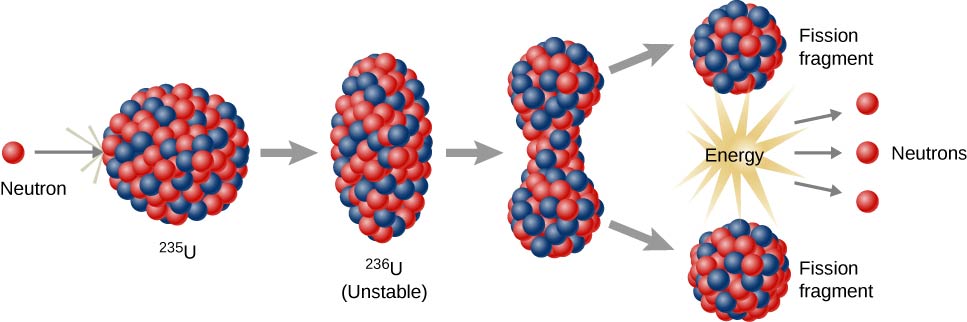
Once we have started to look at the liquid drop model, we can try to ask the question what it predicts for fission, where one can use the liquid drop model to good effect. We are studying how a nuclear fluid drop separates into two smaller ones, either about the same size, or very different in size. This process is indicated in Figure 4.3.1.

The liquid drop elongates, by performing either a quadrupole or octupole type vibration, but it persists until the nucleus falls apart into two pieces. Since the equilibrium shape must be stable against small fluctuations, we find that the energy must go up near the spherical form, as sketched in Figure 4.3.2.

In that figure we sketch the energy - which is really the potential energy - for separation into two fragments, R is the fragment distance. As with any of such processes we can either consider classical fission decays for energy above the fission barrier, or quantum mechanical tunnelling for energies below the barrier. The method used in fission bombs is to use the former, by hitting a 235U nucleus with a slow neutron a state with energy above the barrier is formed, which fissions fast. The fission products are unstable, and emit additional neutrons, which can give rise to a chain reaction.
The mass formula can be used to give an indication what is going on; Let us look at at the symmetric fusion of a nucleus. In that case the Q value is
Q=M(A,Z)−2M(A/2,Z/2)
The mass formula fails in predicting the asymmetry of fission, the splitting process is much more likely to go into two unequal fragments
Exercise 4.3.1
Evaluate the Q value for the fission U236 with 92 protons.


