1.8: Differential Form of Snell's Law
( \newcommand{\kernel}{\mathrm{null}\,}\)
Snell’s law in the form nsinθ = constant is useful in calculating how a light ray is bent in travelling from one medium to another where there is a discrete change of refractive index. If there is a medium in which the refractive index is changing continuously, a differential form of Snell’s law may be useful. This is obtained simply by differentiation of nsinθ = constant, to obtain the differential form of Snell’s law
cotθdθ=−dnn.
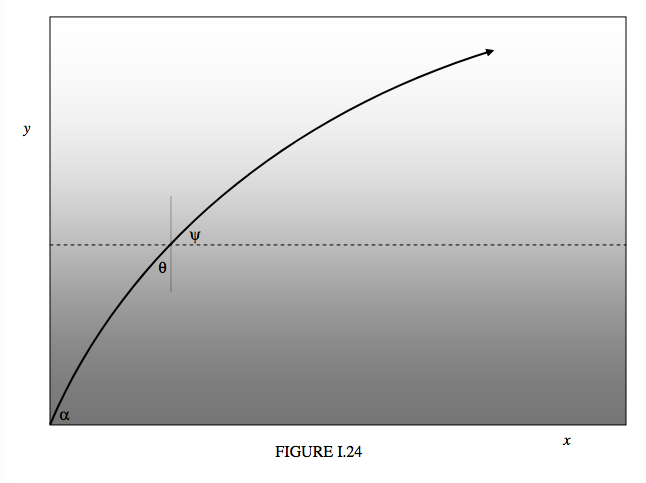
If we express this in terms of the complementary angle Ψ (see Figure I.24), this equation takes the form
tanΨdΨ=dnn.
Let us see how this might be used. Let us suppose, for example, that we have some medium in which the refractive index diminishes linearly with the y coordinate according to
n=n0−(n0−1)ya.
This form has n=n0 at y=0, decreasing linearly to n=1 at y=a. We suppose that n=1 everywhere beyond y=a. The equation is simplified if we write μ for n−1 and for μ0 for n0−1, so that
μ=μ0(1−ya),
which has μ decreasing linearly from μ0 to zero. Let us suppose that we direct a light ray upwards from the origin in a direction making an angle α with the horizontal, and we wish to trace the ray through the medium as the refractive index continuously changes. See Figure I.24. With the refractive index changing as in Equation ???, Snell’s law takes the form
tanΨdΨ=−dyk−y,wherek=n0aμ0.
On integration, this becomes
y=k(1−cosαcosΨ)orcosΨ=kcosαk−y.
This is the (y,Ψ) equation to the path taken by the light. One can see from this equation that the path becomes horizontal when y=k(1−cosα. To find the (x,y) equation, we use the identities cosΨ=(1+tan2Ψ)−1/2 and tanΨ=dydx. Substitution of these into Equation ??? gives the differential equation to the path in (x,y) coordinates:
(dydx)2=(k−y)2−l2l2,wherel=kcosα.
This can be readily integrated to give the (x,y) equation to the path:
x=lln[k(1+sinα)k−y±√(k−y)2−l2].
In Figure I.25 I have taken a=1 (in other words, I am expressing all distances in units of a), and I am taking n0=1.5 (hence k=3), and I calculate the paths for α = 15°, 30°, 45°, 60°, 75°.

Figure I.25 and Equation ??? show the paths of the light if the refractive index is varying from n0 at y=0, to 1 at y=a as described by Equation ???. But now suppose that the refractive index is varying with height according to
n=n0a(n0−1)y+a.
In this model, too, the refractive index goes from n0 at y=0, to 1 at y=a, but the variation is not linear. In fact you might like to convince yourself that it is the speed of light that is increasing linearly from y=0 to y=a. See if you can trace the paths of the light rays in this situation. I think they are arcs of circles, and you might be able to calculate the radii and the coordinates of the centres of the circles. Here’s another one:
n=√n20a(n20−1)y+a.
Here, too, the refractive index goes from n0 at y=0, to 1 at y=a. You might like to try tracing the rays in this model. I think they may be arcs of cycloids. Of course these examples may seem to be very unlikely. Can you imagine a glass block of width a, made of glass whose refractive index varies continuously from 1.5 at one edge to zero at the other? Not very likely, yet there is a situation that comes to mind in which there is a continuous variation of refractive index from some basal value n0 to zero. I am thinking of Earth’s atmosphere (or indeed the atmosphere of any planet). As light from a star travels through Earth’s atmosphere, it moves not in a straight line, but in a slight curve, so that it is deviated through a few arc minutes before it reaches the astronomer’s telescope. For a star low down near the horizon, the refraction amounts to almost half a degree. This has to be taken into account when astronomers are making precise positional measurements. And sound waves, passing through the atmosphere, are also subject to refraction via the differential form of Snell’s law. The speed of sound (and hence the refractive index) varies with the temperature of the atmosphere, and hence with height in the atmosphere. Among the many applications of this sort of theory is the path of sound waves from meteorites hurtling through the atmosphere. This is discussed in a paper published in Meteoritics and Planetary Science 34, 572-585 (1999).


