2.5: Heat Engines and the Second Law of Thermodynamics
( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand\Dalpha
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[1], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dbeta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[2], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dgamma
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[3], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Ddelta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[4], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Depsilon
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[5], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dvarepsilon
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[6], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dzeta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[7], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Deta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[8], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dtheta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[9], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dvartheta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[10], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Diota
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[11], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dkappa
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[12], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dlambda
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[13], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Dvarpi
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[14], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\DGamma
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[15], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\DDelta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[16], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\DTheta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[17], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vmu
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[18], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vnu
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[19], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vxi
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[20], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vom
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[21], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vpi
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[22], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vvarpi
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[23], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vrho
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[24], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vvarrho
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[25], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vsigma
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[26], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vvarsigma
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[27], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vtau
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[28], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vupsilon
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[29], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vphi
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[30], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vvarphi
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[31], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vchi
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[32], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vpsi
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[33], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\Vomega
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[34], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\VGamma
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[35], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\VDelta
Callstack:
at (Template:MathJaxArovas), /content/body/div/p[1]/span[36], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\newcommand\BI{\mib I}}
\)
\newcommand { M}
\newcommand { m}
}
\( \newcommand\tcb{\textcolor{blue}\)
\( \newcommand\tcr{\textcolor{red}\)
1$#1_$
\newcommand\SZ{\textsf Z}} \( \newcommand\kFd{k\ns_{\RF\dar}\)
\newcommand\mutB{\tilde\mu}\ns_\ssr{B}
\( \newcommand\xhihOZ
Callstack:
at (Template:MathJaxArovas), /content/body/div/span[1], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
\( \newcommand\labar
Callstack:
at (Template:MathJaxArovas), /content/body/div/span[2], line 1, column 1
at template()
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/p/span, line 1, column 23
There’s no free lunch so quit asking
A heat engine is a device which takes a thermodynamic system through a repeated cycle which can be represented as a succession of equilibrium states: A→B→C⋯→A. The net result of such a cyclic process is to convert heat into mechanical work, or vice versa.
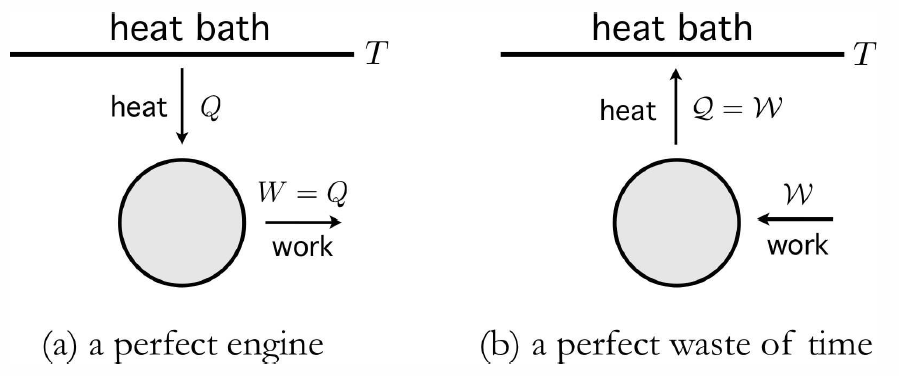
For a system in equilibrium at temperature T, there is a thermodynamically large amount of internal energy stored in the random internal motion of its constituent particles. Later, when we study statistical mechanics, we will see how each ‘quadratic’ degree of freedom in the Hamiltonian contributes 12kBT to the total internal energy. An immense body in equilibrium at temperature T has an enormous heat capacity C, hence extracting a finite quantity of heat Q from it results in a temperature change ΔT=−Q/C which is utterly negligible. Such a body is called a heat bath, or thermal reservoir. A perfect engine would, in each cycle, extract an amount of heat Q from the bath and convert it into work. Since ΔE=0 for a cyclic process, the First Law then gives W=Q. This situation is depicted schematically in Fig. [perfect]. One could imagine running this process virtually indefinitely, slowly sucking energy out of an immense heat bath, converting the random thermal motion of its constituent molecules into useful mechanical work. Sadly, this is not possible:
A transformation whose only final result is to extract heat froma source at fixed temperature and transform that heat into work is impossible.
This is known as the Postulate of Lord Kelvin. It is equivalent to the postulate of Clausius,
A transformation whose only result is to transfer heat from a body at a given temperature to a body at higher temperature is impossible.
These postulates which have been repeatedly validated by empirical observations, constitute the Second Law of Thermodynamics.
Engines and refrigerators
While it is not possible to convert heat into work with 100% efficiency, it is possible to transfer heat from one thermal reservoir to another one, at lower temperature, and to convert some of that heat into work. This is what an engine does. The energy accounting for one cycle of the engine is depicted in the left hand panel of Fig. [engref]. An amount of heat Q∗2>0 is extracted- from the reservoir at temperature T∗2. Since the reservoir is assumed to be enormous, its temperature change ΔT∗2=−Q∗2/C∗2 is negligible, and its temperature remains constant – this is what it means for an object to be a reservoir. A lesser amount of heat, Q∗1, with 0<Q∗1<Q∗2, is deposited in a second reservoir at a lower temperature T∗1. Its temperature change ΔT∗1=+Q∗1/C∗1 is also negligible. The difference W=Q∗2−Q∗1 is extracted as useful work. We define the efficiency, η, of the engine as the ratio of the work done to the heat extracted from the upper reservoir, per cycle: η=WQ∗2=1−Q∗1Q∗2 . This is a natural definition of efficiency, since it will cost us fuel to maintain the temperature of the upper reservoir over many cycles of the engine. Thus, the efficiency is proportional to the ratio of the work done to the cost of the fuel.
A refrigerator works according to the same principles, but the process runs in reverse. An amount of heat Q1 is extracted from the lower reservoir – the inside of our refrigerator – and is pumped into the upper reservoir. As Clausius’ form of the Second Law asserts, it is impossible for this to be the only result of our cycle. Some amount of work W must be performed on the refrigerator in order for it to extract the heat Q∗1. Since ΔE=0 for the cycle, a heat Q∗2=W+Q1 must be deposited into the upper reservoir during each cycle. The analog of efficiency here is called the coefficient of refrigeration, κ, defined as κ=Q∗1W=Q∗1Q∗2−Q∗1 . Thus, κ is proportional to the ratio of the heat extracted to the cost of electricity, per cycle.
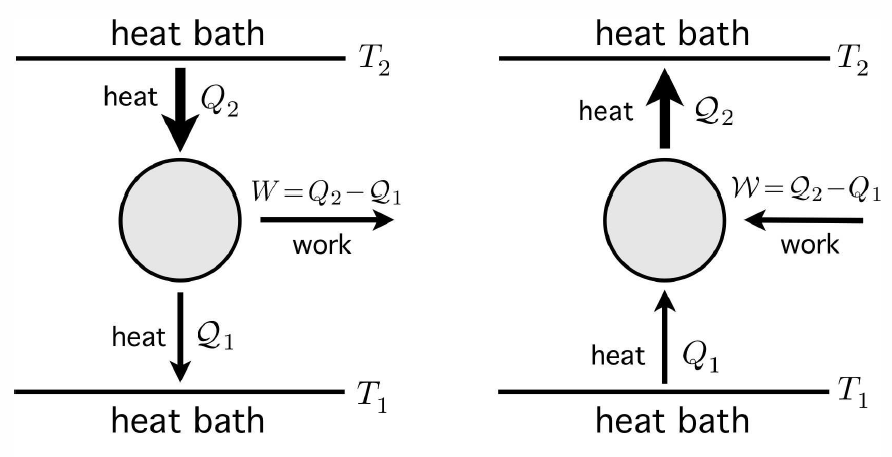
Please note the deliberate notation here. I am using symbols Q and W to denote the heat supplied to the engine (or refrigerator) and the work done by the engine, respectively, and Q and W to denote the heat taken from the engine and the work done on the engine.
A perfect engine has Q∗1=0 and η=1; a perfect refrigerator has Q∗1=Q∗2 and κ=∞. Both violate the Second Law. Sadi Carnot8 (1796 – 1832) realized that a reversible cyclic engine operating between two thermal reservoirs must produce the maximum amount of work W, and that the amount of work produced is independent of the material properties of the engine. We call any such engine a Carnot engine.
The efficiency of a Carnot engine may be used to define a temperature scale. We know from Carnot’s observations that the efficiency \boldsymbol{\eta_\ssr{C}} can only be a function of the temperatures T∗1 and T∗2: \boldsymbol{\eta_\ssr{C}=\eta_\ssr{C}(T\ns_1,T\ns_2)}. We can then define \boldsymbol{{T\ns_1\over T\ns_2}\equiv 1-\eta\ns_\ssr{C}(T\ns_1,T\ns_2)\ .} Below, in §6.4, we will see that how, using an ideal gas as the ‘working substance’ of the Carnot engine, this temperature scale coincides precisely with the ideal gas temperature scale from §2.4.
Nothing beats a Carnot engine
The Carnot engine is the most efficient engine possible operating between two thermal reservoirs. To see this, let’s suppose that an amazing wonder engine has an efficiency even greater than that of the Carnot engine. A key feature of the Carnot engine is its reversibility – we can just go around its cycle in the opposite direction, creating a Carnot refrigerator. Let’s use our notional wonder engine to drive a Carnot refrigerator, as depicted in Fig. [NBC].
We assume that \boldsymbol{{W\over Q\ns_2} = \eta\ns_\ssr{wonder} > \eta\ns_\ssr{Carnot} = {\CW'\over \CQ'_2}\ .} But from the figure, we have W=W′, and therefore the heat energy Q′2−Q∗2 transferred to the upper reservoir is positive. From W=Q∗2−Q∗1=Q′2−Q′1=W′ , we see that this is equal to the heat energy extracted from the lower reservoir, since no external work is done on the system: Q′2−Q∗2=Q′1−Q∗1>0 . Therefore, the existence of the wonder engine entails a violation of the Second Law. Since the Second Law is correct – Lord Kelvin articulated it, and who are we to argue with a Lord? – the wonder engine cannot exist.
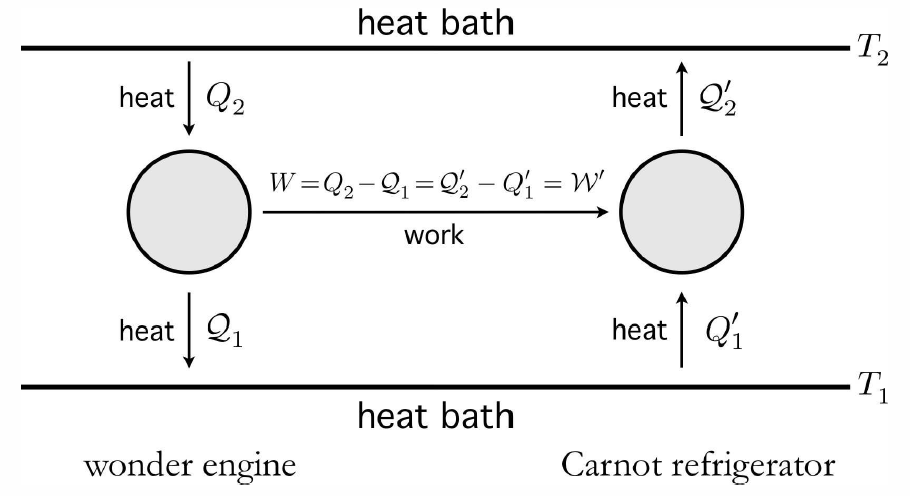
We further conclude that all reversible engines running between two thermal reservoirs have the same efficiency, which is the efficiency of a Carnot engine. For an irreversible engine, we must have \boldsymbol{\eta={W\over Q\ns_2}=1-{\CQ\ns_1\over Q\ns_2} \le 1-{T\ns_1\over T\ns_2}= \eta\ns_\ssr{C}\ .} Thus, Q∗2T∗2−Q∗1T∗1≤0 .
The Carnot cycle
Let us now consider a specific cycle, known as the Carnot cycle, depicted in Fig. [carnot]. The cycle consists of two adiabats and two isotherms. The work done per cycle is simply the area inside the curve on our p−V diagram: W=∮pdV . The gas inside our Carnot engine is called the ‘working substance’. Whatever it may be, the system obeys the First Law, dE=\mathchar′26dQ−\mathchar′26dW=\mathchar′26dQ−pdV .
We will now assume that the working material is an ideal gas, and we compute W as well as Q∗1 and Q∗2 to find the efficiency of this cycle. In order to do this, we will rely upon the ideal gas equations, E=νRTγ−1,pV=νRT , where γ=c∗p/c∗v=1+2f, where f is the effective number of molecular degrees of freedom contributing to the internal energy. Recall f=3 for monatomic gases, f=5 for diatomic gases, and f=6 for polyatomic gases. The finite difference form of the first law is ΔE=E∗f−E∗i=Q∗if−W∗if , where i denotes the initial state and f the final state.
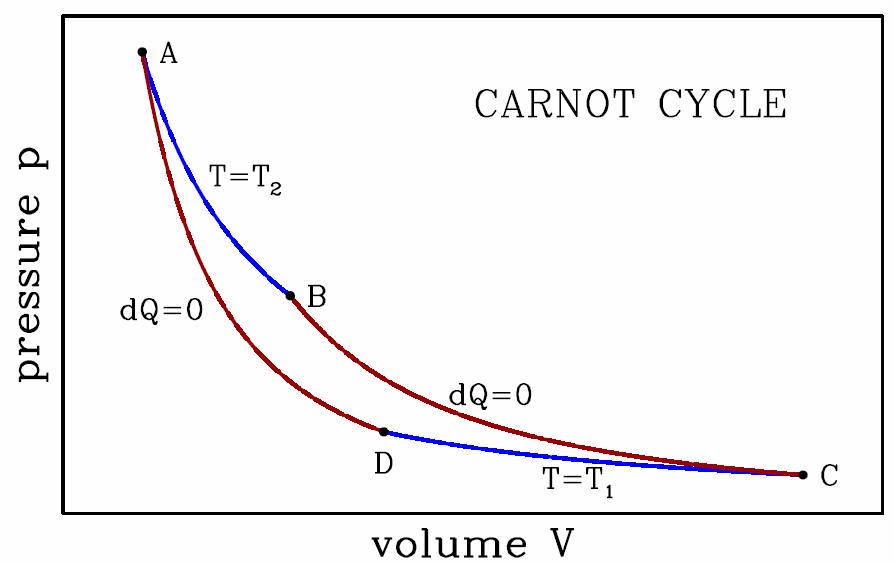
- This stage is an isothermal expansion at temperature T∗2. It is the ‘power stroke’ of the engine. We have \boldsymbol{\begin{aligned} W\ns_\ssr{AB}&=\int\limits_{V\subA}^{V\subB}\!\!dV\,{\nu R T\ns_2\over V} = \nu R T\ns_2\, \ln\bigg({V\subB\over V\subA}\bigg)\\ E\subA&=E\subB={\nu R T\ns_2\over \gamma-1}\ ,\end{aligned}} hence \boldsymbol{Q\ns_\ssr{AB}=\RDelta E\ns_\ssr{AB}+ W\ns_\ssr{AB} = \nu R T\ns_2\,\ln\bigg({V\subB\over V\subA}\bigg)\ .}
- This stage is an adiabatic expansion. We have \boldsymbol{\begin{aligned} Q\ns_\ssr{BC}&=0\\ \RDelta E\ns_\ssr{BC}&=E\ns_\ssr{C}-E\subB = {\nu R\over \gamma-1}\,(T\ns_1 - T\ns_2)\ .\end{aligned}} The energy change is negative, and the heat exchange is zero, so the engine still does some work during this stage: \boldsymbol{W\subBC=Q\ns_\ssr{BC}-\RDelta E\ns_\ssr{BC}= {\nu R\over \gamma-1}\, (T\ns_2 - T\ns_1)\ .}
- This stage is an isothermal compression, and we may apply the analysis of the isothermal expansion, mutatis mutandis: \boldsymbol{\begin{aligned} W\ns_\ssr{CD}&=\int\limits_{V\ns_\ssr{C}}^{V\ns_\ssr{D}}\!\!dV\,{\nu R T\ns_1\over V} = \nu R T\ns_1\, \ln\bigg({V\ns_\ssr{D}\over V\ns_\ssr{C}}\bigg)\\ E\ns_\ssr{C}&=E\ns_\ssr{D}={\nu R T\ns_1\over \gamma-1}\ ,\end{aligned}} hence \boldsymbol{Q\ns_\ssr{CD}=\RDelta E\ns_\ssr{CD}+ W\ns_\ssr{CD} = \nu R T\ns_1\,\ln\bigg({V\ns_\ssr{D}\over V\ns_\ssr{C}}\bigg)\ .}
- This last stage is an adiabatic compression, and we may draw on the results from the adiabatic expansion in BC: \boldsymbol{\begin{aligned} Q\ns_\ssr{DA}&=0\\ \RDelta E\ns_\ssr{DA}&=E\ns_\ssr{D}-E\subA= {\nu R\over \gamma-1}\,(T\ns_2 - T\ns_1)\ .\end{aligned}} The energy change is positive, and the heat exchange is zero, so work is done on the engine: \boldsymbol{W\ns_\ssr{DA}=Q\ns_\ssr{DA}-\RDelta E\ns_\ssr{DA}= {\nu R\over \gamma-1}\,(T\ns_1 - T\ns_2)\ .}
We now add up all the work values from the individual stages to get for the cycle \boldsymbol{\begin{split} W&=W\ns_\ssr{AB}+W\ns_\ssr{BC}+W\ns_\ssr{CD}+W\ns_\ssr{DA}\\ &=\nu R T\ns_2\,\ln\bigg({V\subB\over V\subA}\bigg)+\nu R T\ns_1\,\ln\bigg({V\ns_\ssr{D}\over V\ns_\ssr{C}}\bigg)\ . \end{split}} Since we are analyzing a cyclic process, we must have ΔE=0, we must have Q=W, which can of course be verified explicitly, by computing \boldsymbol{Q=Q\ns_\ssr{AB}+Q\ns_\ssr{BC}+Q\ns_\ssr{CD}+Q\ns_\ssr{DA}}. To finish up, recall the adiabatic ideal gas equation of state, d(TVγ−1)=0. This tells us that \boldsymbol{\begin{aligned} T\ns_2\, V_\ssr{B}^{\gamma-1}&= T\ns_1\, V_\ssr{C}^{\gamma-1} \\ T\ns_2\, V_\ssr{A}^{\gamma-1}&= T\ns_1\, V_\ssr{D}^{\gamma-1} \ .\end{aligned}} Dividing these two equations, we find \boldsymbol{{V\subB\over V\subA}={V\ns_\ssr{C}\over V\ns_\ssr{D}}\ ,} and therefore \boldsymbol{\begin{aligned} W&=\nu R(T\ns_2-T\ns_1)\,\ln\bigg({V\subB\over V\subA}\bigg)\\ Q\ns_\ssr{AB}&=\nu R T\ns_2\,\ln\bigg({V\subB\over V\subA}\bigg)\ .\end{aligned}} Finally, the efficiency is given by the ratio of these two quantities: \boldsymbol{\eta={W\over Q\ns_\ssr{AB}}=1-{T\ns_1\over T\ns_2}\ .}
The Stirling cycle
Many other engine cycles are possible. The Stirling cycle, depicted in Fig. [stirling], consists of two isotherms and two isochores. Recall the isothermal ideal gas equation of state, d(pV)=0. Thus, for an ideal gas Stirling cycle, we have \boldsymbol{p\subA V\ns_1=p\subB V\ns_2 \qquad,\qquad p\ns_\ssr{D} V\ns_1=p\ns_\ssr{C} V\ns_2\ ,} which says \boldsymbol{{p\subB\over p\subA}={p\subC\over p\subD}={V\ns_1\over V\ns_2}\ .}
- This isothermal expansion is the power stroke. Assuming ν moles of ideal gas throughout, we have pV=νRT∗2=p∗1V∗1, hence \boldsymbol{W\subAB=\int\limits_{V\ns_1}^{V\ns_2}\!\!dV\,{\nu R T\ns_2\over V}=\nu R T\ns_2\,\ln\bigg({V\ns_2\over V\ns_1}\bigg)\ .} Since AB is an isotherm, we have \boldsymbol{E\subA=E\subB}, and from \boldsymbol{\RDelta E\subAB=0} we conclude \boldsymbol{Q\subAB=W\subAB}.
- Isochoric cooling. Since dV=0 we have \boldsymbol{W\subBC=0}. The energy change is given by \boldsymbol{\RDelta E\subBC=E\subC-E\subB={\nu R(T\ns_1-T\ns_2)\over\gamma-1} ,} which is negative. Since \boldsymbol{W\subBC=0}, we have \boldsymbol{Q\subBC=\RDelta E\subBC}.
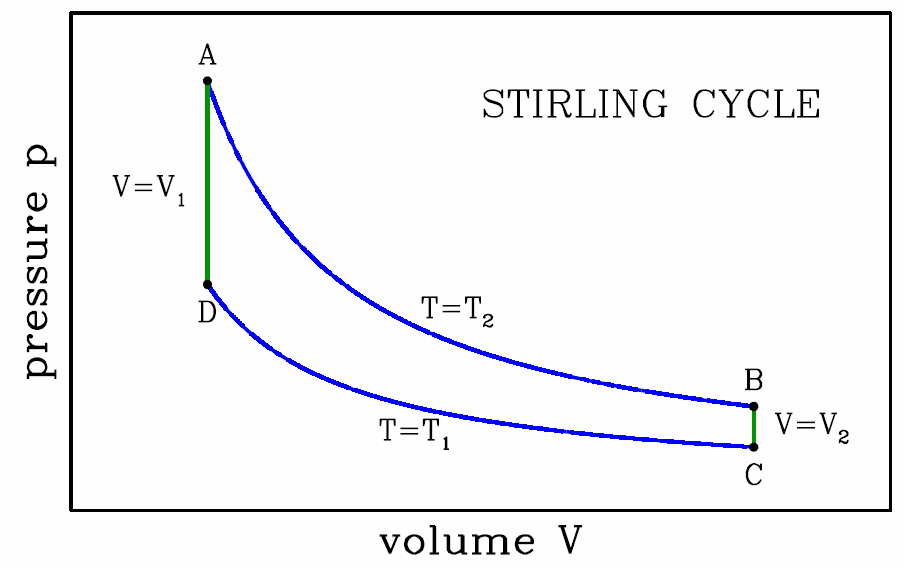
- Isothermal compression. Clearly \boldsymbol{W\subCD=\int\limits_{V\ns_2}^{V\ns_1}\!\!dV\,{\nu R T\ns_1\over V}= -\nu R T\ns_1\,\ln\bigg({V\ns_2\over V\ns_1}\bigg)\ .} Since CD is an isotherm, we have \boldsymbol{E\subC=E\subD}, and from \boldsymbol{\RDelta E\subCD=0} we conclude \boldsymbol{Q\subCD=W\subCD}.
- Isochoric heating. Since dV=0 we have \boldsymbol{W\subDA=0}. The energy change is given by \boldsymbol{\RDelta E\subDA=E\subA-E\subD={\nu R(T\ns_2-T\ns_1)\over\gamma-1}\ ,} which is positive, and opposite to \boldsymbol{\RDelta E\subBC}. Since \boldsymbol{W\subDA=0}, we have \boldsymbol{Q\subDA=\RDelta E\subDA}.
We now add up all the work contributions to obtain \boldsymbol{\begin{split} W&=W\ns_\ssr{AB}+W\ns_\ssr{BC}+W\ns_\ssr{CD}+W\ns_\ssr{DA}\\ &=\nu R (T\ns_2-T\ns_1)\,\ln\bigg({V\ns_2\over V\ns_1}\bigg)\ . \end{split}} The cycle efficiency is once again \boldsymbol{\eta={W\over Q\subAB}=1-{T\ns_1\over T\ns_2}\ .}
The Otto and Diesel cycles
The Otto cycle is a rough approximation to the physics of a gasoline engine. It consists of two adiabats and two isochores, and is depicted in Fig. [otto]. Assuming an ideal gas, along the adiabats we have d(pVγ)=0. Thus, \boldsymbol{p\subA\,V^\gamma_1=p\subB\,V^\gamma_2 \qquad,\qquad p\ns_\ssr{D}\,V^\gamma_1=p\ns_\ssr{C}\,V^\gamma_2\ ,} which says \boldsymbol{{p\subB\over p\subA}={p\subC\over p\subD}= \bigg({V\ns_1\over V\ns_2}\bigg)^{\!\gamma}\ .}
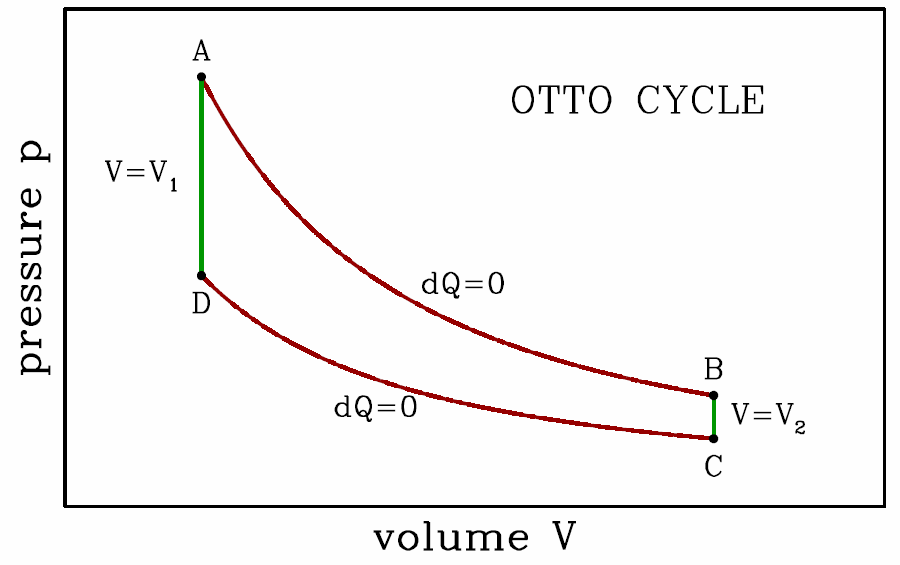
- Adiabatic expansion, the power stroke. The heat transfer is \boldsymbol{Q\subAB=0}, so from the First Law we have \boldsymbol{W\subAB=-\RDelta E\subAB=E\subA-E\subB}, thus \boldsymbol{W\subAB={p\subA V\ns_1-p\subB V\ns_2\over\gamma-1} ={p\subA V\ns_1\over \gamma-1}\Bigg[ 1-\bigg({V\ns_1\over V\ns_2}\bigg)^{\!\gamma-1} \Bigg]\ .} Note that this result can also be obtained from the adiabatic equation of state \boldsymbol{pV^\gamma=p\subA V_1^\gamma}: \boldsymbol{W\subAB=\int\limits_{V\ns_1}^{V\ns_2}\!\!p\,dV= p\subA V_1^\gamma\!\int\limits_{V\ns_1}^{V\ns_2}\!dV\,V^{-\gamma} ={p\subA V\ns_1\over \gamma-1}\Bigg[ 1-\bigg({V\ns_1\over V\ns_2}\bigg)^{\!\gamma-1} \Bigg]\ .}
- Isochoric cooling (exhaust); dV=0 hence \boldsymbol{W\subBC=0}. The heat \boldsymbol{Q\subBC} absorbed is then \boldsymbol{Q\subBC=E\subC-E\subB={V\ns_2\over\gamma-1}\,(p\subC-p\subB)\ .} In a realistic engine, this is the stage in which the old burned gas is ejected and new gas is inserted.
- Adiabatic compression; \boldsymbol{Q\subCD=0} and \boldsymbol{W\subCD=E\subC-E\subD}: \boldsymbol{W\subCD={p\subC V\ns_2 - p\subD V\ns_1\over \gamma-1} =-{p\subD V\ns_1\over \gamma-1}\Bigg[ 1-\bigg({V\ns_1\over V\ns_2}\bigg)^{\!\gamma-1} \Bigg]\ .}
- Isochoric heating, the combustion of the gas. As with BC we have dV=0, and thus \boldsymbol{W\subDA=0}. The heat \boldsymbol{Q\subDA} absorbed by the gas is then \boldsymbol{Q\subDA=E\subA-E\subD={V\ns_1\over\gamma-1}\,(p\subA-p\subD)\ .}

The total work done per cycle is then \boldsymbol{\begin{split} W&=W\ns_\ssr{AB}+W\ns_\ssr{BC}+W\ns_\ssr{CD}+W\ns_\ssr{DA}\\ &={(p\subA-p\subD)V\ns_1\over\gamma-1} \Bigg[ 1-\bigg({V\ns_1\over V\ns_2}\bigg)^{\!\gamma-1}\Bigg]\ , \end{split}} and the efficiency is defined to be \boldsymbol{\eta\equiv{W\over Q\subDA}=1-\bigg({V\ns_1\over V\ns_2}\bigg)^{\!\gamma-1}\ .} The ratio V∗2/V∗1 is called the compression ratio. We can make our Otto cycle more efficient simply by increasing the compression ratio. The problem with this scheme is that if the fuel mixture becomes too hot, it will spontaneously ‘preignite’, and the pressure will jump up before point D in the cycle is reached. A Diesel engine avoids preignition by compressing the air only, and then later spraying the fuel into the cylinder when the air temperature is sufficient for fuel ignition. The rate at which fuel is injected is adjusted so that the ignition process takes place at constant pressure. Thus, in a Diesel engine, step DA is an isobar. The compression ratio is \boldsymbol{r\equiv V\subB/V\subD}, and the cutoff ratio is \boldsymbol{s\equiv V\subA/V\subD}. This refinement of the Otto cycle allows for higher compression ratios (of about 20) in practice, and greater engine efficiency.
For the Diesel cycle, we have, briefly, \boldsymbol{\begin{split} W&=p\subA(V\subA-V\subD) + {p\subA V\subA-p\subB V\subB\over\gamma-1} + {p\subC V\subC-p\subD V\subD\over\gamma-1}\\ &={\gamma\,p\subA(V\subA-V\subD)\over\gamma-1} - {(p\subB-p\subC) V\subB\over\gamma-1} \end{split}} and \boldsymbol{Q\subDA={\gamma\,p\subA(V\subA-V\subD)\over\gamma-1}\ .} To find the efficiency, we will need to eliminate \boldsymbol{p\subB} and \boldsymbol{p\subC} in favor of \boldsymbol{p\subA} using the adiabatic equation of state d(pVγ)=0. Thus, \boldsymbol{p\subB=p\subA\cdot\bigg({V\subA\over V\subB}\bigg)^{\!\!\gamma}\qquad,\qquad p\subC=p\subA\cdot\bigg({V\subD\over V\subB}\bigg)^{\!\!\gamma}\ ,} where we’ve used \boldsymbol{p\subD=p\subA} and \boldsymbol{V\subC=V\subB}. Putting it all together, the efficiency of the Diesel cycle is \boldsymbol{\eta={W\over Q\subDA}=1-{1\over\gamma}\,{r^{1-\gamma} (s^\gamma-1)\over s-1}\ .}
The Joule-Brayton cycle
Our final example is the Joule-Brayton cycle, depicted in Fig. [jbray], consisting of two adiabats and two isobars. Along the adiabats we have Thus, \boldsymbol{p\ns_2\,V^\gamma_\ssr{A}=p\ns_1\,V^\gamma_\ssr{D} \qquad,\qquad p\ns_2\,V^\gamma_\ssr{B}=p\ns_1\,V^\gamma_\ssr{C}\ ,} which says \boldsymbol{{V\subD\over V\subA}={V\subC\over V\subB}= \bigg({p\ns_2\over p\ns_1}\bigg)^{\!\gamma^{-1}}\ .}
- This isobaric expansion at p=p∗2 is the power stroke. We have \boldsymbol{\begin{aligned} W\subAB&=\int\limits_{V\subA}^{V\subB}\!\!dV\,p\ns_2 = p\ns_2\,(V\subB-V\subA)\\ \RDelta E\subAB&=E\subB-E\subA={p\ns_2\,(V\subB-V\subA)\over\gamma-1}\\ Q\subAB&=\RDelta E\subAB + W\subAB= {\gamma \,p\ns_2\,(V\subB-V\subA)\over\gamma-1}\ .\end{aligned}}
- Adiabatic expansion; \boldsymbol{Q\subBC=0} and \boldsymbol{W\subBC=E\subB-E\subC}. The work done by the gas is \boldsymbol{\begin{split} W\subBC&={p\ns_2 V\subB-p\ns_1 V\subC\over\gamma-1}= {p\ns_2 V\subB\over\gamma-1}\bigg(1-{p\ns_1\over p\ns_2} \cdot{V\subC\over V\subB}\bigg)\\ &={p\ns_2\, V\subB\over \gamma-1}\Bigg[ 1-\bigg({p\ns_1\over p\ns_2} \bigg)^{\!1-\gamma^{-1}}\Bigg]\ . \end{split}}
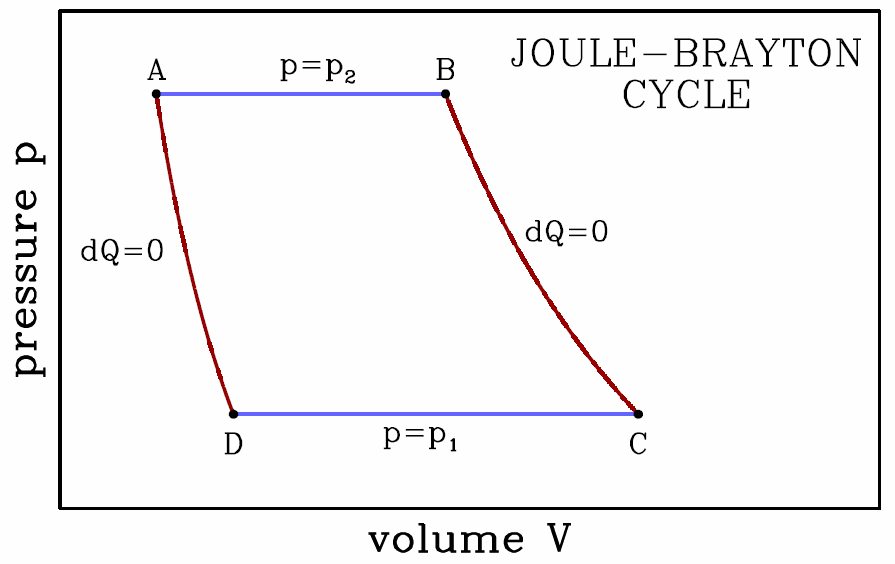
- Isobaric compression at p=p∗1. \boldsymbol{\begin{aligned} W\subCD&=\int\limits_{V\subC}^{V\subD}\!\!dV\,p\ns_1 = p\ns_1\,(V\subD-V\subC) =-p\ns_2\,(V\subB-V\subA)\,\bigg({p\ns_1\over p\ns_2}\bigg)^{\!1-\gamma^{-1}}\\ \RDelta E\subCD&=E\subD-E\subC={p\ns_1\,(V\subD-V\subC)\over\gamma-1}\\ Q\subCD&=\RDelta E\subCD + W\subCD= -{\gamma \,p\ns_2\over\gamma-1}\,(V\subB-V\subA)\,\bigg({p\ns_1\over p\ns_2} \bigg)^{\!1-\gamma^{-1}}\ .\end{aligned}}
- Adiabatic expansion; \boldsymbol{Q\subDA=0} and \boldsymbol{W\subDA=E\subD-E\subA}. The work done by the gas is \boldsymbol{\begin{split} W\subDA&={p\ns_1 V\subD-p\ns_2 V\subA\over\gamma-1}= -{p\ns_2 V\subA\over\gamma-1}\bigg(1-{p\ns_1\over p\ns_2} \cdot{V\subD\over V\subA}\bigg)\\ &=-{p\ns_2\, V\subA\over \gamma-1}\Bigg[ 1-\bigg({p\ns_1\over p\ns_2} \bigg)^{\!1-\gamma^{-1}}\Bigg]\ . \end{split}}
The total work done per cycle is then \boldsymbol{\begin{split} W&=W\ns_\ssr{AB}+W\ns_\ssr{BC}+W\ns_\ssr{CD}+W\ns_\ssr{DA}\\ &={\gamma\,p\ns_2\, (V\subB-V\subA)\over \gamma-1}\Bigg[ 1-\bigg({p\ns_1\over p\ns_2} \bigg)^{\!1-\gamma^{-1}}\Bigg] \end{split}} and the efficiency is defined to be \boldsymbol{\eta\equiv{W\over Q\subAB}=1-\bigg({p\ns_1\over p\ns_2}\bigg)^{\!1-\gamma^{-1}}\ .}
Carnot engine at maximum power output
While the Carnot engine described above in §6.4 has maximum efficiency, it is practically useless, because the isothermal processes must take place infinitely slowly in order for the working material to remain in thermal equilibrium with each reservoir. Thus, while the work done per cycle is finite, the cycle period is infinite, and the engine power is zero.
A modification of the ideal Carnot cycle is necessary to create a practical engine. The idea9 is as follows. During the isothermal expansion stage, the working material is maintained at a temperature T∗2w<T∗2. The temperature difference between the working material and the hot reservoir drives a thermal current, \mathchar′26dQ∗2dt=κ∗2(T∗2−T∗2w) . Here, κ∗2 is a transport coefficient which describes the thermal conductivity of the chamber walls, multiplied by a geometric parameter (which is the ratio of the total wall area to its thickness). Similarly, during the isothermal compression, the working material is maintained at a temperature T∗1w>T∗1, which drives a thermal current to the cold reservoir, \mathchar′26dQ∗1dt=κ∗1(T∗1w−T∗1) . Now let us assume that the upper isothermal stage requires a duration Δt∗2 and the lower isotherm a duration Δt∗1. Then Q∗2=κ∗2Δt†2(T∗2−T∗2w)Q∗1=κ∗1Δt†1(T∗1w−T∗1) . Since the engine is reversible, we must have Q∗1T∗1w=Q∗2T∗2w , which says Δt∗1Δt∗2=κ∗2T∗1w(T∗2−T∗2w)κ∗1T∗2w(T∗1w−T∗1) . The power is P=Q∗2−Q∗1(1+α)(Δt∗1+Δt∗2) , where we assume that the adiabatic stages require a combined time of α(Δt∗1+Δt∗2). Thus, we find P=κ∗1κ∗21+α⋅(T∗2w−T∗1w)(T∗1w−T∗1)(T∗2−T∗2w)κ∗1T∗2w(T∗1w−T∗1)+κ∗2T∗1w(T∗2−T∗2w)
| Power source | T∗1 (∘C) | T∗2 (∘C) | \boldsymbol{\eta\ns_\ssr{Carnot}} | η (theor.) | η (obs.) |
|---|---|---|---|---|---|
| West Thurrock (UK) | |||||
| Coal Fired Steam Plant | ∼25 | 565 | 0.641 | 0.40 | 0.36 |
| CANDU (Canada) | |||||
| PHW Nuclear Reactor | ∼25 | 300 | 0.480 | 0.28 | 0.30 |
| Larderello (Italy) | |||||
| Geothermal Steam Plant | ∼80 | 250 | 0.323 | 0.175 | 0.16 |
We optimize the engine by maximizing P with respect to the temperatures T∗1w and T∗2w. This yields T∗2w=T∗2−T∗2−√T∗1T∗21+√κ∗2/κ∗1T∗1w=T∗1+√T∗1T∗2−T∗11+√κ∗1/κ∗2 . The efficiency at maximum power is then \[\eta={Q\ns_2-\CQ\ns_1\over Q\ns_2}=1-{T\ns_{1\Rw}\over T\ns_{2\Rw}}= 1-\sqrt
Callstack:
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/div[8]/p[3]/span[1], line 1, column 2
Callstack:
at (Bookshelves/Thermodynamics_and_Statistical_Mechanics/Book:_Thermodynamics_and_Statistical_Mechanics_(Arovas)/02:_Thermodynamics/2.05:_Heat_Engines_and_the_Second_Law_of_Thermodynamics), /content/body/div[8]/p[3]/span[2], line 1, column 1
Table [pptab], taken from the article of Curzon and Albhorn (1975), shows how the efficiency of this practical Carnot cycle, given by Equation [MCeff], rather accurately predicts the efficiencies of functioning power plants.


