8.3: Mechanical Energy and Conservation of Energy
- Page ID
- 19414
Recall the Work-Energy Theorem, which relates the net work done on an object to its change in kinetic energy, along a path from point \(A\) to point \(B\):
\[\begin{aligned} W^{net}=\Delta K = K_B - K_A\end{aligned}\]
where \(K_A\) is the object’s initial kinetic energy and \(K_B\) is its final kinetic energy. Generally, the net work done is the sum of the work done by conservative forces, \(W^C\), and the work done by none conservative forces, \(W^{NC}\):
\[\begin{aligned} W^{net}=W^C+W^{NC}\end{aligned}\]
The work done by conservative forces can be expressed in terms of changes in potential energy functions. For example, suppose that two conservative forces, \(\vec F_1\) and \(\vec F_2\), are exerted on the object. The work done by those two forces is given by:
\[\begin{aligned} W_1 &= -\Delta U_1\\[4pt] W_2 &= -\Delta U_2\end{aligned}\]
where \(U_1\) and \(U_2\) are the changes in potential energy associated with forces \(\vec F_1\) and \(\vec F_2\), respectively. We can re-arrange the Work-Energy Theorem as follows1:
\[\begin{aligned} W^{net}=W^C+W^{NC}=-\Delta U_1 - \Delta U_2 +W^{NC} &= \Delta K\\[4pt] \therefore W^{NC} = \Delta U_1 + \Delta U_2 + \Delta K\end{aligned}\]
That is, the work done by non-conservative forces is equal to the sum of the changes in potential and kinetic energies. In general, we can use \(\Delta U\) to represent the change in the total potential energy of the object. The total potential energy is the sum of the potential energies associated with each of the conservative forces acting on the object (\(\Delta U = \Delta U_1 + \Delta U_2\) above). The above expression can thus be written in a more general form:
\[W^{NC}=\Delta U+\Delta K\]
In particular, note that if there are no non-conservative forces doing work on the object:
\[\Delta K+\Delta U=0\text{ if no non-conservative forces}\]
\(-\Delta U=\Delta K\)
That is, the sum of the changes in potential and kinetic energies of the object is always zero. This means that if the potential energy of the object increases, then the kinetic energy of the object must decrease by the same amount.
We can introduce the “mechanical energy”, \(E\), of an object as the sum of the potential and kinetic energies of the object:
\[E=U+K\]
If the object started at position \(A\), with potential energy \(U_A\) and kinetic energy \(K_A\), and ended up at position \(B\) with potential energy \(U_B\) and kinetic energy \(K_B\), then we can write the mechanical energy at both positions and its change \(\Delta E\), as:
\[\begin{aligned} E_A &= U_A + K_A\\[4pt] E_B &= U_B + K_B\\[4pt] \Delta E &= E_B - E_A \\[4pt] &= U_B + K_B - U_A - K_A\\[4pt] \therefore \Delta E &= \Delta U + \Delta K\end{aligned}\]
Thus, the change in mechanical energy of the object is equal to the work done by non-conservative forces:
\[\begin{aligned} W^{NC} = \Delta U + \Delta K = \Delta E\end{aligned}\]
and if there is no work done by non-conservative forces on the object, then the mechanical energy of the object does not change:
\[\begin{aligned} \Delta E &= 0\quad\text{if no non-conservative forces}\\[4pt] \therefore E &= \text{constant}\end{aligned}\]
This is what we generally call the “conservation of mechanical energy”. If there are no non-conservative forces doing work on an object, its mechanical energy is conserved (i.e. constant).
The introduction of mechanical energy gives us a completely different way to think about mechanics. We can now think of an object as having “energy” (potential and/or kinetic), and we can think of forces as changing the energy of the object.
Is the value of an an object’s mechanical energy meaningful, or is it only the difference in mechanical energy that is meaningful?
- Yes, the value of the mechanical energy is meaningful. At any given time, an object will have a quantifiable amount of mechanical energy.
- No, the value is not meaningful because the value of potential energy is arbitrary. Only differences in mechanical energy are meaningful.
- No, the value is not meaningful because both the potential and kinetic energies are arbitrary. Their values will change depending on where you set the energy to be zero.
- It depends on which conservative forces act on the object (and therefore what “kind” of potential energy the object has).
- Answer
-
B.
We can also think of the work done by non-conservative forces as a type of change in energy. For example, the work done by friction can be thought of as a change in thermal energy (feel the burn as you rub your hand vigorously on a table!). If we can model the work done by non-conservative forces as a type of “other” energy, \(-W^{NC}=\Delta E^{other}\), then we can state that:
\[\begin{aligned} \Delta E^{other} + \Delta U + \Delta K =0\end{aligned}\]
which is what we usually refer to as “conservation of energy”. That is, the total energy in a system, including kinetic, potential and any other form (e.g. thermal, electrical, etc.) is constant unless some external agent is acting on the system.
We can always include that external agent in the system so that the total energy of the system is constant. The largest system that we can have is the Universe itself. Thus, the total energy in the Universe is constant and can only transform from one type into another, but no energy can ever be added or removed from the Universe.
Here’s an example that may help you understand the concept of external agents and energy conservation. Say we have a mass that hangs from a spring, so that the mass oscillates up and down like a yo-yo. If we define our system to include the spring, the mass, and gravity, energy will be conserved (the energy is transformed from potential energy to kinetic energy and back again).
Now, what if someone is holding the end of the spring and they start walking so that the whole system accelerates? Energy is not conserved because the system is gaining kinetic energy, seemingly out of nowhere. The system is being acted on by an external agent (the person). If we expand our system so that it includes the spring, the mass, gravity, and the person, energy is conserved. Instead of the kinetic energy “coming out of nowhere”, we can see that it is actually coming from the person converting chemical energy in their body in order to move their muscles.
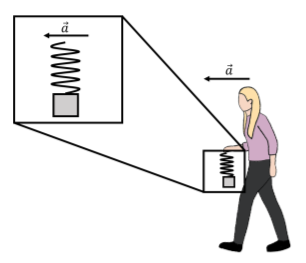
But what if there’s an external agent acting on our new system? We can keep “zooming out” to include more and more external sources in the definition of our system. If you kept zooming out, eventually you would reach the point where the whole Universe was included in your system. At this point, you can’t zoom out any more. This means that, if the Universe is your system, energy must always be conserved because there can’t be any external agents acting on the system.
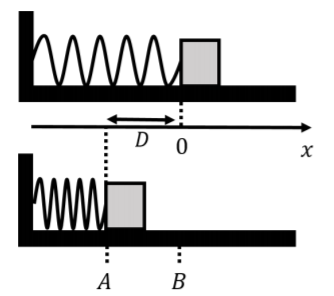
A block of mass \(m\) can slide along a horizontal frictionless surface. A horizontal spring, with spring constant, \(k\), is attached to a wall on one end, while the other end can move, as shown in Figure 8.3.2. A coordinate system is defined such that the \(x\) axis is horizontal and the free end of the spring is at \(x=0\) when the spring is at rest. The block is pushed against the spring so that the spring is compressed by a distance \(D\). The block is then released. What speed will the block have when it leaves the spring?
Solution
This is again the same example that we saw in Chapters 6 and 7. We will show here that it is solved very easily using conservation of energy. The forces acting on the block are:
- Weight, which does no work since it is perpendicular to the block’s displacement.
- The normal force, which does no work since it is perpendicular to the block’s displacement.
- The force from the spring, which is conservative and can be modelled with a potential energy \(U(x)=\frac{1}{2}kx^2\), where \(x\) is the position of the end of the spring.
The block starts at rest at position \(A\) (\(x=-D\)), where the spring is compressed by a distance \(D\), and leaves the spring at position \(B\) (\(x=0\)), where the spring is at its rest position.
At position \(A\), the kinetic energy of the block is \(K_A=0\) since the block is at rest, and the potential energy from the spring force of the block is \(U_A=\frac{1}{2}kD^2\). The mechanical energy of the block at position \(A\) is thus:
\[\begin{aligned} K_A&=0\\[4pt] U_A&=\frac{1}{2}kD^2\\[4pt] \therefore E_A &= U_A + K_A = \frac{1}{2}kD^2\end{aligned}\]
At position \(B\), the spring potential energy of the block is zero (since the spring is at rest), and all of the energy is kinetic:
\[\begin{aligned} K_B&=\frac{1}{2}mv_B^2\\[4pt] U_B&=0\\[4pt] \therefore E_B &= U_B+K_B=\frac{1}{2}mv_B^2\end{aligned}\]
Since there are no non-conservative forces doing work on the block, the mechanical energies at \(A\) and \(B\) are the same:
\[\begin{aligned} W^{NC}&=\Delta E=E_B-E_A= 0\\[4pt] \therefore E_B&=E_A\\[4pt] \frac{1}{2}mv_B^2&= \frac{1}{2}kD^2\\[4pt] v_B &= \sqrt{\frac{kD^2}{m}}\end{aligned}\]
as we found previously.
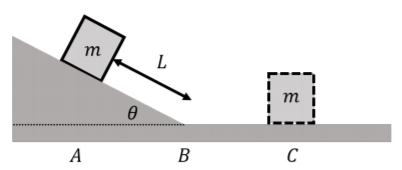
A block of mass \(m\) is placed at rest on an incline that makes an angle \(\theta\) with respect to the horizontal, as shown in Figure \(\PageIndex{3}\). The block is nudged slightly so that the force of static friction is overcome and the block starts to accelerate down the incline. At the bottom of the incline, the block slides on a horizontal surface. The coefficient of kinetic friction between the block and the incline is \(\mu_{k1}\), and the coefficient of kinetic friction between the block and horizontal surface is \(\mu_{k2}\). If one assumes that the block started at rest a distance \(L\) from the bottom of the incline, how far along the horizontal surface will the block slide before stopping?
Solution
This is the same problem we solved in Example 6.2.1. In that case, we solved for the acceleration of the block using Newton’s Second Law and then used kinematics to find how far the block went. We can solve this problem in a much easier way using conservation of energy.
It is still a good idea to think about what forces are applied on the object in order to determine if there are non-conservative forces doing work. In this case, the forces on the block are:
- The normal force, which does no work, as it is always perpendicular to the motion.
- Weight, which does work when the height of the object changes, which we can model with a potential energy function.
- Friction, which is a non-conservative force, whose work we must determine.
Let us divide the motion into two segments: (1) a segment along the incline (positions \(A\) to \(B\) in Figure \(\PageIndex{3}\)), where gravitational potential energy changes, and (2), the horizontal segment from positions \(B\) to position \(C\) on the figure. We can then apply conservation of energy for each segment.
Starting with the first segment, we can choose the gravitational potential energy to be zero when the block is at the bottom of the incline. The block starts at a height \(h=L\sin\theta\) above the bottom of the incline. The gravitational potential energy for the beginning and end of the first segment are thus:
\[\begin{aligned} U_A &= mgL\sin\theta\\[4pt] U_B &= 0\end{aligned}\]
Since the block starts at rest, its kinetic energy is zero at position \(A\), and if the speed of the box is \(v_B\) at position \(B\), we can write its kinetic energy at both positions as:
\[\begin{aligned} K_A &=0\\[4pt] K_B &= \frac{1}{2}mv_B^2\end{aligned}\]
The mechanical energy of the object at positions \(A\) and \(B\) is thus:
\[\begin{aligned} E_A &= U_A+K_A = mgL\sin\theta\\[4pt] E_B &= U_B+K_B = \frac{1}{2}mv_B^2\\[4pt] \Delta E &= E_B - E_A = \frac{1}{2}mv_B^2 - mgL\sin\theta\end{aligned}\]
Finally, since we have a non-conservative force, the force of kinetic friction, acting on the first segment, we need to calculate the work done by that force. We found in Example 6.2.1 that the force of friction had magnitude \(f_k=\mu_{k1}N=\mu_{k1}mg\cos\theta\). Since the force of friction is anti-parallel to the displacement vector, which points down the incline and has length \(L\), the work done by friction is:
\[\begin{aligned} W^{NC}=W_f = -f_kL=-\mu_{k1}mg\cos\theta L\end{aligned}\]
Applying conservation of energy along the first segment, we have:
\[\begin{aligned} W^{NC} &= \Delta E\\[4pt] -\mu_{k1}mg\cos\theta L &= \frac{1}{2}mv_B^2 - mgL\sin\theta\\[4pt] \therefore \frac{1}{2}mv_B^2 &= mgL\sin\theta-\mu_{k1}mg\cos\theta L \end{aligned}\]
Note that the above equation, in words, could be read as, “the change in kinetic energy (\(\frac{1}{2}mv_B^2\)) is equal to the negative change in potential energy (\(mgL\sin\theta\)) minus the work done by friction (\(\mu_{k1}mg\cos\theta L\))”. In other words, the block had potential energy, which was converted into kinetic energy and heat (the work done by friction can be thought of as thermal energy).
We now proceed in an analogous way for the second segment, from position \(B\) to position \(C\). The only force that can do work along this segment (of length \(x\)) is the force of kinetic friction, since both the weight and normal force are perpendicular to the displacement. There are no conservative forces doing work, so there is no change in potential energy. The initial kinetic energy is \(K_B\) (from above), and the final kinetic energy, \(K_C\), is zero. The change in mechanical energy is thus:
\[\begin{aligned} \Delta E &= E_C - E_B = K_C - K_B = -K_B\\[4pt] &=-\frac{1}{2}mv_B^2\\[4pt] &=- mgL\sin\theta+\mu_{k1}mg\cos\theta L \end{aligned}\]
where, in the last line, we used the result from the first segment. The work done by the force of friction along the horizontal segment of (undetermined) length \(x\) is:
\[\begin{aligned} W^{NC}=W_f = -f_kx = -\mu_{k2} N x=-\mu_{k2} mg x\end{aligned}\]
Finally, we can find \(x\) by setting the work done by non-conservative forces equal to the change in mechanical energy:
\[\begin{aligned} W^{NC} &= \Delta E\\[4pt] -\mu_{k2} mg x &=- mgL\sin\theta+\mu_{k1}mg\cos\theta L \\[4pt] \therefore x&= L\frac{1}{\mu_{k2}}\left(\sin\theta - \mu_{k1}\cos\theta\right)\end{aligned}\]
which is the same result that we obtained in Example 6.2.1.
Discussion
By using conservation of energy, we were able to model the motion of the block down the incline in a way that was much easier than what was done in Example 6.2.1. Furthermore, although we modeled friction as a non-conservative force doing work, we gained some insight into the idea that this could be thought of as an energy loss. In terms of energy, we would say that the block initially had gravitational potential energy, which was then converted into kinetic energy as well as thermal energy (in the heat generated by friction).
Footnotes
1. This is why we defined potential energy as negative of the work; it becomes a positive term when we move it to the same side of the equation as the kinetic energy!

