16.5: Momentum and Radiation Pressure
( \newcommand{\kernel}{\mathrm{null}\,}\)
By the end of this section, you will be able to:
- Describe the relationship of the radiation pressure and the energy density of an electromagnetic wave
- Explain how the radiation pressure of light, while small, can produce observable astronomical effects
Material objects consist of charged particles. An electromagnetic wave incident on the object exerts forces on the charged particles, in accordance with the Lorentz force. These forces do work on the particles of the object, increasing its energy, as discussed in the previous section. The energy that sunlight carries is a familiar part of every warm sunny day. A much less familiar feature of electromagnetic radiation is the extremely weak pressure that electromagnetic radiation produces by exerting a force in the direction of the wave. This force occurs because electromagnetic waves contain and transport momentum.
To understand the direction of the force for a very specific case, consider a plane electromagnetic wave incident on a metal in which electron motion, as part of a current, is damped by the resistance of the metal, so that the average electron motion is in phase with the force causing it. This is comparable to an object moving against friction and stopping as soon as the force pushing it stops (Figure 16.5.1). When the electric field is in the direction of the positive y-axis, electrons move in the negative y-direction, with the magnetic field in the direction of the positive z-axis. By applying the right-hand rule, and accounting for the negative charge of the electron, we can see that the force on the electron from the magnetic field is in the direction of the positive x-axis, which is the direction of wave propagation. When the →E field reverses, the →B field does too, and the force is again in the same direction. Maxwell’s equations together with the Lorentz force equation imply the existence of radiation pressure much more generally than this specific example, however.
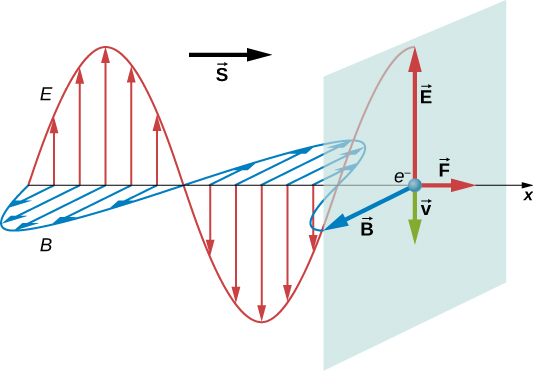
Maxwell predicted that an electromagnetic wave carries momentum. An object absorbing an electromagnetic wave would experience a force in the direction of propagation of the wave. The force corresponds to radiation pressure exerted on the object by the wave. The force would be twice as great if the radiation were reflected rather than absorbed.
Maxwell’s prediction was confirmed in 1903 by Nichols and Hull by precisely measuring radiation pressures with a torsion balance. The schematic arrangement is shown in Figure 16.5.2. The mirrors suspended from a fiber were housed inside a glass container. Nichols and Hull were able to obtain a small measurable deflection of the mirrors from shining light on one of them. From the measured deflection, they could calculate the unbalanced force on the mirror, and obtained agreement with the predicted value of the force.

The radiation pressure prad applied by an electromagnetic wave on a perfectly absorbing surface turns out to be equal to the energy density of the wave:
prad=u ⏟Perfect absorber.
If the material is perfectly reflecting, such as a metal surface, and if the incidence is along the normal to the surface, then the pressure exerted is twice as much because the momentum direction reverses upon reflection:
prad=2u⏟Perfect reflector.
We can confirm that the units are right:
[u]=Jm3=N⋅mm3=Nm2=unitsofpressure.
Equations ??? and ??? give the instantaneous pressure, but because the energy density oscillates rapidly, we are usually interested in the time-averaged radiation pressure, which can be written in terms of intensity:
p=⟨prad⟩={I/cPerfect absorber2I/cPerfect reflector
Radiation pressure plays a role in explaining many observed astronomical phenomena, including the appearance of comets. Comets are basically chunks of icy material in which frozen gases and particles of rock and dust are embedded. When a comet approaches the Sun, it warms up and its surface begins to evaporate. The coma of the comet is the hazy area around it from the gases and dust. Some of the gases and dust form tails when they leave the comet. Notice in Figure 16.5.3 that a comet has two tails. The ion tail (or gas tail) is composed mainly of ionized gases. These ions interact electromagnetically with the solar wind, which is a continuous stream of charged particles emitted by the Sun. The force of the solar wind on the ionized gases is strong enough that the ion tail almost always points directly away from the Sun. The second tail is composed of dust particles. Because the dust tail is electrically neutral, it does not interact with the solar wind. However, this tail is affected by the radiation pressure produced by the light from the Sun. Although quite small, this pressure is strong enough to cause the dust tail to be displaced from the path of the comet.
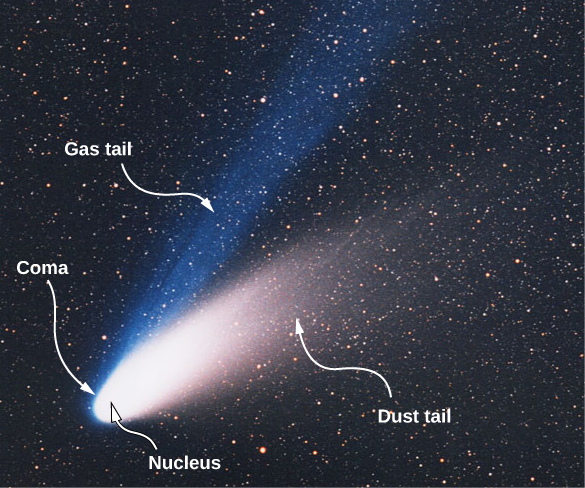
On February 9, 1986, Comet Halley was at its closest point to the Sun, about 9.0×1010m from the center of the Sun. The average power output of the Sun is 3.8×1026W.
- Calculate the radiation pressure on the comet at this point in its orbit. Assume that the comet reflects all the incident light.
- Suppose that a 10-kg chunk of material of cross-sectional area 4.0×10−2m2 breaks loose from the comet. Calculate the force on this chunk due to the solar radiation. Compare this force with the gravitational force of the Sun.
Strategy
Calculate the intensity of solar radiation at the given distance from the Sun and use that to calculate the radiation pressure. From the pressure and area, calculate the force.
Solution
a. The intensity of the solar radiation is the average solar power per unit area. Hence, at 9.0×1010m from the center of the Sun, we have
I=Savg=3.8×1026W4π(9.0×1010m)2=3.7×103W/m2.
Assuming the comet reflects all the incident radiation, we obtain from Equation ???
p=2Ic=2(3.7×103W/m2)3.00×108m/s=2.5×10−5N/m2.
b. The force on the chunk due to the radiation is
F=pA=(2.5×10−5N/m2)(4.0×10−2m2)=1.0×10−6N,
whereas the gravitational force of the Sun is
Fg=GMmr2=(6.67×10−11N⋅m2/kg2)(2.0×1030kg)(10kg)(9.0×1010m)2=0.16N.
Significance
The gravitational force of the Sun on the chunk is therefore much greater than the force of the radiation.
After Maxwell showed that light carried momentum as well as energy, a novel idea eventually emerged, initially only as science fiction. Perhaps a spacecraft with a large reflecting light sail could use radiation pressure for propulsion. Such a vehicle would not have to carry fuel. It would experience a constant but small force from solar radiation, instead of the short bursts from rocket propulsion. It would accelerate slowly, but by being accelerated continuously, it would eventually reach great speeds. A spacecraft with small total mass and a sail with a large area would be necessary to obtain a usable acceleration.
When the space program began in the 1960s, the idea started to receive serious attention from NASA. The most recent development in light propelled spacecraft has come from a citizen-funded group, the Planetary Society. It is currently testing the use of light sails to propel a small vehicle built from CubeSats, tiny satellites that NASA places in orbit for various research projects during space launches intended mainly for other purposes.
The LightSail spacecraft shown below (Figure 16.5.4) consists of three CubeSats bundled together. It has a total mass of only about 5 kg and is about the size as a loaf of bread. Its sails are made of very thin Mylar and open after launch to have a surface area of 32m2.

The first LightSail spacecraft was launched in 2015 to test the sail deployment system. It was placed in low-earth orbit in 2015 by hitching a ride on an Atlas 5 rocket launched for an unrelated mission. The test was successful, but the low-earth orbit allowed too much drag on the spacecraft to accelerate it by sunlight. Eventually, it burned in the atmosphere, as expected. The next Planetary Society’s LightSail solar sailing spacecraft is scheduled for 2018.

The Lightsail is based on the on NASA's NanoSail-D project. (Public domain; NASA).
LightSail Acceleration
The intensity of energy from sunlight at a distance of 1 AU from the Sun is 1370W/m2. The LightSail spacecraft has sails with total area of 32m2 and a total mass of 5.0 kg. Calculate the maximum acceleration LightSail spacecraft could achieve from radiation pressure when it is about 1 AU from the Sun.
Strategy
The maximum acceleration can be expected when the sail is opened directly facing the Sun. Use the light intensity to calculate the radiation pressure and from it, the force on the sails. Then use Newton’s second law to calculate the acceleration.
Solution
The radiation pressure is
F=pA=2uA=2IcA=2(1370W/m2)(32m2)(3.00×108m/s)=2.92×10−4N.
The resulting acceleration is
a=Fm=2.92×10−4N5.0kg=5.8×10−5m/s2.
Significance
If this small acceleration continued for a year, the craft would attain a speed of 1829 m/s, or 6600 km/h.
How would the speed and acceleration of a radiation-propelled spacecraft be affected as it moved farther from the Sun on an interplanetary space flight?
Solution
Its acceleration would decrease because the radiation force is proportional to the intensity of light from the Sun, which decreases with distance. Its speed, however, would not change except for the effects of gravity from the Sun and planets.


