9.6: Rolling Motion
( \newcommand{\kernel}{\mathrm{null}\,}\)
As a step up from a statics problem, we may consider a situation in which the sum of the external forces is zero, as well as the sum of the external torques, yet the system is moving. We call this “unforced motion.” The first condition, ∑→Fext=0, means that the center of mass of the system must be moving with constant velocity; the second condition means that the total angular momentum must be constant. For a rigid body, this means that the most general kind of unforced motion can be described as a translation of the center of mass with constant velocity, accompanied by a rotation with constant angular velocity around the center of mass. For an extended, deformable system, on the other hand, the presence of internal forces can make the general motion a lot more complicated. Just think, for instance, of the solar system: although everything is, loosely speaking, revolving around the sun, the motions of individual planets and (especially) moons can be fairly complicated.
A simple example of (for practical purposes) unforced motion is provided by a symmetric, rigid object (such as a ball, or a wheel) rolling on a flat surface. The normal and gravity forces cancel each other, and since they lie along the same line their torques cancel too, so both →vcm and →L remain constant. In principle, you could imagine removing the ground and gravity and nothing would change: the same motion (in the absence of air resistance) would just continue forever.
In practice, there is energy dissipation associated with rolling motion, primarily because, if the rolling object is not perfectly rigid6, then, as it rolls, different parts of it get compressed under the combined pressure of gravity and the normal force, expand again, get compressed again... This kind of constant “squishing” ends up converting macroscopic kinetic energy into thermal energy: you may have noticed that the tires on a car get warm as you drive around, and you may also be familiar with the fact that you get a better gas mileage (less energy dissipation) when your tires are inflated to the right pressure than when they are low (because they are more “rigid,” less deformable, in the first case).
This conversion of mechanical energy into thermal energy can be formally described by introducing another “friction” force that we call the force of rolling friction. Eventually, rolling friction alone would bring any rolling object to a stop, even in the absence of air resistance. It is, however, usually much weaker than sliding friction, so we will continue to ignore it from now on. You may have noticed already that typically an object can roll on a surface much farther than it can slide without rolling on the same surface. In fact, what happens often is that, if you try to send the object (for instance, a billiard ball) sliding, it will lose kinetic energy rapidly to the force of kinetic friction, but it will also start spinning under the influence of the same force, until a critical point is reached when the condition for rolling without slipping is satisfied:
|→vcm|=R|ω|.
At this point, the object will start rolling without slipping, and losing speed at a much slower rate.
The origin of the condition (???) is fairly straightforward. You can imagine an object that is rolling without slipping as “measuring the surface” as it rolls (or vice-versa, the surface measuring the circumference of the object as its different points are pressed against it in succession). So, after it has completed a revolution (2π radians), it should have literally “covered” a distance on the surface equal to 2πR, that is, advanced a distance 2πR. But the same has to be true, proportionately, for any rotation angle Δθ other that 2π: since the length of the corresponding arc is s=R|Δθ|, in a rotation over an angle |Δθ| the center of mass of the object must have advanced a distance |Δxcm|=s=R|Δθ|. Dividing by Δt as Δt→0 then yields Equation (???).
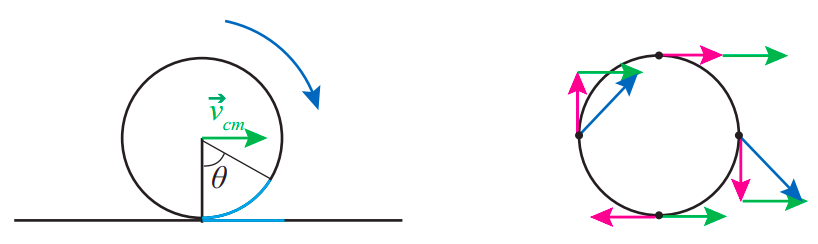
Note that, unlike Equation (8.4.12), which it very much resembles, Equation (???) is not a “vector identity in disguise”: there is nothing like Equation (9.3.6) that we could substitute for it in order to make the signs automatically come out right. You should just treat it as a relationship between the magnitudes of →vcm and →ω and just pick the signs appropriately for each circumstance, based on your convention for positive directions of translation and rotation.
In fact, we could use Equation (9.3.6) to find the velocity of any point on the circle, if we go to a reference frame where the center is at rest—which is to say, the center of mass (CM) reference frame; then, to go back to the Earth frame, we just have to add →vcm (as a vector) to the vector we obtained in the CM frame. Figure 9.6.1 shows the result. Note, particularly, that the point at the very bottom of the circle has a velocity −R|ω| in the CM frame, but when we go back to the Earth frame, its velocity is −R|ω|+vcm=−R|ω|+R|ω|=0 (by the condition (???)). Thus, as long as the condition for rolling without slipping holds, the point (or points) on the rolling object that are momentarily in contact with the surface have zero instantaneous velocity. This means that, even if there was a force acting on the object at that point (such as the force of static friction), it would do no work, since the instantaneous power Fv for a force applied there would always be equal to zero.
We do not actually need the force of static friction to keep an object rolling on a flat surface (as I mentioned above, the motion could in principle go on “unforced” forever), but things are different on an inclined plane. Figure 9.6.2 shows an object rolling down an inclined plane, and the corresponding extended free-body diagram.
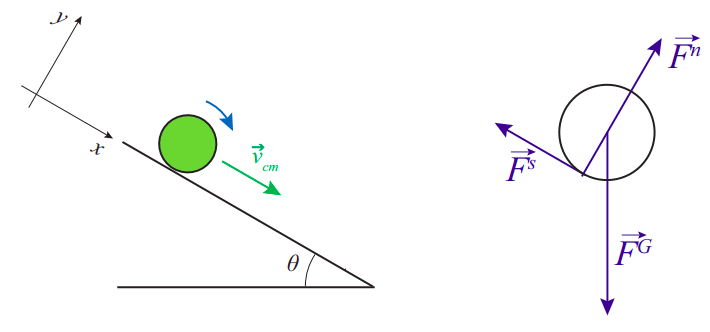
The basic equations we use to solve for the object’s motion are the sum of forces equation:
∑→Fext=M→acm
the net torque equation, with torques taken around the center of mass7
∑→τext=I→α
and the extension of the condition of rolling without slipping, (???), to the accelerations:
For the situation shown in Figure 9.6.2, if we take down the plane as the positive direction for linear motion, and clockwise torques as negative, we have to write acm=−Rα. In the direction perpendicular to the plane, we conclude from (???) that Fn=Mgcosθ, an equation we will not actually need8; in the direction along the plane, we have
Macm=Mgsinθ−Fs
and the torque equation just gives −FsR=Iα, which with acm=−Rα becomes
FsR=IacmR.
We can eliminate Fs in between these two equations and solve for acm:
acm=gsinθ1+I/(MR2).
Now you can see why, earlier in the semester, we were always careful to assume that all the objects we sent down inclined planes were sliding, not rolling! The acceleration for a rolling object is never equal to simply gsinθ. Most remarkably, the correction factor depends only on the shape of the rolling object, and not on its mass or size, since the ratio of I to MR2 is independent of m and R for any given geometry. Thus, for instance, for a disk, I=12MR2, so acm=23gsinθ, whereas for a hoop, I=MR2, so acm=12gsinθ. So any disk or solid cylinder will always roll down the incline faster than any hoop or hollow cylinder, regardless of mass or size.
This rather surprising result may be better understood in terms of energy. First, let me show (a result that is somewhat overdue) that for a rigid object that is rotating around an axis passing through its center of mass with angular velocity ω we can write the total kinetic energy as
K=Kcm+Krot=12Mv2cm+12Iω2.
This is because for every particle the velocity can be written as →v=→vcm+→v′, where →v′ is the velocity relative to the center of mass (that is, in the CM frame). Since in this frame the motion is a simple rotation, we have |v′|=ωr, where r is the particle’s distance to the axis. Therefore, the kinetic energy of that particle will be
12mv2=12→v⋅→v=12m(→vcm+→v′)⋅(→vcm+→v′)=12mv2cm+12mv′2+m→vcm⋅→v′=12mv2cm+12mr2ω2+→vcm⋅→p′
(Note how I have made use of the dot product to calculate the magnitude squared of a vector.) On the last line, the quantity →p′ is the momentum of that particle in the CM frame. Adding those momenta for all the particles should give zero, since, as we saw in an earlier chapter, the center of mass frame is the zero momentum frame. Then, adding the contributions of all particles to the first and second terms in 9.6.9 gives Equation (???).
Coming back to our rolling body, using Equation (???) and the condition of rolling without slipping (???), we see that the ratio of the translational to the rotational kinetic energy is
KcmKrot=mv2cmIω2=mR2I.
The amount of energy available to accelerate the object initially is just the gravitational potential energy of the object-earth system, and that has to be split between translational and rotational in the proportion (???). An object with a proportionately larger I is one that, for a given angular velocity, needs more rotational kinetic energy, because more of its mass is away from the rotation axis. This leaves less energy available for its translational motion.
Resources
Unfortunately, we will not really have enough time this semester to explore further the many interesting effects that follow from the vector nature of Equation (9.4.2), but you are at least subconsciously familiar with some of them if you have ever learned to ride a bicycle! A few interesting Internet references (some of which could perhaps inspire a good Honors project!) are the following:
- Walter Lewin’s lecture on gyroscopic motion (and rolling motion):
https://www.youtube.com/watch?v=N92FYHHT1qM - A “Veritasium” video on “antigravity”:
https://www.youtube.com/watch?v=GeyDf4ooPdo
https://www.youtube.com/watch?v=tLMpdBjA2SU - And the old trick of putting a gyroscope (flywheel) in a suitcase:
https://www.youtube.com/watch?v=zdN6zhZSJKw
If any of the above links are dead, try googling them. (You may want to let me know, too!)
6There is a simple argument, based on Einstein’s theory of relativity, that shows an infinitely rigid object cannot exist: if it did, you could send a signal instantly from one end of it to the other, just by pushing or pulling on your end. In practice, such motion cannot reach the other end faster than the speed of a sound wave (that is to say, a compression wave) in the material. We will study such waves (which imply the medium is not infinitely rigid) in Chapter 12.
7You may feel a little uneasy about the fact that the CM frame is now an accelerated, and therefore non-inertial, frame. How do we know Equation (???) even applies there? This is, indeed, a non-trivial point. However, as we shall see in a later chapter, being in a uniformly-accelerated reference frame is equivalent to being in a uniform gravitational field, and we have just shown that, for all torque-related purposes, one may treat such a field as a single force applied to the center of mass of an object. Such a force (or “pseudoforce” in this case) clearly does not contribute to the torque about the center of mass, and so Equation (???) applies in the CM frame.
8Unless we were trying to answer a question such as “how steep does the plane have to be for rolling without slipping to become impossible?”


