1.1: The Harmonic Oscillator

When you studied mechanics, you probably learned about the harmonic oscillator. We will begin our study of wave phenomena by reviewing this simple but important physical system. Consider a block with mass, m, free to slide on a frictionless air-track, but attached to a \(light^1\) Hooke’s law spring with its other end attached to a fixed wall. A cartoon representation of this physical system is shown in figure 1.1.

\(^1\) "Light" here means that the mass of the spring is small enough to be ignored in the analysis of the motion of the block. We will explain more precisely what this means in chapter 7 when we discuss waves in a massive spring.

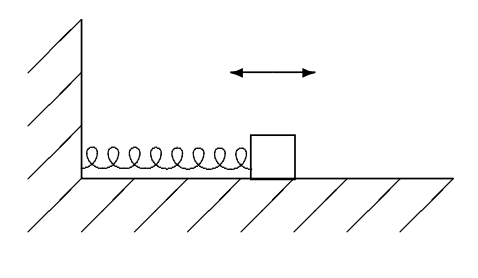
Figure 1.1: A mass on a spring

This system has only one relevant degree of freedom. In general, the number of degrees of freedom of a system is the number of coordinates that must be specified in order to determine the configuration completely. In this case, because the spring is light, we can assume that it is uniformly stretched from the fixed wall to the block. Then the only important coordinate is the position of the block. In this situation, gravity plays no role in the motion of the block. The gravitational force is canceled by a vertical force from the air track. The only relevant force that acts on the block comes from the stretching or compression of the spring. When the spring is relaxed, there is no force on the block and the system is in equilibrium. Hooke’s law tells us that the force from the spring is given by a negative constant, −K, times the displacement of the block from its equilibrium position. Thus if the position of the block at some time is x and its equilibrium position is \(x_0\), then the force on the block at that moment is:

\[F=-K(x-x_0)\]

The constant, K, is called the “spring constant.” It has units of force per unit distance, or \(MT^{−2}\) in terms of M (the unit of mass), L (the unit of length) and T (the unit of time). We can always choose to measure the position, x, of the block with our origin at the equilibrium position. If we do this, then \(x_o = 0\) in (1.1.1) and the force on the block takes the simpler form.

\[F=-Kx\]

Harmonic oscillation results from the interplay between the Hooke’s law force and Newton’s law, \(F = ma\). Let x(t) be the displacement of the block as a function of time, t. Then Newton’s law implies

\[m\frac{d^2}{dt^2}x(t) = -K x(t)\]

An equation of this form, involving not only the function x(t), but also its derivatives is called a “differential equation.” The differential equation, (1.1.3), is the “equation of motion” for the system of figure 1.1. Because the system has only one degree of freedom, there is only one equation of motion. In general, there must be one equation of motion for each independent coordinate required to specify the configuration of the system. The most general solution to the differential equation of motion, (1.1.3), is a sum of a constant times cos ωt plus a constant times sin ωt,

\[x(t) = a cos(ωt) + b sin (ωt)\]

where

\[ω ≡ \sqrt{\frac{K}{m}}\]

is a constant with units of \(T^{-1}\) called the “angular frequency.” The angular frequency will be a very important quantity in our study of wave phenomena. We will almost always denote it by the lower case Greek letter, ω (omega).

Because the equation involves a second time derivative but no higher derivatives, the most general solution involves two constants. This is just what we expect from the physics, because we can get a different solution for each value of the position and velocity of the block at the starting time. Generally, we will think about determining the solution in terms of the position and velocity of the block when we first get the motion started, at a time that we conventionally take to be t=0 For this reason, the process of determining the solution in terms of the position and velocity at a given time is called the “initial value problem.” The values of position and velocity at t = 0 are called initial conditions. For example, we can write the most general solution, (1.1.4), in terms of x(0) and x'(0), the displacement and velocity of the block at time t = 0. Setting t = 0 in (1.1.4) gives a = x(0). Differentiating and then setting t = 0 gives \(b = ω x'(0)\). Thus

\[x(t) = x(0)cosωt +\frac{1}{w}x'(0)sinωt\]

For example, suppose that the block has a mass of 1 kilogram and that the spring is 0.5 meters \(long^2\) with a spring constant K of 100 newtons per meter. To get a sense of what this spring constant means, consider hanging the spring vertically (see problem (1.1.1)). The gravitational force on the block is

\(^2\)(The length of the spring plays no role in the equations below, but we include it to allow you to build a mental picture of the physical system)

\[mg ≈ 9.8 newtons\]

In equilibrium, the gravitational force cancels the force from the spring, thus the spring is stretched by

\[\frac{mg}{K} ≈ 0.098 meters = 9.8 centimeters\]

For this mass and spring constant, the angular frequency, ω, of the system in figure 1.1 is

\[ω = \sqrt{\frac{K}{m}} = \sqrt{\frac{100\frac{N}{m}}{1 kg}} = 10\frac{1}{s}\]

If, for example, the block is displaced by 0.01 m (1 cm) from its equilibrium position and released from rest at time, t = 0, the position at any later time t is given (in meters) by

\[x(t) = 0.01 • cost(10t)\]

The velocity (in meters per second) is

\[x'(t) = -0.1 • sin(10t)\]

The motion is periodic, in the sense that the system oscillates — it repeats the same motion over and over again indefinitely. After a time

\[τ = \frac{2π}{ω} ≈ 0.628 s\]

the system returns exactly to where it was at t = 0, with the block instantaneously at rest with displacement 0.01 meter. The time, τ (Greek letter tau) is called the “period” of the oscillation. However, the solution, (1.1.6), is more than just periodic. It is “simple harmonic” motion, which means that only a single frequency appears in the motion. The angular frequency, ω, is the inverse of the time required for the phase of the wave to change by one radian. The “frequency”, usually denoted by the Greek letter, \(ν\) (nu), is the inverse of the time required for the phase to change by one complete cycle, or \(2π\) radians, and thus get back to its original state. The frequency is measured in hertz, or cycles/second. Thus the angular frequency is larger than the frequency by a factor of \(2π\),

\[ω(in radians/second) = 2π (radians/cycle) • ν (cycles/second)\]

The frequency, \(ν\), is the inverse of the period, τ , of (1.1.12),

\[ν = \frac{1}{τ}\]

Simple harmonic motion like (1.1.6) occurs in a very wide variety of physical systems. The question with which we will start our study of wave phenomena is the following: Why do solutions of the form of (1.1.6) appear so ubiquitously in physics? What do harmonically oscillating systems have in common? Of course, the mathematical answer to this question is that all of these systems have equations of motion of essentially the same form as (1.3). We will find a deeper and more physical answer that we will then be able to generalize to more complicated systems. The key features that all these systems have in common with the mass on the spring are (at least approximate) linearity and time translation invariance of the equations of motion. It is these two features that determine oscillatory behavior in systems from springs to inductors and capacitors. Each of these two properties is interesting on its own, but together, they are much more powerful. They almost completely determine the form of the solutions. We will see that if the system is linear and time translation invariant, we can always write its motion as a sum of simple motions in which the time dependence is either harmonic oscillation or exponential decay (or growth).