10.4: Refraction

Learning Objectives

By the end of this section, you will be able to:

• Describe how rays change direction upon entering a medium
• Apply the law of refraction in problem solving
• Explain the phenomenon of total internal reflection
• Describe the workings and uses of optical fibers
• Analyze the reason for the sparkle of diamonds
• Explain the cause of dispersion in a prism
• Describe the effects of dispersion in producing rainbows
• Summarize the advantages and disadvantages of dispersion

You may often notice some odd things when looking into a fish tank. For example, you may see the same fish appearing to be in two different places (Figure \(\PageIndex{1}\)). This happens because light coming from the fish to you changes direction when it leaves the tank, and in this case, it can travel two different paths to get to your eyes. The changing of a light ray’s direction (loosely called bending) when it passes through substances of different refractive indices is called refraction and is related to changes in the speed of light, \(v=c/n\). Refraction is responsible for a tremendous range of optical phenomena, from the action of lenses to data transmission through optical fibers.

Figure \(\PageIndex{1}\): (a) Looking at the fish tank as shown, we can see the same fish in two different locations, because light changes directions when it passes from water to air. In this case, the light can reach the observer by two different paths, so the fish seems to be in two different places. This bending of light is called refraction and is responsible for many optical phenomena. (b) This image shows refraction of light from a fish near the top of a fish tank.

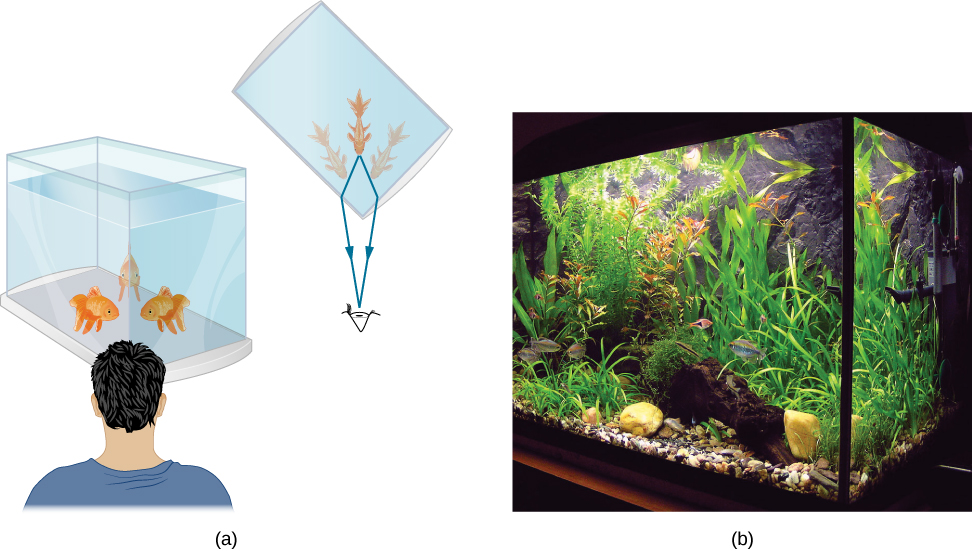
Figure \(\PageIndex{2}\) shows how a ray of light changes direction when it passes from one medium to another. As before, the angles are measured relative to a perpendicular to the surface at the point where the light ray crosses it. (Some of the incident light is reflected from the surface, but for now we concentrate on the light that is transmitted.) The change in direction of the light ray depends on the relative values of the indices of refraction of the two media involved. In the situations shown, medium 2 has a greater index of refraction than medium 1. Note that as shown in Figure \(\PageIndex{1a}\), the direction of the ray moves closer to the perpendicular when it progresses from a medium with a lower index of refraction to one with a higher index of refraction. Conversely, as shown in Figure \(\PageIndex{1b}\), the direction of the ray moves away from the perpendicular when it progresses from a medium with a higher index of refraction to one with a lower index of refraction. The path is exactly reversible.

Figure \(\PageIndex{2}\): The change in direction of a light ray depends on how the index of refraction changes when it crosses from one medium to another. In the situations shown here, the index of refraction is greater in medium 2 than in medium 1. (a) A ray of light moves closer to the perpendicular when entering a medium with a higher index of refraction. (b) A ray of light moves away from the perpendicular when entering a medium with a lower index of refraction.

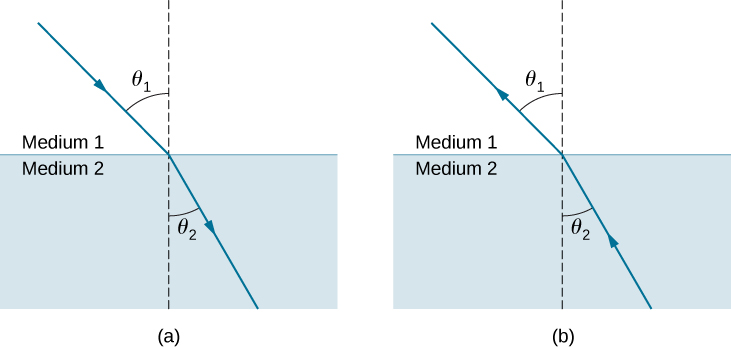
The amount that a light ray changes its direction depends both on the incident angle and the amount that the speed changes. For a ray at a given incident angle, a large change in speed causes a large change in direction and thus a large change in angle. The exact mathematical relationship is the law of refraction, or Snell’s law, after the Dutch mathematician Willebrord Snell (1591–1626), who discovered it in 1621. The law of refraction is stated in equation form as

\[n_1 \, \sin \, θ_1=n_2 \, \sin \, θ_2. \label{snell's law} \]

Here (n_1\) and \(n_2\) are the indices of refraction for media 1 and 2, and \(θ_1\) and \(θ_2\) are the angles between the rays and the perpendicular in media 1 and 2. The incoming ray is called the incident ray, the outgoing ray is called the refracted ray, and the associated angles are the incident angle and the refracted angle, respectively.

Snell’s experiments showed that the law of refraction is obeyed and that a characteristic index of refraction \(n\) could be assigned to a given medium and its value measured. Snell was not aware that the speed of light varied in different media, a key fact used when we derive the law of refraction theoretically using Huygens’s Principle.

Example \(\PageIndex{1}\): Determining the Index of Refraction

Find the index of refraction for medium 2 in Figure \(\PageIndex{1a}\), assuming medium 1 is air and given that the incident angle is 30.0° and the angle of refraction is 22.0°.

Strategy

The index of refraction for air is taken to be 1 in most cases (and up to four significant figures, it is 1.000). Thus, \(n_1=1.00\) here. From the given information, \(θ_1=30.0°\) and \(θ_2=22.0°\). With this information, the only unknown in Snell’s law is \(n_2\), so we can use Snell’s law (Equation \ref{snell's law}) to find it.

Solution

From Snell’s law (Equation \ref{snell's law}), we have

\[\begin{align*} n_1\sin θ_1 &=n_2 \sin θ_2 \\[4pt] n_2 &= n_1\dfrac{\sin θ_1}{\sin θ_2}. \end{align*} \nonumber \]

Entering known values,

\[\begin{align*} n_2 &=1.00 \dfrac{\sin 30.0°}{\sin 22.0°} \\[4pt] &= \dfrac{0.500}{0.375} \\[4pt] &=1.33. \end{align*} \nonumber \]

Significance

This is the index of refraction for water, and Snell could have determined it by measuring the angles and performing this calculation. He would then have found 1.33 to be the appropriate index of refraction for water in all other situations, such as when a ray passes from water to glass. Today, we can verify that the index of refraction is related to the speed of light in a medium by measuring that speed directly.

Explore bending of light between two media with different indices of refraction. Use the “Intro” simulation and see how changing from air to water to glass changes the bending angle. Use the protractor tool to measure the angles and see if you can recreate the configuration in Example \(\PageIndex{1}\). Also by measurement, confirm that the angle of reflection equals the angle of incidence.

Example \(\PageIndex{2}\): A Larger Change in Direction

Suppose that in a situation like that in Example \(\PageIndex{1}\), light goes from air to diamond and that the incident angle is 30.0°. Calculate the angle of refraction θ₂ in the diamond.

Strategy

Again, the index of refraction for air is taken to be n₁=1.00, and we are given θ₁=30.0°. We can look up the index of refraction for diamond, finding n₂=2.419. The only unknown in Snell’s law is \(θ_2\), which we wish to determine.

Solution

Solving Snell’s law (Equation \ref{snell's law}) for \(\sin θ_2\) yields

\[\sin θ_2=\frac{n_1}{n_2}\sin θ_1. \nonumber \]

Entering known values,

\[\sin θ_2=\frac{1.00}{2.419}\sin30.0°=(0.413)(0.500)=0.207. \nonumber \]

The angle is thus

\[θ_2=\sin^{−1}(0.207)=11.9°. \nonumber \]

Significance

For the same 30.0° angle of incidence, the angle of refraction in diamond is significantly smaller than in water (11.9° rather than 22.0°—see Example \(\PageIndex{2}\)). This means there is a larger change in direction in diamond. The cause of a large change in direction is a large change in the index of refraction (or speed). In general, the larger the change in speed, the greater the effect on the direction of the ray.

Exercise \(\PageIndex{1}\): Zircon

The solid with the next highest index of refraction after diamond is zircon. If the diamond in Example \(\PageIndex{2}\) were replaced with a piece of zircon, what would be the new angle of refraction?

Answer

15.1°

Total Internal Reflection

A good-quality mirror may reflect more than 90% of the light that falls on it, absorbing the rest. But it would be useful to have a mirror that reflects all of the light that falls on it. Interestingly, we can produce total reflection using an aspect of refraction.

Consider what happens when a ray of light strikes the surface between two materials, as shown in Figure \(\PageIndex{1a}\). Part of the light crosses the boundary and is refracted; the rest is reflected. If, as shown in the figure, the index of refraction for the second medium is less than for the first, the ray bends away from the perpendicular. (Since \(n_1>n_2\), the angle of refraction is greater than the angle of incidence—that is, \(θ_1>θ_2\).) Now imagine what happens as the incident angle increases. This causes \(θ_2\) to increase also. The largest the angle of refraction \(θ_2\) can be is \(90°\), as shown in Figure \(\PageIndex{1b}\).

Figure \(\PageIndex{1}\): (a) A ray of light crosses a boundary where the index of refraction decreases. That is, \(n_2<n_1\). The ray bends away from the perpendicular. (b) The critical angle θ_c is the angle of incidence for which the angle of refraction is 90°. (c) Total internal reflection occurs when the incident angle is greater than the critical angle.

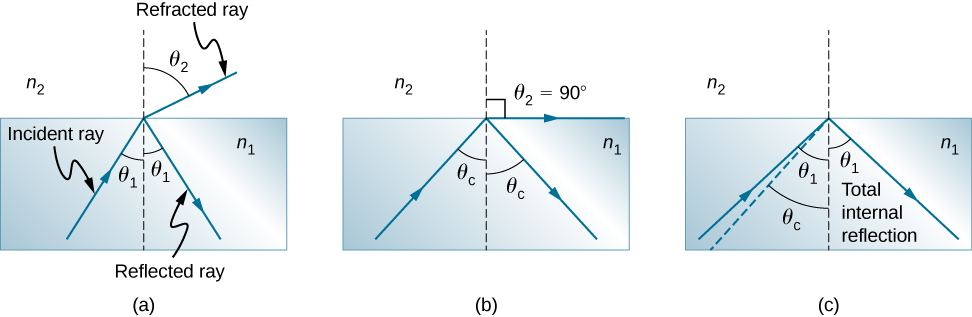
The critical angle \(θ_c\) for a combination of materials is defined to be the incident angle \(θ_1\) that produces an angle of refraction of \(90°\). That is, \(θ_c\) is the incident angle for which \(θ_2=90°\). If the incident angle \(θ_1\) is greater than the critical angle, as shown in Figure \(\PageIndex{1c}\), then all of the light is reflected back into medium 1, a condition called total internal reflection. (As Figure \(\PageIndex{1}\) shows, the reflected rays obey the law of reflection so that the angle of reflection is equal to the angle of incidence in all three cases.)

Snell’s law states the relationship between angles and indices of refraction. It is given by

\[n_1\sin θ_1=n_2 \sin θ_2. \nonumber \]

When the incident angle equals the critical angle (\(θ_1=θ_c\)), the angle of refraction is \(90°\) (\(θ_2=90°\)). Noting that \(\sin 90°=1\), Snell’s law in this case becomes

\[n_1 \, \sin \, θ_1 = n_2. \nonumber \]

The critical angle \(θ_c\) for a given combination of materials is thus

\[ θ_c = \sin^{−1}\left(\frac{n_2}{n_1}\right)\label{critical} \]

for \(n_1>n_2\).

Total internal reflection occurs for any incident angle greater than the critical angle \(θ_c\), and it can only occur when the second medium has an index of refraction less than the first. Note that this equation is written for a light ray that travels in medium 1 and reflects from medium 2, as shown in Figure \(\PageIndex{1}\).

Example \(\PageIndex{1}\): Determining a Critical Angle

What is the critical angle for light traveling in a polystyrene (a type of plastic) pipe surrounded by air? The index of refraction for polystyrene is 1.49.

Strategy

The index of refraction of air can be taken to be 1.00, as before. Thus, the condition that the second medium (air) has an index of refraction less than the first (plastic) is satisfied, and we can use the equation

\[θ_c=\sin^{−1}\left(\frac{n_2}{n_1}\right) \nonumber \]

to find the critical angle \(θ_c\), where \(n_2=1.00\) and \(n_1=1.49\).

Solution

Substituting the identified values gives

\[\begin{align} θ_c &= \sin^{−1}\left(\frac{1.00}{1.49}\right) \nonumber \\[4pt] &= \sin^{−1}(0.671) \nonumber \\[4pt] &= 42.2°. \nonumber \end{align} \nonumber \]

Significance

This result means that any ray of light inside the plastic that strikes the surface at an angle greater than 42.2° is totally reflected. This makes the inside surface of the clear plastic a perfect mirror for such rays, without any need for the silvering used on common mirrors. Different combinations of materials have different critical angles, but any combination with \(n_1>n_2\) can produce total internal reflection. The same calculation as made here shows that the critical angle for a ray going from water to air is 48.6°, whereas that from diamond to air is 24.4°, and that from flint glass to crown glass is 66.3°.

Exercise \(\PageIndex{1}\)

At the surface between air and water, light rays can go from air to water and from water to air. For which ray is there no possibility of total internal reflection?

Answer

air to water, because the condition that the second medium must have a smaller index of refraction is not satisfied

In the photo that opens this chapter, the image of a swimmer underwater is captured by a camera that is also underwater. The swimmer in the upper half of the photograph, apparently facing upward, is, in fact, a reflected image of the swimmer below. The circular ripple near the photograph’s center is actually on the water surface. The undisturbed water surrounding it makes a good reflecting surface when viewed from below, thanks to total internal reflection. However, at the very top edge of this photograph, rays from below strike the surface with incident angles less than the critical angle, allowing the camera to capture a view of activities on the pool deck above water.

Fiber Optics: Endoscopes to Telephones

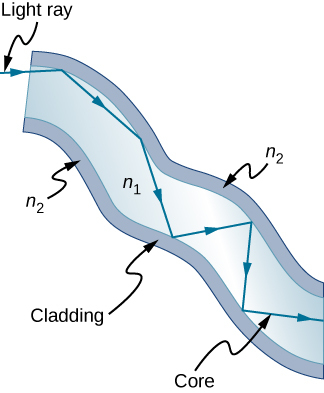
Fiber optics is one application of total internal reflection that is in wide use. In communications, it is used to transmit telephone, internet, and cable TV signals. Fiber optics employs the transmission of light down fibers of plastic or glass. Because the fibers are thin, light entering one is likely to strike the inside surface at an angle greater than the critical angle and, thus, be totally reflected (Figure \(\PageIndex{2}\)). The index of refraction outside the fiber must be smaller than inside. In fact, most fibers have a varying refractive index to allow more light to be guided along the fiber through total internal refraction. Rays are reflected around corners as shown, making the fibers into tiny light pipes.

Figure \(\PageIndex{2}\): Light entering a thin optic fiber may strike the inside surface at large or grazing angles and is completely reflected if these angles exceed the critical angle. Such rays continue down the fiber, even following it around corners, since the angles of reflection and incidence remain large.

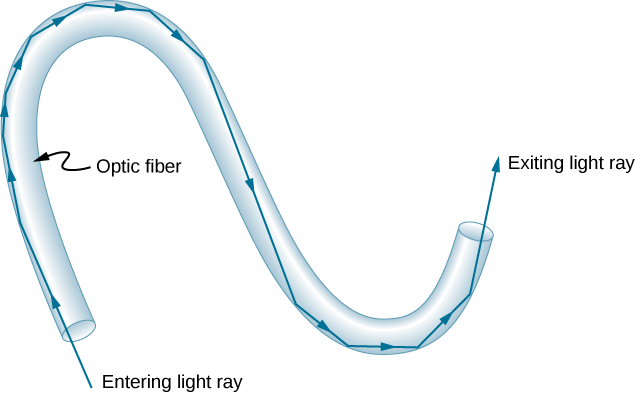
Bundles of fibers can be used to transmit an image without a lens, as illustrated in Figure \(\PageIndex{3}\). The output of a device called an endoscope is shown in Figure \(\PageIndex{1b}\). Endoscopes are used to explore the interior of the body through its natural orifices or minor incisions. Light is transmitted down one fiber bundle to illuminate internal parts, and the reflected light is transmitted back out through another bundle to be observed.

Figure \(\PageIndex{3}\): (a) An image “A” is transmitted by a bundle of optical fibers. (b) An endoscope is used to probe the body, both transmitting light to the interior and returning an image such as the one shown of a human epiglottis (a structure at the base of the tongue). (credit b: modification of work by “Med_Chaos”/Wikimedia Commons)

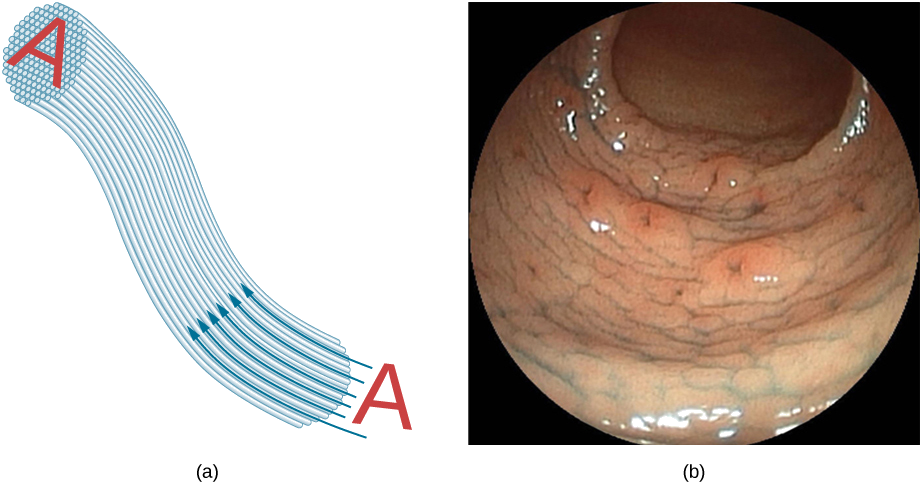
Fiber optics has revolutionized surgical techniques and observations within the body, with a host of medical diagnostic and therapeutic uses. Surgery can be performed, such as arthroscopic surgery on a knee or shoulder joint, employing cutting tools attached to and observed with the endoscope. Samples can also be obtained, such as by lassoing an intestinal polyp for external examination. The flexibility of the fiber optic bundle allows doctors to navigate it around small and difficult-to-reach regions in the body, such as the intestines, the heart, blood vessels, and joints. Transmission of an intense laser beam to burn away obstructing plaques in major arteries, as well as delivering light to activate chemotherapy drugs, are becoming commonplace. Optical fibers have in fact enabled microsurgery and remote surgery where the incisions are small and the surgeon’s fingers do not need to touch the diseased tissue.

Optical fibers in bundles are surrounded by a cladding material that has a lower index of refraction than the core (Figure \(\PageIndex{4}\)). The cladding prevents light from being transmitted between fibers in a bundle. Without cladding, light could pass between fibers in contact, since their indices of refraction are identical. Since no light gets into the cladding (there is total internal reflection back into the core), none can be transmitted between clad fibers that are in contact with one another. Instead, the light is propagated along the length of the fiber, minimizing the loss of signal and ensuring that a quality image is formed at the other end. The cladding and an additional protective layer make optical fibers durable as well as flexible.

Figure \(\PageIndex{4}\): Fibers in bundles are clad by a material that has a lower index of refraction than the core to ensure total internal reflection, even when fibers are in contact with one another.

Special tiny lenses that can be attached to the ends of bundles of fibers have been designed and fabricated. Light emerging from a fiber bundle can be focused through such a lens, imaging a tiny spot. In some cases, the spot can be scanned, allowing quality imaging of a region inside the body. Special minute optical filters inserted at the end of the fiber bundle have the capacity to image the interior of organs located tens of microns below the surface without cutting the surface—an area known as nonintrusive diagnostics. This is particularly useful for determining the extent of cancers in the stomach and bowel.

In another type of application, optical fibers are commonly used to carry signals for telephone conversations and internet communications. Extensive optical fiber cables have been placed on the ocean floor and underground to enable optical communications. Optical fiber communication systems offer several advantages over electrical (copper)-based systems, particularly for long distances. The fibers can be made so transparent that light can travel many kilometers before it becomes dim enough to require amplification—much superior to copper conductors. This property of optical fibers is called low loss. Lasers emit light with characteristics that allow far more conversations in one fiber than are possible with electric signals on a single conductor. This property of optical fibers is called high bandwidth. Optical signals in one fiber do not produce undesirable effects in other adjacent fibers. This property of optical fibers is called reduced crosstalk. We shall explore the unique characteristics of laser radiation in a later chapter.

Corner Reflectors and Diamonds

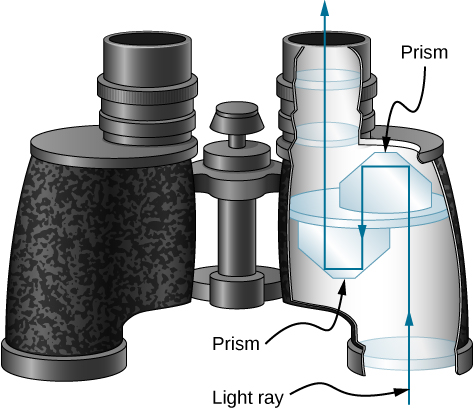
Corner reflectors are perfectly efficient when the conditions for total internal reflection are satisfied. With common materials, it is easy to obtain a critical angle that is less than 45°. One use of these perfect mirrors is in binoculars, as shown in Figure \(\PageIndex{5}\). Another use is in periscopes found in submarines.

Figure \(\PageIndex{5}\): These binoculars employ corner reflectors (prisms) with total internal reflection to get light to the observer’s eyes.

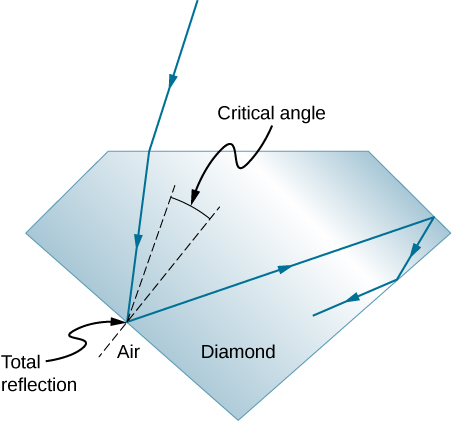
Total internal reflection, coupled with a large index of refraction, explains why diamonds sparkle more than other materials. The critical angle for a diamond-to-air surface is only 24.4°, so when light enters a diamond, it has trouble getting back out (Figure \(\PageIndex{6}\)). Although light freely enters the diamond, it can exit only if it makes an angle less than 24.4°. Facets on diamonds are specifically intended to make this unlikely. Good diamonds are very clear, so that the light makes many internal reflections and is concentrated before exiting—hence the bright sparkle. (Zircon is a natural gemstone that has an exceptionally large index of refraction, but it is not as large as diamond, so it is not as highly prized. Cubic zirconia is manufactured and has an even higher index of refraction (≈2.17), but it is still less than that of diamond.) The colors you see emerging from a clear diamond are not due to the diamond’s color, which is usually nearly colorless, but result from dispersion. Colored diamonds get their color from structural defects of the crystal lattice and the inclusion of minute quantities of graphite and other materials. The Argyle Mine in Western Australia produces around 90% of the world’s pink, red, champagne, and cognac diamonds, whereas around 50% of the world’s clear diamonds come from central and southern Africa.

Figure \(\PageIndex{6}\): Light cannot easily escape a diamond, because its critical angle with air is so small. Most reflections are total, and the facets are placed so that light can exit only in particular ways—thus concentrating the light and making the diamond sparkle brightly.

Dispersion

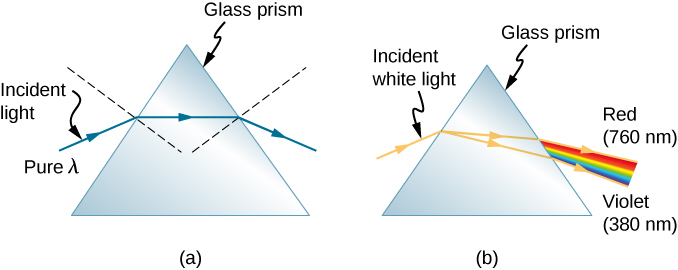
Everyone enjoys the spectacle of a rainbow glimmering against a dark stormy sky. How does sunlight falling on clear drops of rain get broken into the rainbow of colors we see? The same process causes white light to be broken into colors by a clear glass prism or a diamond (Figure \(\PageIndex{1}\)).

Figure \(\PageIndex{1}\): The colors of the rainbow (a) and those produced by a prism (b) are identical. (credit a: modification of work by “Alfredo55”/Wikimedia Commons; credit b: modification of work by NASA)

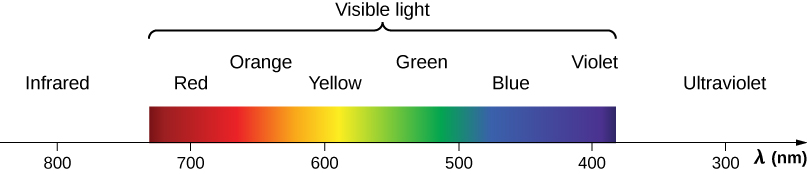
We see about six colors in a rainbow—red, orange, yellow, green, blue, and violet; sometimes indigo is listed, too. These colors are associated with different wavelengths of light, as shown in Figure \(\PageIndex{2}\). When our eye receives pure-wavelength light, we tend to see only one of the six colors, depending on wavelength. The thousands of other hues we can sense in other situations are our eye’s response to various mixtures of wavelengths. White light, in particular, is a fairly uniform mixture of all visible wavelengths. Sunlight, considered to be white, actually appears to be a bit yellow, because of its mixture of wavelengths, but it does contain all visible wavelengths. The sequence of colors in rainbows is the same sequence as the colors shown in the figure. This implies that white light is spread out in a rainbow according to wavelength. Dispersion is defined as the spreading of white light into its full spectrum of wavelengths. More technically, dispersion occurs whenever the propagation of light depends on wavelength.

Figure \(\PageIndex{2}\): Even though rainbows are associated with six colors, the rainbow is a continuous distribution of colors according to wavelengths.

Any type of wave can exhibit dispersion. For example, sound waves, all types of electromagnetic waves, and water waves can be dispersed according to wavelength. Dispersion may require special circumstances and can result in spectacular displays such as in the production of a rainbow. This is also true for sound, since all frequencies ordinarily travel at the same speed. If you listen to sound through a long tube, such as a vacuum cleaner hose, you can easily hear it dispersed by interaction with the tube. Dispersion, in fact, can reveal a great deal about what the wave has encountered that disperses its wavelengths. The dispersion of electromagnetic radiation from outer space, for example, has revealed much about what exists between the stars—the so-called interstellar medium.

Nick Moore’s video discusses dispersion of a pulse as he taps a long spring. Follow his explanation as Moore replays the high-speed footage showing high frequency waves outrunning the lower frequency waves. https://www.youtube.com/watch?v=KbmOcT5sX7I

Refraction is responsible for dispersion in rainbows and many other situations. The angle of refraction depends on the index of refraction, as we know from Snell’s law. We know that the index of refraction n depends on the medium. But for a given medium, n also depends on wavelength (Table \(\PageIndex{1}\)).

Table \(\PageIndex{1}\): Index of Refraction (\(n\)) in Selected Media at Various Wavelengths

Medium	Red (660 nm)	Orange (610 nm)	Yellow (580 nm)	Green (550 nm)	Blue (470 nm)	Violet (410 nm)
Water	1.331	1.332	1.333	1.335	1.338	1.342
Diamond	2.410	2.415	2.417	2.426	2.444	2.458
Glass, crown	1.512	1.514	1.518	1.519	1.524	1.530
Glass, flint	1.662	1.665	1.667	1.674	1.684	1.698
Polystyrene	1.488	1.490	1.492	1.493	1.499	1.506
Quartz, fused	1.455	1.456	1.458	1.459	1.462	1.468

Note that for a given medium, n increases as wavelength decreases and is greatest for violet light. Thus, violet light is bent more than red light, as shown for a prism in Figure \(\PageIndex{3b}\). White light is dispersed into the same sequence of wavelengths as seen in Figures \(\PageIndex{1}\) and \(\PageIndex{2}\).

Figure \(\PageIndex{3}\): (a) A pure wavelength of light falls onto a prism and is refracted at both surfaces. (b) White light is dispersed by the prism (shown exaggerated). Since the index of refraction varies with wavelength, the angles of refraction vary with wavelength. A sequence of red to violet is produced, because the index of refraction increases steadily with decreasing wavelength.

Example \(\PageIndex{1}\): Dispersion of White Light by Flint Glass

A beam of white light goes from air into flint glass at an incidence angle of 43.2°. What is the angle between the red (660 nm) and violet (410 nm) parts of the refracted light?

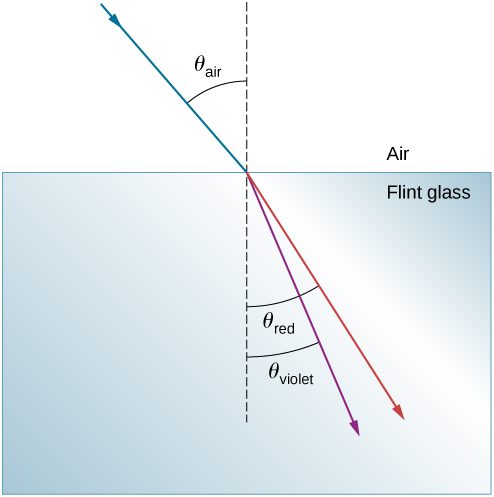
Strategy

Values for the indices of refraction for flint glass at various wavelengths are listed in Table \(\PageIndex{1}\). Use these values for calculate the angle of refraction for each color and then take the difference to find the dispersion angle.

Solution

Applying the law of refraction for the red part of the beam

\[n_{air}\sin θ_{air}=n_{red} \sinθ_{red}, \nonumber \]

we can solve for the angle of refraction as

\[θ_{red}=\sin^{−1}(\frac{n_{air}\sin θ_{air}}{n_{red}})=\sin^{−1}[\frac{(1.000)\sin43.2°}{(1.512)}]=27.0°. \nonumber \]

Similarly, the angle of incidence for the violet part of the beam is

\[θ_{violet}=\sin^{−1}(\frac{n_{air}sinθ_{air}}{n_{violet}})=\sin^{−1}[\frac{(1.000)\sin43.2°}{(1.530)}]=26.4°. \nonumber \]

The difference between these two angles is

\[θ_{red}−θ_{violet}=27.0°−26.4°=0.6°. \nonumber \]

Significance

Although 0.6° may seem like a negligibly small angle, if this beam is allowed to propagate a long enough distance, the dispersion of colors becomes quite noticeable.

Exercise \(\PageIndex{1}\)

In the preceding example, how much distance inside the block of flint glass would the red and the violet rays have to progress before they are separated by 1.0 mm?

Answer

9.3 cm

Rainbows are produced by a combination of refraction and reflection. You may have noticed that you see a rainbow only when you look away from the Sun. Light enters a drop of water and is reflected from the back of the drop (Figure \(\PageIndex{4}\)).

Figure \(\PageIndex{4}\): A ray of light falling on this water drop enters and is reflected from the back of the drop. This light is refracted and dispersed both as it enters and as it leaves the drop.

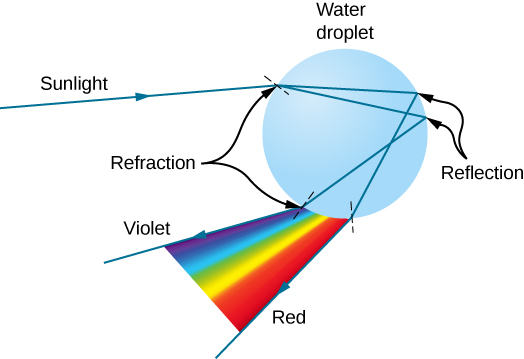
The light is refracted both as it enters and as it leaves the drop. Since the index of refraction of water varies with wavelength, the light is dispersed, and a rainbow is observed (Figure \(\PageIndex{4a}\)). (No dispersion occurs at the back surface, because the law of reflection does not depend on wavelength.) The actual rainbow of colors seen by an observer depends on the myriad rays being refracted and reflected toward the observer’s eyes from numerous drops of water. The effect is most spectacular when the background is dark, as in stormy weather, but can also be observed in waterfalls and lawn sprinklers. The arc of a rainbow comes from the need to be looking at a specific angle relative to the direction of the Sun, as illustrated in Figure \(\PageIndex{4b}\). If two reflections of light occur within the water drop, another “secondary” rainbow is produced. This rare event produces an arc that lies above the primary rainbow arc, as in Figure \(\PageIndex{4c}\), and produces colors in the reverse order of the primary rainbow, with red at the lowest angle and violet at the largest angle.

Figure \(\PageIndex{5}\): (a) Different colors emerge in different directions, and so you must look at different locations to see the various colors of a rainbow. (b) The arc of a rainbow results from the fact that a line between the observer and any point on the arc must make the correct angle with the parallel rays of sunlight for the observer to receive the refracted rays. (c) Double rainbow. (credit c: modification of work by “Nicholas”/Wikimedia Commons)

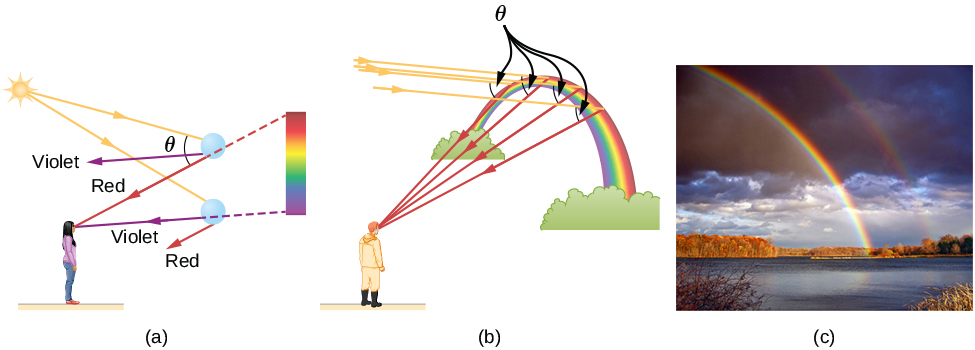
Dispersion may produce beautiful rainbows, but it can cause problems in optical systems. White light used to transmit messages in a fiber is dispersed, spreading out in time and eventually overlapping with other messages. Since a laser produces a nearly pure wavelength, its light experiences little dispersion, an advantage over white light for transmission of information. In contrast, dispersion of electromagnetic waves coming to us from outer space can be used to determine the amount of matter they pass through.