2.1: Where Are We Headed?
- Page ID
- 2057
This page is a draft and is under active development.
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)This is the second chapter that deals directly with the Energy-Interaction Model. We add all kinds of mechanical interactions to the thermal interactions we treated in Chapter 1. (Note: the term “mechanical” as in the phase “mechanical interactions” is typically used to imply everything other than thermal. Since the Energy-Interaction Model literally applies to every kind of interaction that scientists have ever encountered, we will be just scratching the surface of the realm of applications of this powerful model. We will, however, devote some attention to one area of application that occurs frequently in many phenomena–all kinds of things vibrate, from atoms and molecules to bridges and skyscrapers; that is, they move back and forth or oscillate in very predictable ways.
A fundamental understanding of vibrational (or oscillatory as it is often called) motion is very useful. Therefore, we introduce the Intro Spring-Mass Oscillator Model in this chapter as an application of the Energy-Interaction Model. The Intro Spring-Mass Oscillator Model will also play an important role in Chapter 3 when we develop particle models of matter.
Overview
In Chapter 2 we continue our focus on interacting systems. We intentionally focus on the systems as they exist before the interaction and then immediately following the interaction; we stay away from the details of what happens during the interaction itself. This is a very general and a very powerful approach. We saw in Chapter 1 that energy transfers are fundamentally related to interactions.
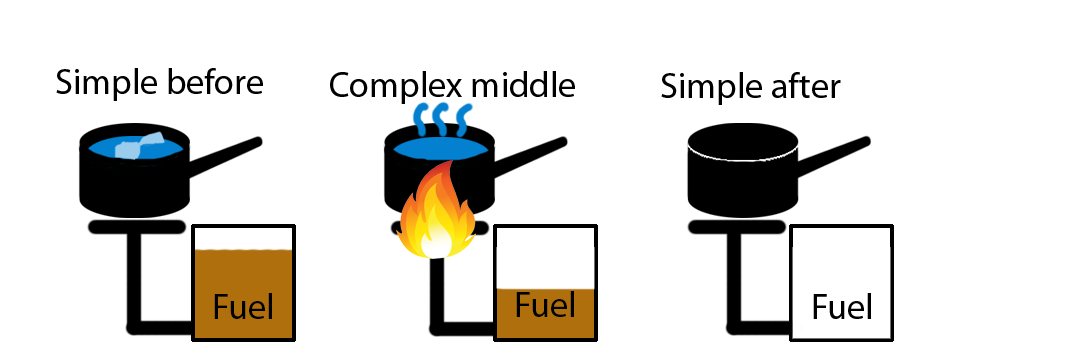
When one physical system interacts with another, or when parts of the same physical system interact, we were able to identify energy-systems that either increased or decreased in energy. If the physical system is closed, i.e., we have included all of the energy systems that change during the interaction, then energy conservation tells us that the total of all the increases equals the total of all the decreases. If the physical system is open, then the net change in all of the energy-systems equals the net energy added to the physical system from outside that physical system. We continue this approach in Chapter 2 with non-thermal energy-systems. In discussion/lab activities, you will identify several kinds of energy-systems and the transfers that occur among them. A surprising result for many of us is that when we consider typical activities such as driving a car or riding a bike, most of the energies involved ultimately end up being transferred to various thermal systems. Thermal energy systems seem to have a way of “grabbing and hanging on to” most of the energy. (This tendency has to do with the vast number of particles involved in thermal systems. We explicitly discuss this in Chapter 4.)
Thermal energies are associated with the random or disordered motions of the particles making up matter. In this chapter we turn our attention to energy-systems in which the common motion all of the particles making up the matter is important. We can often describe this common motion with just two variables: a position variable (x) and a speed variable (v). These energies (ones that can be described by position and speed of the entire object) are commonly called mechanical energies.
As you begin the activities of this chapter in your discussion/lab, you might be tempted to ask, “How many kinds of energy can there be?” The answer is simple and reassuring: there are only two fundamental kinds: these are energies that depend on the square of the speed of a particle or object (kinetic energy, abbreviated KE) and energies that depend on the positions or configurations of particles or objects (potential energy, abbreviated PE). All energies, no matter what names we give them, are either a kinetic energy or a potential energy or some combination of the two. This is true for the two energies we have discussed up to this point: bond energy and thermal energy. Bond energy depends on the positions of the atoms making up the molecules, so it is a potential energy. Thermal energy is a combination of the kinetic energies of the individual atoms and the potential energies associated with the motions of the atoms about their equilibrium positions. We will discuss these relations in much greater detail in Chapter 3.
Where are we going with this energy stuff and why? You will begin to see as you carry out the activities and work on the assignments in this chapter that using an Energy-Interaction Model allows us to answer many interesting questions about sports, bikes, objects falling off buildings, and other common (or not so common) everyday activities. Mechanical energies involve position and speed variables, and because transfers of mechanical energy involve work (instead of heat), we need to understand a little more about work. Work involves the notion of forces acting through distances, which means we will have to know a little more about force itself. The payoff is that we will be able to calculate or predict many unknown distances, speeds, and forces without ever having to know the details of the interactions. And the beauty of this approach is that it works for all kinds of physical situations. We don’t have to learn lots of different ways of approaching questions that depend on the particulars of the situation!
Another significant benefit of starting the way we did in Chapter 1 with thermal phenomena is that we can also look much more realistically at real-world phenomena where friction is always present. If you look at a conventional introductory physics text, you will find that for the first third of the book, it seems everyone just pretends that there is no friction! It is as if we lived in a distant galaxy where friction didn’t exist. But that is a pretty idealized galaxy. Because we are treating energy within a general model that works for mechanical energies as well as thermal energies, rather than from a purely mechanics approach, we can deal directly with the transfers of energy to thermal systems and treat a lot of phenomena much more realistically than we could if we narrowly focused on the mechanics of frictionless systems.


