5.8.6: Rods
- Page ID
- 8150
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Refer to figure \(\text{V.5}\). The potential at \(\text{P}\) due to the element \(δx\) is \(-\frac{Gλδx}{r} = − Gλ \sec θδθ\). The total potential at \(\text{P}\) is therefore
\[ψ = - G λ \int_α^β \sec θdθ = - Gλ \ln \left[ \frac{\sec β + \tan β}{\sec α + \tan α} \right] . \label{5.8.15} \tag{5.8.15}\]
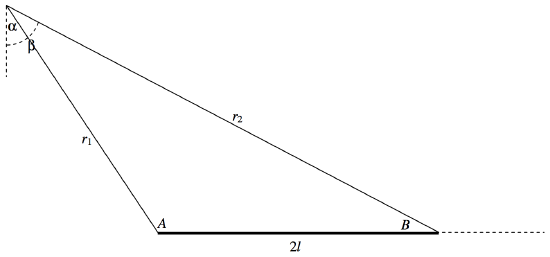
\(\text{FIGURE V.24}\)
Refer now to figure \(\text{V.24}\), in which \(A = 90^\circ + α\) and \(B = 90^\circ − β\).
\[\frac{\sec β + \tan β}{\sec α + \tan α} = \frac{\cos α (1+ \sin β)}{\cos β (1 + \sin α)} = \frac{\sin A (1+ \cos B)}{\sin B(1-\cos A)} = \frac{2 \sin \frac{1}{2}A \cos \frac{1}{2} A . 2 \cos^2 \frac{1}{2} B}{2\sin \frac{1}{2} B \cos \frac{1}{2} B . 2 \sin^2 \frac{1}{2} A} = \cot \frac{1}{2} A \cot \frac{1}{2} B = \sqrt{\frac{s(s-r_2)}{(s-r_1)(s-2l)}}\cdot \sqrt{\frac{s(s-r_1)}{(s-2l)(s-r_2)}},\]
where \(s = \frac{1}{2} (r_1 + r_2 + 2l)\). (You may want to refer here to the formulas on pp. 37 and 38 of Chapter 2.)
Hence \[ψ = - Gλ \ln \left[ \frac{r_1 + r_2 + 2l}{r_1 + r_2 -2l} \right]. \label{5.8.16} \tag{5.8.16}\]
If \(r_1\) and \(r_2\) are very large compared with \(l\), they are nearly equal, so let’s put \(r_1 + r_2 = 2r\) and write Equation 5.8.17 as
\[ψ = -\frac{Gm}{2l} \ln \left[ \frac{2r \left( 1 + \frac{2l}{2r} \right)}{2r \left( 1 - \frac{2l}{2r} \right)} \right] = -\frac{Gm}{2l} \left[ \ln \left(1 + \frac{l}{r} \right) - \ln \left( 1 - \frac{l}{r} \right) \right] .\]
Maclaurin expand the logarithms, and you will see that, at large distances from the rod, the potential is, expected, \(−Gm/r\).
Let us return to the near vicinity of the rod and to Equation \(\ref{5.8.16}\). We see that if we move around the rod in such a manner that we keep \(r_1 + r_2\) constant and equal to \(2a\), say − that is to say if we move around the rod in an ellipse (see our definition of an ellipse in Chapter 2, Section 2.3) − the potential is constant. In other words the equipotentials are confocal ellipses, with the foci at the ends of the rod. Equation \(\ref{5.8.16}\) can be written
\[ψ = - Gλ \ln \left( \frac{a+l}{a-l} \right) . \label{5.8.17} \tag{5.8.17}\]
For a given potential \(ψ\), the equipotential is an ellipse of major axis
\[2a = 2l \left( \frac{e^{ψ/(Gλ)}+1}{e^{ψ/(Gλ)}-1} \right), \label{5.8.20} \tag{5.8.20}\]
where \(2l\) is the length of the rod. This knowledge is useful if you are exploring space and you encounter an alien spacecraft or an asteroid in the form of a uniform rod of length \(2l\).