5.12: Gravitational Potential of any Massive Body

You might just want to look at Chapter 2 of Classical Mechanics (Moments of Inertia) before proceeding further with this chapter.

In figure \(\text{VIII.26}\) I draw a massive body whose centre of mass is \(\text{C}\), and an external point \(\text{P}\) at a distance \(R\) from \(\text{C}\). I draw a set of \(\text{C}xyz\) axes, such that \(\text{P}\) is on the \(z\)-axis, the coordinates of \(\text{P}\) being \((0, 0, z)\). I indicate an element \(δm\) of mass, distant \(r\) from \(\text{C}\) and \(l\) from \(\text{P}\). I’ll suppose that the density at \(δm\) is \(ρ\) and the volume of the mass element is \(δτ\), so that \(δm = ρδτ\).

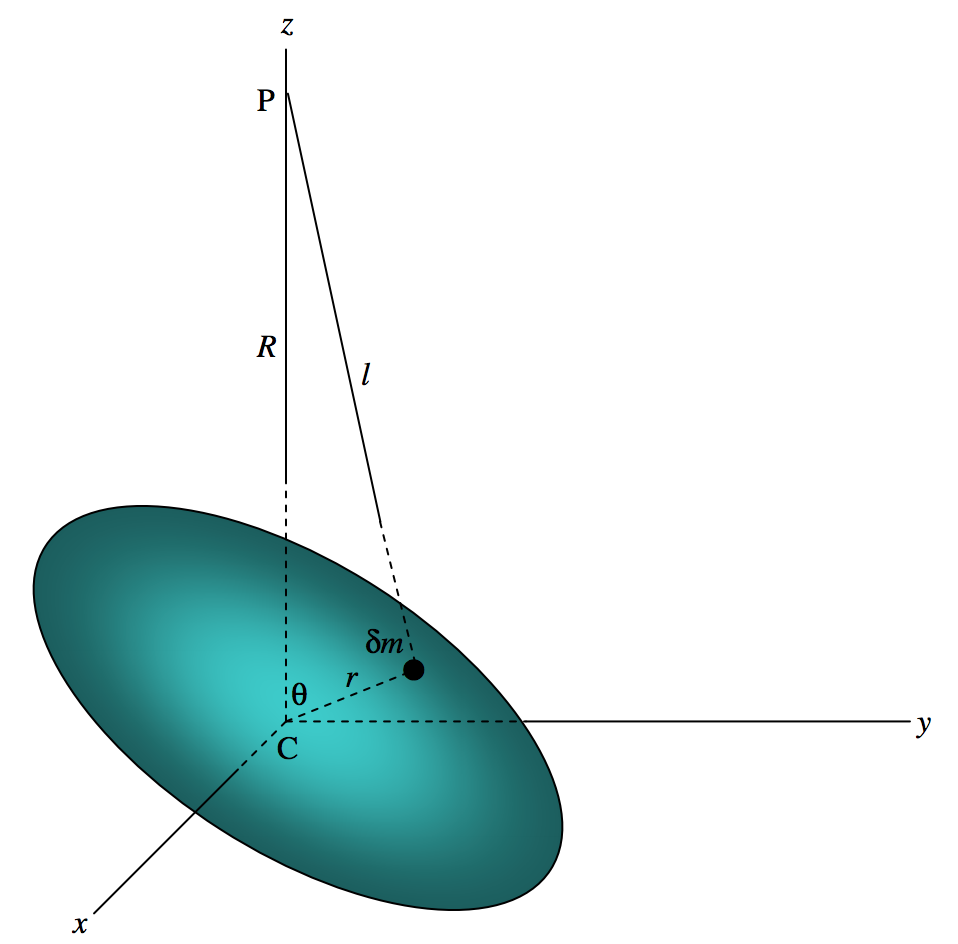
\(\text{FIGURE V.26}\)

The potential at \(\text{P}\) is

\[ψ = -G \int \frac{dm}{l} = -G \int \frac{ρdτ}{l}. \label{5.12.1} \tag{5.12.1}\]

But \(l^2 = R^2 + r^2 - 2Rr \cos 2 θ\),

so \[ψ = -G \left[ \frac{1}{R} \int ρ dτ + \frac{1}{R^2} \int ρ r \cos θ d τ + \frac{1}{R^3} \int ρ r^2 P_2 (\cos θ) dτ + \frac{1}{R^4} \int ρ r^3 P_3 (\cos θ) d τ ... \right]. \label{5.12.2} \tag{5.12.2}\]

The integral is to be taken over the entire body, so that \(∫ ρdτ = M\), where \(M\) is the mass of the body. Also \(∫ ρr \cos θd τ = \int z dm\), which is zero, since \(\text{C}\) is the centre of mass. The third term is

\[\frac{1}{2R^3} \int ρ r^2 (3 \cos^2 θ - 1) dτ = \frac{1}{2R^3} \int ρ r^2 (2-3\sin^2 θ ) dτ . \label{5.12.3} \tag{5.12.3}\]

Now

\[\int 2 ρ r^2 d τ = \int 2r^2 d m = \int \left[ (y^2 + z^2) + (z^2 + x^2) + (x^2 + y^2) \right] dm = A + B + C\]

where \(A\), \(B\) and \(C\) are the second moments of inertia with respect to the axes \(\text{C}x\), \(\text{C}y\), \(\text{C}z\) respectively. But \(A + B + C\) is invariant with respect to rotation of axes, so it is also equal to \(A_0 + B_0 + C_0\), where \(A_0, \ B_0, \ C_0\) are the principal moments of inertia.

Lastly, \(\int ρ r^2 \sin^2 θ dτ\) is equal to \(C\), the moment of inertia with respect to the axis \(\text{C}z\).

Thus, if \(R\) is sufficiently larger than \(r\) so that we can neglect terms of order \((r/R)^3\) and higher, we obtain

\[ψ = - \frac{GM (2MR^2 + A_0 + B_0 + C_0 -3C)}{2R^3}. \label{5.12.4} \tag{5.12.4}\]

In the special case of an oblate symmetric top, in which \(A_0 = B_0 < C_0\), and the line \(\text{CP}\) makes an angle \(γ\) with the principal axis, we have

\[C = A_0 + (C_0 - A_0) \cos^2 γ = A_0 + (C_0 - A_0) Z^2/R^2, \label{5.12.5} \tag{5.12.5}\]

so that \[ψ = -\frac{G}{R} \left[ M + \frac{C_0 - A_0}{2R^2} \left( 1 - \frac{3Z^2}{R^2} \right) \right]. \label{5.12.6} \tag{5.12.6}\]

Now consider a uniform oblate spheroid of polar and equatorial diameters \(2c\) and \(2a\) respectively. It is easy to show that

\[C_0 = \frac{2}{5} Ma^2. \label{5.12.7} \tag{5.12.7}\]

Exercise \(\PageIndex{1}\)

Confirm Equation \ref{5.12.7}.

It is slightly less easy to show (Exercise: Show it.) that

\[A_0 = \frac{1}{5} M \left( a^2 + c^2 \right) . \label{5.12.8} \tag{5.12.8}\]

For a symmetric top, the integrals of the odd polynomials of Equation \(\ref{5.12.2}\) are zero, and the potential is generally written in the form

\[ψ = - \frac{GM}{R} \left[ 1 + \left( \frac{a}{R} \right)^2 J_2 P_2 (\cos γ) + \left( \frac{a}{R} \right) J_4 P_4 (\cos γ) ... \right] \label{5.12.9} \tag{5.12.9}\]

Here \(γ\) is the angle between \(\text{CP}\) and the principal axis. For a uniform oblate spheroid, \(J_2 = \frac{C_0 - A_0}{Mc^2}\). This result will be useful in a later chapter when we discuss precession.