3.2: Mathematical Definition of Dipole Moment

In the introductory section 3.1 we gave a physical definition of dipole moment. I am now about to give a mathematical definition.

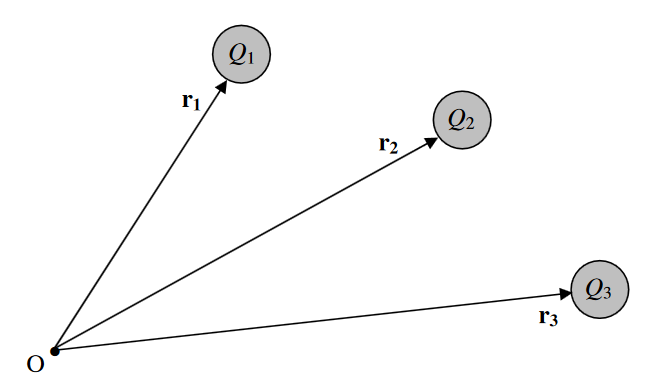
$\text{FIGURE III.2}$

Consider a set of charges $Q_1, Q_2, Q_3 ...$ whose position vectors with respect to a point $O$ are $\textbf{r}_1$, $\textbf{r}_2$, $\textbf{r}_3$... with respect to some point O. The vector sum

$$\textbf{p} = \sum_i Q_i \textbf{r}_i$$

is the dipole moment of the system of charges with respect to the point O. You can see immediately that the SI unit has to be C m.

Convince yourself that if the system as a whole is electrically neutral, so that there is as much positive charge as negative charge, the dipole moment so defined is independent of the position of the point O. One can then talk of “the dipole moment of the system” without adding the rider “with respect to the point O”.

the physical and the mathematical – are equivalent.

While thinking about these two, also convince yourself (from mathematics or from physics) that the moment of a simple dipole consisting of two charges, $+Q$ and $-Q$ separated by a distance $l$ is $Ql$. We have already noted that C m is an acceptable SI unit for dipole moment.