4.1: Bound States
- Page ID
- 14772
One of the simplest potentials to study the properties of is the so-called square well potential (Figure \(\PageIndex{1}\)),
\[ V(x) = \left\{\begin{aligned}
&0 && | x | > a \\
&-V_0 && | x | < a
\end{aligned}
\right. \label{4.1}\]
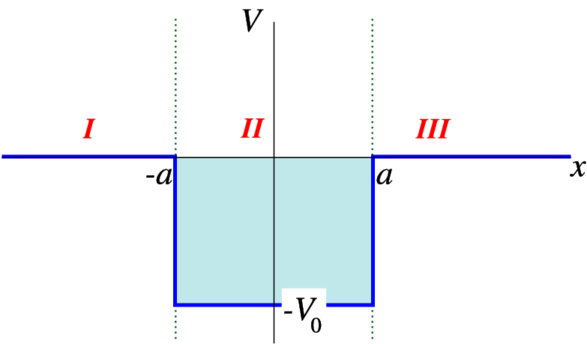
Figure \(\PageIndex{1}\): The square well potential
We define three areas, from left to right in Figure \(\PageIndex{1}\): I, II and III. In areas I and III we have the Schrödinger equation for a free particle
\[− \dfrac{ℏ^2}{2 m} \dfrac{d^2}{d x^ 2} ψ ( x ) = E ψ ( x ) \label{4.2}\]
whereas in area II we have the equation
\[− \dfrac{ℏ^2}{2 m} \dfrac{d^2}{d x^ 2} ψ ( x ) = ( E + V_0 ) ψ ( x ) \label{4.3}\]
In this class we shall quite often encounter the ordinary differential equations
\[ \dfrac{d^2}{d x^2} f( x)=−α^2 f( x) \label{4.4}\]
which has as solution
\[\begin{align} f( x) &= A_1 \cos (α x)+ B_1 \sin (α x) \\[5pt] &= C_1 e^{iα x} + D_1 e^{− iα x}, \label{4.5} \end{align}\]
and
\[ \dfrac{d^2}{d x^ 2} g( x)=+α^2 g( x) \label{4.6}\]
which has as solution
\[\begin{align} g( x) &= A_2 \cosh (α x)+ B_2 \sinh (α x) \\[5pt] &= C_2 e^{α x} + D_2 e^{−α x}. \label{4.7} \end{align}\]
Case 1: E> 0
Let us first look at \(E> 0\). In that case the equation in regions I and III can be written as
\[\dfrac{d^2}{d x^2} ψ ( x ) = − \dfrac{2 m}{ℏ^2} E ψ ( x ) = − k^2 ψ (x), \label{4.8}\]
where
\[k = \sqrt{ \dfrac{2 m}{ℏ^2}} E \label{4.9}\]
The solution to this equation is a sum of sines and cosines of \(k x\), which cannot be normalized: Write
\[ψ_{III}( x)= A \cos ( k x)+ B \sin ( k x)\]
where (\(A\) and \(B\) can be complex) and calculate the part of the norm originating in region III,
\[ \begin{align} \int_a^{∞} |ψ(x)|^2 dx &= \int_a^∞ |A|^2 \cos^2 kx + |B|^2 \sin^2 k x + 2 ℜ ( A B^∗ ) \sin (kx) \cos (kx) dx \\[5pt] &= \lim_{N → ∞} N \int_a^{2 π ∕ k} | A |^2 \cos^2 (kx) + | B |^2 \sin^2 (kx) \\[5pt] &= \lim_{N → ∞} N \left( \dfrac{| A |^ 2}{ 2} + \dfrac{| B |^2}{2} \right ) = ∞ . \label{4.10} \end{align} \]
We also find that the energy cannot be less than \(−V_0 \), since we cannot construct a solution for that value of the energy. We thus restrict ourselves to \(− V_0< E< 0\). We write
\[E = − \dfrac{ ℏ^2}{ k^2 2 m} \]
and
\[E + V_0 = \dfrac{ℏ^2κ^2}{2 m} . \label{4.11}\]
The solutions in the areas I and III are of the form (\(i=1,\,3\))
\[ψ ( x ) = A_i e^{k x} + B_i e^{− k x}. \label{4.12}\]
In region II we have the oscillatory solution
\[ψ ( x ) = A_2 \cos ( κ x ) + B_2 \sin ( κ x ). \label{4.13}\]
Now we have to impose the conditions on the wave functions we have discussed before, continuity of \(ψ\) and its derivatives. Actually we also have to impose normalisability, which means that \(B_1= A_3= 0\) (exponentially growing functions can not be normalized). As we shall see we only have solutions at certain energies. Continuity implies that
\[ \begin{align} A_1 e^{ − k a} + B_1 e^{ k a} &= A_2 \cos ( κ a ) − B_2 \sin ( κ a ) \label{4.14A} \\[5pt] A_3 e^{k a} + B_3 e^{− k a} &= A_2 \cos ( κ a ) + B_2 \sin ( κ a ) \label{4.14B} \\[5pt] k A_1 e^{k a} − k B_1 e^{k a} &= κ A_2 \sin ( κ a ) + κ B_2 \cos ( κ a ) \label{4.14C} \\[5pt] k A_3 e^{ k a} − k B_3 e^{− k a} &= − κ A_2 \sin ( κ a ) + κ B_2 \cos ( κ a ) \label{4.14D} \end{align}\]
Tactical Approach
We wish to find a relation between \(k\) and \(κ\) (why?), removing as maby of the constants \(A\) and B. The trick is to first find an equation that only contains \(A_2\) and \(B_2\). To this end we take the ratio of Equations \ref{4.14A} and \ref{4.14C} and then the ratio of Equations \ref{4.14B} and \ref{4.14D}:
\[\begin{align} k &= \dfrac{κ [ A_2 \sin ( κ a ) + B_2 \cos ( κ a ) ]}{ A_2 \cos ( κ a ) − B_2 \sin ( κ a )} \label{4.15A} \\[5pt] k &= \dfrac{κ [ A_2 \sin ( κ a ) − B_2 \cos ( κ a ) ]}{ A_2 \cos ( κ a ) + B_2 \sin ( κ a )} \label{4.15B} \end{align}\]
We can combine Equations \ref{4.15A} and \ref4.15B} to a single one by equating the right-hand sides. After deleting the common factor \(κ\), and multiplying with the denominators we find
\[[ A_2 \cos ( κ a ) + B_2 \sin ( κ a ) ] [ A_2 \sin ( κ a ) − B_2 \cos ( κ a ) ] = [ A_2 \sin ( κ a ) + B_2 \cos ( κ a ) ] [ A_2 \cos ( κ a ) − B_2 \sin ( κ a ) ] , \label{4.16}\]
which simplifies to
\[A_2 B_2 = 0 \label{4.17}\]
We thus have two families of solutions, those characterized by \(B_2= 0\) and those that have \(A_2= 0\).