6.2: Potential step

Last updated
Save as PDF

Page ID: 14782

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Consider a potential step

\[ V(x) = \left\{\begin{aligned}
& V_0 && x < a \\
& V_1 && x > a
\end{aligned}
\right. \label{6.3}\]

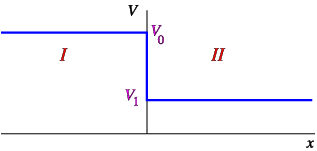
Figure \(\PageIndex{1}\): The step potential discussed in the text

Let me define

\[\begin{align} k_0 &= \sqrt{ \dfrac{2 m}{ ℏ^ 2} ( E − V_0 )} , \label{6.4} \\[5pt] k_1 &= \sqrt{ \dfrac{2 m}{ℏ^ 2} ( E − V_1 )} . \label{6.5} \end{align} \]

I assume a beam of particles comes in from the left,

\[ϕ(x) = A_0 e^{ i k_0 x} \, \, \text{for } x < 0 . \label{6.6}\]

At the potential step the particles either get reﬂected back to region I, or are transmitted to region II. There can thus only be a wave moving to the right in region II, but in region I we have both the incoming and a reﬂected wave,

\[\begin{align} ϕ_I (x) &= A 0 e^{ i k_0 x} + B_0 e^{ − i k_0 x} , \label{6.7} \\[5pt] ϕ_{II} (x) &= A_1 e^{ i k_1 x} . \label{6.8} \end{align} \]

We define a transmission (\(T\)) and reﬂection (\(R\)) coefficient as the ratio of currents between reﬂected or transmitted wave and the incoming wave, where we have canceled a common factor

\[\begin{align} R &= \dfrac{| B_0 |^2}{ | A_0 |^2} \\[5pt] T &= \dfrac{k_1 | A_1 |^2 }{ k_0 | A_0 |^2} . \label{6.9} \end{align} \]

Even though we have given up normalisability, we still have the two continuity conditions. At \(x= 0\) these imply, using continuity of \(ϕ\) and \(\dfrac{d}{dx}ϕ\),

\[\begin{align} A_0 + B_0 &= A_1 , \label{6.10} \\[5pt] i k_0 ( A_0 − B_0 ) &= i k_1 A_1 . \label{6.11} \end{align} \]

We thus find

\[\begin{align} A_1 &= \dfrac{2 k_0}{ k_0 + k_1 A_0} \label{6.12} \\[5pt] B_0 &= \dfrac{k_0 − k_1 }{k_0 + k_1 A_0} , \label{6.13} \end{align} \]

and the reﬂection and transmission coefficients can thus be expressed as

\[\begin{align} R &= \left( \dfrac{k_0 − k_1}{k_0 + k_1} \right)^2 , \label{6.14} \\[5pt] T &= \dfrac{ 4 k_1 k_0}{ ( k_0 + k_1 )^2} . \label{6.15} \end{align} \]

Notice that \(R+ T= 1\)!

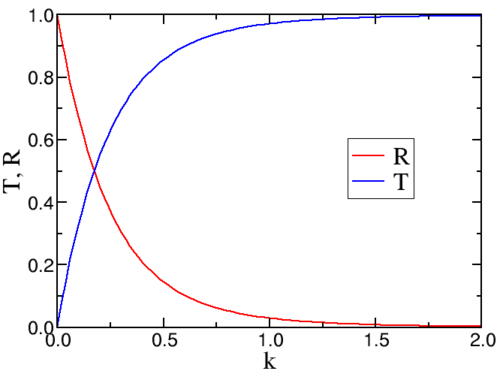
Figure \(\PageIndex{2}\): The transmission and reﬂection coefficients for a square barrier.

In Figure \(\PageIndex{2}\) we have plotted the behaviour of the transmission and reﬂection of a beam of Hydrogen atoms impinging on a barrier of height 2 meV.