17.E: Sound (Exercises)
( \newcommand{\kernel}{\mathrm{null}\,}\)
Conceptual Questions
17.1 Sound Waves
- What is the difference between sound and hearing?
- You will learn that light is an electromagnetic wave that can travel through a vacuum. Can sound waves travel through a vacuum?
- Sound waves can be modeled as a change in pressure. Why is the change in pressure used and not the actual pressure?
17.2 Speed of Sound
- How do sound vibrations of atoms differ from thermal motion?
- When sound passes from one medium to another where its propagation speed is different, does its frequency or wavelength change? Explain your answer briefly.
- A popular party trick is to inhale helium and speak in a high-frequency, funny voice. Explain this phenomenon.
- You may have used a sonic range finder in lab to measure the distance of an object using a clicking sound from a sound transducer. What is the principle used in this device?
- The sonic range finder discussed in the preceding question often needs to be calibrated. During the calibration, the software asks for the room temperature. Why do you suppose the room temperature is required?
17.3 Sound Intensity
- Six members of a synchronized swim team wear earplugs to protect themselves against water pressure at depths, but they can still hear the music and perform the combinations in the water perfectly. One day, they were asked to leave the pool so the dive team could practice a few dives, and they tried to practice on a mat, but seemed to have a lot more difficulty. Why might this be?
- A community is concerned about a plan to bring train service to their downtown from the town’s outskirts. The current sound intensity level, even though the rail yard is blocks away, is 70 dB downtown. The mayor assures the public that there will be a difference of only 30 dB in sound in the downtown area. Should the townspeople be concerned? Why?
17.4 Normal Modes of a Standing Sound Wave
- You are given two wind instruments of identical length. One is open at both ends, whereas the other is closed at one end. Which is able to produce the lowest frequency?
- What is the difference between an overtone and a harmonic? Are all harmonics overtones? Are all overtones harmonics?
- Two identical columns, open at both ends, are in separate rooms. In room A, the temperature is T = 20 °C and in room B, the temperature is T = 25 °C. A speaker is attached to the end of each tube, causing the tubes to resonate at the fundamental frequency. Is the frequency the same for both tubes? Which has the higher frequency?
17.5 Sources of Musical Sound
- 14. How does an unamplified guitar produce sounds so much more intense than those of a plucked string held taut by a simple stick?
- Consider three pipes of the same length (L). Pipe A is open at both ends, pipe B is closed at both ends, and pipe C has one open end and one closed end. If the velocity of sound is the same in each of the three tubes, in which of the tubes could the lowest fundamental frequency be produced? In which of the tubes could the highest fundamental frequency be produced?
- Pipe A has a length L and is open at both ends. Pipe B has a length L2 and has one open end and one closed end. Assume the speed of sound to be the same in both tubes. Which of the harmonics in each tube would be equal?
- A string is tied between two lab posts a distance L apart. The tension in the string and the linear mass density is such that the speed of a wave on the string is v = 343 m/s. A tube with symmetric boundary conditions has a length L and the speed of sound in the tube is v = 343 m/s. What could be said about the frequencies of the harmonics in the string and the tube? What if the velocity in the string were v = 686 m/s?
17.6 Beats
- Two speakers are attached to variable-frequency signal generator. Speaker A produces a constant-frequency sound wave of 1.00 kHz, and speaker B produces a tone of 1.10 kHz. The beat frequency is 0.10 kHz. If the frequency of each speaker is doubled, what is the beat frequency produced?
- The label has been scratched off a tuning fork and you need to know its frequency. From its size, you suspect that it is somewhere around 250 Hz. You find a 250-Hz tuning fork and a 270-Hz tuning fork. When you strike the 250-Hz fork and the fork of unknown frequency, a beat frequency of 5 Hz is produced. When you strike the unknown with the 270-Hz fork, the beat frequency is 15 Hz. What is the unknown frequency? Could you have deduced the frequency using just the 250-Hz fork?
- Referring to the preceding question, if you had only the 250-Hz fork, could you come up with a solution to the problem of finding the unknown frequency? 21. A “showy” custom-built car has two brass horns that are supposed to produce the same frequency but actually emit 263.8 and 264.5 Hz. What beat frequency is produced?
17.7 The Doppler Effect
- Is the Doppler shift real or just a sensory illusion?
- Three stationary observers observe the Doppler shift from a source moving at a constant velocity. The observers are stationed as shown below. Which observer will observe the highest frequency? Which observer will observe the lowest frequency? What can be said about the frequency observed by observer 3?
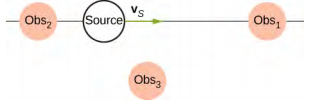
- Shown below is a stationary source and moving observers. Describe the frequencies observed by the observers for this configuration
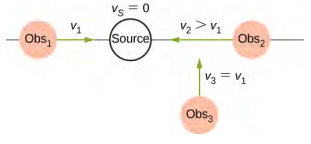
- Prior to 1980, conventional radar was used by weather forecasters. In the 1960s, weather forecasters began to experiment with Doppler radar. What do you think is the advantage of using Doppler radar?
17.8 Shock Waves
- What is the difference between a sonic boom and a shock wave?
- Due to efficiency considerations related to its bow wake, the supersonic transport aircraft must maintain a cruising speed that is a constant ratio to the speed of sound (a constant Mach number). If the aircraft flies from warm air into colder air, should it increase or decrease its speed? Explain your answer.
- When you hear a sonic boom, you often cannot see the plane that made it. Why is that?
Problems
- Consider a sound wave modeled with the equation s(x, t) = 4.00 nm cos(3.66 m−1 x − 1256 s−1 t). What is the maximum displacement, the wavelength, the frequency, and the speed of the sound wave?
- Consider a sound wave moving through the air modeled with the equation s(x, t) = 6.00 nm cos(54.93 m−1 x − 18.84 x 103 s−1 t). What is the shortest time required for an air molecule to move between 3.00 nm and –3.00 nm?
- Consider a diagnostic ultrasound of frequency 5.00 MHz that is used to examine an irregularity in soft tissue. (a) What is the wavelength in air of such a sound wave if the speed of sound is 343 m/s? (b) If the speed of sound in tissue is 1800 m/s, what is the wavelength of this wave in tissue?
- A sound wave is modeled as ΔP = 1.80 Pa sin(55.41 m−1 x − 18,840 s−1 t). What is the maximum change in pressure, the wavelength, the frequency, and the speed of the sound wave?
- A sound wave is modeled with the wave function ΔP = 1.20 Pa sin(kx − 6.28 x 104 s−1 t) and the sound wave travels in air at a speed of v = 343.00 m/s. (a) What is the wave number of the sound wave? (b) What is the value for ΔP(3.00 m, 20.00 s)?
- The displacement of the air molecules in sound wave is modeled with the wave function s(x, t) = 5.00 nm cos(91.54 m−1 x − 3.14 x 104 s −1 t). (a) What is the wave speed of the sound wave? (b) What is the maximum speed of the air molecules as they oscillate in simple harmonic motion? (c) What is the magnitude of the maximum acceleration of the air molecules as they oscillate in simple harmonic motion?
- A speaker is placed at the opening of a long horizontal tube. The speaker oscillates at a frequency f, creating a sound wave that moves down the tube. The wave moves through the tube at a speed of v = 340.00 m/s. The sound wave is modeled with the wave function s(x, t) = smax cos(kx − ωt + ϕ. At time t = 0.00 s, an air molecule at x = 3.5 m is at the maximum displacement of 7.00 nm. At the same time, another molecule at x = 3.7 m has a displacement of 3.00 nm. What is the frequency at which the speaker is oscillating?
- A 250-Hz tuning fork is struck and begins to vibrate. A sound-level meter is located 34.00 m away. It takes the sound Δt = 0.10 s to reach the meter. The maximum displacement of the tuning fork is 1.00 mm. Write a wave function for the sound.
- A sound wave produced by an ultrasonic transducer, moving in air, is modeled with the wave equation s(x, t) = 4.50 nm cos(9.15 x 104 m −1 x − 2π(5.00 MHz)t). The transducer is to be used in nondestructive testing to test for fractures in steel beams. The speed of sound in the steel beam is v = 5950 m/s. Find the wave function for the sound wave in the steel beam.
- Porpoises emit sound waves that they use for navigation. If the wavelength of the sound wave emitted is 4.5 cm, and the speed of sound in the water is v = 1530 m/s, what is the period of the sound?
- Bats use sound waves to catch insects. Bats can detect frequencies up to 100 kHz. If the sound waves travel through air at a speed of v = 343 m/s, what is the wavelength of the sound waves?
- A bat sends of a sound wave 100 kHz and the sound waves travel through air at a speed of v = 343 m/s. (a) If the maximum pressure difference is 1.30 Pa, what is a wave function that would model the sound wave, assuming the wave is sinusoidal? (Assume the phase shift is zero.) (b) What are the period and wavelength of the sound wave?
- Consider the graph shown below of a compression wave. Shown are snapshots of the wave function for t = 0.000 s (blue) and t = 0.005 s (orange). What are the wavelength, maximum displacement, velocity, and period of the compression wave?
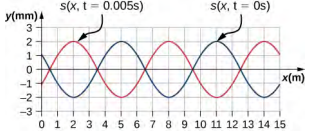
- Consider the graph in the preceding problem of a compression wave. Shown are snapshots of the wave function for t = 0.000 s (blue) and t = 0.005 s (orange). Given that the displacement of the molecule at time t = 0.00 s and position x = 0.00 m is s(0.00 m, 0.00 s) = 1.08 mm, derive a wave function to model the compression wave.
- A guitar string oscillates at a frequency of 100 Hz and produces a sound wave. (a) What do you think the frequency of the sound wave is that the vibrating string produces? (b) If the speed of the sound wave is v = 343 m/s, what is the wavelength of the sound wave?
17.2 Speed of Sound
- When poked by a spear, an operatic soprano lets out a 1200-Hz shriek. What is its wavelength if the speed of sound is 345 m/s?
- What frequency sound has a 0.10-m wavelength when the speed of sound is 340 m/s?
- Calculate the speed of sound on a day when a 1500-Hz frequency has a wavelength of 0.221 m.
- (a) What is the speed of sound in a medium where a 100-kHz frequency produces a 5.96-cm wavelength? (b) Which substance in Table 17.1 is this likely to be?
- Show that the speed of sound in 20.0 °C air is 343 m/s, as claimed in the text.
- Air temperature in the Sahara Desert can reach 56.0 °C (about 134 °F). What is the speed of sound in air at that temperature?
- Dolphins make sounds in air and water. What is the ratio of the wavelength of a sound in air to its wavelength in seawater? Assume air temperature is 20.0 °C.
- A sonar echo returns to a submarine 1.20 s after being emitted. What is the distance to the object creating the echo? (Assume that the submarine is in the ocean, not in fresh water.)
- (a) If a submarine’s sonar can measure echo times with a precision of 0.0100 s, what is the smallest difference in distances it can detect? (Assume that the submarine is in the ocean, not in fresh water.) (b) Discuss the limits this time resolution imposes on the ability of the sonar system to detect the size and shape of the object creating the echo.
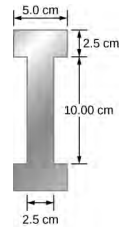
- Ultrasonic sound waves are often used in methods of nondestructive testing. For example, this method can be used to find structural faults in a steel I-beams used in building. Consider a 10.00 meter long, steel I-beam with a cross-section shown below. The weight of the I-beam is 3846.50 N. What would be the speed of sound through in the I-beam? (Ysteel = 200 GPa, βsteel = 159 GPa).
- A physicist at a fireworks display times the lag between seeing an explosion and hearing its sound, and finds it to be 0.400 s. (a) How far away is the explosion if air temperature is 24.0 °C and if you neglect the time taken for light to reach the physicist? (b) Calculate the distance to the explosion taking the speed of light into account. Note that this distance is negligibly greater.
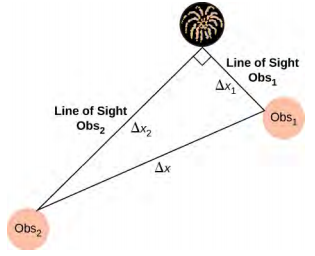
- During a 4th of July celebration, an M80 firework explodes on the ground, producing a bright flash and a loud bang. The air temperature of the night air is TF = 90.00 °F. Two observers see the flash and hear the bang. The first observer notes the time between the flash and the bang as 1.00 second. The second observer notes the difference as 3.00 seconds. The line of sight between the two observers meet at a right angle as shown below. What is the distance Δx between the two observers?
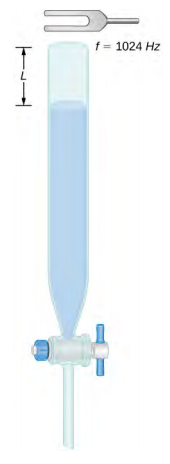
- The density of a sample of water is ρ = 998.00 kg/m3 and the bulk modulus is β = 2.15 GPa. What is the speed of sound through the sample?
- Suppose a bat uses sound echoes to locate its insect prey, 3.00 m away. (See Figure 17.6.) (a) Calculate the echo times for temperatures of 5.00 °C and 35.0 °C. (b) What percent uncertainty does this cause for the bat in locating the insect? (c) Discuss the significance of this uncertainty and whether it could cause difficulties for the bat. (In practice, the bat continues to use sound as it closes in, eliminating most of any difficulties imposed by this and other effects, such as motion of the prey.)
17.3 Sound Intensity
- What is the intensity in watts per meter squared of a 85.0-dB sound?
- The warning tag on a lawn mower states that it produces noise at a level of 91.0 dB. What is this in watts per meter squared?
- A sound wave traveling in air has a pressure amplitude of 0.5 Pa. What is the intensity of the wave?
- What intensity level does the sound in the preceding problem correspond to?
- What sound intensity level in dB is produced by earphones that create an intensity of 4.00 x 10−2 W/m2?
- What is the decibel level of a sound that is twice as intense as a 90.0-dB sound? (b) What is the decibel level of a sound that is one-fifth as intense as a 90.0-dB sound?
- What is the intensity of a sound that has a level 7.00 dB lower than a 4.00 x 10−9 W/m2 sound? (b) What is the intensity of a sound that is 3.00 dB higher than a 4.00 x 10−9 -W/m2 sound?
- People with good hearing can perceive sounds as low as −8.00 dB at a frequency of 3000 Hz. What is the intensity of this sound in watts per meter squared?
- If a large housefly 3.0 m away from you makes a noise of 40.0 dB, what is the noise level of 1000 flies at that distance, assuming interference has a negligible effect?
- Ten cars in a circle at a boom box competition produce a 120-dB sound intensity level at the center of the circle. What is the average sound intensity level produced there by each stereo, assuming interference effects can be neglected?
- The amplitude of a sound wave is measured in terms of its maximum gauge pressure. By what factor does the amplitude of a sound wave increase if the sound intensity level goes up by 40.0 dB?
- If a sound intensity level of 0 dB at 1000 Hz corresponds to a maximum gauge pressure (sound amplitude) of 10−9 atm, what is the maximum gauge pressure in a 60-dB sound? What is the maximum gauge pressure in a 120-dB sound?
- An 8-hour exposure to a sound intensity level of 90.0 dB may cause hearing damage. What energy in joules falls on a 0.800-cm-diameter eardrum so exposed?
- Sound is more effectively transmitted into a stethoscope by direct contact rather than through the air, and it is further intensified by being concentrated on the smaller area of the eardrum. It is reasonable to assume that sound is transmitted into a stethoscope 100 times as effectively compared with transmission though the air. What, then, is the gain in decibels produced by a stethoscope that has a sound gathering area of 15.0 cm2, and concentrates the sound onto two eardrums with a total area of 0.900 cm2 with an efficiency of 40.0%?
- Loudspeakers can produce intense sounds with surprisingly small energy input in spite of their low efficiencies. Calculate the power input needed to produce a 90.0-dB sound intensity level for a 12.0-cm-diameter speaker that has an efficiency of 1.00%. (This value is the sound intensity level right at the speaker.)
- The factor of 10-12 in the range of intensities to which the ear can respond, from threshold to that causing damage after brief exposure, is truly remarkable. If you could measure distances over the same range with a single instrument and the smallest distance you could measure was 1 mm, what would the largest be?
- What are the closest frequencies to 500 Hz that an average person can clearly distinguish as being different in frequency from 500 Hz? The sounds are not present simultaneously.
- Can you tell that your roommate turned up the sound on the TV if its average sound intensity level goes from 70 to 73 dB?
- If a woman needs an amplification of 5.0 x 105 times the threshold intensity to enable her to hear at all frequencies, what is her overall hearing loss in dB? Note that smaller amplification is appropriate for more intense sounds to avoid further damage to her hearing from levels above 90 dB.
- A person has a hearing threshold 10 dB above normal at 100 Hz and 50 dB above normal at 4000 Hz. How much more intense must a 100-Hz tone be than a 4000-Hz tone if they are both barely audible to this person?
17.4 Normal Modes of a Standing Sound Wave
- (a) What is the fundamental frequency of a 0.672-mlong tube, open at both ends, on a day when the speed of sound is 344 m/s? (b) What is the frequency of its second harmonic?
- What is the length of a tube that has a fundamental frequency of 176 Hz and a first overtone of 352 Hz if the speed of sound is 343 m/s?
- The ear canal resonates like a tube closed at one end. (See Figure 17.19.) If ear canals range in length from 1.80 to 2.60 cm in an average population, what is the range of fundamental resonant frequencies? Take air temperature to be 37.0 °C, which is the same as body temperature.
- Calculate the first overtone in an ear canal, which resonates like a 2.40-cm-long tube closed at one end, by taking air temperature to be 37.0 °C. Is the ear particularly sensitive to such a frequency? (The resonances of the ear canal are complicated by its nonuniform shape, which we shall ignore.)
- A crude approximation of voice production is to consider the breathing passages and mouth to be a resonating tube closed at one end. (a) What is the fundamental frequency if the tube is 0.240 m long, by taking air temperature to be 37.0 °C ? (b) What would this frequency become if the person replaced the air with helium? Assume the same temperature dependence for helium as for air.
- A 4.0-m-long pipe, open at one end and closed at one end, is in a room where the temperature is T = 22 °C. A speaker capable of producing variable frequencies is placed at the open end and is used to cause the tube to resonate. (a) What is the wavelength and the frequency of the fundamental frequency? (b) What is the frequency and wavelength of the first overtone?
- A 4.0-m-long pipe, open at both ends, is placed in a room where the temperature is T = 25 °C. A speaker capable of producing variable frequencies is placed at the open end and is used to cause the tube to resonate. (a) What are the wavelength and the frequency of the fundamental frequency? (b) What are the frequency and wavelength of the first overtone?
- A nylon guitar string is fixed between two lab posts 2.00 m apart. The string has a linear mass density of μ = 7.20 g/m and is placed under a tension of 160.00 N. The string is placed next to a tube, open at both ends, of length L. The string is plucked and the tube resonates at the n = 3 mode. The speed of sound is 343 m/s. What is the length of the tube?
- A 512-Hz tuning fork is struck and placed next to a tube with a movable piston, creating a tube with a variable length. The piston is slid down the pipe and resonance is reached when the piston is 115.50 cm from the open end. The next resonance is reached when the piston is 82.50 cm from the open end. (a) What is the speed of sound in the tube? (b) How far from the open end will the piston cause the next mode of resonance?
- Students in a physics lab are asked to find the length of an air column in a tube closed at one end that has a fundamental frequency of 256 Hz. They hold the tube vertically and fill it with water to the top, then lower the water while a 256-Hz tuning fork is rung and listen for the first resonance. (a) What is the air temperature if the resonance occurs for a length of 0.336 m? (b) At what length will they observe the second resonance (first overtone)?
17.5 Sources of Musical Sound
- If a wind instrument, such as a tuba, has a fundamental frequency of 32.0 Hz, what are its first three overtones? It is closed at one end. (The overtones of a real tuba are more complex than this example, because it is a tapered tube.)
- What are the first three overtones of a bassoon that has a fundamental frequency of 90.0 Hz? It is open at both ends. (The overtones of a real bassoon are more complex than this example, because its double reed makes it act more like a tube closed at one end.)
- How long must a flute be in order to have a fundamental frequency of 262 Hz (this frequency corresponds to middle C on the evenly tempered chromatic scale) on a day when air temperature is 20.0°C ? It is open at both ends.
- What length should an oboe have to produce a fundamental frequency of 110 Hz on a day when the speed of sound is 343 m/s? It is open at both ends.
- (a) Find the length of an organ pipe closed at one end that produces a fundamental frequency of 256 Hz when air temperature is 18.0 °C. (b) What is its fundamental frequency at 25.0 °C?
- An organ pipe (L = 3.00 m) is closed at both ends. Compute the wavelengths and frequencies of the first three modes of resonance. Assume the speed of sound is v = 343.00 m/s.
- An organ pipe (L = 3.00 m) is closed at one end. Compute the wavelengths and frequencies of the first three modes of resonance. Assume the speed of sound is v = 343.00 m/s.
- A sound wave of a frequency of 2.00 kHz is produced by a string oscillating in the n = 6 mode. The linear mass density of the string is μ = 0.0065 kg/m and the length of the string is 1.50 m. What is the tension in the string?
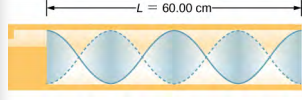
- Consider the sound created by resonating the tube shown below. The air temperature is TC = 30.00 °C. What are the wavelength, wave speed, and frequency of the sound produced?
- A student holds an 80.00-cm lab pole one quarter of the length from the end of the pole. The lab pole is made of aluminum. The student strikes the lab pole with a hammer. The pole resonates at the lowest possible frequency. What is that frequency?
- A string on the violin has a length of 24.00 cm and a mass of 0.860 g. The fundamental frequency of the string is 1.00 kHz. (a) What is the speed of the wave on the string? (b) What is the tension in the string?
- By what fraction will the frequencies produced by a wind instrument change when air temperature goes from 10.0 °C to 30.0 °C? That is, find the ratio of the frequencies at those temperatures.
17.6 Beats
- What beat frequencies are present: (a) If the musical notes A and C are played together (frequencies of 220 and 264 Hz)? (b) If D and F are played together (frequencies of 297 and 352 Hz)? (c) If all four are played together?
- What beat frequencies result if a piano hammer hits three strings that emit frequencies of 127.8, 128.1, and 128.3 Hz?
- A piano tuner hears a beat every 2.00 s when listening to a 264.0-Hz tuning fork and a single piano string. What are the two possible frequencies of the string?
- Two identical strings, of identical lengths of 2.00 m and linear mass density of μ = 0.0065 kg/m, are fixed on both ends. String A is under a tension of 120.00 N. String B is under a tension of 130.00 N. They are each plucked and produce sound at the n = 10 mode. What is the beat frequency?
- A piano tuner uses a 512-Hz tuning fork to tune a piano. He strikes the fork and hits a key on the piano and hears a beat frequency of 5 Hz. He tightens the string of the piano, and repeats the procedure. Once again he hears a beat frequency of 5 Hz. What happened?
- A string with a linear mass density of μ = 0.0062 kg/m is stretched between two posts 1.30 m apart. The tension in the string is 150.00 N. The string oscillates and produces a sound wave. A 1024-Hz tuning fork is struck and the beat frequency between the two sources is 52.83 Hz. What are the possible frequency and wavelength of the wave on the string?
- A car has two horns, one emitting a frequency of 199 Hz and the other emitting a frequency of 203 Hz. What beat frequency do they produce?
- The middle C hammer of a piano hits two strings, producing beats of 1.50 Hz. One of the strings is tuned to 260.00 Hz. What frequencies could the other string have?
- Two tuning forks having frequencies of 460 and 464 Hz are struck simultaneously. What average frequency will you hear, and what will the beat frequency be?
- Twin jet engines on an airplane are producing an average sound frequency of 4100 Hz with a beat frequency of 0.500 Hz. What are their individual frequencies?
- Three adjacent keys on a piano (F, F-sharp, and G) are struck simultaneously, producing frequencies of 349, 370, and 392 Hz. What beat frequencies are produced by this discordant combination?
17.7 The Doppler Effect
- (a) What frequency is received by a person watching an oncoming ambulance moving at 110 km/h and emitting a steady 800-Hz sound from its siren? The speed of sound on this day is 345 m/s. (b) What frequency does she receive after the ambulance has passed?
- (a) At an air show a jet flies directly toward the stands at a speed of 1200 km/h, emitting a frequency of 3500 Hz, on a day when the speed of sound is 342 m/s. What frequency is received by the observers? (b) What frequency do they receive as the plane flies directly away from them?
- What frequency is received by a mouse just before being dispatched by a hawk flying at it at 25.0 m/s and emitting a screech of frequency 3500 Hz? Take the speed of sound to be 331 m/s.
- A spectator at a parade receives an 888-Hz tone from an oncoming trumpeter who is playing an 880-Hz note. At what speed is the musician approaching if the speed of sound is 338 m/s?
- A commuter train blows its 200-Hz horn as it approaches a crossing. The speed of sound is 335 m/s. (a) An observer waiting at the crossing receives a frequency of 208 Hz. What is the speed of the train? (b) What frequency does the observer receive as the train moves away?
- Can you perceive the shift in frequency produced when you pull a tuning fork toward you at 10.0 m/s on a day when the speed of sound is 344 m/s? To answer this question, calculate the factor by which the frequency shifts and see if it is greater than 0.300%.
- Two eagles fly directly toward one another, the first at 15.0 m/s and the second at 20.0 m/s. Both screech, the first one emitting a frequency of 3200 Hz and the second one emitting a frequency of 3800 Hz. What frequencies do they receive if the speed of sound is 330 m/s?
- Student A runs down the hallway of the school at a speed of vo = 5.00 m/s, carrying a ringing 1024.00-Hz tuning fork toward a concrete wall. The speed of sound is v = 343.00 m/s. Student B stands at rest at the wall. (a) What is the frequency heard by student B? (b) What is the beat frequency heard by student A?
- An ambulance with a siren (f = 1.00kHz) blaring is approaching an accident scene. The ambulance is moving at 70.00 mph. A nurse is approaching the scene from the opposite direction, running at vo = 7.00 m/s. What frequency does the nurse observe? Assume the speed of sound is v = 343.00 m/s.
- The frequency of the siren of an ambulance is 900 Hz and is approaching you. You are standing on a corner and observe a frequency of 960 Hz. What is the speed of the ambulance (in mph) if the speed of sound is v = 340.00 m/s?
- What is the minimum speed at which a source must travel toward you for you to be able to hear that its frequency is Doppler shifted? That is, what speed produces a shift of 0.300% on a day when the speed of sound is 331 m/s?
17.8 Shock Waves
- An airplane is flying at Mach 1.50 at an altitude of 7500.00 meters, where the speed of sound is v = 343.00 m/s. How far away from a stationary observer will the plane be when the observer hears the sonic boom?
- A jet flying at an altitude of 8.50 km has a speed of Mach 2.00, where the speed of sound is v = 340.00 m/s. How long after the jet is directly overhead, will a stationary observer hear a sonic boom?
- The shock wave off the front of a fighter jet has an angle of θ = 70.00°. The jet is flying at 1200 km/h. What is the speed of sound?
- A plane is flying at Mach 1.2, and an observer on the ground hears the sonic boom 15.00 seconds after the plane is directly overhead. What is the altitude of the plane? Assume the speed of sound is vw = 343.00 m/s.
- A bullet is fired and moves at a speed of 1342 mph. Assume the speed of sound is v = 340.00 m/s. What is the angle of the shock wave produced?
- A speaker is placed at the opening of a long horizontal tube. The speaker oscillates at a frequency of f, creating a sound wave that moves down the tube. The wave moves through the tube at a speed of v = 340.00 m/s. The sound wave is modeled with the wave function s(x, t) = smax cos(kx − ωt + ϕ). At time t = 0.00 s, an air molecule at x = 2.3 m is at the maximum displacement of 6.34 nm. At the same time, another molecule at x = 2.7 m has a displacement of 2.30 nm. What is the wave function of the sound wave, that is, find the wave number, angular frequency, and the initial phase shift?
- An airplane moves at Mach 1.2 and produces a shock wave. (a) What is the speed of the plane in meters per second? (b) What is the angle that the shock wave moves?
Additional Problems
- A 0.80-m-long tube is opened at both ends. The air temperature is 26 °C. The air in the tube is oscillated using a speaker attached to a signal generator. What are the wavelengths and frequencies of first two modes of sound waves that resonate in the tube?
- A tube filled with water has a valve at the bottom to allow the water to flow out of the tube. As the water is emptied from the tube, the length L of the air column changes. A 1024-Hz tuning fork is placed at the opening of the tube. Water is removed from the tube until the n = 5 mode of a sound wave resonates. What is the length of the air column if the temperature of the air in the room is 18 °C?
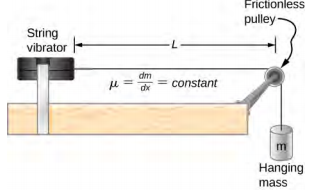
- Consider the following figure. The length of the string between the string vibrator and the pulley is L = 1.00 m. The linear density of the string is μ = 0.006 kg/m. The string vibrator can oscillate at any frequency. The hanging mass is 2.00 kg. (a)What are the wavelength and frequency of n = 6 mode? (b) The string oscillates the air around the string. What is the wavelength of the sound if the speed of the sound is vs = 343.00 m/s?
- Early Doppler shift experiments were conducted using a band playing music on a train. A trumpet player on a moving railroad flatcar plays a 320-Hz note. The sound waves heard by a stationary observer on a train platform hears a frequency of 350 Hz. What is the flatcar’s speed in mph? The temperature of the air is TC = 22 °C.
- Two cars move toward one another, both sounding their horns (fs = 800 Hz). Car A is moving at 65 mph and Car B is at 75 mph. What is the beat frequency heard by each driver? The air temperature is TC = 22.00 °C.
- Student A runs after Student B. Student A carries a tuning fork ringing at 1024 Hz, and student B carries a tuning fork ringing at 1000 Hz. Student A is running at a speed of vA = 5.00 m/s and Student B is running at vB = 6.00 m/s. What is the beat frequency heard by each student? The speed of sound is v = 343.00 m/s.
- Suppose that the sound level from a source is 75 dB and then drops to 52 dB, with a frequency of 600 Hz. Determine the (a) initial and (b) final sound intensities and the (c) initial and (d) final sound wave amplitudes. The air temperature is TC = 24.00 °C and the air density is ρ = 1.184 kg/m3.
- The Doppler shift for a Doppler radar is found by f = fR (1+vc1−vc), where fR is the frequency of the radar, f is the frequency observed by the radar, c is the speed of light, and v is the speed of the target. What is the beat frequency observed at the radar, assuming the speed of the target is much slower than the speed of light?
- A stationary observer hears a frequency of 1000.00 Hz as a source approaches and a frequency of 850.00 Hz as a source departs. The source moves at a constant velocity of 75 mph. What is the temperature of the air?
- A flute plays a note with a frequency of 600 Hz. The flute can be modeled as a pipe open at both ends, where the flute player changes the length with his finger positions. What is the length of the tube if this is the fundamental frequency?
Challenge Problems
- Two sound speakers are separated by a distance d, each sounding a frequency f. An observer stands at one speaker and walks in a straight line a distance x, perpendicular to the the two speakers, until he comes to the first maximum intensity of sound. The speed of sound is v. How far is he from the speaker?
- Consider the beats shown below. This is a graph of the gauge pressure versus time for the position x = 0.00 m. The wave moves with a speed of v = 343.00 m/s. (a) How many beats are there per second? (b) How many times does the wave oscillate per second? (c) Write a wave function for the gauge pressure as a function of time.

- Two speakers producing the same frequency of sound are a distance of d apart. Consider an arc along a circle of radius R, centered at the midpoint of the speakers, as shown below. (a) At what angles will there be maxima? (b) At what angle will there be minima?
- A string has a length of 1.5 m, a linear mass density μ = 0.008 kg/m, and a tension of 120 N. If the air temperature is T = 22 °C, what should the length of a pipe open at both ends for it to have the same frequency for the n = 3 mode?
- A string (μ = 0.006 kg/m, L = 1.50 m) is fixed at both ends and is under a tension of 155 N. It oscillates in the n = 10 mode and produces sound. A tuning fork is ringing nearby, producing a beat frequency of 23.76 Hz. (a) What is the frequency of the sound from the string? (b) What is the frequency of the tuning fork if the tuning fork frequency is lower? (c) What should be the tension of the string for the beat frequency to be zero?
- A string has a linear mass density μ, a length L, and a tension of FT, and oscillates in a mode n at a frequency f. Find the ratio of Δff for a small change in tension.
- A string has a linear mass density μ = 0.007 kg/m, a length L = 0.70 m, a tension of FT = 110 N, and oscillates in a mode n = 3. (a) What is the frequency of the oscillations? (b) Use the result in the preceding problem to find the change in the frequency when the tension is increased by 1.00%.
- A speaker powered by a signal generator is used to study resonance in a tube. The signal generator can be adjusted from a frequency of 1000 Hz to 1800 Hz. First, a 0.75-m-long tube, open at both ends, is studied. The temperature in the room is TF = 85.00 °F. (a) Which normal modes of the pipe can be studied? What are the frequencies and wavelengths? Next a cap is place on one end of the 0.75-meter-long pipe. (b) Which normal modes of the pipe can be studied? What are the frequencies and wavelengths?
- A string on the violin has a length of 23.00 cm and a mass of 0.900 grams. The tension in the string 850.00 N. The temperature in the room is TC = 24.00 °C. The string is plucked and oscillates in the n = 9 mode. (a) What is the speed of the wave on the string? (b) What is the wavelength of the sounding wave produced? (c) What is the frequency of the oscillating string? (d) What is the frequency of the sound produced? (e) What is the wavelength of the sound produced?
Contributors and Attributions
Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).


